Elementary Stats Lesson #8
TLDRThis video lesson, part of a probability series, introduces the concept of independent events and the multiplication rule for calculating the probability of multiple independent events occurring together. The instructor emphasizes the importance of distinguishing between independent and mutually exclusive events. Through examples, the lesson demonstrates how to apply the multiplication rule for counting sample spaces and events, as well as the use of permutations and combinations in probability calculations. The session also prepares students for an upcoming test, providing insights into the test format and offering strategies for success.
Takeaways
- 📚 The class is in week four, covering chapter five of the textbook, focusing on part two of probability.
- 🗓️ Next week, week five, will feature the first test, with Group A testing on Tuesday and Group B on Thursday due to pandemic protocols.
- 📝 The instructor will provide information on the test format, formula sheets, and preparation tools via email and the canvas site.
- 🧩 The lesson reviewed basic probability principles, including the rules of addition and complements, setting the stage for discussing independent events.
- 🎯 The concept of independent events was introduced, emphasizing that the occurrence of one event does not affect the probability of another.
- ❌ A clear distinction was made between independent and mutually exclusive (disjoint) events, noting that disjoint events cannot be independent.
- 🔢 The multiplication method for calculating the probability of independent events occurring together was explained, simplifying the process compared to classical methods.
- 🌳 A tree diagram was used to visually justify why the multiplication method works for calculating probabilities in multi-stage experiments.
- 📉 The multiplication rule for independent events was extended to more than two events, demonstrating its application in complex scenarios like survival probabilities.
- 💡 The lesson highlighted the importance of identifying independence in events to apply the multiplication rule effectively in probability calculations.
- 📝 The 'at least one' type of probability problems was addressed, showing how to simplify them by calculating the complement event (none of the event occurring) instead.
Q & A
What is the main topic discussed in this lesson?
-The main topic discussed in this lesson is the continuation of the probability discussion, specifically focusing on chapter five, part two, which includes the multiplication rule for independent events and counting techniques.
What is the basic rule for any probability experiment involving events?
-The basic rule for any probability experiment involving events is that the probability of any event must be between 0 and 1, inclusive, and the sum of all possible simple event probabilities must equal 1.
What is the addition rule for calculating probabilities?
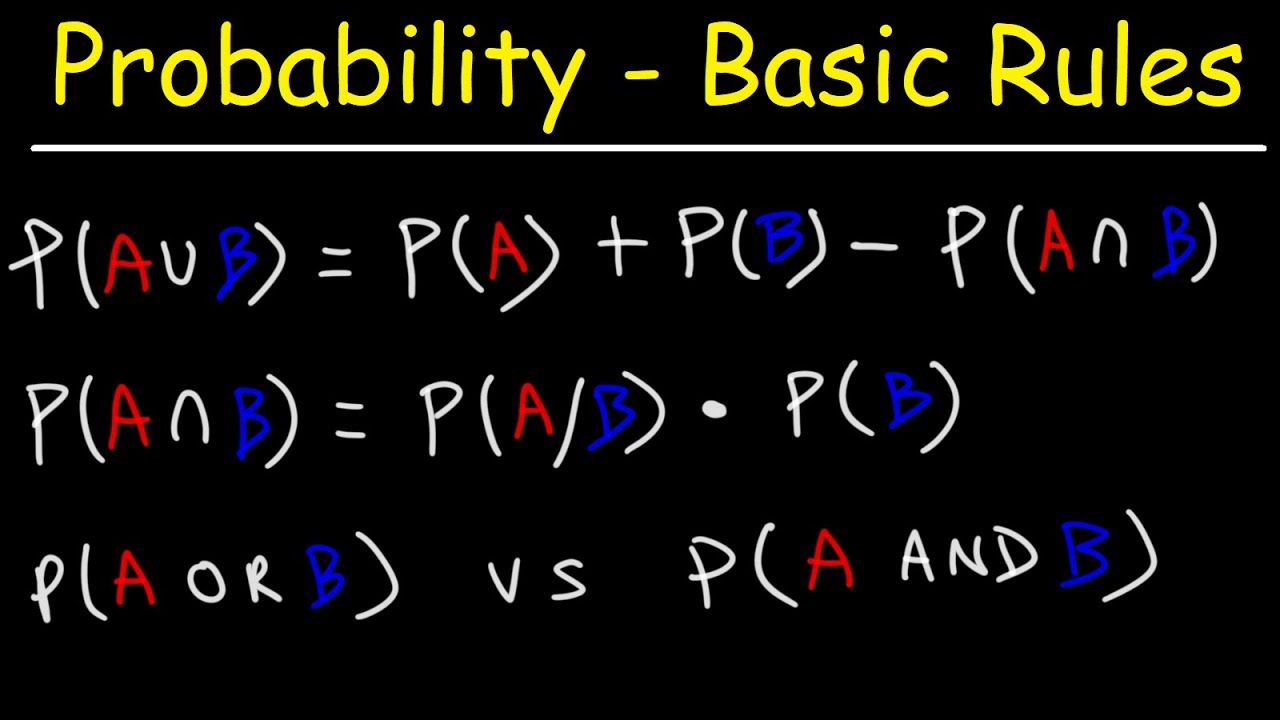
-The addition rule for calculating probabilities states that the probability of either event E or event F occurring is the probability of E plus the probability of F minus the probability of E and F occurring together (the intersection).
What is the rule of complements in probability?
-The rule of complements states that the probability of an event E not happening (E complement) is equal to one minus the probability that E does happen.
What is the definition of independent events in probability?
-Two events E and F are considered independent if the occurrence of one event does not affect the probability of the other event occurring.
What is the multiplication rule for independent events?
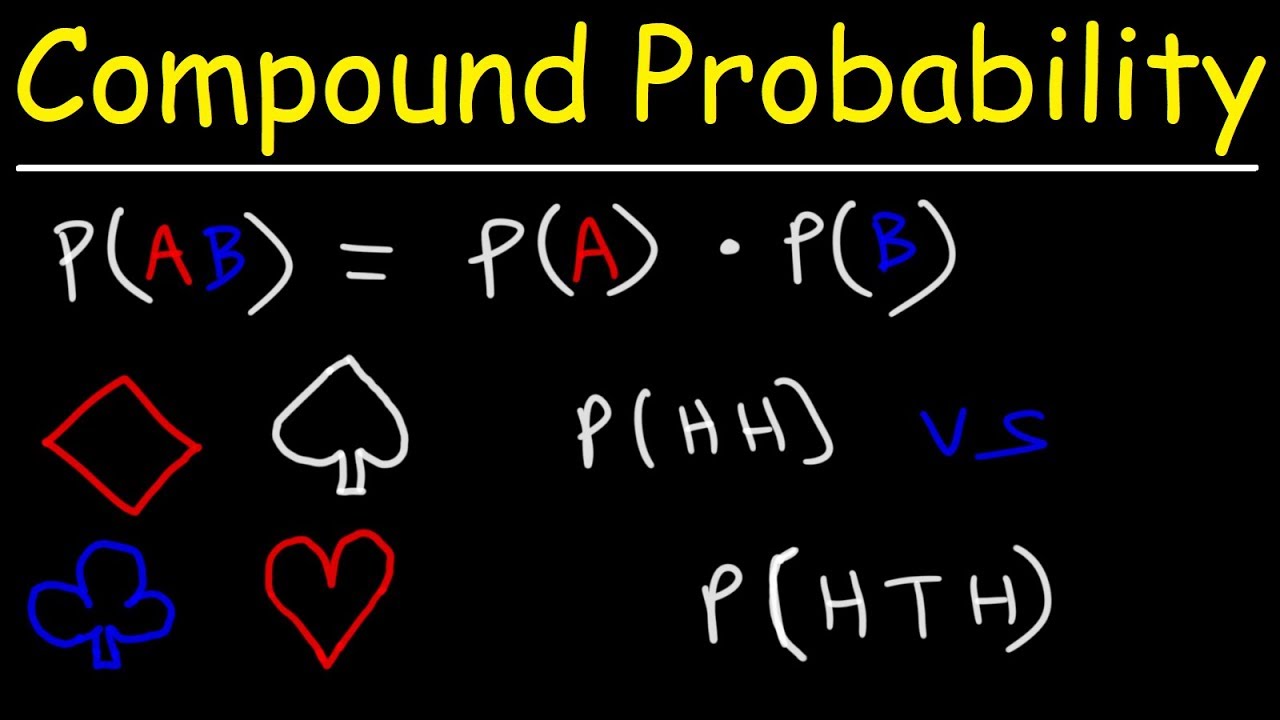
-The multiplication rule for independent events states that if events E and F are independent, the probability of both E and F happening is the product of their individual probabilities (P(E and F) = P(E) * P(F)).
What is the difference between independent events and mutually exclusive events?
-Independent events are those where the occurrence of one does not affect the probability of the other, whereas mutually exclusive events cannot occur at the same time. If two events are mutually exclusive, they are not independent.
What is the multiplication rule for counting and when is it used?
-The multiplication rule for counting is used when an entire process takes place in several independent stages, and it states that the total number of outcomes for the entire process is the product of the number of outcomes for each independent stage.
What is the distinction between permutations and combinations in counting?
-Permutations are ordered selections where the order matters, while combinations are unordered selections where the order does not matter.
How can you determine if you should use permutations or combinations for a counting problem?
-You should use permutations if the order of selection matters in the context of the problem, and combinations if the order does not matter.
What is the role of the calculator in solving permutation and combination problems?
-The calculator, specifically the npr and ncr programs on a TI-84 calculator, can be used to compute the number of permutations and combinations quickly, avoiding the need for manual calculations and reducing the chance of errors.
Outlines
📚 Course Update and Probability Discussion
The instructor welcomes students to a lesson focusing on chapter five of the textbook, specifically part two of the probability discussion. This is week four of the semester, and the class is reminded of the upcoming first test in week five. Group A will take the test on Tuesday, and Group B on Thursday. The instructor will provide information about the test format, formula sheets, and tools to prepare for the test. The lesson recaps basic probability principles, including the rules of probability and the addition rule for calculating the probability of two events, e or f. The concept of the complement rule is also explained, setting the stage for further exploration of probability.
🎯 Understanding Independent Events in Probability
The concept of independent events is introduced, explaining that two events, e and f, are independent if the occurrence of one does not affect the probability of the other. This is contrasted with disjoint events, which cannot occur simultaneously and thus are not independent. The instructor uses the example of flipping a fair coin twice to illustrate independent events, where getting a head on the first toss does not influence the outcome of the second toss. Two methods for calculating the probability of both events happening are discussed: the classical approach, which involves listing all possible outcomes, and the multiplication method, which leverages the independence of the events to simplify calculations.
🔢 Multiplication Rule for Independent Events
The multiplication rule for independent events is established as a fundamental principle for calculating the probability of multiple events occurring together. The rule states that if events e and f are independent, the probability of both happening is the product of their individual probabilities. This rule is extended to more than two events, allowing for the calculation of the probability of a series of independent events by multiplying their individual probabilities. An example involving the probability of two randomly selected 60-year-old females surviving the year is used to demonstrate the application of the multiplication rule.
🧩 Applying the Multiplication Rule to Complex Scenarios
The lesson extends the application of the multiplication rule to more complex scenarios, such as calculating the probability of 25 randomly selected 60-year-old females surviving the year. The rule of complements is introduced as a strategy for simplifying complex probability calculations by considering the complement of the event. An example is provided where the probability of at least one of the 25 females dying during the year is calculated by first determining the probability of all surviving and then subtracting from one. The importance of independence in applying the multiplication rule is emphasized, and a rule of thumb for assuming independence in random sampling is discussed.
📝 Counting Techniques for Large Sample Spaces
The instructor outlines basic techniques for counting large sample spaces and events, focusing on the multiplication rule for counting. This rule allows for the calculation of the size of a sample space when it is not possible to list all outcomes. The rule is applied to an example involving rolling two dice and tossing three coins, demonstrating how to break down the process into independent stages and multiply the number of outcomes at each stage to find the total number of possible outcomes.
🔄 Counting with Replacement and the Multiplication Rule
The concept of counting with replacement is introduced, where once an item is selected, it is replaced, allowing it to be chosen again. This is contrasted with counting without replacement. Examples are given to illustrate how the multiplication rule for counting can be used in different scenarios, such as creating three-letter code words using the first eight letters of the alphabet, with and without the possibility of letter repetition. The importance of understanding whether replacement is allowed is highlighted as it significantly affects the count of possible outcomes.
🛠️ Applying Counting Techniques to Real-World Scenarios
The application of counting techniques is demonstrated in real-world scenarios, such as configuring a computer with various options for processors, monitors, memory sizes, and hard drives. The multiplication rule for counting is used to determine the total number of distinct configurations possible. The principles of counting are further explained with the introduction of permutations and combinations, which are essential for solving more complex counting problems, with examples provided to illustrate their use.
🔄 Permutations and Combinations in Counting
The difference between permutations and combinations is clarified, with permutations referring to ordered selections and combinations to unordered selections. The instructor provides formulas for both types of counts and explains the notation used in these formulas. Examples are given to demonstrate how to calculate the number of ways to select a president, vice president, and secretary from a committee, and how to determine the number of ways to form a three-person subcommittee from a larger group, highlighting the importance of understanding whether the order of selection matters.
🎰 Lottery Odds and Combination Counting
The lesson concludes with an application of combination counting to determine the odds of winning a lottery, specifically the Texas Lotto, where players must choose six numbers out of 54. The combination formula is used to calculate the total number of possible ticket combinations, illustrating the vast number of outcomes and the corresponding low probability of winning the jackpot. This example underscores the importance of combination counting in understanding probabilities in everyday situations.
Mindmap
Keywords
💡Probability
💡Independence
💡Complement Rule
💡Multiplication Rule
💡Addition Rule
💡Sample Space
💡Random Sampling
💡Permutations
💡Combinations
💡Factorial
💡Tree Diagram
Highlights
Introduction to the lesson, emphasizing the continuation of probability discussion from the previous week.
Overview of the week's plan, including finishing chapter five and preparing for the first test in week five.
Announcement of test schedules for Group A and Group B due to pandemic situation.
Introduction of basic probability principles, including the probability of any event being between 0 and 1, and the sum of all simple events probabilities equating to one.
Explanation of the general addition rule for calculating probabilities of combined events.
Discussion of the rule of complements, which states the probability of an event not happening is one minus the probability of it happening.
Introduction of the concept of independent events and the difference between independent and mutually exclusive events.
Development of a rule for calculating the probability of two independent events occurring together.
Illustration of the multiplication method for calculating probabilities of independent events through the example of coin tosses.
Explanation of the multiplication rule for independent events and its application to more than two events.
Application of the multiplication rule to calculate the probability of multiple independent events, such as the survival of 25 individuals over a year.
Introduction of the concept of permutations and combinations in the context of selecting committee members.
Differentiation between ordered selections (permutations) and unordered selections (combinations).
Presentation of formulas and calculator programs for permutations and combinations to simplify counting large sample spaces.
Discussion of the importance of sample size in relation to population size for assuming independence in random sampling.
Introduction of the multiplication rule for counting as a technique for counting large sample spaces without listing all outcomes.
Application of counting techniques to probability calculations, such as the probability of selecting exactly one defective item in a sample.
Conclusion of the lesson with a reminder of the upcoming assignment and exam preparation.
Transcripts
Browse More Related Video

AP Stats Test Quick Review: Probability

Chapter 4 Probability Part 2
Multiplication & Addition Rule - Probability - Mutually Exclusive & Independent Events
Math 119 Chapter 4 part 3
Compound Probability of Independent Events - Coins & 52 Playing Cards

Probability Formulas, Symbols & Notations - Marginal, Joint, & Conditional Probabilities
5.0 / 5 (0 votes)
Thanks for rating: