AP Calculus AB: Lesson 7.5: Shell Method
TLDRThis educational video introduces the shell method for calculating the volume of a solid of revolution, contrasting it with the washer and disk methods. It reviews the washer method, highlighting its limitations with certain functions and the difficulty of solving equations for X or Y. The video demonstrates step-by-step how to set up and solve integrals using the shell method for various functions and rotation axes, emphasizing the importance of correctly identifying the radius and height of cylindrical shells in different scenarios. The summary also encourages practice and critical thinking in choosing the most efficient method for each problem.
Takeaways
- 📚 The video introduces the shell method for finding the volume of a solid of revolution, in addition to the previously discussed disk and washer methods.
- 🔄 It's important to review the washer method before delving into the new technique, as understanding both is crucial for solving various problems.
- 📏 The washer method involves using two separate disk methods and subtracting the results to account for the empty space between the solid and the axis of revolution.
- ⭕️ The formula for the washer method is PI times the integral from A to B of the outer radius squared minus the inner radius squared, times DX.
- 📐 Setting up the washer method involves drawing reference rectangles from the axis of rotation to the shaded region, representing outer and inner radii.
- 🤔 The video demonstrates how to find the point of intersection for the functions to determine the limits of integration in the washer method.
- 🚫 The shell method is preferred when rotating around an axis where the function does not pass the horizontal line test, making the washer method difficult.
- 📉 The shell method is represented by revolving a vertical or horizontal slice around the axis of revolution, creating a cylindrical shell.
- 📐 For the shell method, the volume calculation is 2 times pi times the integral from a to b of the radius times the height times DX.
- 🔢 The video provides step-by-step instructions for setting up and calculating integrals using both the washer and shell methods, emphasizing the importance of correctly identifying the radius and height for each method.
- 💡 The script concludes with a reminder to practice and develop a keen sense for choosing the appropriate method for different problems, as this skill is essential for successfully calculating volumes of solids of revolution.
Q & A
What is the main focus of the video?
-The main focus of the video is to learn a technique for finding the volume of a solid of revolution using the shell method.
What method is reviewed before introducing the shell method?
-The washer method is reviewed before introducing the shell method.
How is the washer method used to find the volume of a solid of revolution?
-The washer method involves using two separate disk methods and subtracting the results to account for the empty space between the region and the axis of revolution. The volume is calculated using the integral of the difference between the squares of the outer and inner radii.
What is the general formula for the washer method?
-The general formula for the washer method is V = π ∫[a to b] (R_outer^2 - R_inner^2) dx.
Why is it difficult to use the washer method for the second example problem?
-It is difficult to use the washer method for the second example problem because x is not a function of y, making it hard to solve for x and leading to complications in setting up the integral.
What is the basic setup for the shell method?
-The basic setup for the shell method involves calculating the lateral surface area of a cylinder and multiplying it by the thickness. The formula is V = 2π ∫[a to b] (radius * height) dx.
In the shell method, how is the radius determined?
-In the shell method, the radius is the horizontal distance from the axis of rotation to the rectangle, which is represented as x in the integral setup.
How is the height of the shell determined in the shell method?
-The height of the shell is the vertical distance from the top function to the bottom function within the region being rotated.
Why might the shell method be preferred over the washer method in certain problems?
-The shell method might be preferred over the washer method when it is difficult to solve the equation for x or y, or when the washer method requires breaking the function into piecewise parts, making the calculation more complex.
What are the key indicators that suggest using the shell method over the washer method?
-Key indicators for using the shell method over the washer method include when the region fails the horizontal line test, making it difficult to solve for x in terms of y, and when the right and left sides of the rectangle touch the same function, complicating the setup for the washer method.
What was the approximate volume of the solid formed by rotating the region y = sine(x) and y = 0 from x = 0 to x = π about the y-axis using the shell method?
-The approximate volume of the solid formed was 19.739 cubic units.
How is the cylindrical shell visualized in the shell method?
-The cylindrical shell is visualized by rotating a vertical rectangle around the y-axis, creating a cylinder with thickness dx, and calculating its lateral surface area.
What is the significance of the reference rectangle in the shell method?
-The reference rectangle helps in setting up the integral by providing a visual guide to determine the radius and height of the cylindrical shell.
How is the integral for the shell method set up for a problem involving the region bounded by y = x - x^2 and y = 0 rotated around the y-axis?
-The integral is set up as V = 2π ∫[0 to 1] x(2x^2 - x^3) dx, where the radius is x and the height is the difference between the functions.
Outlines
📚 Introduction to the Shell Method for Solid of Revolution
This paragraph introduces a new mathematical technique known as the Shell Method for calculating the volume of a solid of revolution, following a review of the Washer Method. The Washer Method involves using two separate disk methods and subtracting the results to account for the empty space within the solid. The paragraph explains the basic setup for the Washer Method, which includes multiplying PI by the integral from a to b of the outer radius squared minus the inner radius squared, times dx. The process involves finding the volume of the larger disc and subtracting the volume of the smaller disc, which represents the empty space. The paragraph also emphasizes the importance of drawing reference rectangles and understanding the interval for the integral when setting up the problem.
🔍 Detailed Explanation of the Washer Method Application
The second paragraph delves deeper into the application of the Washer Method by providing a step-by-step guide on how to find the point of intersection between two functions, f(x) and g(x), using a graphing calculator. It explains the process of setting up the integral for the volume of the solid formed by rotating the region between these two functions around the x-axis. The paragraph illustrates how to calculate the outer and inner radii for the Washer Method and how to perform the integration using a calculator. It concludes with a brief mention of considering alternative methods for more complex problems where the Washer Method might not be the best choice.
🚫 Challenges with the Washer Method for Certain Functions
This paragraph discusses the limitations of using the Washer Method for certain functions, particularly when the function does not pass the horizontal line test, indicating that x is not a function of y. The speaker explains that in such cases, the Washer Method becomes impractical because it requires solving the equation for x, which can be complex. The paragraph uses the example of the function y = sin(x) and explains why it's difficult to apply the Washer Method in this scenario. It also highlights the importance of recognizing when a horizontal slice would not result in a washer shape, suggesting that the Shell Method might be more appropriate for such cases.
🛠 Transition to the Shell Method with a Demonstrative Example
The speaker transitions from discussing the limitations of the Washer Method to introducing the Shell Method with a demonstrative example. They explain how to visualize and calculate the volume of a solid formed by rotating a region around the y-axis using horizontal slices, resulting in cylindrical shells. The paragraph outlines the process of finding the volume of a single shell and then summing these volumes using an integral. It emphasizes the lateral surface area of the cylinder and its relation to the radius and height, which are derived from the function and the axis of rotation.
📐 Setting Up the Shell Method for a Specific Function
In this paragraph, the speaker provides a detailed setup for using the Shell Method with the function y = sin(x) rotated around the y-axis. They explain how to determine the interval for the integral and how to rewrite the function in terms of x to avoid using multivariable calculus. The paragraph includes a step-by-step guide on setting up the integral, performing the integration on a calculator, and interpreting the result to find the volume of the solid. It also emphasizes the importance of drawing a well-labeled reference rectangle and a cylinder to visualize the problem.
🔄 Comparing Shell Method with Washer Method for a Given Region
The paragraph presents a comparison between the Shell Method and the Washer Method for finding the volume of a solid formed by revolving a region bounded by two functions around the y-axis. It discusses the difficulties associated with the Washer Method, such as the challenge of separating the graph into distinct parts and solving the equation for x. The speaker advises using the Shell Method for this particular problem and outlines the steps for setting up the integral, including determining the radius and height of the cylindrical shells.
📉 Shell Method Application with a Complex Function
This paragraph demonstrates the application of the Shell Method to a complex function, y = 2x^2 - x^3, rotated around the y-axis. The speaker explains the process of identifying the interval for the integral and setting up the integral with the correct expressions for the radius and height of the cylindrical shells. They also discuss the importance of drawing a reference rectangle and a cylinder to visualize the problem and to ensure the correct setup for the integral.
📐 Shell Method for a Region Rotated Around a Vertical Line
The paragraph discusses the use of the Shell Method for a region rotated around a vertical line, x = -1, using the function y = sqrt(x). It explains how to determine the interval for the integral and how to calculate the radius of the cylindrical shells, which is the horizontal distance from the axis of rotation to the curve. The speaker also provides a step-by-step guide on setting up the integral and performing the calculation to find the volume of the solid.
🔄 Shell Method Versus Washer Method for a Region Around x = 2
This paragraph explores the use of both the Shell Method and the Washer Method for finding the volume of a solid formed by revolving a region around the line x = 2. The speaker explains why the Washer Method would be difficult to apply due to the complexity of solving the equation for x and suggests using the Shell Method instead. They provide a detailed setup for the Shell Method, including determining the radius and height of the cylindrical shells and setting up the integral.
📊 Comparing Methods for a Region Between Two Functions
The paragraph presents a comparison of the Shell Method and the Washer Method for a region between two functions, y = x and y = x^2, rotated around the y-axis. The speaker demonstrates the setup for both methods, emphasizing the importance of understanding the geometry of the problem and the functions involved. They show that both methods, when applied correctly, yield the same result for the volume of the solid.
🔍 Shell Method for a Region Rotated Around a Horizontal Axis
This paragraph discusses the application of the Shell Method for a region rotated around a horizontal axis, specifically the x-axis. The speaker explains how to set up the integral for the volume of the solid formed by the region under the curve y = sqrt(x) from x = 0 to x = 1. They provide a detailed explanation of determining the radius and height of the cylindrical shells and how to rewrite the expressions in terms of the variable of integration.
📉 Shell and Washer Methods for a Region Between Two Curves
The final paragraph presents a comparison between the Shell Method and the Washer Method for finding the volume of a solid formed by revolving a region between two curves, x = y^3 and y = x, around the line y = -1. The speaker outlines the setup for both methods, including determining the radius and height for the cylindrical shells in the Shell Method and calculating the outer and inner radii for the Washer Method. They emphasize the importance of converting all expressions into terms of the variable of integration and highlight that both methods should yield the same result when applied correctly.
Mindmap
Keywords
💡Solid of Revolution
💡Disk Method
💡Washer Method
💡Shell Method
💡Region R
💡Integral
💡Function Notation
💡Horizontal Line Test
💡Cylindrical Shell
💡Lateral Surface Area
💡Piecewise Function
Highlights
Introduction to a new technique for finding the volume of a solid of revolution, the shell method.
Review of the washer method for calculating the volume of a solid of revolution using disk methods.
Explanation of the washer method's limitation when there is no empty space between the region and the axis of revolution.
Demonstration of setting up the washer method using the functions f(x) and g(x) to find the volume of a solid.
Guide on using a graphing calculator to find the point of intersection of two functions.
Process of setting up the integral for the washer method to calculate the volume of a solid.
Illustration of why the washer method is not suitable for solids formed by rotating around the y-axis with certain functions.
Introduction of the shell method as an alternative to the washer method for specific problems.
Explanation of the shell method's formula and its application in calculating the volume of a solid.
Demonstration of the shell method using the function y = sin(x) rotated around the y-axis.
Comparison between the shapes produced by horizontal and vertical slices when using the shell method.
Visualization of the cylindrical shell and its lateral surface area in the context of the shell method.
Application of the shell method to find the volume of a solid formed by the region bounded by y = 2x^2 - x^3 and y = 0, rotated around the y-axis.
Guide on choosing between the shell and washer methods based on the functions and the axis of rotation.
Practical examples demonstrating the use of both the shell and washer methods to solve for the volume of solids.
Final thoughts on the importance of drawing well-labeled diagrams and choosing the appropriate method for volume calculations.
Transcripts
Browse More Related Video

Calculus 1 Lecture 5.3: Volume of Solids By Cylindrical Shells Method
Disc/Washer Method vs. Shell Method (rotated about different lines)
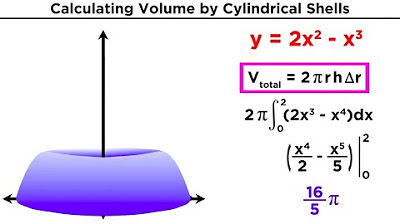
Calculating Volume by Cylindrical Shells

AP Calculus AB: Lesson 7.4: Disk and Washer Method

Calculus: Volumes by Cylindrical Shells (Section 6.3) | Math with Professor V
Volume The Disc Method
5.0 / 5 (0 votes)
Thanks for rating: