Integration into Inverse trigonometric functions using Substitution
TLDRThis video tutorial delves into the process of integrating functions involving inverse trigonometric functions. It introduces three key formulas for integrating expressions involving arcsine, arctangent, and arcsecant. The video proceeds with a series of examples, illustrating how to identify and apply these formulas through u-substitution and completing the square techniques. Each step is methodically explained, allowing viewers to follow along and gain a solid understanding of the integration process for such functions.
Takeaways
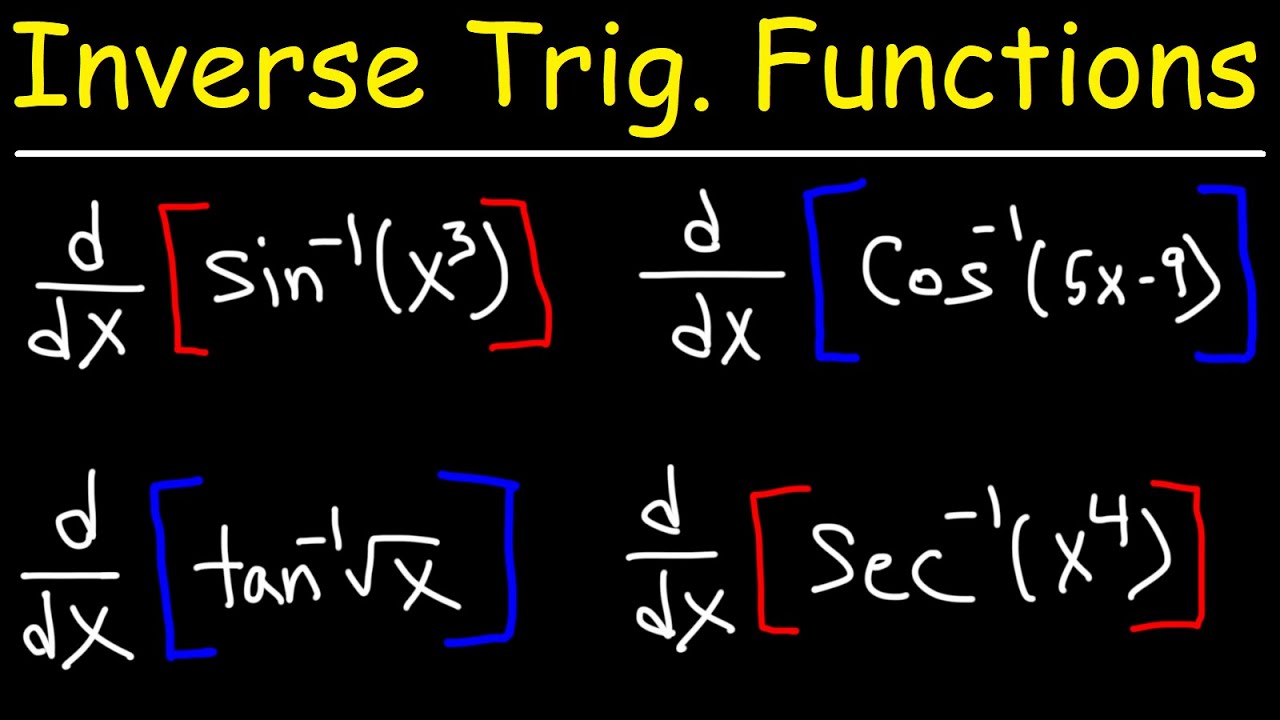
- 📚 Three key formulas for integrating inverse trigonometric functions were discussed: arcsine, arctangent, and arcsecant.
- 🔍 The first formula involves the integral of du/(√(a^2 - u^2)) which equals arcsin(u/a) + C.
- 📐 The second formula is the integral of du/(a^2 + u^2) which equals 1/a * arctan(u/a) + C.
- 🛠️ The third formula presented is the integral of du/(u * √(u^2 - a^2)) which equals 1/a * arcsec(u/a) + C.
- 🧩 The script provides examples of how to apply these formulas by identifying the values of u and a in the given integrals.
- 🌟 The concept of completing the square is used in several examples to simplify the integrals before applying the formulas.
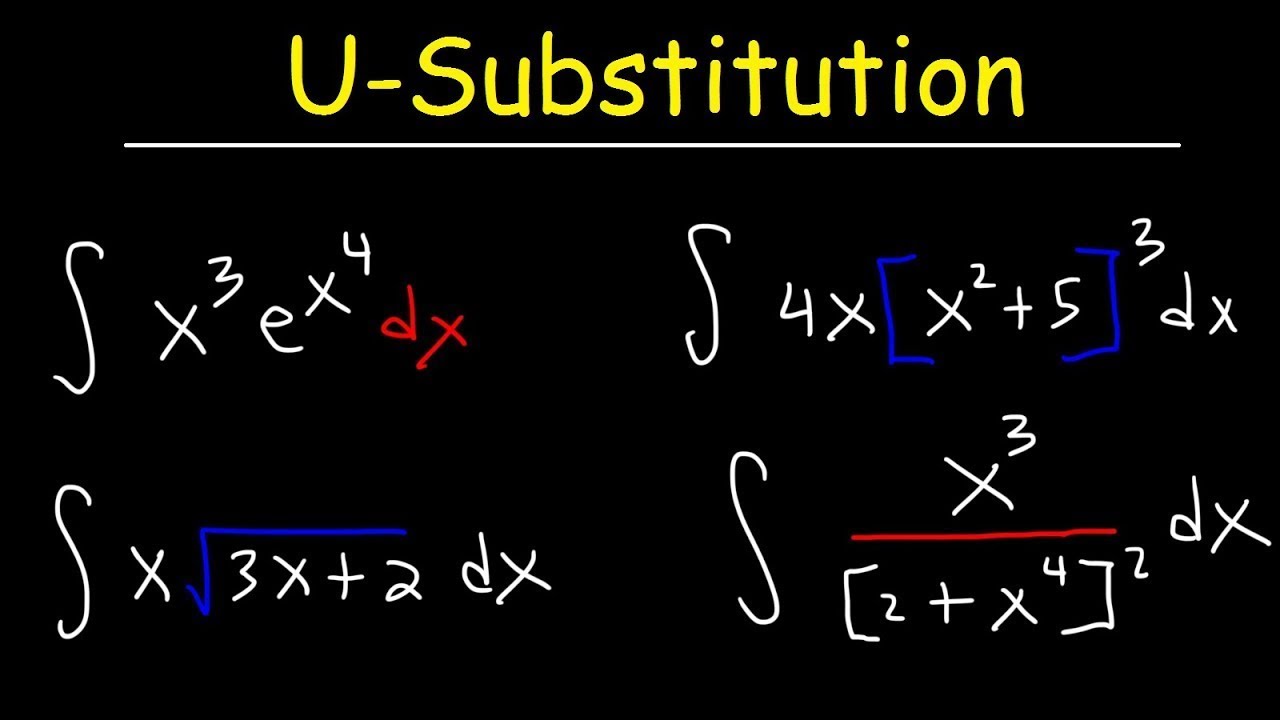
- 📝 The process of substitution, particularly u-substitution, is emphasized as a crucial technique for solving complex integrals.
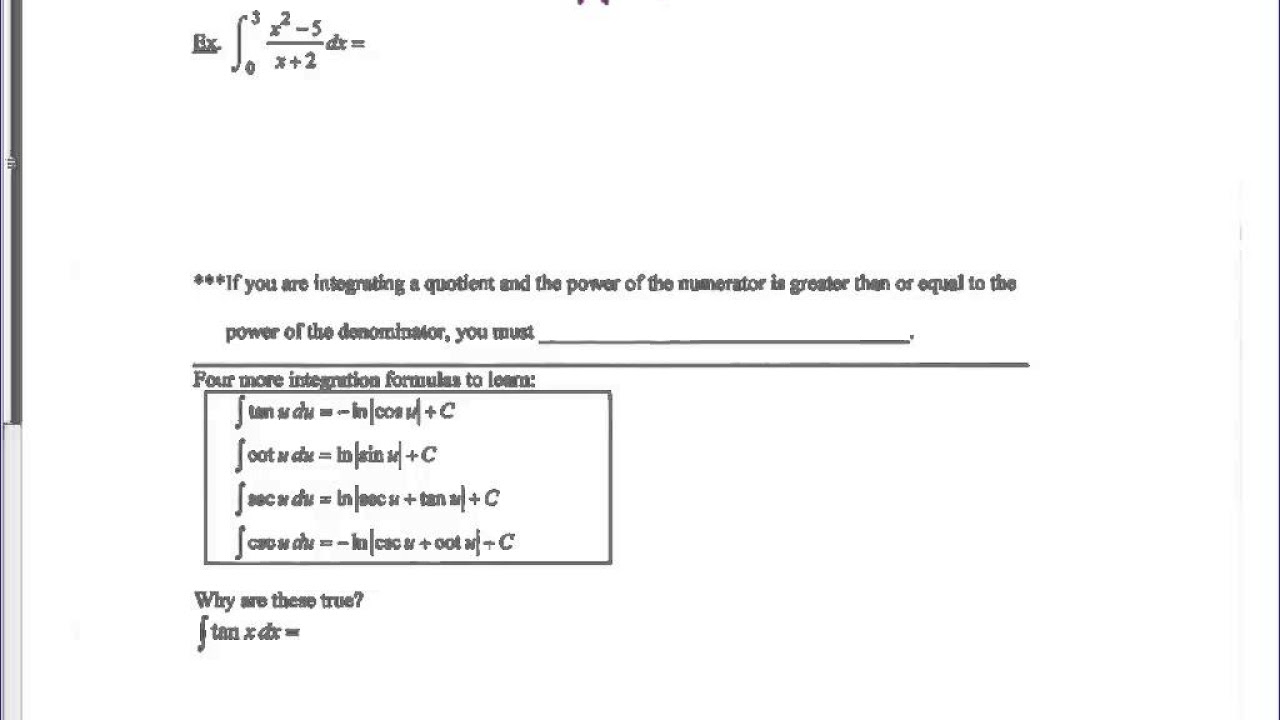
- 🔗 The script demonstrates how to deal with integrals involving high-degree polynomials in the numerator using long division.
- 🤔 The importance of recognizing and applying the correct inverse trigonometric formula based on the structure of the integral is highlighted.
- 📊 A definite integral example is worked out, showing the process of evaluating the integral within specific limits.
- 📈 The script concludes with a clear demonstration of how to find the antiderivative of a complex function involving a negative sign and a square root.
Q & A
What are the three key formulas for integrating functions involving inverse trigonometric functions?
-The three key formulas are: (1) the antiderivative of du/(sqrt(a^2 - u^2)) is arcsin(u/a) + C, (2) the integral of du/(a^2 + u^2) is 1/a * arctan(u/a) + C, and (3) the integral of du/(u * sqrt(u^2 - a^2)) is 1/a * arcsec(u/a) + C.
How is the value of 'a' determined in the context of the first formula?
-In the first formula, 'a' is determined by the expression under the square root in the denominator, specifically as the square root of that expression (e.g., if the denominator is under a square root of 16 - u^2, then 'a' would be the square root of 16, which is 4).
What is the role of 'u' in the integration formulas?
-'U' serves as a substitution variable that represents the expression being differentiated, typically the variable in the function whose antiderivative is being sought.
How does the script demonstrate the use of the arc sine formula in an example?
-The script demonstrates the arc sine formula by integrating dx/(sqrt(16 - x^2)). It identifies 'u' as x and 'a' as 4, then applies the formula arcsin(u/a) + C to find the antiderivative, resulting in arcsin(x/4) + C.
What is the purpose of completing the square in integration problems?
-Completing the square is used to transform a quadratic expression into a perfect square trinomial, which can then be more easily integrated using the arc tangent or other relevant trigonometric integration formulas.
How does the script handle the integration of a function with a cubic term in the numerator, like x^3/(x^2 + 1) dx?
-The script uses long division to separate the integrand into simpler parts. It divides x^3 by x^2 to get x, and then combines like terms to get a remainder of -x. The result is then integrated in two parts: the antiderivative of x and the natural logarithm of the expression involving the remainder.
What is the significance of the constant term in the denominator of the second formula?
-The constant term in the denominator of the second formula (a^2 + u^2) is significant because it represents the pattern for integrating functions using the arctangent formula, where 'a' is a constant and 'u' is the variable being integrated.
How does the script approach the integration of a function with a radical in the numerator, like 8/(x*sqrt(4x^2 - 1)) dx?
-The script recognizes the similarity to the arc secant formula and sets up the integration by identifying 'u' as 2x and 'a' as 1. It then performs the substitution and simplification to arrive at the antiderivative involving the arc secant function.
What is the method used in the script to solve for the antiderivative of a function with a complex denominator, like x/(sqrt(1 - x^4)) dx?
-The script uses a method of completing the square for the denominator and then applies the arc sine formula after setting 'a' to 1 and 'u' to x^2. The integration involves solving for 'dx' in terms of 'du' and substituting the values into the arc sine formula.
How does the script handle the definite integral of a function involving an arctan expression, like the integral from 3 to 6 of du/(25 + (x - 3)^2)?
-The script identifies 'a' as 5 and 'u' as x - 3. It then evaluates the antiderivative at the upper and lower limits of integration, using the arc tangent function, and finds the difference between these values to get the definite integral.
What is the final result of the definite integral example in the script?
-The final result of the definite integral example is 0.108, which is the value of the arc tangent of 3/5 minus the arc tangent of 0, when the calculator is in radian mode.
Outlines
📚 Introduction to Integration of Inverse Trigonometric Functions
This paragraph introduces the concept of integrating functions that lead to inverse trigonometric functions. It emphasizes the importance of knowing three key formulas for the antiderivative of specific expressions involving trigonometric functions. The first formula involves the arcsine function, the second is related to the arctangent function, and the third pertains to the arcsecant function. The paragraph stresses the need to memorize these formulas for their frequent use throughout the video.
🧠 Application of Arcsine Formula
The paragraph demonstrates the application of the arcsine formula through an example. It explains the process of identifying the values of 'a' and 'u', where 'u' is equivalent to 'x' and 'a' is determined based on the given expression. The example shows the step-by-step substitution of 'u' and 'a' into the arcsine formula to find the antiderivative of a function involving the square root of 16 minus x squared. The explanation is clear and methodical, guiding the viewer through the integration process.
🔢 Solving More Integration Problems
This paragraph continues the theme of integration by tackling more complex problems involving inverse trigonometric functions. It presents a series of examples, each requiring the use of the previously introduced formulas. The paragraph covers the integration of expressions involving arctangent and arcsecant functions, with a focus on identifying the correct 'u' substitution and the appropriate formula to apply. The examples are worked out in detail, providing a comprehensive understanding of the integration process for these types of functions.
📈 Completing the Square and Integration
The paragraph delves into the technique of completing the square as it applies to integration problems. It explains how to transform expressions into a form that allows for the use of the arctan formula. The paragraph provides a step-by-step guide on how to complete the square, factor the expression, and then apply the arctan formula. It also introduces the concept of long division for integration when the degree of the numerator is higher than the denominator, offering a method for dealing with more complex integrands.
🌟 Advanced Integration Techniques
This paragraph presents advanced integration techniques, focusing on problems that cannot be solved with simple substitution or completing the square. It introduces the concept of using the natural logarithm function in integration and demonstrates how to handle more complex expressions through u-substitution and factoring. The paragraph also covers the integration of exponential functions in the context of inverse trigonometric functions, showcasing a variety of methods to tackle different types of integration problems.
📊 Solving Definite Integral Problems
The paragraph concludes the video script with a discussion on definite integral problems. It explains the process of evaluating a definite integral by applying the antiderivative formulas and using the limits of integration. The paragraph emphasizes the removal of the constant 'c' in definite integrals and provides a clear example of calculating the value of a definite integral involving the arctan function. The explanation is concise and focuses on the practical application of integration techniques.
Mindmap
Keywords
💡Integration
💡Antiderivative
💡Inverse Trigonometric Functions
💡Substitution
💡Completing the Square
💡Arc Sine (arcsin)
💡Arc Tangent (arctan)
💡Arc Secant (arcsec)
💡Trigonometric Identities
💡Definite Integral
Highlights
The video discusses the integration of functions that lead to inverse trigonometric functions, focusing on three key formulas.
The first formula presented is the antiderivative of du/(a^2*sqrt(a^2-u^2)) which equals arcsin(u/a) + C.
The second formula is the integration of du/(a^2+u^2) which equals 1/a*arctan(u/a) + C.
The third formula is the integration of du/(u*sqrt(u^2-a^2)) which equals 1/a*arcsec(u/a) + C.
An example is provided where the antiderivative of dx/(sqrt(16-x^2)) is calculated, demonstrating the use of the first formula.
Another example involves the antiderivative of (3/(25+x^2))dx, showcasing the application of the second formula.
The video also covers the integration of functions with more complex denominators, such as 8/(x*sqrt(4x^2-1))dx, using the third formula.
The process of identifying the values of 'a' and 'u' within the given function is explained in detail for each example.
The video demonstrates the method of completing the square to simplify the integration process for certain types of functions.
Long division is introduced as a technique to handle integration problems where the degree of the numerator is higher than the denominator.
The video shows how to integrate a function with an exponential term, such as e^(3x)/sqrt(9+e^(6x))dx.
A method for dealing with integrals that cannot be directly solved using the given formulas is presented, involving splitting the integral into manageable parts.
The video concludes with a demonstration of solving a definite integral problem, highlighting the difference between definite and indefinite integrals.
Throughout the video, the importance of substituting variables correctly and simplifying expressions is emphasized.
The video provides a comprehensive guide on integrating functions involving inverse trigonometric functions, showcasing various techniques and methods.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: