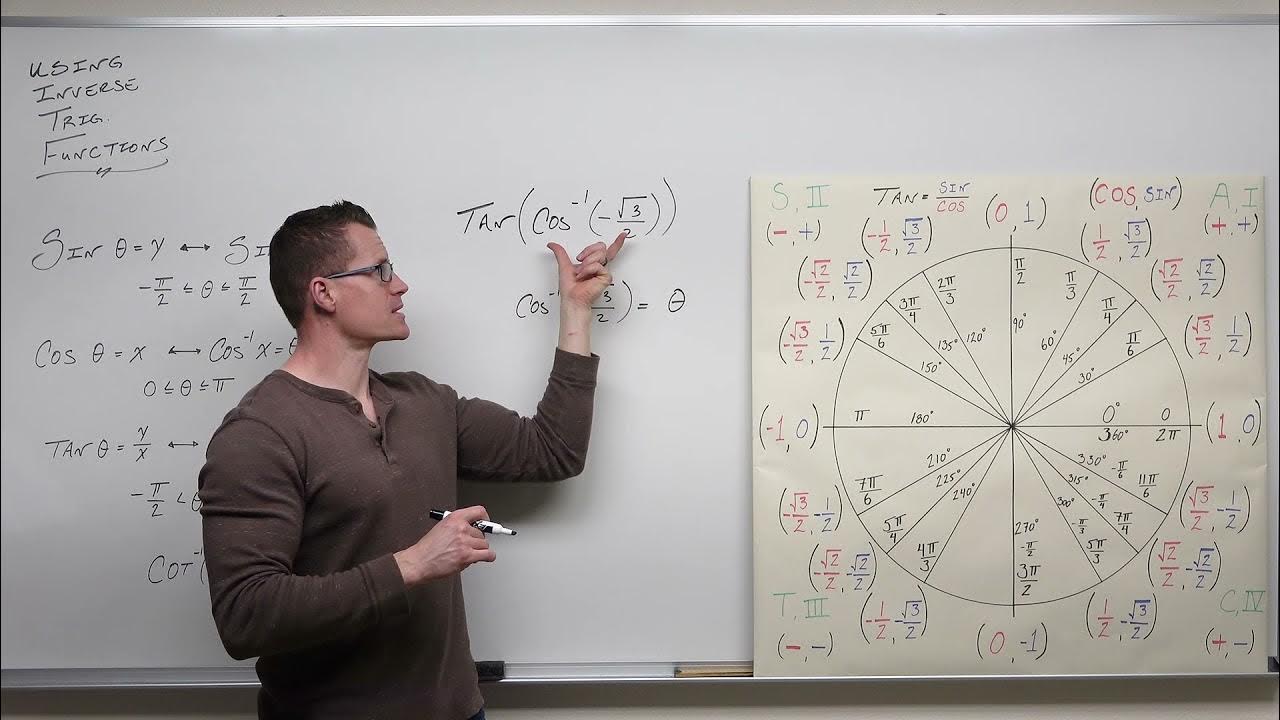Trigonometric Functions of Any Angle - Unit Circle, Radians, Degrees, Coterminal & Reference Angles

The Organic Chemistry Tutor

9 Nov 201659:17

EducationalLearning

32 Likes 10 Comments

TLDRThis video delves into evaluating trigonometric functions at various angles, explaining the SOHCAHTOA mnemonic for sine, cosine, and tangent. It covers the concepts of reciprocal trigonometric functions and provides examples of applying these functions to right triangles, including special triangles like the 3-4-5 and 5-12-13. The video also addresses how to find missing sides or angles using the Pythagorean theorem and how to determine the quadrant of a triangle based on given trigonometric values. Additionally, it introduces the unit circle, the conversion between degrees and radians, and the concept of coterminal angles and reference angles for evaluating trig functions beyond the basic unit circle.

Takeaways

📐 The acronym SOHCAHTOA helps remember trigonometric ratios: Sine (opposite/hypotenuse), Cosine (adjacent/hypotenuse), and Tangent (opposite/adjacent).
📐 Reciprocal trigonometric identities: Secant (1/cosine), Cosecant (1/sine), and Cotangent (1/tangent).
📐 To find missing sides or angles in a right triangle, use the Pythagorean theorem (a² + b² = c²) and trigonometric functions.
📐 Special triangles with easy-to-remember side ratios include 3-4-5, 5-12-13, 8-15-17, 7-24-25, 9-40-41, and 11-60-61.
📐 Quadrants and signs of trigonometric functions: Sine is positive in I and II, Cosine in I and IV, and Tangent in I and III.
📐 To solve for unknowns in trigonometric problems, identify the quadrant in which the triangle lies based on the signs of the trigonometric functions.
📐 The unit circle is a circle with a radius of 1, where sine corresponds to the y-coordinate, cosine to the x-coordinate, and tangent to y/x.
📐 Convert between degrees and radians using the formulas: degrees × (π/180) for radians and radians × (180/π) for degrees.
📐 Coterminal angles have the same trigonometric function values, differing only in their initial direction from the positive x-axis.
📐 Reference angles are used to find the values of trigonometric functions for angles greater than 90 degrees (or π/2 radians) by finding the acute angle formed with the x-axis.

Q & A

What does SOHCAHTOA stand for in trigonometry?
-SOHCAHTOA is a mnemonic that stands for Sine (opposite/hypotenuse), Cosine (adjacent/hypotenuse), and Tangent (opposite/adjacent). The last three letters represent the reciprocal trigonometric functions: Secant (1/cosine), Cosecant (1/sine), and Cotangent (1/tangent).
How do you find the missing side of a right triangle given two sides and an angle?
-You can use the Pythagorean theorem (a^2 + b^2 = c^2) to find the missing side of a right triangle when you know two sides and an angle. Label the known sides as 'a' and 'b' and the hypotenuse as 'c', then solve for 'c' algebraically.
What are special right triangles and why are they important?
-Special right triangles are those with side lengths that have specific ratios. The most common ones are the 3-4-5 and 5-12-13 triangles. They are important because they allow you to easily find the trigonometric function values for the angles without a calculator, by memorizing the ratios and using the properties of the triangle.
How do you determine the quadrant in which a right triangle lies based on the values of its trigonometric functions?
-You can determine the quadrant by considering the signs of the trigonometric functions. For example, if sine is positive and cosine is also positive, the triangle is in the first quadrant. If cosine is negative and sine is positive, it's in the second quadrant, and so on.
What is the unit circle and how does it relate to trigonometric functions?
-The unit circle is a circle with a radius of 1 centered at the origin of a coordinate plane. It's used to define the trigonometric functions sine, cosine, and tangent for angles other than just those at the vertices of a right triangle. The y-coordinate of a point on the unit circle corresponds to the sine of the angle, and the x-coordinate corresponds to the cosine.
How do you convert degrees to radians?
-To convert degrees to radians, multiply the degree measure by π/180. For example, 180 degrees is π radians, 90 degrees is π/2 radians, and so on.
What are coterminal angles and how do you find them?
-Coterminal angles are angles that share the same initial side and terminal side, differing only by a full rotation (360 degrees or 2π radians). To find a coterminal angle, add or subtract multiples of 360 degrees (or 2π radians) from the given angle until you get an angle within the desired range (usually between 0 and 360 degrees or 0 and 2π radians).
What is a reference angle and how is it used?
-A reference angle is the acute angle formed by the terminal side of an angle and the x-axis. It is used to find the values of trigonometric functions for angles greater than 90 degrees (or π/2 radians) by finding the equivalent acute angle in the first quadrant that has the same trigonometric values.
How do you find the value of a trigonometric function for an angle in a different quadrant?
-To find the value of a trigonometric function for an angle in a different quadrant, first determine the reference angle, which is the acute angle formed by the terminal side of the given angle and the x-axis. Then, use the sign of the function in the quadrant and the value of the function at the reference angle to find the value at the given angle.
What is the process for evaluating trigonometric functions for negative angles?
-To evaluate trigonometric functions for negative angles, first find a positive coterminal angle by adding 360 degrees (or 2π radians) to the negative angle. Then, use the properties of the quadrant where the coterminal angle lies to determine the sign of the trigonometric function values.
How do you rationalize a trigonometric expression with a radical in the denominator?
-To rationalize a trigonometric expression with a radical in the denominator, multiply both the numerator and the denominator by the conjugate of the denominator. The conjugate is the expression obtained by changing the sign of the radical in the denominator.

Outlines

00:00

📚 Introduction to Trigonometric Functions and SOHCAHTOA

This paragraph introduces the concept of evaluating trigonometric functions at different angles, focusing on the SOHCAHTOA mnemonic. It explains the sine, cosine, and tangent ratios in relation to a right triangle's sides and angle. Additionally, it introduces reciprocal trigonometric functions—secant, cosecant, and cotangent—as well as their relationships to the primary functions. The paragraph also provides an example of how to apply this information to a triangle with given sides.

05:03

📐 Special Triangles and Trigonometric Function Evaluation

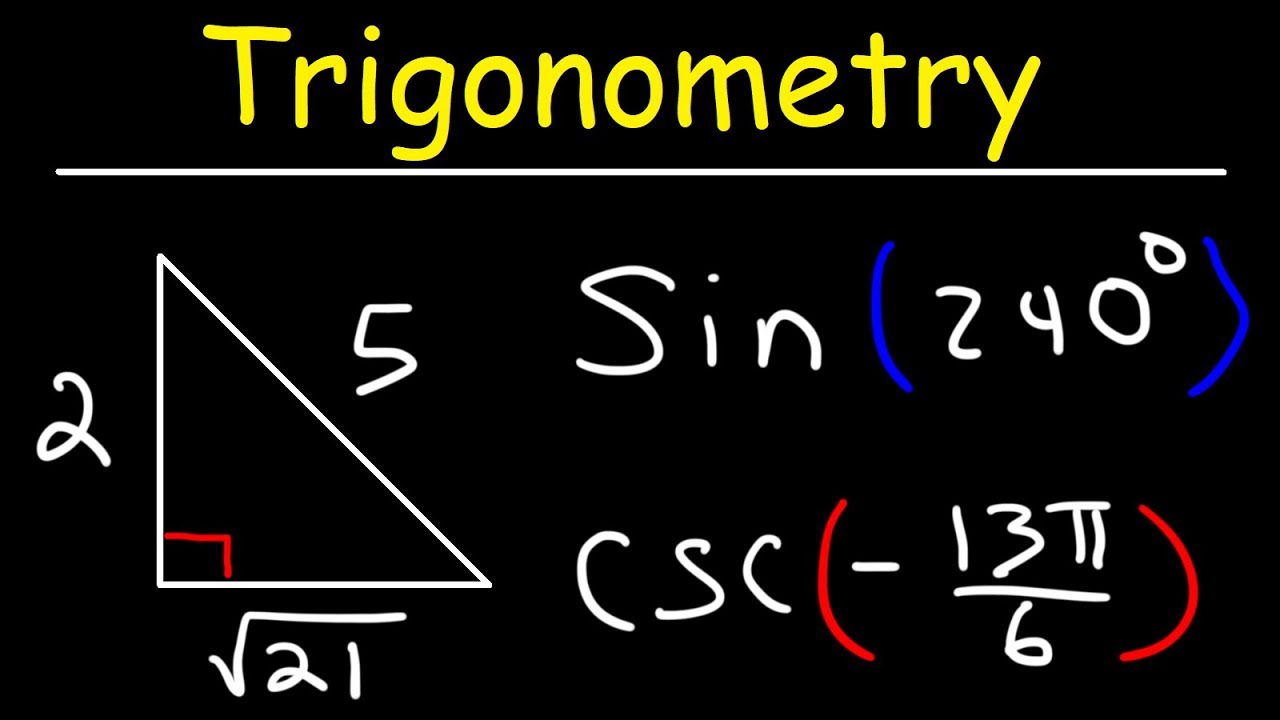
The second paragraph delves into special triangles, such as the 3-4-5 and 5-12-13 triangles, and how to use the Pythagorean theorem to find missing sides. It then demonstrates how to calculate all six trigonometric functions for a given right triangle, using both the ratios and the properties of these special triangles. The paragraph also explains how to determine the quadrant in which a right triangle lies based on the signs of sine and cosine, and how to use this information to find the remaining trigonometric functions given one value.

10:06

🔢 Solving Trigonometric Problems with Quadrants and Reference Angles

This paragraph discusses solving trigonometric problems by identifying the quadrant in which the angle lies and using reference angles to find the values of trigonometric functions. It explains how to find the reference angle for an angle in a specific quadrant and how to use the signs of trigonometric functions in different quadrants to determine the correct values. The paragraph also covers how to find the values of trigonometric functions for angles larger than 360 degrees or in negative form by finding coterminal angles.

15:07

📐 Applications of Trigonometry: Solving for Missing Sides and Angles

The fourth paragraph focuses on applying trigonometry to solve for missing sides and angles in right triangles. It presents a method for using tangent, sine, and cosine to find the height of a building given the angle of elevation and distance from the base. The paragraph also explains how to use the inverse trigonometric functions to find the missing angle when the opposite side and hypotenuse are known.

20:13

🌀 Understanding the Unit Circle and Converting Degrees to Radians

This paragraph introduces the unit circle and its significance in trigonometry. It explains how the unit circle relates to the trigonometric functions and how to find the terminal point of an angle on the unit circle. The paragraph also covers the conversion between degrees and radians, providing formulas and examples to illustrate the process. Additionally, it discusses the concept of coterminal angles and how they relate to the unit circle.

25:15

🔄 Finding Coterminal and Reference Angles for Advanced Trigonometry

The sixth paragraph delves into the concepts of coterminal and reference angles, which are crucial for evaluating trigonometric functions for angles greater than 90 degrees or in negative form. It explains how to find both positive and negative coterminal angles by adding or subtracting multiples of 360 degrees or 2π radians. The paragraph also describes how to calculate reference angles for angles in different quadrants and provides formulas for finding reference angles based on the quadrant of the given angle.

30:17

📐 Evaluating Trigonometric Functions Using Special Triangles and Reference Angles

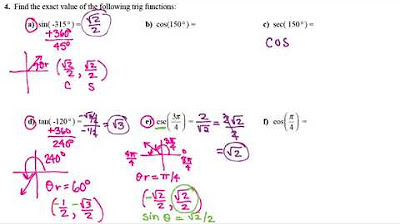
The seventh paragraph demonstrates how to evaluate trigonometric functions without a calculator by using special triangles and reference angles. It explains how to find the values of sine, cosine, and tangent for angles like 30, 60, and 45 degrees using the 30-60-90 and 45-45-90 triangles. The paragraph also shows how to use reference angles to determine the sign and value of trigonometric functions for angles not found in these special triangles.

35:17

🤔 Solving Trigonometric Problems with Negative and Large Angles

The eighth paragraph addresses the evaluation of trigonometric functions for negative and large angles. It explains how to find coterminal angles and reference angles to determine the correct values of sine, cosine, and tangent. The paragraph provides examples of how to handle angles that are negative or exceed 360 degrees by adding or subtracting multiples of 360 degrees or 2π radians. It also covers how to rationalize trigonometric expressions to simplify the values.

40:22

📐 Advanced Trigonometric Function Evaluation: Secant, Cosecant, and Cotangent

The final paragraph focuses on evaluating the reciprocal trigonometric functions—secant, cosecant, and cotangent—for angles in different quadrants. It explains how to find the values of these functions by using the primary trigonometric functions and the properties of the unit circle. The paragraph provides examples of how to calculate secant, cosecant, and cotangent for various angles, including those in quadrants where these functions are negative or positive.

Mindmap

Keywords

💡Trigonometric Functions

Trigonometric functions are mathematical functions that relate the angles of a right triangle to the lengths of its sides. These functions, including sine, cosine, and tangent, are fundamental in solving problems involving triangles and are used extensively in various fields such as physics and engineering. In the video, the functions are explained using the SOHCAHTOA mnemonic to remember their relationships with the sides of a right triangle and how to apply them to find missing side lengths or angles.

💡SOHCAHTOA

SOHCAHTOA is a mnemonic used to remember the basic trigonometric ratios for a right triangle. It stands for Sine (opposite/hypotenuse), Opposite (side across from the angle), Hypotenuse (longest side), Cosine (adjacent/hypotenuse), Angle (theta), and Tangent (opposite/adjacent). The video uses this mnemonic to explain how to calculate the trigonometric functions for a given angle in a right triangle.

💡Reciprocal Identities

Reciprocal identities are relationships in trigonometry that connect the basic trigonometric functions with their reciprocals. Specifically, the secant (1/cosine), cosecant (1/sine), and cotangent (1/tangent) are the reciprocals of the primary trigonometric functions. These identities are used to find the values of these reciprocal functions when the values of sine, cosine, and tangent are known.

💡Pythagorean Theorem

The Pythagorean theorem is a fundamental principle in geometry that states that in a right triangle, the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the lengths of the other two sides. This theorem is essential for finding the missing side of a right triangle when the other two sides are known.

💡Special Triangles

Special triangles are right triangles with side lengths that have specific ratios, making it easier to remember and use in trigonometry. The most common special triangles mentioned in the video are the 3-4-5 and 5-12-13 triangles, which have side lengths that are multiples of small integers. Knowing these special triangles can simplify the process of solving trigonometric problems.

💡Quadrants

In the context of trigonometry, quadrants refer to the four sections of the coordinate plane, each defined by the intersection of the positive x-axis and the positive y-axis. Quadrants are important because they help determine the signs of the trigonometric functions. For instance, sine is positive in the first and second quadrants, while cosine is positive in the first and fourth quadrants.

💡Unit Circle

The unit circle is a circle with a radius of 1 centered at the origin of the coordinate plane. It is used in trigonometry to define the trigonometric functions for angles measured in radians. The coordinates on the unit circle correspond to the values of cosine and sine for a given angle, with the x-coordinate representing cosine and the y-coordinate representing sine.

💡Reference Angle

A reference angle is the acute angle formed by the terminal side of an angle and the x-axis in the counterclockwise direction. It is used to find the values of trigonometric functions for angles greater than 90 degrees or in different quadrants. The reference angle is the same for coterminal angles, which are angles that share the same terminal side.

💡Coterminal Angles

Coterminal angles are angles that share the same terminal side and therefore have the same trigonometric values. They can be found by adding or subtracting multiples of 360 degrees (or 2π radians) to the given angle. Coterminal angles are useful for simplifying the evaluation of trigonometric functions for angles that are not easily managed within the standard range of 0 to 360 degrees or 0 to 2π radians.

💡Trigonometric Identities

Trigonometric identities are equations that relate different trigonometric functions or the same function at different angles. They are used to simplify trigonometric expressions and to solve trigonometric equations. The video covers reciprocal identities, which are a type of trigonometric identity.

💡Angle Conversion

Angle conversion involves changing an angle from one unit of measurement to another, such as from degrees to radians or vice versa. This is necessary when working with trigonometric functions, as some problems require angles to be expressed in different units. The conversion factor between degrees and radians is π, with 180 degrees equal to π radians.

Highlights

Introduction to evaluating trigonometric functions at different angles using the SOHCAHTOA mnemonic.

Explanation of sine, cosine, and tangent as ratios of sides in a right triangle relative to an angle.

Discussion of reciprocal trigonometric functions: secant, cosecant, and cotangent.

Application of trigonometric functions using an example right triangle with sides 3, 4, and 5.

Use of the Pythagorean theorem to find the missing side of a right triangle and evaluate trigonometric functions.

Explanation of special right triangles and their side ratios: 3-4-5, 5-12-13, 8-15-17, 7-24-25, 9-40-41, and 11-60-61.

Method for finding the values of all six trigonometric functions given two sides of a right triangle.

Determination of the quadrant in which a right triangle lies based on the signs of sine and cosine.

Process for finding the missing side of a triangle and evaluating trigonometric functions using given values of sine and cosine.

Explanation of how to find the missing trigonometric functions given the value of tangent and the quadrant of the angle.

Use of the unit circle to understand and evaluate trigonometric functions for angles on the coordinate plane.

Conversion of degrees to radians and vice versa, with examples and formulas.

Introduction to coterminal angles and their properties, including how to find positive and negative coterminal angles.

Explanation of reference angles and how they are used to evaluate trigonometric functions for angles greater than 90 degrees.

Examples of evaluating trigonometric functions without a calculator using the 30-60-90 and 45-45-90 triangles.

Method for evaluating trigonometric functions for angles in quadrants two, three, and four using reference angles.

Process for finding the values of trigonometric functions for angles with negative measures or larger than 360 degrees.

Detailed examples of evaluating secant, cosecant, and cotangent using the concepts of reference angles and coterminal angles.

Transcripts

Browse More Related Video

Precalc 4.1-4.4 Review!

Introduction to Right Triangle Trigonometry (Precalculus - Trigonometry 30)

Trigonometry For Beginners!

An Indepth Look at Using Inverse Trig Functions (Precalculus - Trigonometry 21)

Trigonometry

Trigonometry: Unit Circle

Related Tags

Trigonometry SOHCAHTOA Right Triangles Sine Function Cosine Function Tangent Function Reciprocal Identities Special Triangles Trigonometric Ratios Math Education Problem Solving