Calculus 1 Lecture 5.3: Volume of Solids By Cylindrical Shells Method
TLDRThe video script is an in-depth exploration of the cylindrical shells method for calculating the volume of a solid of revolution. The speaker begins by contrasting this method with the disk and washer methods, emphasizing the importance of aligning the function's terms with the axis of revolution. The script then delves into step-by-step instructions on how to apply the cylindrical shells method, highlighting the need to consider the function in terms of the variable associated with the axis of revolution. Several examples are provided to illustrate the process, including calculating the volume of a region bounded by curves and revolving it around different axes. The speaker also discusses the scenarios where the cylindrical shells method is more advantageous than the washer method, particularly when dealing with functions that are naturally expressed in terms of one variable. The summary underscores the efficiency of the method when it comes to setup and integration, encouraging students to choose the approach that requires the least amount of work and is most straightforward for their specific problem. The script concludes with an assurance that with the right setup, the integral calculations become a matter of plugging in numbers, making the cylindrical shells method a powerful tool in the calculus student's arsenal.
Takeaways
- 📚 The cylindrical shells method is used to find the volume of a solid of revolution created by revolving a region around the y-axis.
- 🎨 When using cylindrical shells, the function should be in terms of x, even when revolving around the y-axis, which might seem counterintuitive.
- 🔄 The general formula for the volume using cylindrical shells is V = 2π ∫(a to b) (r1 - r2) f(x) dx, where r1 and r2 are the radii of the larger and smaller circles, respectively.
- ⭕️ The cross-sectional area of each shell is a circle, and the volume of a single shell is found by multiplying the area of the cross-section by the height (function value) and thickness of the shell.
- 📐 The height of each shell is determined by the function value at an arbitrary point, often chosen as the midpoint of the interval for integration.
- 🚫 It's crucial not to confuse the cylindrical shells method with the disk or washer method, which have different applications and require functions in terms of the axis of revolution.
- 🧮 For regions bounded by two curves, the volume is found by subtracting the volume of the lower function from the volume of the upper function when using the cylindrical shells method.
- ⛔ Negative volumes are not possible in these calculations; if encountered, it indicates an error in the setup or the choice of which function represents the top or bottom of the revolution.
- 🔢 The setup of the integral is the most critical step; once correctly set up, evaluating the integral is often straightforward.
- 📈 The choice between using the washer method or the cylindrical shells method depends on the simplicity of the setup and the least amount of work required to solve the problem.
- ❗ Always double-check the bounds of integration and the function that represents the top surface to ensure the correct volume calculation.
Q & A
What is the cylindrical shells method used for calculating volume?
-The cylindrical shells method is used to calculate the volume of a solid of revolution created by revolving a region bounded by two curves around a central axis.
Why might the cylindrical shells method be preferred over the disk or washer method?
-The cylindrical shells method might be preferred when the region to be revolved is naturally described in terms of one variable (e.g., x), and using the disk or washer method would require considering two different functions in terms of another variable (e.g., y), making the problem more complex.
How does the shape of the cross-section of a cylindrical shell affect its volume calculation?
-The volume of a cylindrical shell is calculated by considering the area of the cross-section (which is a circle) times the height and thickness of the shell. The area of the cross-section is found by subtracting the area of the smaller circle (if present) from the larger circle.
What is the general formula for the volume of a cylindrical shell in terms of the function f(x) and the variable x?
-The general formula for the volume of a cylindrical shell is given by 2*pi*∫[a, b] (average radius)*(height)*(differential thickness), where the average radius is typically (R1 + R2)/2, the height is f(x), and the differential thickness is dx.
What is the significance of choosing an arbitrary point within the interval for the cylindrical shells method?
-Choosing an arbitrary point, often the midpoint, allows for the precise determination of the height of the shell at that point, which is necessary for calculating the volume. As the thickness of the shell approaches zero, this arbitrary point becomes representative of all points within the interval.
How does the cylindrical shells method differ when revolving around the x-axis versus the y-axis?
-When using the cylindrical shells method, the function and the terms must be in terms of the axis around which the revolution occurs. If revolving around the y-axis, the function and terms should be in terms of x, and vice versa for the x-axis. This is because the height of the shell corresponds to the function's value for that axis.
What is the process for calculating the volume of a region bounded by two curves using the cylindrical shells method?
-First, determine the bounds of integration by finding the points where the two curves intersect. Then, set up the integral using the formula for the volume of a cylindrical shell, ensuring the function is in terms of the correct variable based on the axis of revolution. Finally, integrate the expression for the volume over the determined interval.
Why is it important to ensure that the function used in the cylindrical shells method is the correct one on top?
-It is important to ensure the correct function is on top because the volume calculation involves subtracting the volumes of the shells created by the two functions. If the functions are reversed, it could result in a negative volume, which is not possible in a physical context.
How do you determine the bounds of integration (a and b) for the cylindrical shells method?
-The bounds of integration are determined by finding the points where the curves that define the region intersect or where the region starts and ends. These points are then used as the limits for the integral that calculates the volume.
What is the key difference between the cylindrical shells method and the washer method for calculating volumes?
-The key difference is that the cylindrical shells method considers the volume of cylindrical shells created by revolving the region around an axis, while the washer method considers the volume of cylindrical washers (or disks with holes). The choice between the two depends on the geometry of the region and the axis of revolution.
Why might the washer method be more complex or less preferred for certain problems?
-The washer method might be more complex or less preferred when the functions defining the region cannot be easily expressed in terms of the variable associated with the axis of revolution. In such cases, additional steps are required to solve for the necessary variable, which can complicate the setup and integration process.
Outlines
📚 Introduction to Cylindrical Shells Method
The paragraph introduces the cylindrical shells method for calculating the volume of a solid of revolution. It discusses the complexity of using the disk or washer method when revolving around the y-axis, as it would require two different functions. The cylindrical shells method simplifies this by considering the volume in terms of a single function of x, revolving around the y-axis, creating a shape akin to a jello mold with a cavity. The concept involves slicing the volume into infinitesimally thin cylindrical shells and summing their volumes.
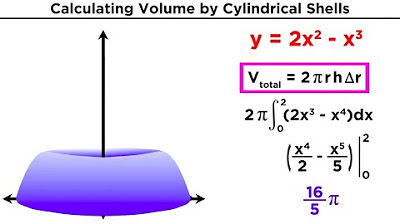
🧮 Calculating the Volume of a Cylinder
This section delves into the specifics of calculating the volume of a cylinder using the cylindrical shells method. It emphasizes that the volume of each cylindrical shell is found by multiplying the area of the cross-section (which is the difference between the areas of the outer and inner circles, πr1^2 - πr2^2) by the height (f(x)) and thickness (the function's value at an arbitrary point within the shell). The process involves integrating this expression over the interval [a, b] to find the total volume.
🏗️ Constructing the Cylindrical Shells Formula
The paragraph focuses on deriving the general formula for the cylindrical shells method. It simplifies the expression for the volume of a single shell by factoring out constants and rearranging terms to express the volume in terms of the average radius, height, and thickness of the shell. The resulting formula is 2π times the average radius, times the height, times the thickness, integrated over the interval of x values.
🔍 Choosing the Correct Midpoint for Shells
The discussion here is about selecting an appropriate midpoint for the interval when setting up the cylindrical shells. It is clarified that the midpoint, denoted as x_kdot, should be the average of x_k and x_(k-1), which corresponds to the midpoint of the interval. This choice ensures that the height of the shell at the arbitrary point x_kdot can be accurately represented by the function f(x) at that point.
🔢 The Limit of Sums and the Exact Volume
This paragraph explains how to find the exact volume of the solid by taking the limit of the sum of the volumes of the individual shells as the number of shells approaches infinity. It highlights that as the thickness of each shell approaches zero, the sum of the volumes of the shells becomes an integral from the starting point a to the endpoint b of the interval. The integral involves 2π times the function of x (f(x)), representing the volume of each shell, and is evaluated over the interval [a, b].
🎯 Appropriate Use of Cylindrical Shells Around the y-axis
The focus is on determining the suitability of using the cylindrical shells method when revolving a region around the y-axis. It emphasizes that the method is appropriate when the function is in terms of x, even when revolving around the y-axis. The paragraph also provides an example problem involving the region enclosed by y = √x and x = 4, revolving around the y-axis, and outlines the steps to set up and evaluate the integral to find the volume.
🔄 Revolving Around the x-axis vs. the y-axis
This section clarifies the difference in approach when revolving a region around the x-axis versus the y-axis using the cylindrical shells method. It stresses the importance of ensuring that the function used is in terms of the correct variable: x for the y-axis and y for the x-axis. The paragraph also addresses a common mistake made by students regarding the axis and the variable in terms of which the function should be expressed.
📏 Setting Up and Solving the Integral
The paragraph provides a detailed walkthrough of setting up and solving an integral using the cylindrical shells method. It involves identifying the correct function to integrate, which in the case of volume between two curves, is the top function minus the bottom function. The integral is then evaluated over the interval [a, b], and the process is illustrated with an example involving functions of x.
🤔 Choosing Between Shells and Washers Methods
This section discusses the decision-making process when choosing between the cylindrical shells and washers methods for finding volumes. It points out that while both methods can be used in many cases, the shells method is often simpler when revolving around the y-axis, as it requires the function to be in terms of x. The washers method, which requires functions in terms of y when revolving around the y-axis, can be more complex due to the need to solve for y, making it less desirable in certain scenarios.
🧐 Understanding the Setup for Cylindrical Shells
The final paragraph emphasizes the importance of setting up the problem correctly when using the cylindrical shells method. It discusses the process of determining the interval of integration and identifying which function is on top. The paragraph also touches on the common pitfalls, such as obtaining a negative volume, which is not possible in these calculations. It concludes by encouraging students to practice and become comfortable with the setup process, as it is crucial for successfully applying the cylindrical shells method.
Mindmap
Keywords
💡Cylindrical Shells Method
💡Disk Method
💡Volume of Revolution
💡Integration
💡Function in Terms of X
💡Function in Terms of Y
💡Midpoint
💡Average Radii
💡Limits
💡Revolving Around the Axis
💡Setup of the Integral
Highlights
Introduction to the cylindrical shells method for calculating volumes of revolution, emphasizing its utility when dealing with functions of X revolved around the Y-axis.
Explanation of why the disk or washer method is not suitable for functions revolving around the Y-axis, as it would require two different functions in terms of Y.
Illustration of the cylindrical shells method using the analogy of a cake with circular cross-sections, demonstrating how to find the volume of each shell.
Derivation of the formula for the volume of a cylindrical shell, emphasizing the importance of considering the average radius, height, and thickness of the shell.
Clarification on the process of taking limits to find the volume of each infinitely thin cylindrical shell, leading to the integral formula for the total volume.
Discussion on the importance of correctly identifying the function in terms of X or Y, depending on the axis of revolution, to avoid mistakes in setting up the integral.
Demonstration of setting up and evaluating an integral using the cylindrical shells method to find the volume enclosed by the function y = sqrt(x) from x = 1 to x = 4, revolved around the Y-axis.
Comparison of the cylindrical shells method with the disk or washer method, highlighting the conditions under which each method is most appropriately used.
Presentation of a method to determine the volume between two functions using cylindrical shells, by subtracting the volumes of the individual shells created by the two functions.
Step-by-step guide on setting up the integral for cylindrical shells around the X-axis, emphasizing the need for functions to be in terms of Y.
Example calculation of the volume for a region bounded by x = -y^2 + 6y and x = 0, revolved around the X-axis, including the process of finding the correct bounds of integration.
Emphasis on the avoidance of negative volumes in calculations, as they are physically impossible and indicate a mistake in the setup or evaluation of the integral.
Instruction on the correct procedure for setting up and evaluating an integral using the cylindrical shells method around the Y-axis for a region bounded by multiple curves.
Comparison between the cylindrical shells and washer methods for a region bounded by y = x^2 + 1, y = -x + 1, and x = 1, highlighting the complexity and potential pitfalls of the washer method.
Final example demonstrating the cylindrical shells method for a region bounded by three curves, with a focus on the importance of correct setup and the ease of integration once setup is complete.
Advice on choosing the appropriate method (shells or washers) for a given problem, based on the simplicity of setup and the least amount of work required.
Encouragement for students to practice and become comfortable with setting up and evaluating integrals for the cylindrical shells method, as it is a powerful tool for calculating volumes of revolution.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: