Calculus: Volumes by Cylindrical Shells (Section 6.3) | Math with Professor V

Math with Professor V

6 May 202023:33

EducationalLearning

32 Likes 10 Comments

TLDRThis video tutorial explores the method of cylindrical shells for calculating volumes of solids of revolution. It contrasts this approach with the disk and washer methods previously covered, highlighting the unique setup where slices are peeled parallel to the axis of rotation. The instructor walks through several examples, demonstrating how to identify the radius and height for the integral, and emphasizes the importance of choosing the correct method based on the problem's geometry and the ease of integration. The video is designed to help viewers understand and apply the cylindrical shells method to a variety of volume computation problems.

Takeaways

📚 The video introduces the method of cylindrical shells for computing volumes of solids of revolution, contrasting it with the previously discussed methods of disks and washers.
🔄 The key formula for the cylindrical shells method is the integral from A to B of \(2\pi RH \, \text{d}x\), where \(R\) is the radius and \(H\) is the height of the cylindrical shell.
📏 The setup for cylindrical shells involves 'peeling' the solid parallel to the axis of rotation, unlike the perpendicular slicing used in the disks and washers method.
📈 The script provides a step-by-step example of finding the volume of a solid formed by revolving a parabolic region around the y-axis, using the cylindrical shells method.
🧩 The process of unrolling a cylindrical shell results in a rectangular solid, which is summed up through integration to find the total volume of the solid.
🤔 The choice between using cylindrical shells or disks and washers can depend on the complexity of integration with respect to a particular variable and the ease of setting up the problem.
📉 An example of a solid formed by revolving a region bounded by a cubic function around the x-axis is given, demonstrating the setup and integration process for cylindrical shells.
📐 The video discusses the importance of determining the correct limits of integration based on the original region to be revolved, rather than the resulting solid.
📘 The method of cylindrical shells is shown to be particularly effective for solids with gaps or irregular shapes, as demonstrated with the example of a triangle revolved around an offset axis.
📝 The script encourages viewers to practice setting up problems using both methods to understand their applications and to decide which they prefer.
🌐 The video concludes with an invitation for viewers to share their preferences and suggest topics for future videos.

Q & A

What is the main topic of the video?
-The main topic of the video is how to compute volumes using the method of cylindrical shells in calculus.
What are the two different methods for computing volumes of solids of revolution mentioned in the script?
-The two methods mentioned are the method of disks or washers and the method of cylindrical shells.
What is the formula used for the volume computation when using the method of cylindrical shells?
-The formula for volume computation using cylindrical shells is the definite integral from A to B of 2π * R * H * dX (or dY depending on the axis of rotation).
How does the orientation of the slices differ between the method of disks/washers and the method of cylindrical shells?
-In the method of disks/washers, slices are taken perpendicular to the axis of rotation, while in the method of cylindrical shells, slices are peeled parallel to the axis of rotation.
What is the geometric interpretation of the formula for the volume using cylindrical shells?
-The geometric interpretation involves unrolling each cylindrical shell into a rectangular solid, where the length is 2πR (the circumference of the circle), the height is H (the function value), and the thickness is dX (or dY).
In the script, what is the first example given to demonstrate the method of cylindrical shells?
-The first example given is revolving the region bounded by y = x^2, y = 0, and x = 1 around the y-axis.
What is the integral set up for the first example in the script?
-The integral set up for the first example is the integral from 0 to 1 of 2π * x * x^2 * dX.
What is the result of the volume computation for the first example in the script?
-The result of the volume computation for the first example is π/2.
In the second example, what is the region being revolved around the x-axis?
-The region being revolved around the x-axis in the second example is bounded by the curve x = 4y^2 - y^3, the y-axis, and x = 0.
What is the integral set up for the volume computation of the second example in the script?
-The integral set up for the second example is the definite integral from 0 to 4 of 2π * y * (4y^2 - y^3) * dY.
What is the result of the volume computation for the second example in the script?
-The result of the volume computation for the second example is 512π/5.
Why might one choose the method of disks over cylindrical shells in certain situations?
-One might choose the method of disks over cylindrical shells when it is easier to integrate with respect to a particular variable, or when the setup for cylindrical shells involves solving for the variable in the equation, which can be complex.
What is the strategy for choosing between the method of cylindrical shells and the method of disks for a given problem?
-The strategy involves assessing which variable is easier to integrate with respect to, and whether the setup for one method is more straightforward than the other based on the function's form and the axis of rotation.

Outlines

00:00

📚 Introduction to Cylindrical Shells Method

This paragraph introduces the method of cylindrical shells for computing volumes of solids of revolution. It contrasts this method with the previously discussed disk and washer methods. The formula for the volume using cylindrical shells is given as the definite integral from A to B of 2πRH dX, where R is the radius and H is the height of the cylindrical shell. The method involves 'peeling' the solid parallel to the axis of rotation, unlike the disk method which slices perpendicularly. The paragraph sets up the foundation for understanding how to apply this method to various examples.

05:01

📐 Example 1: Volume of a Solid Formed by a Parabola

The first example demonstrates how to find the volume of a solid created by revolving the region bounded by y = x^2, y = 0, and x = 1 around the y-axis. The method of cylindrical shells is applied, with the integral set up as 2π times the radius (x) times the height (x^2) with respect to x, from 0 to 1. The integral is then simplified and solved to find the volume, which is π/2. The paragraph also suggests trying the washer method for additional practice.

10:05

📉 Example 2: Solid of Revolution Around the x-axis

The second example involves finding the volume of a solid formed by revolving the region bounded by the curve x = 4y^2 - y^3, the y-axis, and the line x = 0 around the x-axis. The paragraph explains the process of setting up the integral for the volume using cylindrical shells with respect to y, identifying the radius as y and the height as the given function. The integral is then calculated, resulting in a volume of 512π/5.

15:08

🔍 Choosing the Right Method for Volume Calculation

This paragraph discusses the decision-making process for choosing between cylindrical shells and disks when calculating volumes of solids of revolution. It uses the example of a parabola y = -x^2 + 6x - 8, bounded by the x-axis and the lines x = 2 and x = 4, spun around the x-axis. The paragraph explains why the disk method might be more suitable for this particular problem due to the complexity of setting up the cylindrical shell method and encourages the viewer to consider the ease of integration when choosing a method.

20:12

🏞️ Example 3: Volume of a Solid with a Gap

The final example shows how to calculate the volume of a solid formed by revolving the triangle defined by y = x, x = 2, and y = 0 around the line x = -4. The paragraph describes the resulting solid's shape, which has a gap due to the line of revolution. It then demonstrates the setup for the cylindrical shell method, identifying the radius as x + 4 and the height as x. The integral is calculated, yielding a volume of 64π/3, and the paragraph concludes by asking the viewer's preference for the method used.

Mindmap

Keywords

💡Cylindrical Shells

Cylindrical shells refer to a method used in calculus to calculate the volume of a solid of revolution by 'peeling' the solid into infinitesimally thin cylindrical layers parallel to the axis of rotation. In the video, this method is contrasted with the disk and washer methods, highlighting its unique approach to slicing the solid. The script provides a detailed explanation of how to set up and compute the volume using this method, as seen in the examples provided.

💡Disks and Washers

Disks and washers are alternative methods for calculating volumes of solids of revolution, where slices are taken perpendicular to the axis of rotation. The script mentions these methods as a recap from a previous lesson, explaining that they involve integrating the area of circular disks (for solids without holes) or the difference in areas of outer and inner circles (for solids with holes) over a given interval.

💡Solid of Revolution

A solid of revolution is a three-dimensional shape created by rotating a two-dimensional region around an axis. The video's main theme revolves around calculating the volumes of such solids using different methods, including cylindrical shells, disks, and washers. The script provides examples of solids formed by revolving regions bounded by functions around various axes.

💡Definite Integral

A definite integral is a fundamental concept in calculus used to calculate the accumulated value under a curve between two points. In the context of the video, definite integrals are used to sum up the volumes of infinitesimally thin slices (disks, washers, or shells) to find the total volume of the solid of revolution. The script demonstrates how to set up and evaluate these integrals for different examples.

💡Volume Calculation

Volume calculation is the process of determining the amount of space occupied by a solid. The video focuses on this concept, showing how to calculate the volume of solids of revolution using various methods. The script walks through the steps of setting up and solving integrals to find the volume in different scenarios.

💡Axis of Rotation

The axis of rotation is the line around which a two-dimensional region is rotated to form a solid of revolution. The script emphasizes the importance of the axis in determining how to slice the solid (perpendicular or parallel) when using different volume calculation methods.

💡Parabola

A parabola is a U-shaped curve that is often the result of a quadratic function. In the script, a parabola is used as the boundary of a region that, when revolved around an axis, forms a solid whose volume is to be calculated. The video demonstrates how to set up volume calculations for solids formed by revolving parabolic regions.

💡Integration Variable

The integration variable is the variable with respect to which an integral is taken. The script discusses the importance of choosing the correct integration variable (either x or y) based on the geometry of the solid and the axis of rotation, which affects the setup of the volume calculation.

💡Antiderivative

An antiderivative is a function that represents the reverse process of differentiation and is used to evaluate definite integrals. The video script includes examples of finding antiderivatives as part of the process of calculating volumes using the cylindrical shells method.

💡Method Selection

Method selection refers to the process of choosing the most appropriate method for calculating the volume of a solid of revolution based on the given function and axis of rotation. The script illustrates how to decide between using cylindrical shells, disks, or washers based on the simplicity of the setup and the ease of integration.

Highlights

Introduction to computing volumes by cylindrical shells, a method different from the disk or washer method.

Recap of the disk and washer method, where volume is calculated using the formula involving pi, the radius squared, and the definite integral.

Explanation of the cylindrical shells method, emphasizing peeling parallel to the axis of rotation to compute volume.

The formula for volume using cylindrical shells is 2πRH dx, where R is the radius and H is the height of the cylindrical shell.

Demonstration of how to compute the volume of a solid formed by revolving a region around the y-axis using cylindrical shells.

Example of finding the volume of a solid formed by revolving the region under y=x^2 from x=0 to x=1 around the y-axis.

Step-by-step calculation of the volume of the solid in the previous example, resulting in π/2 as the final volume.

Discussion on choosing between cylindrical shells and washers based on the complexity of integration with respect to one variable.

Second example involving the volume of a solid formed by revolving the region bounded by x=4y^2 - y^3 around the x-axis.

Detailed setup for the integral to find the volume of the solid in the second example, integrating with respect to y.

Calculation of the volume for the second example, yielding a final answer of 512π/5.

Third example with a parabola y=-x^2 + 6x - 8, bounded by the x-axis, and revolving around the x-axis, illustrating the choice of method.

Analysis of the difficulty in using cylindrical shells for the third example due to the need to solve for x and the complexity of the height function.

Decision to use the disk method for the third example for simplicity, and setup of the integral for volume calculation.

Fourth example with the line y=x, forming a triangle, revolving around x=-4, creating a unique solid with a gap in the middle.

Application of the cylindrical shells method to the fourth example, identifying the radius and height for the integral setup.

Final calculation of the volume for the fourth example, resulting in a volume of 64π/3.

Invitation for viewers to comment, like, subscribe, and suggest topics for future videos, encouraging engagement with the content.

Transcripts

Browse More Related Video

Calculus 1 Lecture 5.3: Volume of Solids By Cylindrical Shells Method

AP Calculus AB: Lesson 7.5: Shell Method

Calculus Chapter 4 Lecture 33 Complex Volumes

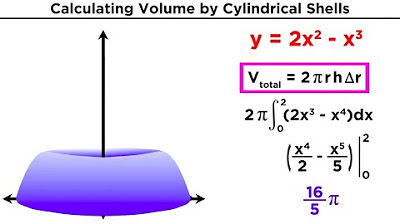
Calculating Volume by Cylindrical Shells

AP Calculus AB: Lesson 7.4: Disk and Washer Method

Unit IV: Lec 3 | MIT Calculus Revisited: Single Variable Calculus

Related Tags

Volume Calculation Cylindrical Shells Math Tutorial Solid Revolution Integral Calculus Disk Method Washer Method Geometry Problems Educational Video Mathematics Education Volume Examples