11 - Learn ArcSin, ArcCos & ArcTan (Inverse Sin, Cos & Tan) - Part 1
TLDRThe video lesson delves into the concepts of inverse trigonometric functions, specifically arc sine, arc cosine, and arc tangent. It emphasizes the importance of understanding these functions' behavior, as they are fundamental to algebra, trigonometry, calculus, physics, and engineering. The lesson clarifies the confusion around the range of angles these functions return, explaining that while there are multiple angles that satisfy a given trigonometric value, calculators and computers will provide the principal angle within specific ranges. The video aims to demystify these concepts by walking through examples and the logic behind the functions, ensuring learners grasp why these operations work as they do and how to apply them effectively.
Takeaways
- 📚 The lesson introduces inverse trigonometric functions: arc sine, arc cosine, and arc tangent, which are the inverse operations of their respective trigonometric functions.
- 🔄 Understanding inverse functions is crucial as they 'undo' the original function, allowing us to solve for angles in various mathematical contexts.
- 🤔 The concept of inverse trig functions can be confusing due to the infinite number of angles that can yield the same sine, cosine, or tangent value, but calculators return a specific range of angles based on conventions.
- 📐 The arc sine function takes values from -1 to 1 and returns angles in the range of -π/2 to π/2 (or -90° to 90°), which are in the first and fourth quadrants.
- 📐 The arc cosine function also takes values from -1 to 1, but its output is limited to angles in the range of 0 to π (or 0° to 180°), covering only the first and fourth quadrants.
- 📐 The arc tangent function can accept input values from negative infinity to positive infinity, but it outputs angles strictly within the range of -π/2 to π/2 (or -90° to 90°), which includes angles in the second and fourth quadrants.
- 🧩 When solving equations using inverse trig functions, it's important to recognize that while there are multiple angles that satisfy a given trigonometric value, the calculator will provide the principal value based on the established ranges.
- 🔢 The input values for inverse sine and inverse cosine are constrained because sine and cosine are limited to the interval [-1, 1], reflecting their respective projections on the unit circle.
- 🔢 The tangent function's ability to reach infinity and negative infinity is due to its nature as the ratio of sine to cosine, where the cosine can approach zero, significantly affecting the tangent's value.
- 💡 Memorizing the ranges for the output of inverse trig functions is essential for their application in algebra, trigonometry, calculus, physics, and engineering.
- 🚀 Practice is key to mastering the nuances of inverse trig functions, and understanding their behavior is crucial for success in advanced mathematical and scientific studies.
Q & A
What are the three main inverse trigonometric functions discussed in the lesson?
-The three main inverse trigonometric functions discussed are arc sine (inverse sine), arc cosine (inverse cosine), and arc tangent (inverse tangent).
What is the primary purpose of inverse trigonometric functions?
-The primary purpose of inverse trigonometric functions is to find the angles from given trigonometric values, effectively 'undoing' the operation of the standard trigonometric functions.
What is the range of input values for the arc sine function?
-The input values for the arc sine function can only be between -1 and 1, as these are the possible outputs of the sine function.
What is the typical range of angles returned by the arc sine function?
-The arc sine function typically returns angles in the range of -π/2 to π/2 radians (or -90 to 90 degrees), which corresponds to the principal values.
How does the arc cosine function differ from the arc sine function in terms of input and output ranges?
-The arc cosine function, like the arc sine, takes input values between -1 and 1. However, it returns angles in the range of 0 to π radians (or 0 to 180 degrees), which is the interval from the positive x-axis to the top of the unit circle.
What is unique about the arc tangent function compared to arc sine and arc cosine functions?
-The arc tangent function is unique because it can take input values ranging from negative infinity to positive infinity, and it returns angles in the range of -π/2 to π/2 radians (or -90 to 90 degrees), covering all possibilities of the tangent function values from negative infinity to positive infinity.
Why does the unit circle play a crucial role in understanding inverse trigonometric functions?
-The unit circle is crucial because it provides a visual representation of the trigonometric values and their corresponding angles. It helps to understand the range of values that the trigonometric functions can take and how these values relate to the angles on the circle.
What is the significance of the 'gotcha' mentioned in the lesson regarding inverse trigonometric functions?
-The 'gotcha' refers to the potential confusion and common mistakes students make when dealing with inverse trigonometric functions, particularly in understanding the multiple angles that can correspond to the same trigonometric value and the specific ranges of angles that calculators return for these functions.
How do you determine the correct angle when there are multiple angles with the same sine, cosine, or tangent value?
-To determine the correct angle, one must consider the quadrant in which the angle lies and any additional information provided by the problem. Typically, inverse trigonometric functions return the principal value, which is the smallest angle within the specified range that satisfies the given trigonometric value.
What is the relationship between the trigonometric functions and their inverses?
-The relationship between the trigonometric functions (sine, cosine, tangent) and their inverses (arc sine, arc cosine, arc tangent) is that the inverse functions essentially 'undo' the operation of the standard functions, allowing us to solve for the angle given a specific trigonometric value.
Why is it important to understand the behavior of inverse trigonometric functions in different quadrants?
-Understanding the behavior of inverse trigonometric functions in different quadrants is important because it helps us to accurately determine the correct angle when solving trigonometric equations, especially when dealing with multiple solutions that may exist in different quadrants.
Outlines
📚 Introduction to Inverse Trigonometric Functions
The lesson begins with an introduction to inverse trigonometric functions, specifically arc sine, arc cosine, and arc tangent. The instructor expresses excitement about teaching these concepts, acknowledging their complexity and the common confusion they cause in traditional textbooks. The goal is to provide a clear understanding of these functions, emphasizing their importance in various fields such as algebra, trigonometry, calculus, physics, and engineering. The lesson aims to clarify the behavior of these functions and address common misconceptions by walking through detailed problem sequences.
🔍 Understanding the Basics of Inverse Trigonometric Functions
This paragraph delves into the basics of inverse trigonometric functions, explaining the concept of the inverse operation. It highlights how the arc sine, arc cosine, and arc tangent functions are the inverses of their respective trigonometric functions, allowing for the reverse engineering of angles from their sine, cosine, or tangent values. The instructor clarifies the input and output ranges for these functions, noting that while the input values are limited (between -1 and 1 for sine and cosine, and between negative infinity and positive infinity for tangent), the output is a specific range of angles (between -90 to 90 degrees or -π/2 to π/2 radians).
🤔 Addressing the Challenges with Inverse Trigonometric Functions
The instructor addresses the challenges of understanding inverse trigonometric functions, particularly the confusion that arises from the multiple angles that can produce the same sine, cosine, or tangent value. This is due to the periodic nature of these functions and the existence of multiple solutions within the unit circle. The lesson emphasizes the importance of understanding the logic behind these functions and the limitations of calculators and computers when providing solutions, which are constrained to specific ranges of angles.
📈 Demonstrating the Inverse Trigonometric Functions with Examples
The paragraph provides examples to illustrate how inverse trigonometric functions work. It explains how the arc sine of a value gives an angle whose sine is that value, and similarly for arc cosine and arc tangent. The examples use known values such as the sine of 30 degrees (π/6 radians) and the cosine of 30 degrees to demonstrate the process of finding the corresponding angles. The paragraph also introduces the notation for inverse functions, clarifying that the negative exponent does not indicate raising the function to a negative power but rather denotes the inverse operation.
🔄 Exploring the Concept of Functions as Boxes and the Gotcha
The instructor uses the analogy of a function as a box to explain the process of inputting numbers into inverse trigonometric functions and getting angles as outputs. It emphasizes the importance of remembering that angles, not just numbers, are the outputs when working with these functions. The paragraph also introduces the concept of a 'gotcha' related to the multiple angles that can satisfy a given trigonometric equation, highlighting the need for a thorough understanding of the underlying principles to avoid confusion.
📊 Solving Equations with Inverse Trigonometric Functions
This section discusses the process of solving equations using inverse trigonometric functions. It explains how to isolate the variable by applying the inverse function to both sides of the equation, effectively 'undoing' the trigonometric operation. The example of solving for an angle when given the sine value of 1/2 is used to illustrate this process. The paragraph also introduces the concept of the unit circle and how it relates to finding the correct angle among the infinite possibilities that satisfy the given trigonometric value.
🌐 Multiple Angles and the Unit Circle
The paragraph explores the concept of multiple angles around the unit circle that can produce the same trigonometric value. It uses the sine function as an example, showing that while the sine of π/6 (30 degrees) is 1/2, other angles such as 5π/6 and 13π/6 also have a sine value of 1/2. This highlights the challenge of determining the correct angle when using inverse trigonometric functions, as there are infinitely many angles that can satisfy a given sine, cosine, or tangent value. The paragraph sets the stage for a deeper discussion on how to handle these multiple solutions in trigonometric equations.
📐 The Range of Inputs and Outputs for Inverse Trigonometric Functions
The lesson continues to clarify the range of inputs and outputs for inverse trigonometric functions. It explains that while the sine function can only output values between -1 and 1, the arc sine function can only accept inputs in the same range and will output angles between -π/2 and π/2. Similarly, the arc cosine function accepts inputs between -1 and 1 and outputs angles between 0 and π. The tangent function, which can output values ranging from negative infinity to positive infinity, accepts inputs in this range as well, but the arc tangent function outputs angles only between -π/2 and π/2, covering all possibilities for the tangent function's range.
🎯 Summarizing the Key Points of Inverse Trigonometric Functions
The paragraph summarizes the key points about inverse trigonometric functions, emphasizing the importance of understanding the range of angles they can output. It reiterates that the calculator will provide the smallest possible angle that satisfies the input value, which is the fundamental angle. The paragraph also reminds that while there are multiple angles around the unit circle that can produce the same trigonometric value, the calculator will only return the fundamental angle, and additional angles can be found by adding or subtracting multiples of 180 degrees or 360 degrees to move into other quadrants.
Mindmap
Keywords
💡Trigonometric Functions
💡Inverse Trigonometric Functions
💡Unit Circle
💡Arc Sine (Inverse Sine)
💡Arc Cosine (Inverse Cosine)
💡Arc Tangent (Inverse Tangent)
💡Range of Outputs
💡Multiple Angles
💡Principal Values
💡Trigonometric Identities
💡Periodic Functions
Highlights
The lesson focuses on understanding the inverse trigonometric functions: arc sine, arc cosine, and arc tangent.
Inverse functions are the opposite operations of their respective trigonometric functions, used to find angles from given values.
Arc sine, arc cosine, and arc tangent can be confusing due to the multiple angles that can produce the same trigonometric value.
The arc sine operation returns angles in the range of negative pi/2 to pi/2 radians, or -90 to 90 degrees.
The arc cosine operation returns angles in the range of 0 to pi radians, or 0 to 180 degrees.
The arc tangent operation returns angles in the range of negative pi/2 to pi/2 radians, or -90 to 90 degrees.
The unit circle plays a crucial role in determining the angles returned by inverse trigonometric functions.
Inverse trigonometric functions are essential in algebra, trigonometry, precalculus, calculus, physics, and engineering.
The lesson emphasizes the importance of not just memorizing but truly understanding the behavior of inverse trigonometric functions.
The concept of inverse functions is used to solve equations involving trigonometric functions by 'undoing' the operation.
The lesson provides a detailed explanation of how to handle the 'gotchas' or potential pitfalls when working with inverse trigonometric functions.
The lesson uses visual aids and examples to clarify the concepts of inverse sine, arc cosine, and arc tangent.
The lesson explains why the calculator or computer only returns a specific range of angles for each inverse trigonometric operation.
The lesson covers the mathematical convention that defines the range of angles returned by inverse trigonometric functions.
The lesson provides a comprehensive overview of the behavior of inverse trigonometric functions, preparing students for their applications in various fields.
The lesson emphasizes the practical applications of inverse trigonometric functions in solving real-world problems.
Transcripts
Browse More Related Video
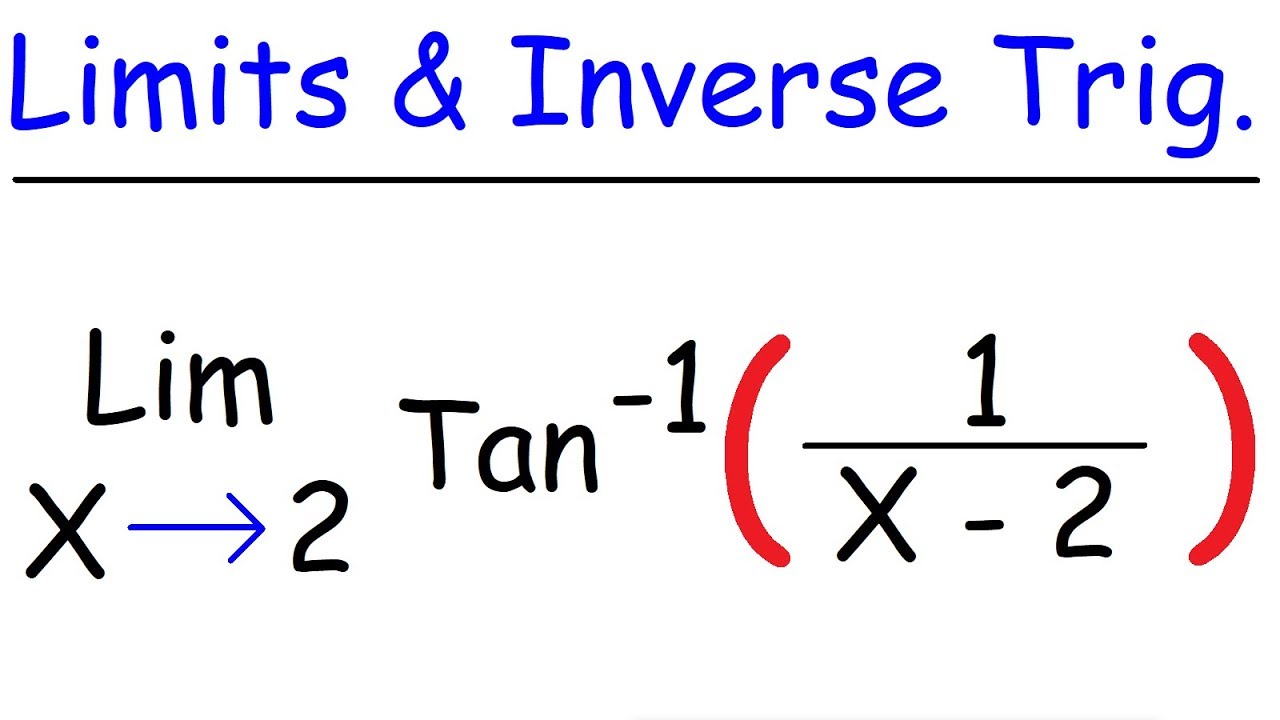
Limits of Inverse Trigonometric Functions | Calculus

Using Sum and Difference Formulas in Trigonometry (Precalculus - Trigonometry 26)
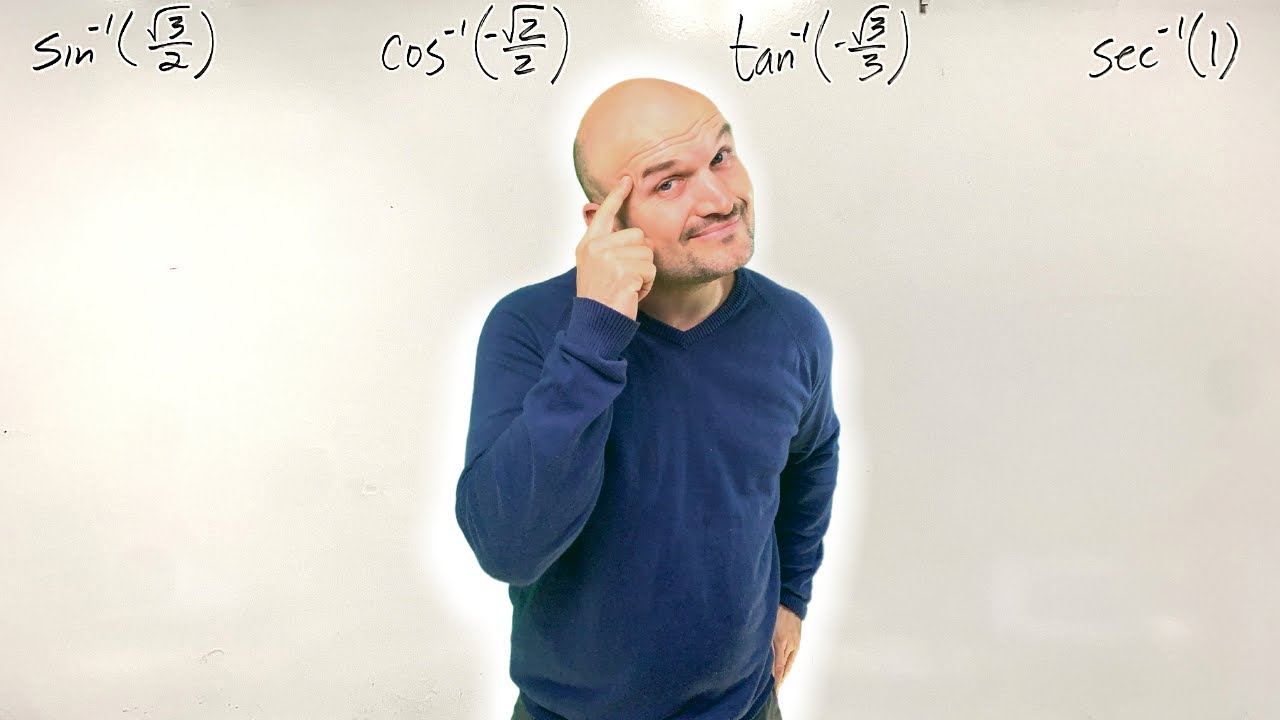
Evaluate Inverse Trig Functions - Step by Step

Trigonometry made easy

Basic Properties of Trigonometric Functions (Precalculus - Trigonometry 8)
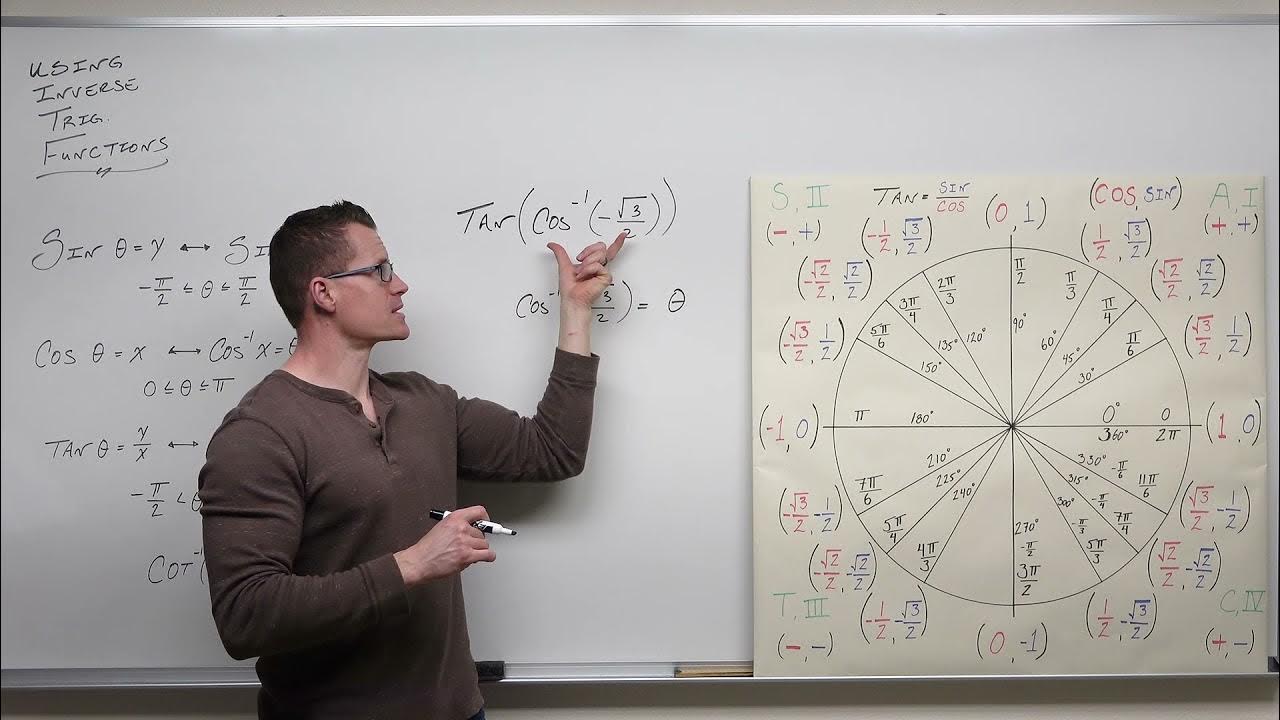
An Indepth Look at Using Inverse Trig Functions (Precalculus - Trigonometry 21)
5.0 / 5 (0 votes)
Thanks for rating: