Evaluate Inverse Trig Functions - Step by Step

Brian McLogan
13 Jan 202108:53
EducationalLearning
32 Likes 10 Comments

TLDRThis video tutorial explains the concept and application of inverse trigonometric functions, emphasizing the importance of understanding their origins and purpose. It covers the restrictions placed on these functions, such as the domain for sine (-π/2 to π/2), cosine (0 to π), and tangent (-π/2 to π/2), to ensure they are one-to-one and invertible. The video uses four examples to illustrate how to evaluate these functions, highlighting the need to find angles that satisfy the given restrictions and the process of using the unit circle to determine these angles.

Takeaways
  • 📚 Understanding inverse trigonometric functions involves knowing their origins and purposes.
  • 🔍 Inverse trigonometric functions 'undo' the regular trigonometric functions.
  • 🤔 The sine of an angle (θ) can be thought of as the y-coordinate of a point on the unit circle.
  • 📊 Evaluating trigonometric functions requires recognizing that they may have multiple solutions, but inverse functions need a single solution.
  • 🚫 To find the inverse, restrict the domain of the sine function to between -π/2 and π/2.
  • 📐 For cosine, the domain is restricted to between 0 and π.
  • 🔢 The tangent function is a ratio of y to x, which complicates the process of finding the inverse.
  • 📍 Tangent is negative in the second and fourth quadrants, which helps in determining the correct angle for the inverse.
  • 🌐 The restrictions ensure that the inverse trigonometric functions are one-to-one, making them invertible.
  • 📌 The restrictions for tangent are the same as for sine, from -π/2 to π/2.
  • ⏩ Secant is often confused with the inverse or reciprocal, but it can be rewritten in terms of cosine for easier evaluation.
Q & A

  • What are inverse trigonometric functions used for?

    -Inverse trigonometric functions are used to 'undo' the results of the standard trigonometric functions, allowing us to find the angle when we know the value of a trigonometric function for that angle.

  • Why do we need to understand where the inverse trigonometric functions come from?

    -Understanding the origin of inverse trigonometric functions helps us grasp the concept of how they can reverse the process of standard trigonometric functions, which is crucial for their application in various mathematical and real-world problems.

  • What is the restriction for the sine function when evaluating its inverse?

    -When evaluating the inverse sine function, the angle (theta) must be between -π/2 and π/2.

  • How many solutions does the equation sin(θ) = √3/2 have within the given restriction?

    -Within the given restriction, there is one solution: θ = π/3.

  • What is the restriction for the cosine function when evaluating its inverse?

    -When evaluating the inverse cosine function, the angle (theta) must be between 0 and π.

  • What angle satisfies the equation cos(θ) = -√2/2 within the given restriction?

    -Within the given restriction, the angle that satisfies the equation is θ = 3π/4.

  • Why are there two potential solutions for the equation tan(θ) = -√3/2?

    -There are two potential solutions because tangent is a periodic function and can have two angles in different quadrants that yield the same value. However, when finding the inverse, we must consider the restriction to select the valid solution.

  • What are the restrictions for the tangent function when evaluating its inverse?

    -When evaluating the inverse tangent function, the angle (theta) must be between -π/2 and π/2.

  • How can we find the angle that satisfies the equation cot(θ) = -√3/2?

    -By rewriting the equation as the cosine of theta equals 1 over √(1 - (√3/2)^2), we find that the angle must be such that cos(θ) = 0, which is at θ = 0 within the given restriction.

  • Why do we have restrictions on the angles in inverse trigonometric functions?

    -Restrictions are necessary to ensure that the inverse trigonometric functions are one-to-one, meaning each output has a unique input. Without restrictions, the functions would fail the horizontal line test and not be invertible.

  • What is the primary reason for the different restrictions on sine, cosine, and tangent functions?

    -The different restrictions arise because of the periodic nature of these functions and their behavior in different quadrants of the unit circle. The restrictions ensure that the inverse functions are valid and can be accurately determined.

Outlines
00:00
📚 Understanding Inverse Trigonometric Functions

This paragraph introduces the concept of inverse trigonometric functions and explains their purpose as the 'undo' operation for trigonometric functions. It emphasizes the importance of understanding their origins and the reasons for their existence. The speaker outlines a plan to cover four different examples to clarify these functions, their restrictions, and their applications. The first example uses the sine function and its inverse to illustrate how to find an angle whose sine value is equal to a given number, highlighting the concept of coterminal angles and the need for restricting the domain of the sine function to between -π/2 and π/2 to ensure one-to-one correspondence.

05:01
📐 Evaluating Inverse Trigonometric Functions with Restrictions

The second paragraph delves into the specifics of evaluating inverse trigonometric functions, focusing on the restrictions that must be applied to ensure the functions are one-to-one. It covers the inverse cosine function, explaining how to find the angle that satisfies a given cosine value within the restricted domain of [0, π]. The discussion then moves to the tangent function, highlighting the challenges of dealing with y/x form and the quadrant restrictions for tangent. The paragraph also touches on the cotangent function and its relationship with the sine function, emphasizing the importance of staying within the defined restrictions. Finally, the secant function is introduced as the inverse of the cosine function, with a focus on its evaluation and the application of restrictions.

Mindmap
Keywords
💡Inverse Trigonometric Functions
Inverse trigonometric functions are mathematical operations that 'undo' the usual trigonometric functions (sine, cosine, tangent, etc.). They allow us to find the angle given the value of a trigonometric function. In the context of the video, these functions are crucial for solving problems where the value of a trigonometric function is known, but the angle is unknown.
💡Unit Circle
The unit circle is a circle with a radius of 1 centered at the origin (0,0) in the coordinate plane. It is fundamental in trigonometry as it helps define the trigonometric functions for all angles. In the video, the unit circle is used to visualize and understand the inverse trigonometric functions by identifying points on the circle that correspond to given trigonometric values.
💡Restrictions
In the context of inverse trigonometric functions, restrictions refer to the specific intervals or ranges of angles for which the inverse functions are defined. These restrictions ensure that the inverse functions are one-to-one, meaning each output has a unique input, which is necessary for them to be true inverses of the original trigonometric functions.
💡Sine Function
The sine function is a trigonometric function that relates the ratio of the opposite side to the hypotenuse in a right-angled triangle to the angle in that triangle. In the video, the sine function is used to illustrate how inverse trigonometric functions can be evaluated by finding angles that satisfy the equation sin(θ) = value.
💡Cosine Function
The cosine function is another trigonometric function that relates the ratio of the adjacent side to the hypotenuse in a right-angled triangle to the angle. The video uses the cosine function to demonstrate how to evaluate inverse cosine by finding the angle that corresponds to a given cosine value.
💡Tangent Function
The tangent function is a trigonometric function that represents the ratio of the opposite side to the adjacent side in a right-angled triangle, equivalent to the sine function divided by the cosine function. In the video, the tangent function is used to explain how to find angles from given tangent values using inverse trigonometric functions.
💡Cotangent Function
The cotangent function, often denoted as 'ctg' or 'cotan', is the reciprocal of the tangent function, equivalent to the cosine function divided by the sine function. It is used to find the angle when the ratio of the adjacent side to the opposite side is known. The video touches on cotangent in relation to restrictions and how it is treated similarly to the sine function.
💡Secant Function
The secant function, denoted as 'sec', is the reciprocal of the cosine function. It is used to find the angle when the ratio of the hypotenuse to the adjacent side in a right-angled triangle is known. The video advises rewriting secant-related equations in terms of cosine to understand and apply the restrictions associated with the cosine function.
💡One-to-One Functions
A function is said to be one-to-one, or injective, if each element of the function's range corresponds to exactly one element of its domain. This property is essential for inverse functions to be well-defined. The video emphasizes that by restricting the domain of trigonometric functions, they become one-to-one, allowing for the existence of unique inverse functions.
💡Horizontal Line Test
The horizontal line test is a method to determine if a function is one-to-one. If any horizontal line intersects the graph of the function more than once, the function is not one-to-one and therefore does not have a unique inverse. The video implies that without the restrictions on trigonometric functions, they would fail this test and not be invertible.
Highlights

The video aims to explain how to evaluate inverse trigonometric functions.

Understanding the origin and purpose of inverse trigonometric functions is crucial.

Four different examples are provided to illustrate the concept.

Inverse trigonometric functions are used to 'undo' trigonometric functions.

The sine function is used to find the angle when given a y-coordinate on the unit circle.

The sine of an angle equals the y-coordinate of the corresponding point on the unit circle.

The example of sine of theta equals square root of 3 over 2 is used to demonstrate the process.

The concept of coterminal angles and their infinite nature is discussed.

Restrictions on the sine function are explained to find the correct angle within the range of negative pi to pi.

The cosine function is explored with the example of cosine of what angle equals a negative value.

The cosine function is restricted between 0 and pi for inverse evaluation.

The tangent function's y over x ratio is discussed with an example.

Tangent is negative in the second and fourth quadrants, and the restrictions for the tangent function are explained.

The cotangent function is related to the tangent function, and its restrictions are aligned with the sine function.

The secant function is the inverse of the cosine function, and it is explained with a specific example.

The restrictions for sine, cosine, and tangent functions are summarized for clarity.

The importance of adhering to the restrictions to ensure the functions are one-to-one is emphasized.

The video provides a comprehensive review of inverse trigonometric functions and their application.

Transcripts

Browse More Related Video

Solving Trigonometric Equations By Finding All Solutions

Trig 0.2 - Trig Functions Defined on the Unit Circle

Quotient Identities - Evaluating Tangent and Cotangent Functions

How To Evaluate Trigonometric Functions Using Periodic Properties - Trigonometry

Limits of trigonometric functions | Limits and continuity | AP Calculus AB | Khan Academy

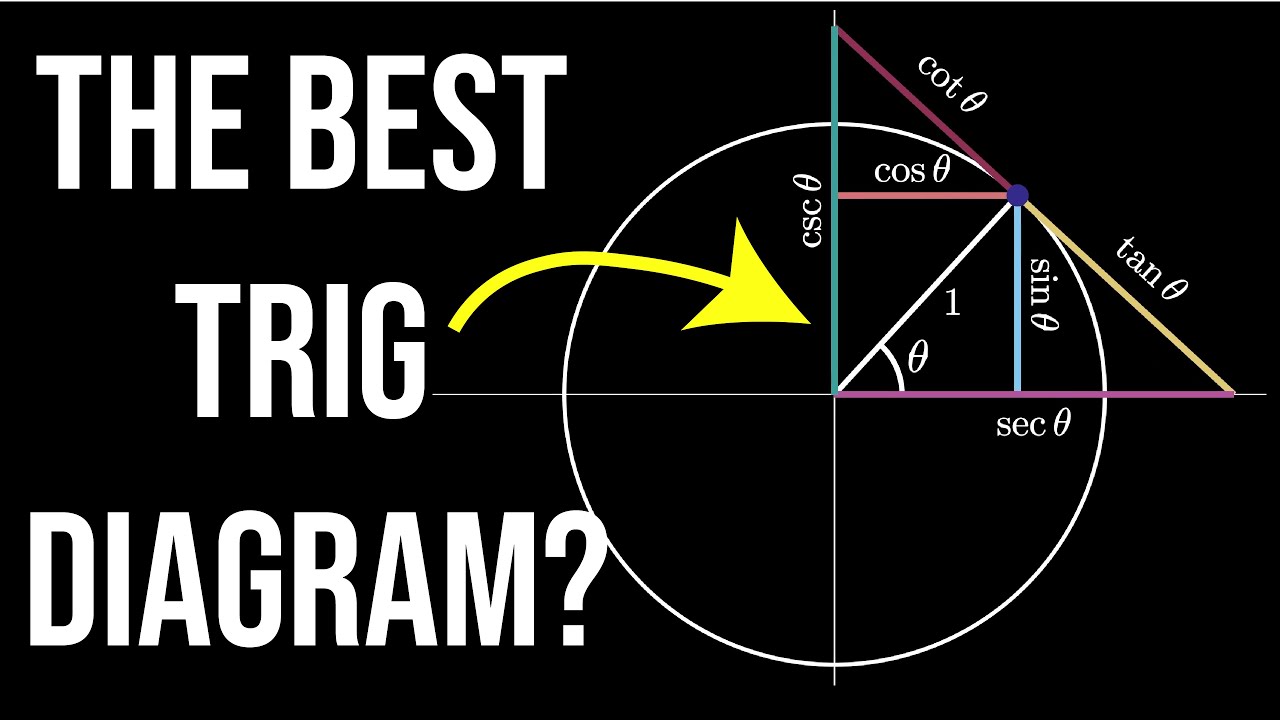
Trig Visualized: One Diagram to Rule them All (six trig functions in one diagram)

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Inverse TrigonometryTrigonometric FunctionsMathematics TutorialUnit CircleSine and CosineTangent and CotangentFunction RestrictionsEducational ContentMathematics EducationOnline Learning