Trigonometric Functions of Any Angle

ProfRobBob

9 Nov 201114:57

EducationalLearning

32 Likes 10 Comments

TLDRIn this informative video, Mr. Tarrou demonstrates how to set up trigonometric functions for unknown angles using a point on the terminal side. He explains the process with examples, including how to determine the quadrant of an angle based on the signs of trig functions. The video covers the use of sine, cosine, tangent, and their reciprocals, emphasizing the importance of understanding reference angles and the unit circle in trigonometry. Mr. Tarrou's approach to trigonometric functions without a calculator makes the content accessible and engaging for viewers seeking a deeper understanding of these mathematical concepts.

Takeaways

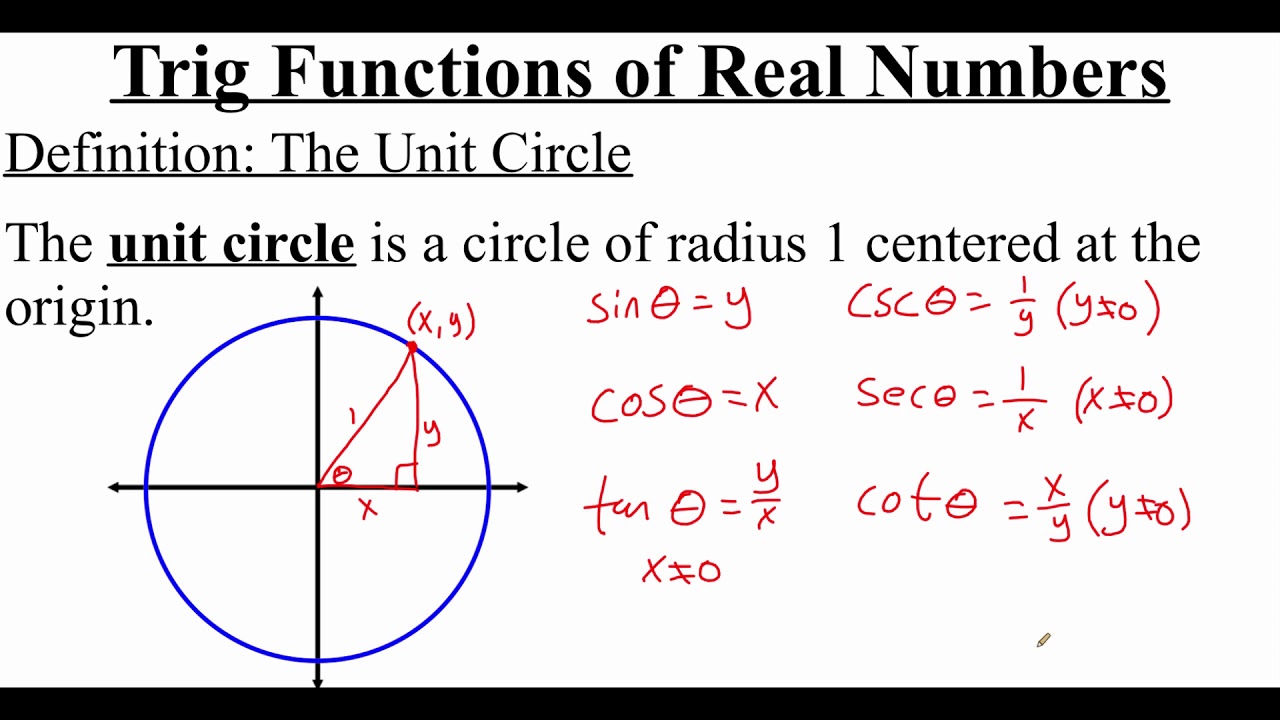
📐 The concept of trigonometric functions for unknown angles is introduced using the unit circle and standard position angles.
📈 The method for setting up trigonometric functions for a point in the coordinate plane, specifically in quadrant three, is demonstrated using the example of (-2, -5).
🤔 The importance of understanding the signs of x and y in relation to the quadrants for determining the values of trigonometric functions is emphasized.
🌟 The process of rationalizing the denominator in trigonometric function values to avoid square roots is explained.
🔍 The use of the Pythagorean theorem to find the hypotenuse (r) of the reference triangle is shown.
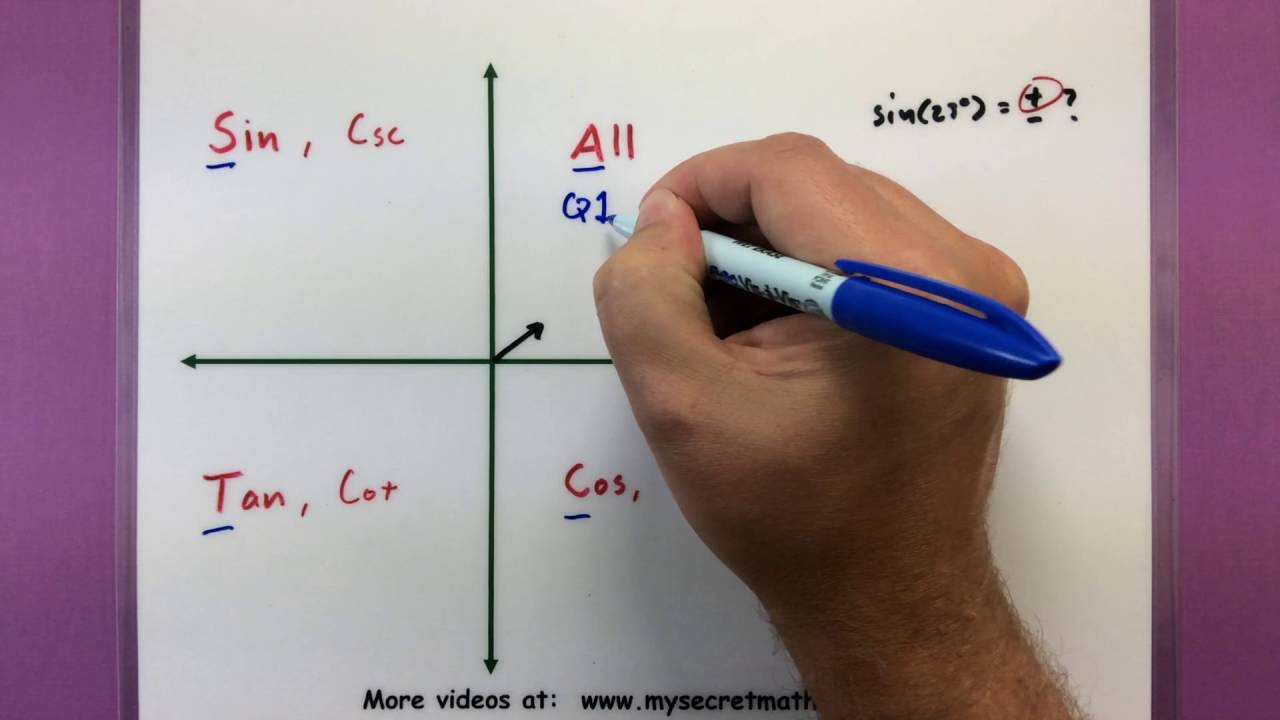
🧐 The method to determine the quadrant of an angle based on the signs of its trigonometric functions (cosine and cosecant) is discussed.
📌 The concept of reference angles and how they relate to the quadrants is clarified.
🔢 The process of finding the remaining trigonometric functions given one function (cosine in this case) and the quadrant is detailed.
🔄 The relationship between the angle of rotation and the reference angle is explained, highlighting the significance of the unit circle.
🎓 The educational nature of the content is highlighted, aiming to aid in the understanding of trigonometry without the use of a calculator.
🚀 The video script serves as an engaging and informative tool for learning about trigonometric functions and their applications.

Q & A

How can trigonometric functions be set up for angles with unknown measures?
-Trigonometric functions for unknown angles can be set up by using the coordinates of a point on the terminal side of the angle, forming a reference triangle, and applying trigonometric ratios and the Pythagorean Theorem.
What is the significance of the point (-2, -5) in setting up trig functions?
-The point (-2, -5) indicates the coordinates on the terminal side of an angle in standard position. These coordinates are used to determine the sine, cosine, and other trigonometric functions based on the reference triangle formed.
Why is it important to consider the quadrant where the point lies when setting up trig functions?
-The quadrant determines the signs of the x and y coordinates, which affect the signs of the trigonometric functions since these functions are based on ratios involving these coordinates.
How is the radius 'r' determined in the context of trigonometric functions?
-The radius 'r' is calculated using the Pythagorean Theorem, where 'r' is the hypotenuse of the reference right triangle formed by the x and y coordinates of the given point.
What method is used to rationalize the denominators in trigonometric functions?
-To rationalize the denominator, the numerator and denominator are multiplied by the square root that appears in the denominator. This eliminates the square root from the denominator.
How is the sine of an angle determined in this context?
-The sine of an angle is determined as the y-coordinate of the point on the terminal side divided by the radius 'r' of the reference triangle.
Why is the cosine of theta in the given example negative?
-The cosine of theta is negative because the x-coordinate of the point (-2, -5) is negative, and cosine is calculated as the x-coordinate over the radius.
How can you determine the quadrant of an angle based on the signs of its trigonometric functions?
-The quadrant can be determined by the signs of the cosine and cosecant functions. A negative cosine and positive cosecant suggest that the angle lies in the second quadrant.
What is the significance of a reference triangle in trigonometry?
-A reference triangle is a right triangle formed by the x-axis and a line from the origin to a point on the terminal side of an angle. It's used to determine the trigonometric functions of the angle.
How can you find the reference angle of a rotation?
-The reference angle is the acute angle formed between the terminal side of the angle and the nearest x-axis. It's found by considering the absolute difference in degrees between the terminal side and the x-axis.

Outlines

00:00

📚 Setting Up Trigonometric Functions for Unknown Angles

This paragraph introduces the concept of setting up trigonometric functions for angles when the angle itself is not known. The focus is on using the coordinates of a point on the terminal side of an angle to determine the values of the six trigonometric functions: sine, cosine, tangent, cosecant, secant, and cotangent. The example given involves a point at (-2, -5) and demonstrates how to use the Pythagorean theorem to find the radius (r) and subsequently calculate the trigonometric functions in a quadrant III scenario. The process emphasizes the importance of understanding the signs of x and y values in different quadrants and concludes with a call to action for viewers to engage with the content and spread the word about the math channel.

05:07

🔍 Identifying Quadrants Using Trig Function Signs

In this section, the method of deducing the quadrant of an angle based on the signs of its trigonometric functions is discussed. The example uses the given information that the cosine is negative and the cosecant is positive to determine the quadrant of the angle. The explanation relies on understanding the signs of x and y in different quadrants and the definitions of cosine and cosecant. The conclusion is that the angle must be in quadrant II, as this is the only quadrant where x is negative (cosine is negative) and y is positive (cosecant is positive). The paragraph also touches on the concept of reference triangles and their role in evaluating trig functions without the actual measure of the angle.

10:11

📐 Further Exploration of Trig Functions and Quadrants

This paragraph delves deeper into the relationship between trigonometric functions and the quadrants in which angles reside. It presents a problem involving the tangent of theta being positive and the sine of theta being negative, leading to the determination that the angle is in quadrant III. The explanation involves creating a reference triangle and applying the Pythagorean theorem to find the sides and subsequently the trig functions. The paragraph also discusses the concept of reference angles, their importance in trigonometry, and how they relate to the unit circle. The content is wrapped up with a reminder of the importance of understanding these concepts and an encouragement for viewers to practice their homework.

Mindmap

Keywords

💡Trigonometric functions

Trigonometric functions are mathematical functions that relate the angles of a right triangle to the ratios of its sides. In the video, these functions are used to determine the values of sine, cosine, tangent, and their reciprocals for given angles. They are essential for solving problems involving right triangles and the rotation of points on a unit circle.

💡Unit circle

A unit circle is a circle with a radius of 1, centered at the origin of a coordinate plane. It is used in trigonometry to define the trigonometric functions for all angles, not just those in a right triangle. The video uses the unit circle concept to discuss angles and their corresponding trigonometric function values.

💡Reference triangle

A reference triangle is a right triangle used as a standard for determining the values of trigonometric functions for a given angle. It is set up based on the coordinates of a point on the terminal side of the angle and helps in visualizing the relationship between the angle and its trigonometric functions.

💡Quadrant

In a Cartesian coordinate system, the quadrants are the four regions defined by the intersection of the positive and negative x and y axes. Each quadrant is characterized by the signs of the coordinates of the points within it. Quadrants are crucial in determining the signs of trigonometric function values based on the angle's location.

💡Pythagorean theorem

The Pythagorean theorem states that in a right triangle, the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the lengths of the other two sides. It is used to find the length of one side of a right triangle when the lengths of the other two sides are known.

💡Rationalize

To rationalize a denominator in a fraction means to eliminate the radical (such as a square root) from the denominator, often by multiplying both the numerator and the denominator by a form of the conjugate of the denominator. This is done to simplify expressions and to ensure that the denominator does not contain radicals.

💡Sine (sin)

The sine of an angle in a right triangle is the ratio of the length of the side opposite the angle to the length of the hypotenuse. It is one of the fundamental trigonometric functions and is used to find the y-coordinate of a point on the terminal side of an angle.

💡Cosine (cos)

The cosine of an angle in a right triangle is the ratio of the length of the adjacent side to the length of the hypotenuse. It is another fundamental trigonometric function and is used to find the x-coordinate of a point on the terminal side of an angle.

💡Tangent (tan)

The tangent of an angle is the ratio of the length of the side opposite the angle to the length of the adjacent side. It is a trigonometric function used to find the slope of the terminal side of an angle with respect to the x-axis.

💡Cosecant (csc)

The cosecant of an angle is the reciprocal of the sine function, representing the ratio of the hypotenuse to the side opposite the angle. It is used to find the y-coordinate in terms of the hypotenuse when the angle is known.

💡Secant (sec)

The secant of an angle is the reciprocal of the cosine function, representing the ratio of the hypotenuse to the side adjacent to the angle. It is used to find the x-coordinate in terms of the hypotenuse when the angle is known.

💡Reference angle

The reference angle is the acute angle formed by the terminal side of an angle and the x-axis in standard position. It is used to determine the quadrant in which the angle lies and to find the trigonometric function values for the original angle based on those of the reference angle.

Highlights

Introduction to setting up trigonometric functions for unknown angles using the unit circle and reference triangles.

Explanation of sine, cosine, and other trig functions in the context of a right triangle with a point on the terminal side of (-2, -5).

Demonstration of how to determine the quadrant of an angle based on the signs of its trigonometric functions.

Procedure for rationalizing the denominator in trigonometric expressions to avoid square roots.

Discussion on how to find the quadrant of an angle when given the cosine is negative and the cosecant is positive.

Method for calculating the reference triangle and trig functions for a given angle in a specific quadrant.

Explanation of the relationship between the signs of x and y coordinates and the quadrant in which the angle lies.

Process of using the Pythagorean theorem to find the hypotenuse of a reference triangle.

Detailed example of finding the sine and tangent of an angle in quadrant two given the cosine value.

Clarification on the concept of reference angles and their significance in trigonometry.

Explanation of how to determine the quadrant of an angle given the signs of its sine and tangent functions.

Procedure for sketching a reference triangle in a specific quadrant based on the signs of trigonometric function values.

Discussion on the importance of understanding reference angles in the context of the unit circle.

Illustration of how to calculate trig functions for an angle in quadrant three given the tangent value.

Explanation of how to find the reference angle when given a rotation in degrees.

Conclusion emphasizing the practical application of these methods in solving trigonometric problems without knowing the exact angle measure.

Transcripts

Browse More Related Video

Signs of Trigonometric Function Values

Trigonometric Functions of Any Angle - Unit Circle, Radians, Degrees, Coterminal & Reference Angles

Finding Exact Values of Trig Functions

Trigonometry - The signs of trigonometric functions

Trigonometric Functions and the Unit Circle (Precalculus - Trigonometry 6)

4.3.4 Trigonometric Functions of Real Numbers