AP Calculus AB: Lesson 6.6 Fundamental Theorem of Calculus, Part 2
TLDRIn this lesson, Michelle Crummel explores the second part of the Fundamental Theorem of Calculus, demonstrating how to find antiderivatives and their derivatives, and how these operations relate to the original functions. She uses various functions to illustrate the process, including polynomials and trigonometric functions, and explains how to handle definite integrals with variable limits. The lesson also covers the application of the Fundamental Theorem to evaluate definite integrals and find derivatives of integrals, including examples with constant and variable bounds, and concludes with practice problems to solidify understanding.
Takeaways
- 📚 The lesson focuses on the second part of the Fundamental Theorem of Calculus, which involves finding the derivative of a definite integral.
- 🔍 The process demonstrates how to find the antiderivative of a function and then take the derivative of that antiderivative, which should result in the original function.
- 📈 The script provides examples of how to work with functions and their antiderivatives, including the use of power rules and substitution.
- 📝 The importance of understanding the constant of integration 'C' when rewriting antiderivatives in terms of a different variable is highlighted.
- 🤔 The script explains how to apply the chain rule when the limits of integration are variable expressions, not just constants.
- 📉 The concept of definite integrals is used to evaluate areas under curves, which are then related to the function's values at specific points.
- 📌 The script shows how to use the first part of the Fundamental Theorem of Calculus to evaluate definite integrals by substituting the limits of integration.
- 🔢 The shortcut method for finding the derivative of a definite integral is introduced, which simplifies the process by directly substituting the upper limit of integration.
- 📚 The lesson also covers how to handle definite integrals with variable bounds, using both the shortcut method and the traditional substitution approach.
- 📉 The application of the second part of the Fundamental Theorem of Calculus is demonstrated through various examples, including those with complex functions and bounds.
- 🔍 The script concludes with practice problems that reinforce the concepts taught, encouraging students to apply what they've learned to new scenarios.
Q & A
What is the main topic of the video lesson?
-The main topic of the video lesson is the second part of the Fundamental Theorem of Calculus.
What is the antiderivative of the function f(t) = 4t - t^2?
-The antiderivative, denoted as F(t), is F(t) = 2t^2 - t^3/3 + C, where C is the constant of integration.
If the antiderivative of f(t) is found, how can you find the derivative of the antiderivative?
-You can find the derivative of the antiderivative by applying the power rule to the antiderivative function and then substituting the variable back in, which in this case results in F'(x) = 4x - x^2.
What does the second part of the Fundamental Theorem of Calculus state?
-The second part of the Fundamental Theorem of Calculus states that if F(x) is the antiderivative of f(t), then the derivative of F(x) with respect to x is equal to f(x), i.e., F'(x) = f(x).
How does the process of taking the derivative of an antiderivative relate to the original function?
-Taking the derivative of an antiderivative essentially 'undoes' the integration process, bringing you back to the original function, except now in terms of the new variable.
What is the antiderivative of f(t) = cos(t), and what is its derivative?
-The antiderivative of f(t) = cos(t) is F(t) = sin(t) + C. The derivative of F(t) is F'(t) = cos(t), which is the original function.
Can you explain the process of finding the derivative of a definite integral with variable limits?
-To find the derivative of a definite integral with variable limits, you substitute the upper limit of integration into the integrand, multiply by the derivative of the upper limit, and then subtract the result of doing the same with the lower limit of integration.
What is the shortcut method for finding the derivative of a definite integral?
-The shortcut method involves substituting the upper limit of integration for the variable of integration in the integrand, multiplying by the derivative of the upper limit, and ignoring the lower limit if it's a constant, as its derivative is zero.
How does the Fundamental Theorem of Calculus apply to definite integrals with variable expressions as limits?
-When the limits of integration are variable expressions, the derivative of the definite integral is found by applying the chain rule to the upper and lower limits of integration, multiplying the integrand evaluated at the limits by the derivatives of those limits.
What is the significance of the second derivative in the context of the Fundamental Theorem of Calculus?
-The second derivative of a function defined as a definite integral can provide information about the concavity of the original function, as it is equivalent to the first derivative of the integrand.
Can you provide an example of how to find the second derivative of a function defined by a definite integral?
-To find the second derivative, you first take the derivative of the function defined by the definite integral to get the first derivative, and then take the derivative of that result to get the second derivative. For example, if F(x) = ∫(a to x) f(t) dt, then F''(x) = f'(x), the first derivative of the integrand.
Outlines
📚 Introduction to the Fundamental Theorem of Calculus, Part 2
In this segment, Michelle Crummel introduces the second part of the Fundamental Theorem of Calculus. She explains the concept of antiderivatives and demonstrates how to find the antiderivative of a given function, using the reverse power rule. Michelle then shows how to substitute variables to rewrite the antiderivative in terms of a different variable and proceeds to illustrate the process of taking the derivative of an antiderivative, which results in the original function. This is followed by examples using different functions, such as cosine of t and t cubed, to further clarify the theorem's application. Michelle emphasizes the relationship between the derivative of the antiderivative and the original function.
🔍 Applying the Fundamental Theorem to Definite Integrals
This paragraph delves into applying the second part of the Fundamental Theorem of Calculus to definite integrals. Michelle explains how to evaluate a definite integral using the antiderivative function and then demonstrates how taking the derivative of a definite integral results in the original function, with the constant term disappearing. She uses the chain rule to show how to handle definite integrals with variable limits of integration and simplifies the process using a shortcut. Michelle also explains how to deal with situations where the lower limit of integration is a constant, which simplifies the derivative calculation.
📉 Understanding Increasing and Decreasing Intervals with Definite Integrals
In this section, Michelle explores how to determine the intervals on which a function defined by a definite integral is increasing or decreasing. She explains that the function is increasing where its derivative is positive and decreasing where the derivative is negative. Michelle uses the graph of a function to illustrate this concept and provides a step-by-step guide on how to identify these intervals based on the function's values and the x-axis. She also touches on the concept of concavity, explaining how to determine where the function is concave up or down by examining the sign of the second derivative.
📈 Calculating Areas and Derivatives from Graphs
Michelle continues the lesson by showing how to calculate areas under a curve from a given graph and how to determine derivatives at specific points. She uses the graph of a function to find the definite integral's value at certain points, which corresponds to the area under the curve. She also explains how to find the first and second derivatives of a function at given values by using the fundamental theorem of calculus and the concept of the derivative. Michelle demonstrates this with examples, including reading values from the graph and applying the chain rule to find the second derivative.
📘 Comprehensive Examples of Calculus Applications
This paragraph presents a series of comprehensive examples that apply the concepts of the fundamental theorem of calculus, including finding the antiderivative, evaluating definite integrals, and calculating derivatives. Michelle works through problems that involve various functions and intervals, showing how to compute the areas under the curve and how to find the function's values and derivatives at specific points. She also addresses the implications of integrating over intervals that proceed from right to left, which affects the sign of the calculated areas.
📚 Summary of the Second Fundamental Theorem of Calculus
In the concluding segment, Michelle summarizes the key points covered in the lesson about the second part of the Fundamental Theorem of Calculus. She reiterates the process of finding antiderivatives, evaluating definite integrals, and calculating derivatives of these integrals. Michelle also highlights the importance of understanding how to work with variable limits of integration and the significance of the theorem in calculus. She wraps up the lesson by previewing the next topic, which is u-substitution, and thanks the viewers for joining the session.
Mindmap
Keywords
💡Fundamental Theorem of Calculus
💡Antiderivative
💡Derivative
💡Power Rule
💡Constant of Integration
💡Definite Integral
💡Chain Rule
💡Variable Limits of Integration
💡Second Derivative
💡Concavity
💡U-Substitution
Highlights
Introduction to the second part of the Fundamental Theorem of Calculus.
Finding the antiderivative of a given function using the reverse power rule.
Substituting x for t to rewrite the antiderivative in terms of x.
Deriving the relationship between the derivative of the antiderivative and the original function.
Demonstrating the process with a new function, f(t) = cosine(t), and its antiderivative.
Using the chain rule to find the derivative of an antiderivative with variable limits of integration.
Applying the first part of the Fundamental Theorem of Calculus to evaluate definite integrals.
Explaining the shortcut for finding the derivative of a definite integral without intermediate steps.
Generalizing the process for variable expressions as limits of integration.
Practical examples of taking the derivative of definite integrals with constant and variable bounds.
Illustrating the method with a function defined as an integral and finding its values at specific points.
Finding the first and second derivatives of functions defined by integrals using the Fundamental Theorem of Calculus.
Determining the intervals where a function defined by an integral is increasing or decreasing.
Analyzing the concavity of a function by examining the sign of its second derivative.
Using a combination of algebraic and integral terms to define a function and finding its derivative.
Concluding the lesson with a summary of the key points and a preview of the next topic, u-substitution.
Transcripts
Browse More Related Video

AP Calculus AB: Lesson 6.3 Fundamental Theorem of Calculus Part 1
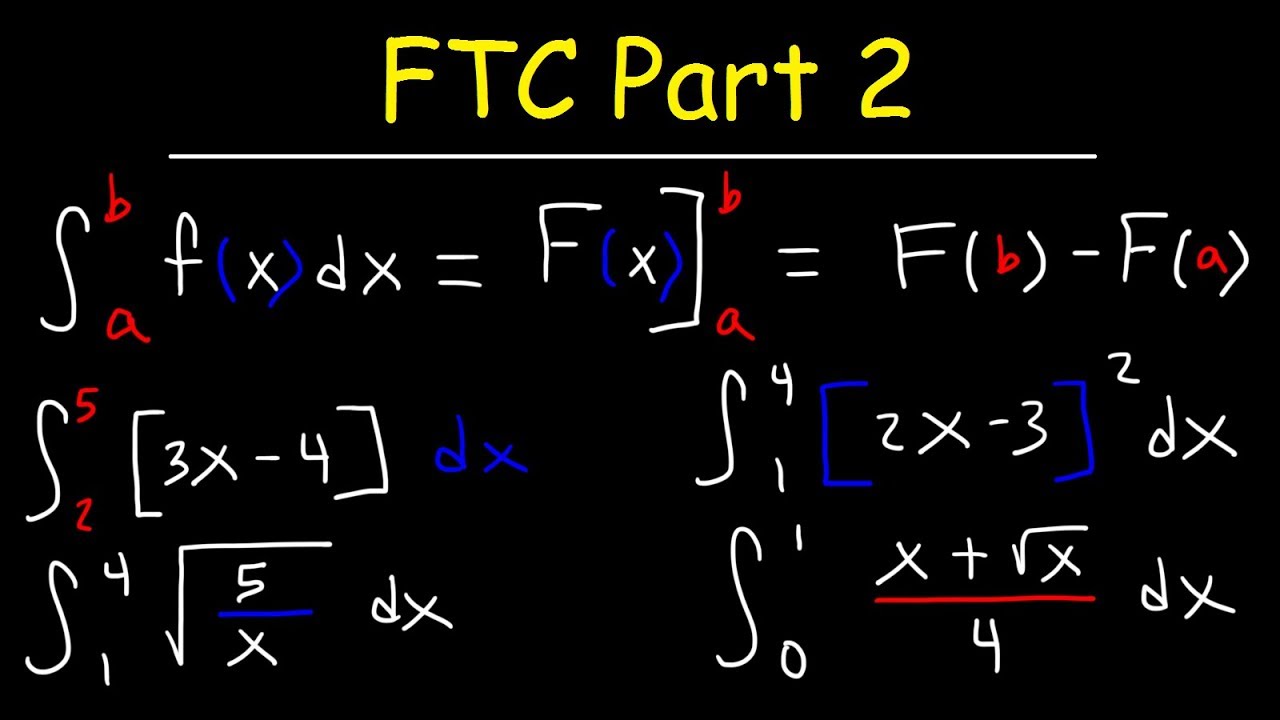
Fundamental Theorem of Calculus Part 2

Calculus AB Homework 6.6: Fundamental Theorem of Calculus Part II
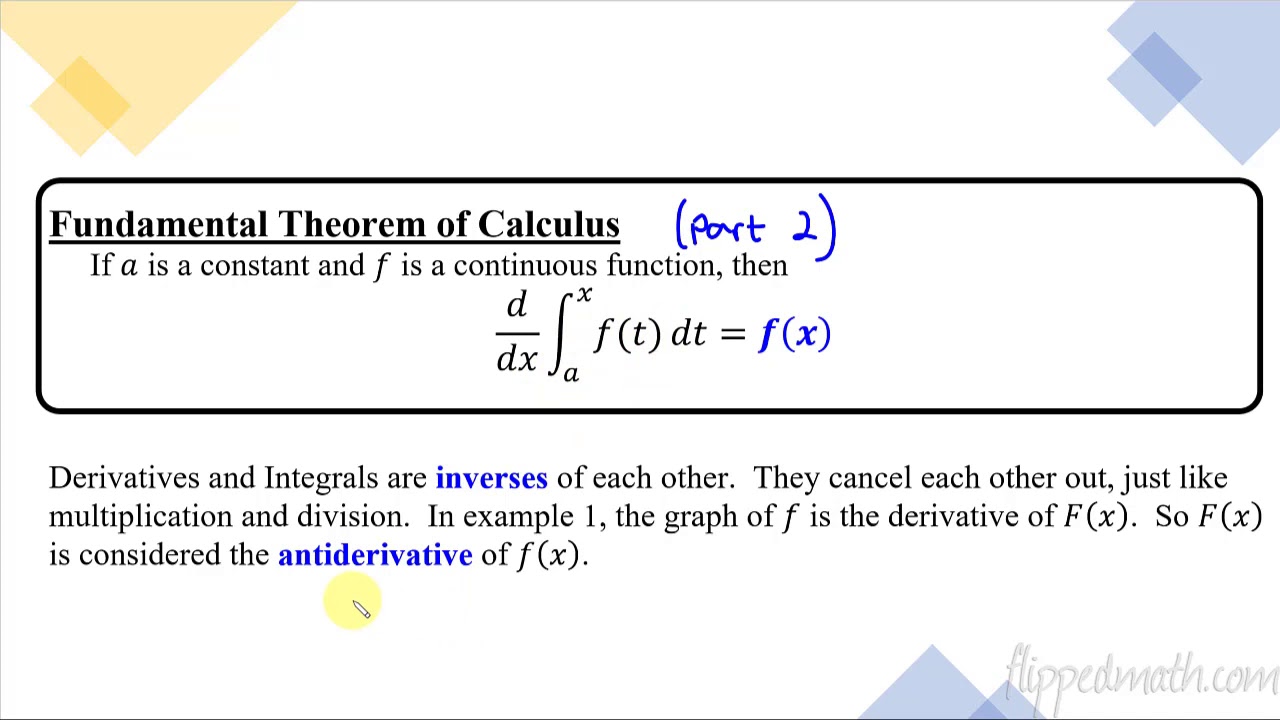
Calculus AB/BC – 6.4 The Fundamental Theorem of Calculus and Accumulation Functions
Calculus AB Homework 6.3 Fundamental Theorem of Calculus, Part I
Improper Integrals
5.0 / 5 (0 votes)
Thanks for rating: