Calculus AB Homework 6.6: Fundamental Theorem of Calculus Part II

Michelle Krummel

25 Feb 201825:15

EducationalLearning

32 Likes 10 Comments

TLDRThis video tutorial guides viewers through solving calculus homework problems involving derivatives of integrals. It demonstrates the use of the fundamental theorem of calculus to simplify the process, showing step-by-step solutions for problems 46 to 55. The script covers various integrals, including definite integrals with variable limits, and applies shortcuts to find derivatives efficiently. It also explores second derivatives and uses graphical analysis to find function values and slopes at specific points, offering a comprehensive understanding of calculus concepts.

Takeaways

📚 The video is a tutorial focusing on solving calculus problems, specifically finding derivatives of integrals, which are often simplified by the fundamental theorem of calculus.
🔍 The script explains that the derivative of an antiderivative cancels out, but when dealing with definite integrals, the process involves substituting the limits of integration and multiplying by their respective derivatives.
📝 For problem 46, the shortcut method is demonstrated by substituting the upper limit of integration (3x) into the integrand, multiplying by the derivative of the upper limit, and similarly for the lower limit (2), resulting in a simplified expression for the derivative.
📈 In problem 47, the derivative of an integral with a variable upper limit is found by substituting the upper limit into the integrand, multiplying by the derivative of the upper limit, and ignoring the lower limit's derivative as it is zero.
📉 Problem 48 involves a constant upper limit of integration, and the derivative is found by substituting the lower limit into the integrand and multiplying by its derivative, with the upper limit's contribution being zero.
🔢 The script provides a step-by-step solution for problem 49, showing the process of substituting the lower limit of integration into the integrand, distributing the negative sign, and simplifying to find the derivative.
📉 For problem 50, the derivative of an integral with a trigonometric function as the upper limit involves substituting the upper limit into the integrand and multiplying by the derivative of the trigonometric function, with the lower limit's contribution being zero.
📚 The video also covers how to find higher-order derivatives, such as in problem 51, where the derivative of an integral involving an exponential function is found by using the chain rule and constant multiple rule.
📉 In problem 53, the process of finding the second derivative of an integral is demonstrated, which involves using the product rule and understanding the derivative of a square root function.
📊 The script explains how to interpret the graph of a function to find the value of an integral and its derivative at specific points, as shown in problems 54 and 55.
📝 The final part of the script involves calculating areas under a curve from a graph to find the value of an integral at specific points, demonstrating the application of integral calculus in real-world scenarios.

Q & A

What is the primary goal of the video?
-The primary goal of the video is to work through Unit 6 homework problems 46 through 55.
What is the integral given in problem 46, and how is its derivative found?
-The integral given in problem 46 is an integral of 2t + 3. Its derivative is found by substituting the upper limit 3x into the integral, multiplying by the derivative of 3x, and subtracting the result of substituting the lower limit 2, which results in 18x + 9.
What technique is used to find H' of X in problem 47?
-In problem 47, the upper limit of integration X^4 is substituted for T, and then multiplied by the derivative of X^4, which simplifies to 12x^5.
How is the derivative handled when the upper limit of integration is a constant in problem 48?
-When the upper limit of integration is a constant in problem 48, the result is 0. The lower limit 2x is then substituted in for T, and multiplied by the derivative of 2x, resulting in -8x^2 - 8x.
What steps are taken to find H' of X in problem 49?
-To find H' of X in problem 49, cosine X is substituted in for T and multiplied by the derivative of cosine X, which is negative sine X, resulting in -2 cosine^2(X) sin(X).
Describe the process to find P' of X in problem 50.
-In problem 50, x^2 + 2x is substituted in for T and multiplied by the derivative of x^2 + 2x. After simplifying and distributing, the final result is 6x^3 + 18x^2 + 8x - 4.
How is F' of X determined in problem 51?
-In problem 51, the lower limit ln(X) is substituted into the integral, multiplied by the derivative of ln(X), and simplified to -1 - ln(X)/X.
What method is used to find G' of X and G'' of X in problem 52?
-G' of X is found by substituting X into the integral and multiplying by the derivative, resulting in X^3 e^X. G'' of X is found using the product rule, resulting in 4e for G''(1).
How is H' of X and H'' of 1 found in problem 53?
-H' of X is found by substituting x^2 into the integral, multiplying by the derivative of x^2, and simplifying. H'' of 1 is found using the product rule, resulting in -6√2.
What is the approach to find the value of G(3) in problem 55?
-G(3) is found by calculating the area under the curve of the graph of f(T) from -2 to 3, which involves integrating and summing the areas of various geometric shapes under the curve, resulting in π - 11.

Outlines

00:00

📚 Calculus Homework: Derivatives of Integrals

This paragraph introduces a calculus video tutorial focusing on solving homework problems involving the derivative of integrals. The instructor explains the concept of antiderivatives and definite integrals, using the fundamental theorem of calculus to simplify the process. The tutorial covers problems 46 to 55, starting with finding the derivative of an integral of '2t + 3' from 2 to 3x, using substitution and the properties of derivatives to simplify the calculation. The explanation includes step-by-step processes for each problem, emphasizing the shortcut of substituting the limits of integration and multiplying by their derivatives to find the derivative of the integral directly.

05:01

🔍 Advanced Derivatives of Definite Integrals

The second paragraph continues the calculus lesson, delving into more complex problems involving the derivative of definite integrals with variable limits. The examples include integrals with functions such as '3√T', 'T^2 + 2T', and 'e^(T+T)'. The instructor demonstrates the use of substitution for the upper and lower limits of integration, the application of the derivative of these limits, and simplification techniques. The summary also touches on the derivative of a function defined as an integral, showcasing the process of finding 'H' and 'F' prime of 'X' for given integrals.

10:04

📈 Derivatives of Integrals with Trigonometric Functions

In this segment, the focus shifts to integrals involving trigonometric functions, specifically the derivative of an integral with '2t^2' from '−5' to 'cos(x)'. The instructor clarifies the process of substituting the upper limit of integration and multiplying by its derivative, which in this case is the derivative of 'cos(x)', resulting in '−2sin(x)cos^2(x)'. The explanation also covers the derivative of an integral with a constant upper limit and a variable lower limit, illustrating the steps with the integral of 'T^2 + 2T' from '2x' to '−1'.

15:04

📘 Derivatives of Integrals: Exponential and Logarithmic Functions

The fourth paragraph presents problems involving the derivative of integrals with exponential and logarithmic functions. The video script explains how to find the derivative of an integral of 'e^(T+T)' from 'ln(x)' to '2', simplifying the process by substituting the limits of integration and their derivatives. The explanation includes the simplification of 'e^(ln(x))' to 'x' and the application of the chain rule and constant multiple rule to find the second derivative of 'G' at '1'.

20:04

📊 Area Calculations from Graphs and Derivatives

This paragraph discusses the process of calculating areas under a curve from a given graph and using these to find the value of a function defined as an integral. The video script provides a step-by-step method for finding 'big f of 0' and 'big f of negative 1/2' by integrating 'little f of T' from '−6' to '0' and '−6' to '1', respectively. It also explains how to find 'big f prime of negative two' by substituting '−2' into the derivative of the integral and reading the value from the graph. The explanation concludes with finding 'big f double prime of 0' using the chain rule and constant multiple rule.

📐 Derivatives and Integrals from Graphs: Geometric Interpretation

The final paragraph wraps up the calculus lesson with a geometric interpretation of derivatives and integrals using a graph of 'little F of T'. It demonstrates how to find the value of 'G of 3' by calculating the area under the curve from '−2' to '3' and explains the process of finding 'G of negative four' by considering the integral in the reverse order. The paragraph also covers finding 'G prime of negative two' by taking the derivative of the integral and reading the value directly from the graph. Finally, it discusses finding 'G double prime of negative five' by determining the slope of the function at that point.

Mindmap

Keywords

💡Derivative

The derivative is a fundamental concept in calculus representing the rate at which a function changes at a given point. In the context of the video, the derivative of an integral (anti-derivative) is discussed, which is a process that essentially 'undoes' the integration, as seen in the explanation of problem 46 where the derivative of the integral of 2t + 3 from 2 to 3x is calculated.

💡Definite Integral

A definite integral is the calculation of the area under a curve between two points on the x-axis. The video discusses finding the derivative of a definite integral, which involves using the fundamental theorem of calculus and substituting the upper and lower limits of integration, as demonstrated in the problems involving functions G, H, and F.

💡Anti-derivative

An anti-derivative, also known as an indefinite integral, is a function that is the reverse process of taking a derivative. The video script explains that finding the derivative of an anti-derivative will cancel out, leaving the original function, which is a key concept in the problems presented.

💡Substitution

Substitution is a method used in calculus to simplify the process of integration or differentiation. In the video, substitution is used to replace the variable of integration with the variable's expression in terms of the upper and lower limits, as shown in the shortcut method for finding the derivative of an integral.

💡Chain Rule

The chain rule is a fundamental principle in calculus for differentiating composite functions. While not explicitly named in the script, the concept is applied when finding the second derivative of functions like G and H, where the derivative of the outer function and the derivative of the inner function are multiplied together.

💡Product Rule

The product rule is used to differentiate the product of two functions. In the script, the product rule is mentioned when finding the second derivative of functions, where the derivative of one part of the product is multiplied by the other part, and vice versa, as seen in the explanation of G double prime of X.

💡Integration by Parts

Integration by parts is a specific method for integrating products of functions. Although not directly mentioned in the script, the concept is alluded to when discussing the shortcut for finding the derivative of an integral, which is a form of integration by parts in reverse.

💡Constant Multiple Rule

The constant multiple rule states that the derivative of a constant times a function is the constant times the derivative of the function. This rule is applied when differentiating expressions that involve constants multiplied by functions, as seen in the calculation of F double prime of X.

💡Graph Interpretation

Graph interpretation involves understanding the graphical representation of functions and their properties. In the video, the script uses the graph of a function f(t) to find areas under the curve and to determine values of integrals and derivatives, as demonstrated in problems 54 and 55.

💡Slope

Slope is a measure of the steepness of a line and, in calculus, it corresponds to the derivative of a function at a certain point. The script discusses finding the slope of a function at specific points, such as G prime of negative 2 and G double prime of negative 5, by interpreting the graph or using the derivative of the function.

Highlights

The video covers unit six homework problems 46 through 55, focusing on finding derivatives of integrals.

Derivative of an integral and integral of a derivative are inverse operations, simplifying the process.

A shortcut for definite integrals involves substituting the upper limit of integration and multiplying by its derivative.

For problem 46, the derivative of an integral of 2t + 3 from 2 to 3x results in 18x + 9.

Problem 47 uses the integral of 3√t dt from -2 to x^4, resulting in 12x^5.

In problem 48, the integral of t^2 + 2t dt from 2x to -1 simplifies to -8x^2 - 8x.

Problem 49 involves the integral of 2t^2 dt from -5 to cos(x), yielding -2 cos^2(x) sin(x).

For problem 50, the integral of 3t - 2 dt from 2 to x^2 + 2x is simplified to 6x^3 + 18x^2 + 8x - 6.

Problem 51 demonstrates that the derivative of an integral with a constant upper limit results in zero.

Problem 52 explores finding G'(1) and G''(1) for an integral involving t^3 * e^t from 0 to x.

Problem 53 involves the integral of √(1 + t) to the fourth power dt from x^2 to 2, with H'(X) and H''(1) calculated.

Problem 54 requires finding f(0) and f'(-2) using the graph of f(t) and the integral from -6 to 2x.

Problem 55 involves calculating G(3), G(-4), G'(-2), and G''(-5) using the integral from -2 to x of f(t) dt.

The video explains how to find the area under a curve using integrals and graphical interpretation.

The importance of considering the sign of the area when calculating definite integrals is emphasized.

The video demonstrates the use of the chain rule and constant multiple rule in finding derivatives of integrals.

The process of finding second derivatives of integrals using the product rule is shown.

The video concludes with practical applications of integrals and derivatives in solving calculus problems.

Transcripts

Browse More Related Video

AP Calculus AB: Lesson 6.6 Fundamental Theorem of Calculus, Part 2

Calculus AB Homework 4.5: Second Derivative Test

Calculus AB Homework 6.3 Fundamental Theorem of Calculus, Part I

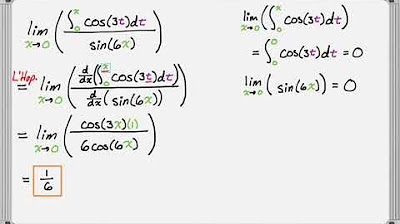
Evaluating Limits with L’Hopital’s Rule and the Second Fundamental Theorem of Calculus

AP Calculus BC 2003 Multiple Choice (calculator) - Questions 76 - 92

Calculus AB Homework 3.2 The Product Rule

Related Tags

Calculus Homework Integral Solutions Derivative Walkthrough Mathematics Education Definite Integrals Antiderivatives Shortcut Methods Calculus Techniques Educational Content Math Tutorial Problem Solving