Calculus AB Homework 6.3 Fundamental Theorem of Calculus, Part I
TLDRThis instructional video guides viewers through solving a series of calculus problems, focusing on the Fundamental Theorem of Calculus. It demonstrates how to find antiderivatives and evaluate definite integrals for various functions, including polynomials and trigonometric expressions. The video also covers techniques for calculating integrals using a graph, and employs properties of integrals to simplify complex problems. Additionally, it explores the relationship between the integral of a function's derivative and the function's values at given points.
Takeaways
- 📚 The video covers unit six homework problems 20 through 34, focusing on the Fundamental Theorem of Calculus.
- 🔍 Problem 20 involves evaluating the integral of T^2 - T from -1 to 1, resulting in the answer 2/3.
- 📉 For problem 21, the integral of 3/x^2 - 1 from 1 to 2 is simplified using the power rule and results in 1/2.
- 📈 Problem 22's integral of u - 2 over the square root of u from 1 to 4 is simplified and solved to get 2/3.
- ➡️ In problem 23, the integral of x - 1/x from -1 to -2 is solved by rewriting the expression in polynomial form, yielding 2.
- 🌟 Problem 24 integrates 1 + sin(x) from 0 to pi and uses the antiderivative properties to find the answer pi + 2.
- 📚 Problem 25 integrates 3x^2 + 5x - 4 from 1 to 3, applying the power rule to get the final answer of 38.
- 📊 The subsequent problems involve using the graph of a function to find areas under the curve for various intervals.
- 📐 The video explains how to use geometric shapes to find areas under the curve when the function's equation is not given.
- 🔄 The Fundamental Theorem of Calculus is applied to find the definite integrals when the derivative of a function is given.
- 📘 The properties of definite integrals are discussed, including how to handle addition, subtraction, and constant multiples.
Q & A
What does the video cover in terms of calculus problems?
-The video covers Unit Six homework problems 20 through 34, which deal with the Fundamental Theorem of Calculus.
How does one find the antiderivative of T squared and negative T?
-The antiderivative of T squared is T cubed over 3, and the antiderivative of negative T is T squared over 2, using the reverse power rule.
What is the process for evaluating the definite integral from negative 1 to 1 for the function T squared minus T?
-Substitute the upper limit (1) and lower limit (-1) into the antiderivative, then subtract the lower limit result from the upper limit result to find the definite integral.
How does the video demonstrate checking the answer for problem 20 using a calculator?
-The video shows two methods: entering the function into Y1 and using math 9 to evaluate the integral, or directly entering the bounds and function into math 9 without storing the function in Y1.
What is the antiderivative of 3 over x squared minus 1?
-The antiderivative is 3 times x to the negative 1 divided by negative 1 minus x, which simplifies to -3x to the negative 1 minus x.
How is the integral of u minus 2 over the square root of u simplified before finding the antiderivative?
-The integral is split into u over square root of u and 2 over square root of u, which simplifies to u to the one-half minus 2 times square root of u.
What is the final answer for the integral of x minus one over x squared from negative 1 to negative 2?
-The final answer is 2, after evaluating the antiderivative at the bounds and considering the direction of integration.
How does the video approach the problem of integrating 1 plus sine x from 0 to PI?
-The antiderivative of 1 is x, and the antiderivative of sine x is negative cosine x. The integral is then evaluated by substituting the upper and lower bounds.
What strategy is used to find the integral of a function from its graph?
-The strategy involves breaking the area under the curve into recognizable geometric shapes, calculating the areas of these shapes, and summing them to find the definite integral.
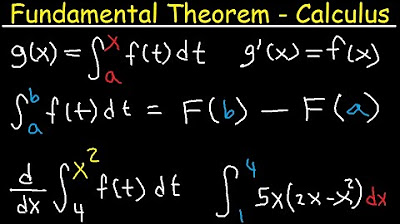
How does the video explain the use of the Fundamental Theorem of Calculus to find the value of f(x) from its derivative?
-The video explains that the integral of the derivative function (f'(x)) from a to b is equal to f(b) - f(a), which allows for finding the value of f(x) at a specific point by evaluating the definite integral of the derivative.
What properties of definite integrals are used to find the values of integrals when given the integrals of other functions?
-The video demonstrates using properties such as splitting integrals, constant multiple, and reversing bounds to find the values of new integrals based on given integrals.
How does the video handle the case where the integral of a function divided by another function is asked?
-The video clarifies that properties allowing for splitting integrals apply to addition and subtraction but not to multiplication or division unless it's by a constant, thus such integrals cannot be determined with the given information.
Outlines
📚 Calculus Homework Solutions
This paragraph discusses solving calculus homework problems from unit six, specifically numbers 20 to 34. The focus is on the Fundamental Theorem of Calculus. For problem 20, the integral of \( T^2 - T \) from -1 to 1 is evaluated by finding antiderivatives and applying the bounds, resulting in a final answer of 2/3. The process is checked using a calculator. Problem 21 involves integrating \( \frac{3}{x^2-1} \) from 1 to 2, rewritten as \( 3x^{-2} - 1 \), and evaluated similarly, yielding 1/2. The paragraph also covers checking answers with a calculator and understanding the optional step of storing the integrand in 'y1'.
🔍 Detailed Integration Techniques
The second paragraph delves into the integration of complex functions. It starts with integrating \( u - 2 \) over the square root of \( u \) from 1 to 4, simplifying the integrand and applying the power rule to find the antiderivative, which results in 2/3 after evaluation. The paragraph also covers integrating a function from -1 to -2, emphasizing the correct substitution order and sign management, leading to an answer of 2. Problem 24 involves integrating \( 1 + \sin(x) \) from 0 to \( \pi \), using the antiderivative of \( \sin(x) \) as \( -\cos(x) \), and ends with \( \pi + 2 \). Problem 25 integrates a polynomial from 1 to 3, resulting in 38, and the process includes checking the answer with a calculator.
📉 Area Under the Curve Calculations
This paragraph explains how to calculate definite integrals using the area under a graph of a function. It discusses breaking down the area into recognizable shapes for problem 26, which involves integrating from -4 to 2, and results in an area of -6.5. Problem 27 calculates the integral from 0 to 3, using half of a rectangle and resulting in 1. Problem 29 uses the Fundamental Theorem of Calculus to find the integral of \( F'(x) \) from -4 to 0, which equals 3. The paragraph also covers the integral of \( F'(x) \) from -1 to 1, resulting in 4, and from 1 to 3, resulting in -4.
📝 Properties of Definite Integrals
The fourth paragraph explores the properties of definite integrals, using given integral values to find new ones. It starts with the integral of \( F + G \) from 2 to 6, which is calculated as 8 using the sum of given integrals. It continues with the integral of \( -f - 3G \) from 2 to 6, resulting in 26, and the integral of \( G \) from 6 to 2, which is 12 due to the reversal of bounds and multiplication by -1. The paragraph also addresses an integral that cannot be determined with the given information due to the lack of a property for division in integrals.
📌 Definite Integrals with Given Values
This paragraph continues the application of properties of definite integrals with new given values. It calculates the integral of \( F + 4 \) from -2 to 4 as 18 and the integral of \( 3G + X \) from -2 to 4 as 18. It also discusses the integral of \( \frac{1}{2}F + 3X^2 \) from -2 to 4, resulting in 69, and uses the graph of \( f'(x) \) to answer questions about the integral from 0 to 7, which sums up to 9.
📘 Calculus Theorems and Graph Interpretation
The final paragraph uses the graph of \( f'(x) \) to answer questions about definite integrals and the values of the original function \( f(x) \). It explains how to find \( f(3) \) given \( f(0) \) and the integral of \( f'(x) \) from 0 to 3, resulting in \( f(3) = 0 \). It also finds \( f(7) \) using the integral from 3 to 7, concluding \( f(7) = 5 \). The paragraph emphasizes the relationship between the integral of a derivative and the function values, as per the Fundamental Theorem of Calculus.
Mindmap
Keywords
💡Fundamental Theorem of Calculus
💡Antiderivative
💡Integration
💡Definite Integral
💡Reverse Power Rule
💡Simplification
💡Substitution
💡Calculator Verification
💡Area under the Curve
💡Properties of Integrals
Highlights
Introduction to solving Unit Six homework problems 20 through 34 involving the Fundamental Theorem of Calculus.
Evaluation of the integral of \( T^2 - T \) from -1 to 1 using the reverse power rule to find antiderivatives.
Substitution of the upper and lower bounds to find the definite integral result of 2/3.
Verification of the integral result using a calculator and the optional step of storing the function in Y1.
Integration of \( \frac{3}{x^2} - 1 \) from 1 to 2 with a focus on rewriting the function for easier antiderivative finding.
Explanation of the antiderivative process for \( 3x^{-2} \) and the subsequent evaluation from 1 to 2 resulting in 1/2.
Integration of \( u - 2 \) over the square root of \( u \) from 1 to 4, with simplification and application of the power rule.
Multiplication of the denominator and numerator by 2 to balance the equation and integrate resulting in two-thirds.
Integration of \( x - 1 \) over \( x^2 \) from -1 to -2, with polynomial form rewriting and area calculation.
Calculation of the definite integral using the reverse order of limits and resulting in the answer of 2.
Integration of \( 1 + \sin(x) \) from 0 to \( \pi \), with direct substitution into the antiderivative and resulting in \( \pi + 2 \).
Integration of \( 3x^2 + 5x - 4 \) from 1 to 3, with straightforward application of the power rule and final result of 38.
Use of the graph of a function to determine definite integrals by calculating areas under the curve.
Determination of the integral of \( f \) from -4 to 2 by breaking down the area into recognizable geometric shapes.
Integration of \( f' \) from -4 to 0 using the fundamental theorem of calculus and graph values to find the result of 3.
Application of properties of definite integrals to find new integrals based on given integral values.
Explanation of the inability to split integrals involving multiplication or division without a constant multiple.
Use of the graph of \( f' \) to determine the value of the integral from 0 to 7 by summing areas of geometric shapes.
Determination of \( f(3) \) given \( f(0) = -3 \) and the integral of \( f' \) from 0 to 3, resulting in \( f(3) = 0 \).
Calculation of \( f(7) \) from \( f(3) \) using the integral of \( f' \) from 3 to 7 and finding \( f(7) = 5 \).
Transcripts
Browse More Related Video

Calculus AB Homework 6.6: Fundamental Theorem of Calculus Part II

AP Calculus AB: Lesson 6.6 Fundamental Theorem of Calculus, Part 2

Definite Integral Calculus Examples, Integration - Basic Introduction, Practice Problems
Fundamental Thereom of Calculus Explained - Part 1 & 2 Examples - Definite Integral

AP Calculus AB: Lesson 6.3 Fundamental Theorem of Calculus Part 1

2019 AP Calculus AB & BC Free Response Question #3
5.0 / 5 (0 votes)
Thanks for rating: