Rational Inequalities
TLDRThis educational video offers a step-by-step guide on solving rational inequalities. It begins with an example, demonstrating how to use a number line to find where a given rational expression is greater than or equal to zero. The video explains the importance of identifying points of interest, testing regions for sign changes, and shading the appropriate areas to represent the solution. It then progresses to additional examples, each time emphasizing different aspects like moving constants to the other side of the inequality and finding common denominators. The video concludes by suggesting viewers check out a precalculus playlist for more related content, making it an informative resource for those learning to tackle rational inequalities.
Takeaways
- 📉 Rational inequalities involve finding the values of x that make a rational expression positive, negative, or zero.
- 📏 To solve these inequalities, identify critical points by setting the numerator and denominator equal to zero.
- 📍 The critical points divide the number line into regions that need to be tested to determine if the rational expression is positive or negative.
- ➕ A positive divided by a positive or a negative divided by a negative results in a positive value.
- ➖ A negative divided by a positive or a positive divided by a negative results in a negative value.
- ⚪ Use open circles on the number line for points where the denominator is zero (undefined) and closed circles where the numerator equals zero (if the inequality includes equal to).
- 🔎 To solve, test points within each region created by the critical points to determine the sign of the rational expression in those intervals.
- 📝 Use interval notation to express the solution, considering open and closed intervals based on the inequality.
- 🆚 Inequality notation can also express the solution by combining the intervals into statements using inequality symbols.
- 🔄 Steps include setting up the inequality, moving terms, finding common denominators, simplifying the expression, and testing intervals.
Q & A
What is the main topic of the video?
-The main topic of the video is solving rational inequalities.
What is the first example of a rational inequality presented in the video?
-The first example is \( \frac{x - 3}{x + 2} \geq 0 \).
How does the video suggest to start solving a rational inequality?
-The video suggests starting by setting up a number line and identifying points of interest where the numerator or denominator equals zero.
What are the points of interest for the first example in the video?
-The points of interest for the first example are x = 3 (where the numerator equals zero) and x = -2 (where the denominator equals zero).
Why is there an open circle at x = -2 on the number line in the first example?
-There is an open circle at x = -2 because the denominator cannot equal zero, making the function undefined at that point.
How does the video determine the sign of each region in the number line for the first example?
-The video determines the sign by plugging in test points from each region into the rational expression and observing the sign of the result.
What is the solution to the first example in interval notation?
-The solution in interval notation is \( (-\infty, -2) \cup [3, \infty) \).
What is the second example of a rational inequality presented in the video?
-The second example is \( \frac{(x - 4)(x + 1)}{x - 3} < 0 \).
How does the video approach solving the second example where the inequality is less than zero?
-The video approaches it by finding the regions that are negative, not positive, and then shading the appropriate regions on the number line.
What is the solution to the second example using interval notation?
-The solution is \( (-\infty, -1) \cup (3, 4) \).
What is the third example presented in the video, and what is the inequality?
-The third example is \( \frac{x + 2}{x - 1} \leq 3 \), which is transformed into \( \frac{x + 2 - 3(x - 1)}{x - 1} \leq 0 \) or \( \frac{-2x + 5}{x - 1} \leq 0 \).
How does the video handle the inequality in the third example that is not equal to zero?
-The video moves the constant term to the other side, combines the terms over a common denominator, and then simplifies the expression before proceeding with the number line and sign analysis.
What are the points of interest for the third example in the video?
-The points of interest for the third example are x = 1 (where the denominator equals zero and is not included) and x = 2.5 (where the numerator equals zero).
What is the solution to the third example using interval notation?
-The solution is \( (-\infty, 1) \cup [2.5, \infty) \).
How does the video suggest expressing the solution to the inequalities?
-The video suggests expressing the solution using interval notation and also as inequalities, such as \( x < -2 \) or \( x \geq 3 \) for the first example.
Outlines
📚 Solving Rational Inequalities with Number Line Analysis
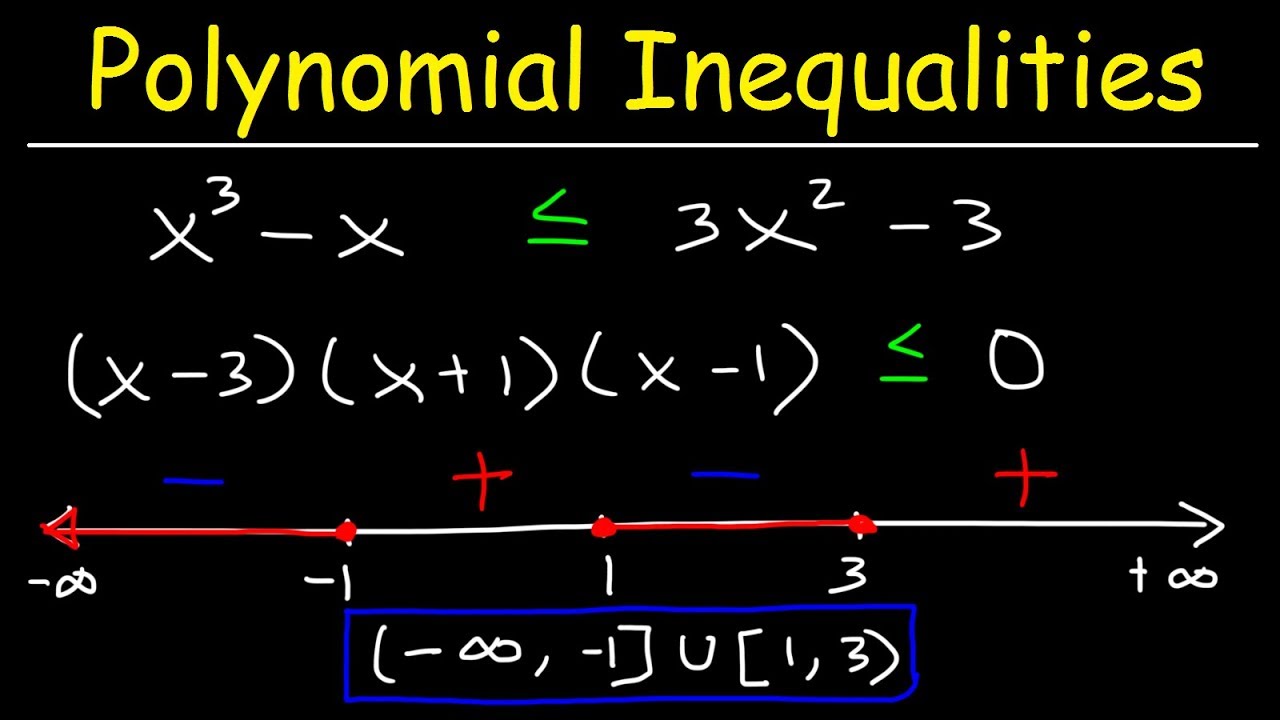
This paragraph introduces the process of solving rational inequalities through a step-by-step example. The example given is the inequality \( \frac{x - 3}{x + 2} \geq 0 \). The approach involves using a number line to identify points of interest, which are determined by setting the numerator and denominator equal to zero, resulting in x = 3 and x = -2. The video explains the importance of considering where the function is undefined (x + 2 ≠ 0, hence x ≠ -2) and where the numerator can be zero (x = 3). The number line is then divided into regions, and the sign of the expression in each region is determined by testing values. The solution to the inequality is found by identifying the regions where the expression is positive, resulting in the intervals (-∞, -2) with an open circle at -2, and [3, ∞) with a closed circle at 3. The paragraph concludes by showing how to express the solution both in interval notation and as inequalities.
🔍 Further Exploration of Rational Inequalities with Different Scenarios
The second paragraph continues the discussion on solving rational inequalities, presenting additional examples and variations. The first example is the inequality \( \frac{x - 4}{x + 1} \cdot \frac{x + 1}{x - 3} < 0 \), which simplifies to \( \frac{x - 4}{x - 3} < 0 \). The video script details the process of identifying points of interest (-1, 3, and 4) and determining the sign of the expression in the regions defined by these points. The solution is given in interval notation as (-∞, -1) ∪ (3, 4), and as inequalities x < -1 or 3 < x < 4. The second example involves moving a constant to the other side of the inequality and simplifying before solving, resulting in the solution x < 1 or x ≥ 5/2. The paragraph emphasizes the importance of understanding the multiplicity of zeros and their impact on the sign of the expression. It concludes by directing viewers to a precalculus playlist for further learning.
Mindmap
Keywords
💡Rational Inequalities
💡Number Line
💡Numerator
💡Denominator
💡Critical Points
💡Sign Analysis
💡Interval Notation
💡Open and Closed Circles
💡Inequality Constraints
💡Simplifying Rational Expressions
Highlights
The video focuses on solving rational inequalities.
An example is given where \( \frac{x-3}{x+2} \geq 0 \).
A number line is used to visualize the inequality.
Points of interest are identified as -2 and 3.
The function is undefined at x = -2, indicated by an open circle.
The numerator can be zero at x = 3, indicated by a closed circle.
Three regions on the number line are considered for the inequality.
The sign of each region is determined by plugging in test points.
The solution is expressed in interval notation and as inequalities.
A second example with \( \frac{(x-4)(x+1)}{x-3} < 0 \) is presented.
Points of interest for the second example are -1, 3, and 4.
All points of interest are open circles since the inequality is strict.
Regions are tested for negativity to satisfy the inequality.
The solution for the second example is given in interval notation and inequalities.
A third example involves solving \( \frac{x+2}{x-1} - 3 \leq 0 \).
The inequality is rearranged to have a common denominator.
Points of interest for the third example are 1 and 2.5.
The solution for the third example is provided in interval notation and inequalities.
The video encourages viewers to check out the precalculus playlist for more.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: