Solving Quadratic Inequalities (Precalculus - College Algebra 25)
TLDRThis educational video script delves into the topic of quadratic inequalities, explaining their concept and solving methods. It begins by defining quadratic inequalities as parabolic functions intersecting with the x-axis and discusses their graphical representation. The script offers a step-by-step guide on solving these inequalities by setting the equations to zero, factoring, and identifying x-intercepts to determine intervals where the parabola is above or below the x-axis. The explanation is aimed at preparing viewers for tackling rational inequalities in future lessons, making the content not only informative but also foundational for more complex topics.
Takeaways
- 📚 Quadratic inequalities are used to determine intervals where a parabola is above or below a certain value or function, typically the x-axis (y=0).
- 🔍 To solve a quadratic inequality, first, express everything on one side of the inequality and zero on the other, then find the x-intercepts.
- 📈 The direction in which a parabola opens (upward or downward) is crucial for understanding where it will be above or below the x-axis.
- 🤔 Factoring is a common method to find x-intercepts, but it is not the only way; the quadratic formula can also be used if factoring is not possible.
- 📊 The graph of a quadratic inequality is a parabola, and the solution involves finding where this parabola lies in relation to the x-axis.
- 🔗 The concept of symmetry is important in quadratic inequalities because parabolas are symmetrical about their axis of symmetry, which affects the intervals of truth.
- 📌 If a parabola does not intersect the x-axis (no real x-intercepts), the inequality will hold true for all values on one side of the parabola or have no solution at all.
- ✅ When using the quadratic formula, a negative discriminant (b² - 4ac < 0) indicates there are no real x-intercepts, meaning the parabola does not cross the x-axis.
- 📉 The method of plugging in a number (like zero) to test an interval can be a quick way to determine if the inequality holds true or false for that interval.
- 🚫 Changing the direction of the inequality by multiplying or dividing by a negative number requires flipping the inequality sign.
- ➡️ Understanding the graph and the behavior of the parabola makes it easier to visualize and solve quadratic inequalities, especially when dealing with more complex inequalities like rational inequalities.
Q & A
What is a quadratic inequality?
-A quadratic inequality is a mathematical expression involving a quadratic function (a parabola) and an inequality operator, which defines an interval or regions where the quadratic function is greater than, less than, equal to, or not equal to a certain value or function.
How does the direction of the parabola (upward or downward opening) affect the solution of a quadratic inequality?
-The direction of the parabola affects which side of the parabola the solution interval will be on. An upward-opening parabola will have solutions below the x-axis, while a downward-opening parabola will have solutions above the x-axis.
What is the first step in solving a quadratic inequality?
-The first step in solving a quadratic inequality is to get all terms on one side of the inequality and zero on the other side, so that you can more easily analyze the parabola's relationship to the x-axis.
How can you find the x-intercepts of a quadratic inequality?
-You can find the x-intercepts by setting the quadratic expression equal to zero and solving for x. These x-intercepts will help you determine where the parabola crosses the x-axis.
What is the significance of the x-intercepts in solving quadratic inequalities?
-The x-intercepts are significant because they divide the number line into intervals where the parabola will be either above or below the x-axis. This helps in determining the intervals that satisfy the inequality.
How does the nature of parabolas as even functions affect the solution of quadratic inequalities?
-Parabolas, being even functions, exhibit symmetry about the axis of symmetry. This means that the intervals on either side of the axis of symmetry will have the same truth value with respect to the inequality, leading to alternating true and false intervals.
What is the difference between using brackets and parentheses when representing the solution interval of a quadratic inequality?
-Brackets are used to include the endpoint of an interval, indicating that the endpoint is part of the solution set. Parentheses are used to exclude the endpoint, indicating that the endpoint is not part of the solution set. This distinction depends on whether the inequality is strict or includes equality.
How can you determine the solution set for a quadratic inequality without graphing?
-You can determine the solution set by using the quadratic formula to find the x-intercepts, and then by testing intervals with a chosen test point (often zero or another convenient value) to see if the inequality holds true or false, which will indicate the solution intervals.
What is the role of the discriminant in solving quadratic inequalities?
-The discriminant (the value under the square root in the quadratic formula) determines the nature of the roots of the quadratic equation. A positive discriminant means two real x-intercepts, a discriminant of zero means one real x-intercept, and a negative discriminant means no real x-intercepts, which affects the solution set of the inequality.
How does changing the direction of the inequality sign affect the solution of a quadratic inequality?
-Changing the direction of the inequality sign can change the solution set. For example, if you multiply or divide both sides of an inequality by a negative number, you must reverse the direction of the inequality sign. This can shift the solution set from one side of the parabola to the other.
What is the relationship between the parabola's y-intercept and the solution set of a quadratic inequality?
-The y-intercept of the parabola does not directly affect the solution set of the inequality. The solution set is determined by where the parabola is above or below the x-axis, which is independent of the y-intercept unless the inequality involves the y-axis directly.
Outlines
📚 Introduction to Quadratic Inequalities
The video begins with an introduction to quadratic inequalities, explaining what they are, their representation, and their role in mathematics. The presenter emphasizes that understanding these inequalities is crucial for future discussions on rational inequalities. A quadratic inequality is described as a mathematical statement involving a parabola and an interval where the value is either above or below a certain threshold. The process of solving these inequalities involves graphing the parabola and determining where it lies in relation to the x-axis (y=0). Key steps include moving all terms to one side of the inequality, finding the x-intercepts, and identifying the intervals where the inequality holds true.
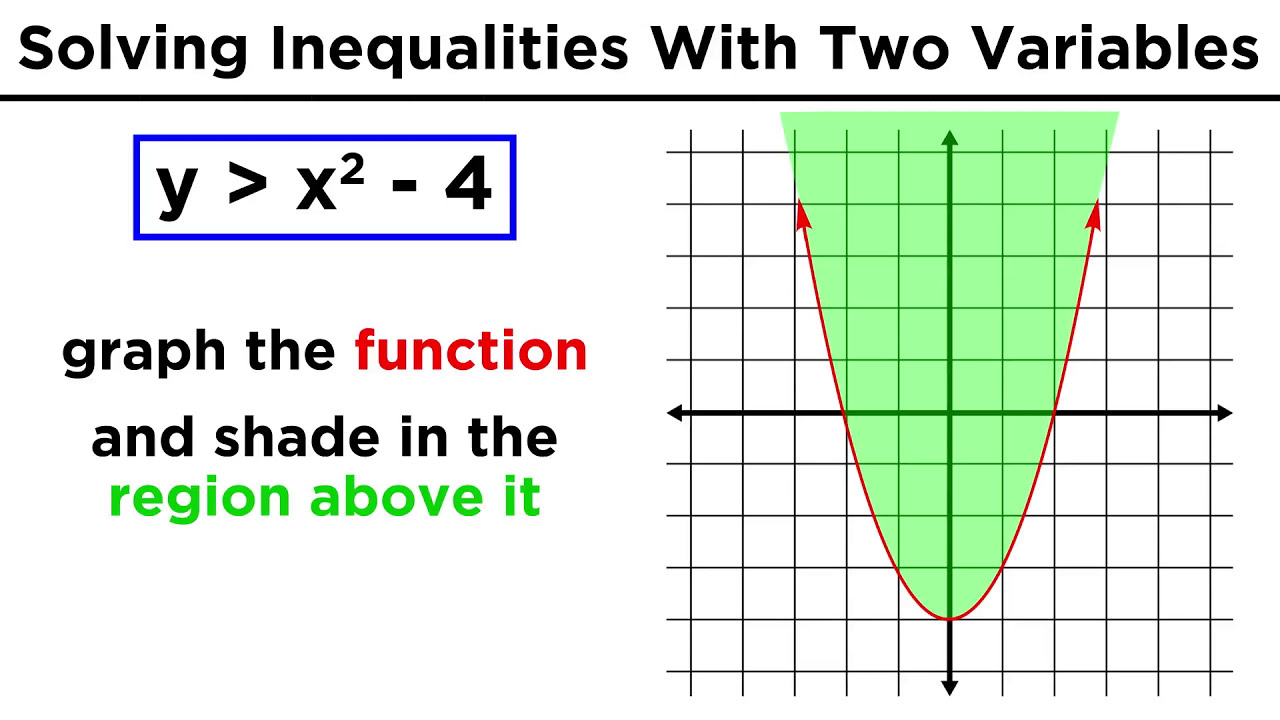
📈 Solving Quadratic Inequalities Graphically
The presenter discusses two methods for solving quadratic inequalities: graphically and algebraically. The graphical method is preferred as it aligns with future topics on rational inequalities. The process involves finding where the parabola intersects the x-axis and determining the intervals where the parabola is below the x-axis. The video explains the use of brackets and parentheses to denote whether the endpoints of the interval are included or excluded based on the type of inequality. An alternative algebraic method is also mentioned, which involves understanding the symmetry of parabolas and using truth statements to determine the solution intervals.
🔍 Factoring and Interpreting Quadratic Inequalities
This paragraph delves into the process of factoring a quadratic expression to find the x-intercepts, which are crucial for interpreting the inequality. The presenter clarifies that finding the x-intercepts does not solve the inequality but provides the necessary information to determine the intervals where the parabola is above or below the x-axis. The video also illustrates how to translate the problem of a parabola being below a line into a simpler form by getting everything on one side and setting the other side to zero, which effectively turns the line into the x-axis for the purpose of the inequality.
🤔 Dealing with Upward and Downward Opening Parabolas
The presenter addresses the question of downward opening parabolas and explains that the direction of the parabola's opening does not affect the solution process. Whether the parabola opens upward or downward, the method for finding the solution interval remains the same. The video demonstrates that changing the signs of the terms in the inequality and solving for an upward opening parabola yields the same interval as solving for a downward opening parabola above the x-axis. The importance of changing the inequality sign when dividing by a negative number is also highlighted.
🧩 Manipulating Inequalities and Visualizing Parabolas
The video continues with an example of an inequality involving a parabola and a horizontal line. The presenter shows how to manipulate the inequality to transform the problem into one where the parabola is compared to the x-axis instead of the horizontal line. Factoring the quadratic expression is key to finding the x-intercepts and determining the intervals where the parabola is above the x-axis. The use of interval notation and the decision between using parentheses and brackets based on the inequality's strictness are also discussed.
📉 Inequalities with No Real Solutions
The presenter tackles a scenario where the quadratic inequality has no real solutions. This occurs when the parabola has no x-intercepts, meaning it never crosses the x-axis. The video explains how to use the discriminant from the quadratic formula to determine if there are real solutions. If the discriminant is negative, it indicates that the parabola does not intersect the x-axis, and thus there are no values for which the inequality is true. The presenter also discusses how to approach the problem if the inequality sign were changed, emphasizing that the parabola would always be above the x-axis with no x-intercepts.
🌟 Conclusion and Future Applications
In the final paragraph, the presenter concludes the discussion on quadratic inequalities and hints at future applications, including rational inequalities. The video emphasizes the importance of visualizing the parabola and the x-axis, as this approach tends to be more intuitive and memorable for students. The presenter also mentions that understanding the graphical representation of inequalities will be beneficial when dealing with more complex topics such as multiplicity and the even or odd nature of vertical asymptotes.
Mindmap
Keywords
💡Quadratic Inequalities
💡Parabola
💡X-Intercepts
💡Inequality Operator
💡Interval Notation
💡Graphing
💡Discriminant
💡Quadratic Formula
💡Continuous Function
💡Rational Inequalities
💡Multiplicity
Highlights
Quadratic inequalities represent intervals where a parabola is above or below a certain value or function.
Solving quadratic inequalities involves finding where the parabola intersects the x-axis and determining the intervals above or below it.
To solve a quadratic inequality, get all terms on one side and zero on the other, then factor to find x-intercepts.
The nature of parabolas means that their truth statements alternate, being either true or false across different intervals.
Graphing the parabola and the x-axis can visually represent the solution to the inequality.
If a parabola does not intersect the x-axis, it means there are no real solutions to the inequality.
The use of brackets or parentheses in the solution indicates whether the endpoints are included or excluded.
An upward-opening parabola will always have an interval where it is below the x-axis, and vice versa for a downward-opening parabola.
Changing the direction of a parabola's opening by multiplying by -1 requires flipping the inequality sign.
When dividing by a negative number in an inequality, the direction of the inequality must be reversed.
The discriminant (b^2 - 4ac) determines the nature of the roots of a quadratic equation and thus the x-intercepts.
If the discriminant is negative, the parabola does not intersect the x-axis, indicating no real solutions for certain inequalities.
The quadratic formula can be used to find the x-intercepts when factoring is not possible.
Completing the square or using the quadratic formula can help determine the intervals where the parabola is above or below the x-axis.
The concept of even and odd functions and the multiplicity of roots are important for understanding more complex inequalities.
Visualizing the parabola and its relationship to the x-axis is a powerful tool for solving quadratic inequalities.
The method of plugging in a number to determine the truth value of an interval is a practical approach when graphing is not preferred.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: