College Physics 1: Lecture 3 - Significant Figures and Scientific Notation

Spahn's Science Lectures
23 Aug 202016:17
EducationalLearning
32 Likes 10 Comments

TLDRThe lecture introduces significant figures and scientific notation as essential tools in physics for accurately representing measurements. It emphasizes the importance of precision in scientific measurements and explains how significant figures convey the reliability of known digits in a number. The transcript outlines rules for determining significant figures, including the treatment of leading, in-between, and trailing zeros. It also discusses how to handle significant figures during mathematical operations like addition, subtraction, multiplication, and division. Furthermore, the lecture explains the concept of scientific notation for expressing both large and small numbers concisely and accurately. It demonstrates how to convert numbers into scientific notation format, highlighting its advantages for clarity and compactness. The speaker concludes by mentioning the next topic: units and unit conversions, which is crucial for understanding different measurement systems used globally.

Takeaways
  • 📏 Significant figures are crucial for conveying the precision of measurements in physics.
  • 🔍 Scientific notation is used to express both very large and very small numbers in a compact form.
  • 📐 The precision of a measurement depends on the instrument used and the skill of the person taking the measurement.
  • 🔢 Significant figures include all non-zero digits, digits between non-zero digits, and trailing zeros after a decimal point.
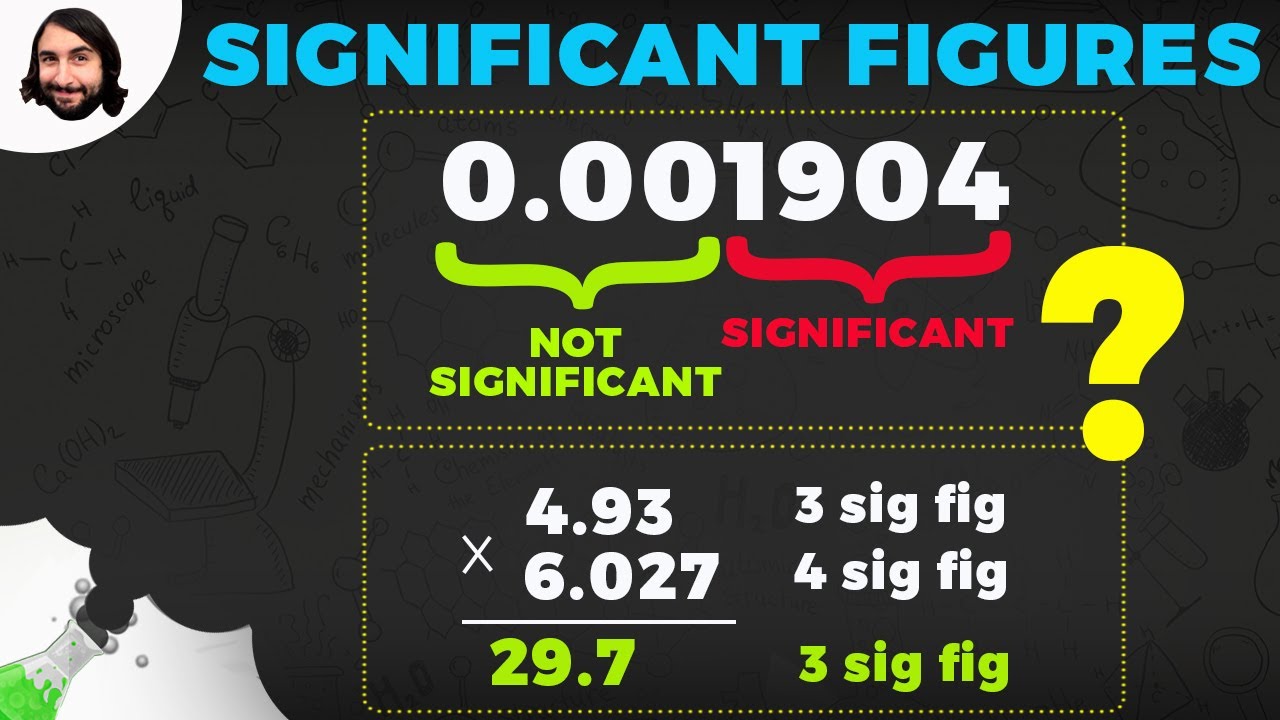
  • ✂️ Leading zeros are not considered significant as they do not affect the precision of the measurement.
  • 📉 Trailing zeros without a decimal point are not significant, as they may be the result of rounding.
  • 🔄 When performing addition or subtraction, the number of decimal places in the result should match the number with the fewest decimal places.
  • 🔢 In multiplication or division, the result should have the same number of significant figures as the number with the fewest significant figures.
  • 🌌 Scientific notation is expressed as a number between 1 and 10 multiplied by 10 raised to a power, making it easier to handle large and small numbers.
  • 🌐 The use of scientific notation helps to clearly articulate the number of significant figures present in a number, aiding in clarity and precision.
Q & A

  • What is the purpose of significant figures in scientific measurements?

    -Significant figures are used to convey the precision of a measurement. They represent the digits in a number that are reliably known, ensuring that the reported measurements are accurate and precise.

  • How does the precision of a measuring instrument affect the precision of a measurement?

    -The precision of a measurement is directly dependent on the precision of the instrument used. For example, a ruler with millimeter divisions can only provide measurements accurate to plus or minus 0.1 millimeter, whereas a caliper can be more precise, potentially accurate to 0.01 millimeters.

  • What impact does the user's skill or judgment have on the precision of a measurement?

    -The user's skill or judgment can significantly affect the precision of a measurement. Factors such as viewing angle, reaction time, and interpretation of the instrument's readings can introduce errors, reducing the precision of the measurement.

  • What are the rules for determining significant figures in a number?

    -The rules for determining significant figures are: (1) All non-zero numbers are significant. (2) Leading zeros are not significant. (3) In between zeros that fall between two non-zero numbers are significant. (4) Trailing zeros to the right of a decimal place are significant, but trailing zeros to the left of a decimal point without a decimal shown are not significant.

  • How do you handle trailing zeros when counting significant figures?

    -Trailing zeros to the right of a decimal point are significant. However, if there is no decimal point shown, trailing zeros are not significant, as they may be the result of rounding.

  • What changes should be made to significant figures when performing addition and subtraction?

    -When adding or subtracting numbers, the result should have the same number of decimal places as the number with the fewest decimal places to ensure the correct number of significant figures is maintained.

  • How does the process of counting significant figures change when multiplying or dividing numbers?

    -When multiplying or dividing, the result should have the same number of significant figures as the number with the fewest significant figures among the operands, not based on the decimal places.

  • Why is scientific notation used in physics?

    -Scientific notation is used to express very large and very small numbers in a compact form. It simplifies the writing of numbers with many zeros and clearly indicates the number of significant figures.

  • What is the general format for expressing numbers in scientific notation?

    -The general format for scientific notation is m times 10 raised to the nth power, where m is a number between 1 and 10, and n is an integer that can be positive or negative.

  • How do you convert a large number into scientific notation?

    -To convert a large number into scientific notation, move the decimal point to the left until only one non-zero digit remains to its left. The number of places moved becomes the exponent n in the power of 10.

  • How is a small number, less than 1, expressed in scientific notation?

    -For a small number less than 1, move the decimal point to the right until it passes the first non-zero digit. The remaining number is then multiplied by 10 raised to a negative exponent, with the exponent being the number of places moved.

Outlines
00:00
📏 Precision and Significant Figures in Measurement

This paragraph introduces the importance of significant figures in scientific measurements and notation. It explains that physics deals with a vast range of scales and emphasizes the necessity of precision in reporting measurements. The paragraph discusses how the precision of a measurement is determined by the instrument used and the user's skill. It also clarifies the concept of significant figures as the digits in a number that are reliably known. An example is provided to illustrate the difference between reporting measurements with different levels of precision, highlighting the importance of accuracy in scientific communication.

05:03
🔢 Understanding Significant Figures and Scientific Notation

This section delves into the rules for determining the number of significant figures in a number. It explains that non-zero digits are always significant, while leading zeros are not. The significance of zeros between non-zero digits and trailing zeros after a decimal point is also discussed. The paragraph further clarifies the treatment of trailing zeros without a decimal point, which are not considered significant. Additionally, it addresses the impact of mathematical operations on significant figures, particularly in the context of addition and subtraction, where the number of decimal places in the result should match the number with the fewest decimal places.

10:04
🔄 Multiplication, Division, and Scientific Notation

Continuing from the previous discussion, this paragraph focuses on the treatment of significant figures during multiplication and division. It states that the answer should have the same number of significant figures as the number with the fewest significant figures in the operation. An example is given to illustrate this rule. The paragraph then transitions to discussing scientific notation as a means to express very large or very small numbers concisely and clearly. It explains the format of scientific notation (m × 10^n) and its advantages, including compactness and the ability to easily convey the number of significant figures.

15:05
🌌 Converting to Scientific Notation for Clarity

This final paragraph provides a practical guide on converting numbers into scientific notation. It explains the process for both large numbers (where the decimal point is moved to the left) and small numbers (where the decimal point is moved to the right). The paragraph illustrates how to determine the exponent in the scientific notation based on the number of places the decimal point has been moved. It emphasizes the benefits of using scientific notation for expressing measurements in physics, particularly for very large or very small quantities, and concludes with a preview of the next lecture's topic on units and unit conversions.

Mindmap
Keywords
💡Significant Figures
Significant figures are the digits in a number that are known to be reliable and accurately represent the measurement. They are crucial in scientific measurements to convey the precision of the data. In the video, the concept is introduced with the example of measuring an object as 6.2 centimeters, which has two significant figures, the six and the two. Misrepresenting the number of significant figures, such as saying 6.213, would inaccurately suggest a higher level of precision than was actually achieved.
💡Scientific Notation
Scientific notation is a method of expressing very large or very small numbers in a compact form that uses powers of ten. It simplifies the writing of numbers with many zeros and makes it clear how many significant figures are present. The video explains that numbers are written as 'm times 10 raised to the nth power', where 'm' is a number between 1 and 10, and 'n' is an integer. For instance, the radius of a hydrogen atom, which would be cumbersome to write out fully, can be expressed as a small number like 5.3 times 10 to the power of negative 10 meters.
💡Precision
Precision refers to the degree of exactness of a measurement or the repeatability of a measurement when it is made multiple times. The video discusses how the precision of a measurement is determined by the instrument used and the skill of the person taking the measurement. For example, a ruler might only allow for a precision of plus or minus 1 millimeter, while a caliper could be more precise, allowing measurements to the nearest 0.01 millimeters.
💡Measurement
Measurement is the process of determining the size, amount, or degree of something by comparing it with a standard unit. The video emphasizes the importance of measurement in physics for understanding the natural world and the need for precision in these measurements. It also touches on the limitations of measurement precision, such as the limitations imposed by the instruments used, like a ruler or a caliper.
💡Leading Zeros
Leading zeros are zeros that precede the first non-zero digit in a number. They are not counted as significant figures because they do not contribute to the precision of the measurement. The video gives the example of the number 0.046, where the leading zeros are not significant, but the '4' and '6' are, resulting in two significant figures.
💡Trailing Zeros
Trailing zeros are zeros that follow the last non-zero digit in a number. Their significance depends on whether a decimal point is present. If a decimal point is shown, as in 18.00, the trailing zeros are significant. However, if no decimal point is shown, as in the number 240, the trailing zero is not significant, and the number is considered to have only two significant figures, as explained in the video.
💡In-Between Zeros
In-between zeros are zeros that occur between non-zero digits in a number and are always considered significant. They contribute to the precision of the measurement. The video uses the number 2019 as an example, where the zero is between two non-zero digits and is therefore significant, making the number have four significant figures.
💡Mathematical Operations
When performing mathematical operations like addition, subtraction, multiplication, and division, the video explains that the number of significant figures in the result must be considered. For addition and subtraction, the result should have the same number of decimal places as the number with the fewest decimal places. For multiplication and division, the result should have the same number of significant figures as the number with the fewest significant figures. This ensures that the precision of the operation is accurately represented.
💡Decimal Place
A decimal place is the position of a digit in a number to the right of the decimal point. The video explains that trailing zeros to the right of a decimal point are significant, as they indicate a level of precision. For example, the number 18.00 has four significant figures because the zeros after the decimal point indicate that the measurement was precise to the hundredths place.
💡Units and Unit Conversions
Units are the standard quantities used to express the magnitude of physical properties. Unit conversions are necessary when changing from one system of units to another, such as from English to metric units. The video mentions that the next lecture will cover units and conversions, highlighting the importance of understanding different systems of measurement and being able to convert between them, especially when dealing with international standards or diverse scientific literature.
Highlights

Physics attempts to explain the natural world from the very small to the exceedingly large.

The importance of measuring quantities with precision and reporting measurements with correct significant figures.

The use of scientific notation to express large and small numbers in a compact form.

The precision of a measurement depends on the instrument used and the skill or judgment of the user.

Significant figures are the digits in a number that are reliably known.

Non-zero numbers are always considered significant.

Leading zeros are not significant, as they come before the first non-zero number.

In-between zeros between two non-zero numbers are considered significant.

Trailing zeros to the right of a decimal place are considered significant.

When a decimal place is shown, a trailing zero is significant; without it, the trailing zero is not significant.

In mathematical operations, the number of significant figures in the result depends on the operation type.

For addition and subtraction, the result should have the same number of decimal places as the number with the fewest decimal places.

For multiplication and division, the result should have the same number of significant figures as the number with the fewest significant figures.

Scientific notation is used to express very large and very small numbers concisely and clearly.

Scientific notation is expressed in the form of m times 10 raised to the nth power, where m is between 1 and 10, and n is an integer.

Conversion of large numbers to scientific notation involves moving the decimal place to the left until one non-zero digit remains to its left.

Conversion of small numbers to scientific notation involves moving the decimal place to the right until it passes the first non-zero digit.

The next lecture will discuss units and unit conversions, including the differences between English and metric units.

Transcripts

Browse More Related Video

What are Significant Figures, Precision & Accuracy in Chemistry & Physics?

Unit Conversion & Significant Figures: Crash Course Chemistry #2

03 - Significant Figures Rules (Sig Fig Rules) for Calculations in Chemistry & Physics

2.1 Significant Figures | High School Chemistry

Measurement and Significant Figures

Significant Figures Made Easy!

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Significant FiguresScientific NotationPhysics LectureMeasurement PrecisionEducational ContentNumerical AccuracyDecimal PlacesMeasurement UnitsConversion FactorsScientific Method