What are Significant Figures, Precision & Accuracy in Chemistry & Physics?
TLDRThe video script provides an in-depth explanation of the concepts of accuracy, precision, and significant figures, which are fundamental in chemistry and physics. It clarifies that accuracy refers to how close a measurement is to the true value, while precision indicates how close repeated measurements are to each other. The script uses the analogy of a dartboard to illustrate these concepts, explaining that high precision means measurements are clustered closely together, and high accuracy means they are close to the true value. The lesson also distinguishes between exact numbers, which are defined values like the number of eggs in a dozen, and measured numbers, which have inherent variability. It then delves into the rules for determining significant figures in a measurement, emphasizing that non-zero digits are always significant, zeros between non-zero digits are significant, and leading zeros are not, unless there is a decimal point. The script concludes with practical exercises on identifying significant figures and rounding numbers to a specified level of precision, using both standard and scientific notation.
Takeaways
- 🎯 **Accuracy vs. Precision**: Accuracy is how close measurements are to the true value, while precision is the consistency of repeated measurements.
- 📏 **Understanding Measurement**: In science, measurements are either exact (like 12 eggs in a dozen) or approximate (like the mass of an object measured with a scale).
- 🔢 **Significant Figures**: Significant figures are the digits in a number that carry meaning contributing to its precision. This includes all non-zero digits, zeros between significant digits, and trailing zeros in decimal fractions.
- 📐 **Counting Significant Figures**: You can determine the number of significant figures by ignoring leading zeros, counting zeros between non-zero digits, and considering trailing zeros in decimal numbers as significant.
- ⚖️ **Exact Numbers**: Exact numbers are those that are defined by a counting process or a standard conversion factor, such as the number of eggs in a dozen or the number of grams in a kilogram.
- 📊 **Graphical Analogy**: The concept of accuracy and precision can be visualized using a dartboard analogy, where the bullseye represents the true value, and the clustering of darts represents the precision and proximity to the bullseye represents accuracy.
- 🔑 **Rounding Rules**: When rounding numbers to a certain number of significant figures, you look at the digit following the last significant figure to be kept and apply standard rounding rules.
- 🧮 **Scientific Notation**: Scientific notation is a way to express numbers that are too large or too small in a compact form, which also helps in counting significant figures by focusing on the coefficient.
- 📉 **Measurement Scenarios**: Understanding whether a measurement is exact or not is crucial before determining the number of significant figures it contains.
- 🔍 **Identifying Significant Zeros**: In a number, zeros are significant if they are between non-zero digits or if they are trailing zeros in a decimal number.
- 🧠 **Practice and Application**: The best way to become proficient in identifying significant figures is through practice, applying the rules to various numbers and scenarios.
Q & A
What is the main difference between precision and accuracy in scientific measurements?
-Precision refers to how closely repeated measurements agree with each other, while accuracy refers to how closely those measurements agree with the true value of what is being measured.
How does the concept of significant figures relate to the precision and accuracy of measurements?
-Significant figures indicate the precision of a measurement and help ensure that the results of calculations do not imply a greater precision than the original measurements allow.
What is an example of an exact number in the context of scientific measurements?
-An example of an exact number is the conversion factor of 1 kilometer being equal to 1000 meters, as it is a defined standard and does not require measurement.
How can you determine if a zero in a number is significant?
-A zero is significant if it is between non-zero digits or if it is a trailing zero after a decimal point.
What is the general rule for counting significant figures in a number without a decimal point?
-In a number without a decimal point, trailing zeros are generally not considered significant unless they are between non-zero digits.
How does the concept of scientific notation simplify the process of counting significant figures?
-Scientific notation allows us to ignore the power of 10 and focus only on the digits in the number, making it easier to count significant figures.
What is the purpose of rounding a calculated result to a certain number of significant figures?
-Rounding ensures that the result of a calculation does not imply a higher degree of accuracy than the measurements used in the calculation.
How does the presence of a decimal point affect the significance of trailing zeros in a number?
-When a decimal point is present, trailing zeros are considered significant because they indicate the level of precision of the measurement.
What is the difference between significant figures in a measurement and significant figures in a calculated result?
-In a measurement, significant figures reflect the precision of the measuring instrument. In a calculated result, significant figures are determined by the precision of the least precise measurement involved in the calculation.
Why is it important to understand the difference between precision and accuracy when conducting scientific experiments?
-Understanding the difference is crucial because it allows scientists to correctly interpret the reliability and truthfulness of their experimental results and to communicate them effectively to others.
How can you identify if a measurement is exact or not?
-A measurement is exact if it is a defined standard or a countable whole object, such as the number of eggs in a dozen or the number of inches in a mile. If it requires a measuring instrument and can vary slightly, it is not exact.
Outlines
📐 Understanding Accuracy and Precision
This paragraph introduces the concepts of significant figures, precision, and accuracy, which are fundamental in chemistry and physics. The speaker aims to clarify the common confusion between precision and accuracy. Precision is defined as the closeness of repeated measurements to each other, while accuracy is the closeness of those measurements to the true value. The use of dart boards as an analogy helps illustrate the difference: a high precision and accuracy scenario would have darts clustered close to the bullseye, whereas low precision and accuracy would scatter the darts widely and far from the bullseye. The importance of understanding these concepts for all calculations in class is emphasized.
🎯 The Misunderstood Relationship Between Accuracy and Precision
The speaker elaborates on the distinction between accuracy and precision using the example of a balance that has been tampered with by gluing a weight underneath it. This results in measurements that, while precise (consistent with each other), are not accurate (far from the true value). Three scenarios are presented: high precision and accuracy, high precision with low accuracy, and low precision with low accuracy. The paragraph emphasizes the need to remember the visual representation of these scenarios for a clear understanding of the concepts.
🔢 Exact Numbers and the Concept of Significant Figures
The paragraph discusses the difference between exact numbers, which are precise and require no measurement, and measured numbers, which are subject to slight variations. Exact numbers, such as the number of eggs in a dozen or conversion factors like grams in a kilogram, are defined and do not fluctuate. In contrast, measured numbers, even with precision, will have slight differences due to uncontrollable variables. The concept of significant figures is introduced as a way to quantify the reliability of measured numbers, with rules for determining which digits are considered significant.
📉 Reading Measurements and Identifying Significant Figures
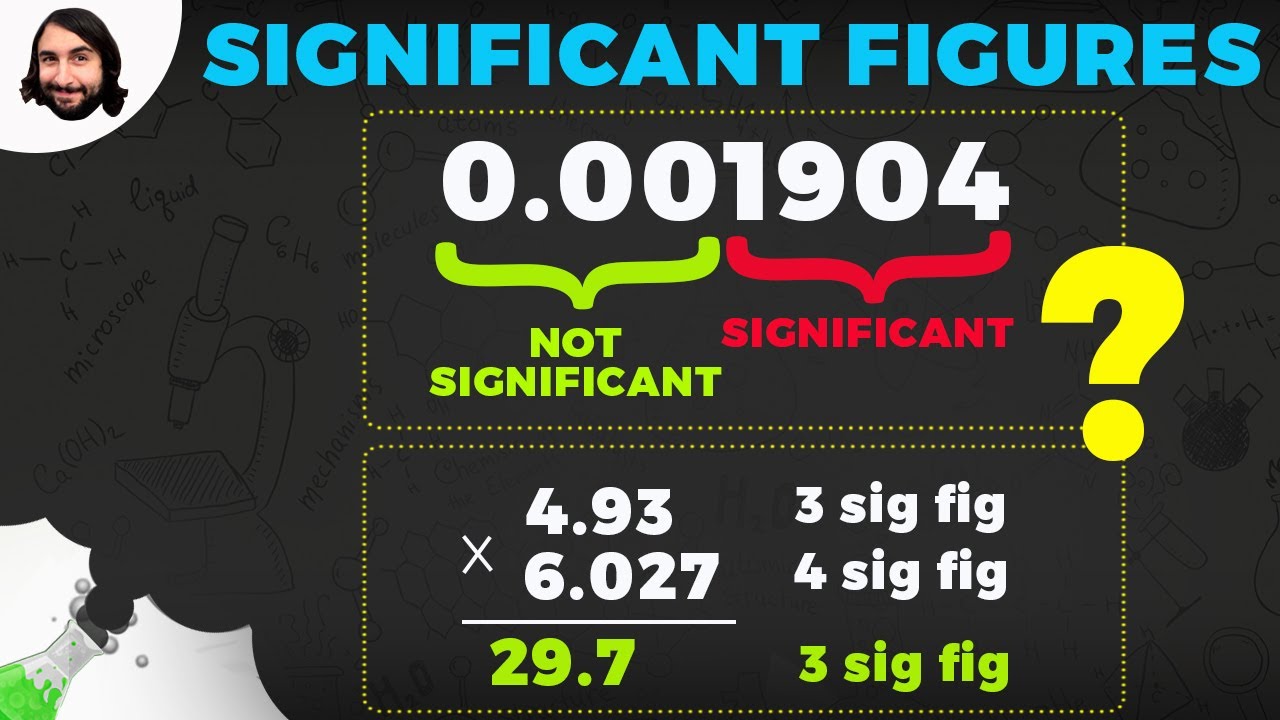
The speaker provides an example using a thermometer to illustrate the uncertainty in measurement and the need for significant figures. It is explained that a measurement cannot have more significant figures than the precision of the measuring instrument allows. Rules for identifying significant figures are presented: non-zero digits are always significant, zeros between non-zero digits are significant, and leading zeros are not significant. Trailing zeros are significant if there is a decimal point involved. The importance of these rules is emphasized for ensuring that the level of precision claimed by a measurement is realistic and meaningful.
🔁 Rounding and Scientific Notation for Significant Figures
This paragraph focuses on the rules for rounding numbers to a specific number of significant figures and the use of scientific notation. It is explained that leading zeros are ignored, and the rounding decision is based on the next significant digit. Trailing zeros are significant if they follow a decimal point. The concept of scientific notation is introduced to simplify the representation of numbers with many significant figures, with the power of ten indicated separately from the significant digits. Examples are provided to demonstrate the process of rounding and converting to scientific notation.
📝 Practice with Significant Figures and Exact Numbers
The speaker presents a series of problems to practice identifying exact numbers and counting significant figures. Various scenarios are given, such as the mass of an index card, the number of ounces in a pound, and the volume of a large coffee, to determine whether the quantities are exact or measured. The participant is then guided through the process of underlining significant zeros and counting significant figures in different numbers. The importance of practice in mastering the concept of significant figures is highlighted.
🧮 Rounding to Significant Figures and Scientific Notation
The paragraph concludes with a set of problems that involve rounding numbers to five significant figures and then rewriting them in scientific notation. The process of rounding is demonstrated, where the decision to round up or down is made based on the digit following the fifth significant figure. Once rounded, the numbers are converted into scientific notation by placing the decimal after the first non-zero digit and adjusting the power of ten accordingly. The examples provided illustrate the correct application of rounding rules and the use of scientific notation for expressing numbers with a specific number of significant figures.
🚀 Recap and Transition to Calculations with Significant Figures
In the final paragraph, the speaker recaps the lesson's content, which includes the concepts of accuracy, precision, measurements, significant figures, and exact numbers. The importance of counting significant figures and understanding their implications for the precision of measurements is emphasized. The speaker then transitions to the next lesson, which will focus on performing calculations with significant figures and determining where to round the answers based on the significant figures in the original numbers.
Mindmap
Keywords
💡Significant Figures
💡Precision
💡Accuracy
💡True Value
💡Exact Numbers
💡Measured Numbers
💡Leading Zeros
💡Trailing Zeros
💡Scientific Notation
💡Rounding
💡Measurement
Highlights
The lesson explains the concepts of accuracy and precision, which are often confused but have distinct meanings in science.
Accuracy refers to how closely repeated measurements agree with the true value, while precision is about how closely repeated measurements agree with each other.
The instructor uses the analogy of a dartboard to illustrate the difference between high and low accuracy and precision.
High accuracy means measurements are close to the true value, while high precision means measurements are clustered closely together.
If measurements are clustered but far from the true value, it represents a situation of low accuracy but high precision.
The lesson emphasizes the importance of understanding the difference between exact numbers and measured numbers.
Exact numbers like counts or defined conversion factors do not have uncertainty, while measured numbers do.
The instructor provides examples of exact numbers, such as the number of eggs in a dozen or the number of inches in a mile.
Significant figures are used to quantify the precision of a measured value.
Non-zero digits are always significant, while leading zeros are not.
Zeros between non-zero digits are significant, as are trailing zeros if there is a decimal point.
The instructor provides rules and examples for counting significant figures in different types of numbers.
Scientific notation can be used to avoid ambiguity when counting significant figures.
The lesson includes many practice problems for identifying significant figures and rounding numbers.
The instructor emphasizes the importance of rounding calculated answers to the correct number of significant figures.
The next lesson will cover how to perform calculations using significant figures and round answers appropriately.
Overall, the lesson provides a clear and easy-to-understand explanation of accuracy, precision, exact numbers, and significant figures.
Transcripts
Browse More Related Video

2.3 Precision and Accuracy | High School Chemistry
Measurement and Significant Figures

Significant Figures Step by Step | How to Pass Chemistry

High School Physics: Significant Figures

College Physics 1: Lecture 3 - Significant Figures and Scientific Notation

Unit Conversion & Significant Figures: Crash Course Chemistry #2
5.0 / 5 (0 votes)
Thanks for rating: