Significant Figures Made Easy!

The Organic Chemistry Tutor

8 Sept 201696:02

EducationalLearning

32 Likes 10 Comments

TLDRThis educational video script offers a comprehensive guide on significant figures, detailing how to count, round, and apply them in arithmetic operations. It covers addition, subtraction, multiplication, division, and scientific notation, providing step-by-step examples to illustrate the rules. The script emphasizes the importance of aligning significant figures correctly and rounding to the appropriate number of significant figures, ensuring accuracy in scientific calculations.

Takeaways

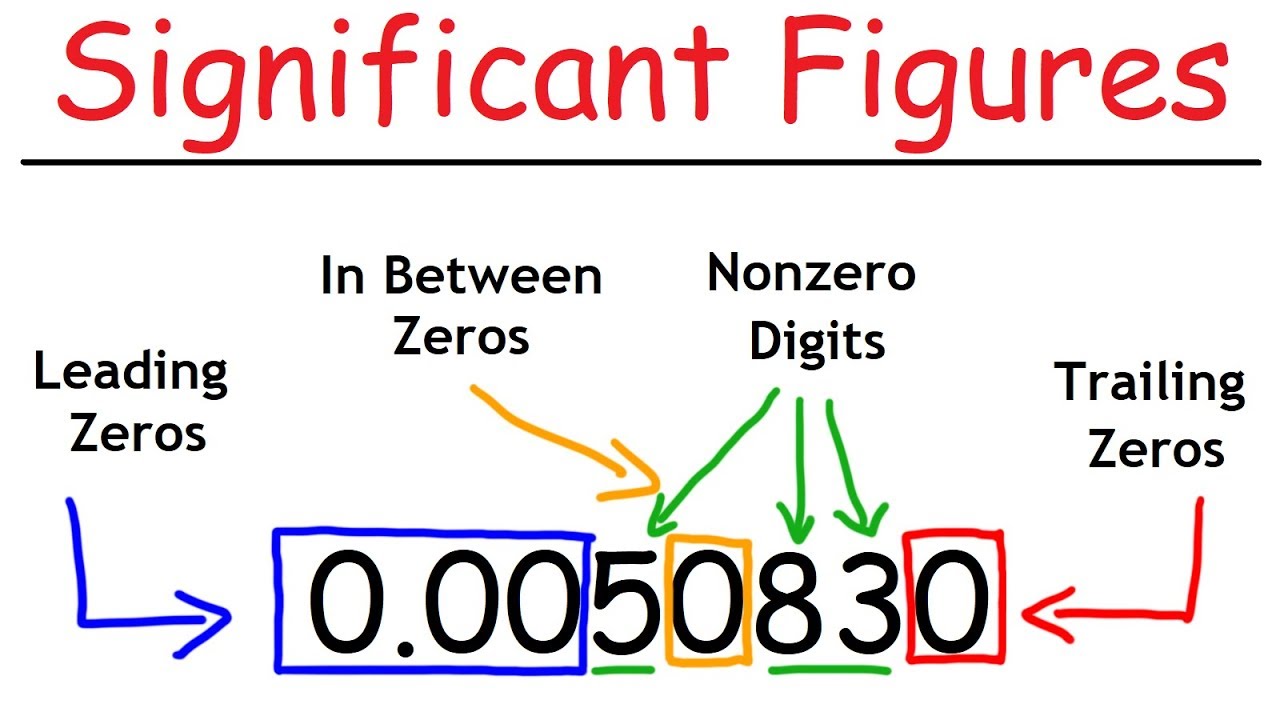
📏 Any nonzero number is counted as a significant figure. For example, 126 has three significant digits.
🔢 Zeros between nonzero digits are always significant. For instance, 404 has three significant figures.
🔍 Trailing zeros are not significant unless there is a decimal point. For example, 8000 has one significant figure, but 8000.0 has five.
📐 Leading zeros are not significant. For instance, 0.004 has one significant figure.
🧮 In scientific notation, only the digits before the multiplier (10^n) are significant. For example, 2.53 x 10^4 has three significant figures.
🔄 When rounding, if the next digit is 5 or greater, round up. Otherwise, round down. For example, 4.25716 rounded to two significant figures is 4.3.
➕ When adding or subtracting, align the numbers by their decimal points and round to the least number of decimal places in the given numbers.
✖️ When multiplying or dividing, round the final answer to the least number of significant figures present in any of the original numbers.
🔢 In combined operations, always perform calculations with the exact values first and round the final result according to the appropriate rules.
🔍 In scientific notation, adjust the decimal point to ensure the number remains between 1 and 10, adjusting the exponent accordingly. For example, 3.65 x 10^5 becomes 365000.

Q & A

What defines a significant figure in a number?
-A significant figure includes all non-zero digits, zeros between non-zero digits, and trailing zeros if there is a decimal point.
How many significant figures does the number 404 have, and why?
-The number 404 has three significant figures because the zeros between the non-zero digits are always counted as significant.
Are trailing zeros significant in the number 8000, and why or why not?
-In the number 8000, trailing zeros are not significant if there is no decimal point. Therefore, 8000 has one significant figure.
How do trailing zeros affect the significant figures in numbers with and without a decimal point?
-Trailing zeros are not significant if there is no decimal point (e.g., 8000 has one significant figure). However, if there is a decimal point, all trailing zeros become significant (e.g., 8000. has four significant figures).
Explain how to determine the number of significant figures in a number like 0.00153.
-Leading zeros are not counted as significant figures. Thus, 0.00153 has three significant figures (1, 5, and 3).
When rounding a number to a specified number of significant figures, what rule should be applied?
-To round a number to a specified number of significant figures, look at the digit immediately after the last significant figure you want to retain. If this digit is 5 or greater, round up the last significant figure; if it is less than 5, round down.
How do you round 4257.16 to three significant figures?
-To round 4257.16 to three significant figures, consider the fourth digit, which is 7. Since it is greater than 5, round the third digit up from 5 to 6, resulting in 4260.
In addition and subtraction, how do you determine the number of significant figures in the result?
-For addition and subtraction, the result should be rounded to the least number of decimal places of any number in the operation.
What is the rule for significant figures when multiplying or dividing numbers?
-When multiplying or dividing, the number of significant figures in the result should match the number in the operand with the fewest significant figures.
How do you convert a number like 3.4 × 10^3 into standard notation?
-To convert 3.4 × 10^3 into standard notation, move the decimal point three places to the right, resulting in 3400.
Explain how to add numbers in scientific notation with different exponents.
-To add numbers in scientific notation with different exponents, first adjust one of the numbers so both exponents match. Then add the coefficients. For example, to add 3.6 × 10^3 and 2.3 × 10^4, convert 3.6 × 10^3 to 0.36 × 10^4 and then add: 0.36 + 2.3 = 2.66 × 10^4.
What is the process for subtracting numbers in scientific notation?
-To subtract numbers in scientific notation, align the exponents by converting one number to match the exponent of the other. Then subtract the coefficients. For instance, to subtract 7.35 × 10^2 from 5.231 × 10^3, convert 7.35 × 10^2 to 0.735 × 10^3, then subtract: 5.231 - 0.735 = 4.496 × 10^3.
How do you handle significant figures when performing combined operations like (4.31 × 10^3) × (2.0 × 10^2) / (3.0 × 10^1)?
-For combined operations, first perform the multiplication and division following the order of operations. Each step should be rounded to the least number of significant figures in the operands involved. For example, (4.31 × 10^3) × (2.0 × 10^2) gives 8.62 × 10^5 (rounded to two significant figures), then divide by 3.0 × 10^1 to get 2.9 × 10^4.
How do you average numbers with different significant figures, such as 8.4, 3.21, and 5.436?
-To average numbers, add them together and then divide by the number of values. The result should be rounded to the number of significant figures based on the smallest number of significant figures among the original values. For 8.4 (2 significant figures), 3.21 (3 significant figures), and 5.436 (4 significant figures), the average is 5.682, rounded to 2 significant figures, resulting in 5.7.

Outlines

00:00

🔢 Introduction to Significant Figures

The video begins with an introduction to significant figures, explaining how to count them in various numerical formats, including whole numbers and decimals. It clarifies that any non-zero number counts as a significant figure, and zeros between non-zero numbers are also significant. The video provides examples to illustrate the counting of significant figures and discusses the importance of trailing zeros in the context of decimals and whole numbers.

05:02

📉 Rounding Significant Figures

This section of the video focuses on the process of rounding numbers to a specific number of significant figures. It explains the rounding rules, emphasizing the importance of the uncertain digit and how to decide whether to round up or down based on the next digit. The video provides several examples, including rounding large numbers and small numbers, and discusses the impact of rounding on the accuracy of the final result.

10:03

🧪 Scientific Notation and Significant Figures

The video script explains the concept of scientific notation and its relation to significant figures. It demonstrates how to express numbers in scientific notation and how to determine the number of significant figures in such expressions. The video also addresses the rounding of numbers in scientific notation, providing examples to show how multipliers in the notation affect the count of significant figures.

15:04

🔍 Advanced Rounding Scenarios

This part of the video tackles more complex scenarios involving rounding. It discusses situations where the number of significant figures in the final answer is less than the number of digits in the original number, such as when rounding 100 to two significant figures. The video introduces the use of scientific notation as a tool for managing significant figures in such cases, with examples to illustrate the process.

20:06

➕ Addition and Significant Figures

The script covers the rules for adding numbers while considering significant figures. It explains how to align numbers for addition, calculate the exact sum, and then round the result to the correct number of significant figures based on the least significant digit. The video provides multiple examples, including adding numbers with varying numbers of decimal places, and discusses the technique of drawing a line to determine the rounding position.

25:07

➖ Subtraction and Significant Figures

This section of the video script discusses the process of subtraction with regard to significant figures. It parallels the rules for addition, showing how to perform the exact subtraction and then round the result to the appropriate number of significant figures. The video illustrates this with examples, emphasizing the importance of identifying the uncertain digit for accurate rounding.

30:10

✖️ Multiplication and Division with Significant Figures

The video script explains the rules for multiplication and division when dealing with significant figures. It states that the final answer should be rounded to the least number of significant figures present in the individual numbers involved in the operation. The video provides examples of both multiplication and division, showing how to calculate the exact result and then round it to the correct number of significant figures.

35:11

🔢 Combined Operations and Significant Figures

This part of the video deals with combined mathematical operations and their impact on significant figures. It provides examples of sequences involving multiplication, addition, and division, showing how to keep track of significant figures at each step. The video emphasizes the importance of using exact values for intermediate steps and rounding only the final answer to maintain accuracy.

40:12

🌐 Scientific Notation in Combined Operations

The script introduces the use of scientific notation in combined operations, explaining how to convert between standard and scientific notation. It provides examples of adding and subtracting numbers in scientific notation, emphasizing the need to align the exponents and add the coefficients. The video also discusses the process of rounding the results of these operations to the appropriate number of significant figures.

45:13

🏁 Conclusion and Final Thoughts

In the final part of the video, the script wraps up the discussion on significant figures in various mathematical operations. It reiterates the importance of rounding to the correct number of significant figures and provides a final example involving division in scientific notation. The video concludes by summarizing the key points covered and wishing the viewers a great day.

Mindmap

Keywords

💡Significant Figures

Significant figures are the digits in a number that carry meaning contributing to its precision. In the video, the concept is central to understanding how to accurately represent the precision of measurements and results in science and mathematics. For example, the script explains that any non-zero digit is counted as a significant figure, and zeros between non-zero digits are also significant.

💡Rounding

Rounding is the process of adjusting a number to a certain number of significant figures or decimal places. The video script discusses various scenarios where rounding is necessary, such as when adding, subtracting, multiplying, or dividing numbers, and how to determine whether to round up or down based on the digit following the last significant figure.

💡Scientific Notation

Scientific notation is a way of expressing numbers as a product of a number between 1 and 10 and a power of 10, which simplifies calculations with very large or very small numbers. The video explains how to convert numbers into and out of scientific notation, and how it affects the counting of significant figures.

💡Decimal Point

The decimal point is used to indicate the separation between the integer and fractional parts of a number. In the context of the video, the position of the decimal point is crucial when determining significant figures, especially in numbers with trailing zeros or in scientific notation.

💡Leading Zeros

Leading zeros are zeros that precede all the non-zero digits of a number. The video script clarifies that leading zeros are not counted as significant figures unless there is a decimal point involved, which affects the counting of significant figures.

💡Trailing Zeros

Trailing zeros are zeros that follow the last non-zero digit of a number. The video explains that trailing zeros are not significant unless there is a decimal point, which makes them significant and must be considered when counting significant figures.

💡Addition and Subtraction

The video script provides examples of how to perform addition and subtraction while keeping track of significant figures. It explains the process of aligning numbers properly and determining the uncertain digit in the result, which dictates the number of significant figures in the final answer.

💡Multiplication and Division

The script discusses the rules for rounding numbers when performing multiplication and division. It emphasizes that the final answer should be rounded to the least number of significant figures present in the numbers being multiplied or divided, ensuring the precision of the result.

💡Order of Operations

The order of operations, often remembered by the acronym PEMDAS (Parentheses, Exponents, Multiplication and Division, Addition and Subtraction), dictates the sequence in which operations in an expression should be performed. The video script refers to this concept when explaining how to handle complex problems involving multiple operations.

💡Uncertain Digit

The uncertain digit is the last significant figure in a number that is being rounded. The video script frequently refers to the uncertain digit when explaining the process of rounding numbers to a specific number of significant figures, which is the digit that determines whether to round up or down.

💡Exact Numbers

Exact numbers, such as divisors in the context of the video, are considered to have an infinite number of significant figures. The script mentions that when dividing by an exact number, the resulting significant figures are based on the numerator rather than the divisor, which is an exception to the usual rounding rules.

Highlights

Significant figures are crucial for scientific calculations and are counted from the first non-zero digit.

Numbers with only zeros between non-zero digits have all those zeros counted as significant figures.

Trailing zeros without a decimal point are not significant, but they become significant if a decimal point is present.

Scientific notation allows for the accurate counting of significant figures irrespective of the number's size.

Rounding numbers to a specific number of significant figures involves looking at the next digit and applying the rule of rounding up if it's 5 or more.

When adding or subtracting numbers, the number of significant figures in the result is determined by the least significant digit among the numbers involved.

Multiplication and division of numbers require rounding the result to the least number of significant figures present in the numbers being operated on.

In complex operations, it's essential to perform intermediate steps accurately and keep track of significant figures to avoid rounding errors.

When expressing numbers in scientific notation, the decimal point's position affects the exponent, with leftward movements increasing the exponent.

Converting between standard and scientific notation involves moving the decimal point相应 to the exponent's value.

Adding numbers in scientific notation with different multipliers requires adjusting the exponents to match before adding the coefficients.

Subtraction in scientific notation is similar to addition, with the need to align the numbers by their significant figures and exponents.

Performing division with scientific notation requires careful handling of exponents, subtracting them when dividing the bases.

When averaging numbers, the result should be rounded to the same number of significant figures as the original data, treating the divisor as having an infinite number of significant figures.

The video provides a comprehensive guide on handling significant figures in various mathematical operations, ensuring accuracy in scientific computations.

Practical examples demonstrate the step-by-step process of rounding and calculating with significant figures in addition, subtraction, multiplication, and division.

The importance of using exact values in intermediate steps of calculations is emphasized to maintain accuracy before final rounding.

The video concludes with a summary of the key points regarding significant figures, reinforcing the rules and techniques demonstrated throughout.

Transcripts

Browse More Related Video

03 - Significant Figures Rules (Sig Fig Rules) for Calculations in Chemistry & Physics

Rounding and Order of Operations Used in Significant Figures

Significant Figures - A Fast Review!

College Physics 1: Lecture 3 - Significant Figures and Scientific Notation

2.1 Significant Figures | High School Chemistry

Mass balance and Significant Figures

Related Tags

Significant Figures Mathematics Guide Rounding Rules Scientific Notation Addition Subtraction Multiplication Division Educational Content Math Skills Decimal Points