03 - Significant Figures Rules (Sig Fig Rules) for Calculations in Chemistry & Physics
TLDRThe video script provides an in-depth tutorial on the concept of significant figures in chemistry calculations. It explains the importance of accurate measurement representation through significant digits, using real-world examples to illustrate the concept. The script outlines rules for counting significant figures, emphasizing the exclusion of leading zeros before a decimal point and the inclusion of trailing zeros. It further clarifies the application of these rules in arithmetic operations, such as multiplication, division, addition, and subtraction, and the use of scientific notation to avoid ambiguity. The emphasis is on aligning the precision of the final answer with the precision of the input data, ensuring logical and scientifically valid results in chemistry calculations.
Takeaways
- 📏 The concept of significant figures is crucial in chemistry as it reflects the accuracy of measured data used in calculations.
- 🔢 The number of significant figures in a number is determined by counting all non-zero digits, including those after a decimal point.
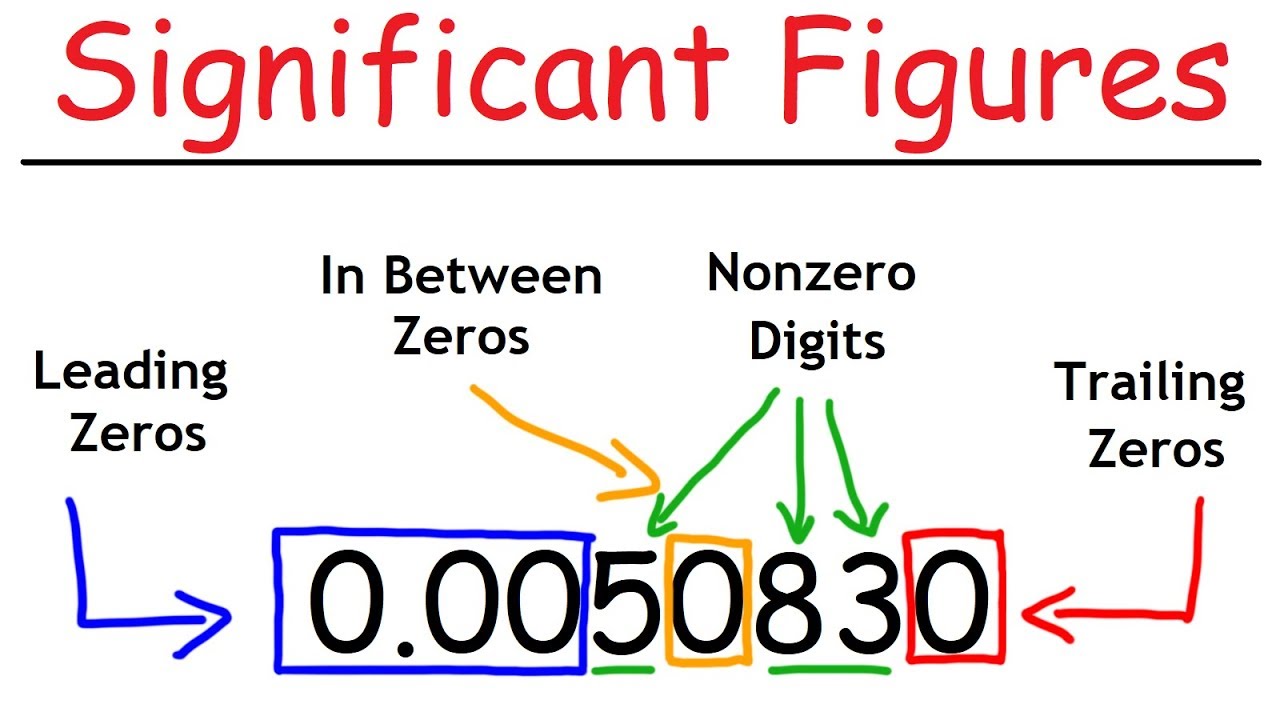
- 🚫 Leading zeros before a decimal point are not counted as they do not add to the accuracy of the measurement.
- 📉 Trailing zeros after a decimal point and after non-zero digits are counted because they indicate the precision of the measurement.
- 🌟 A zero between two significant digits is considered significant as it contributes to the number's precision.
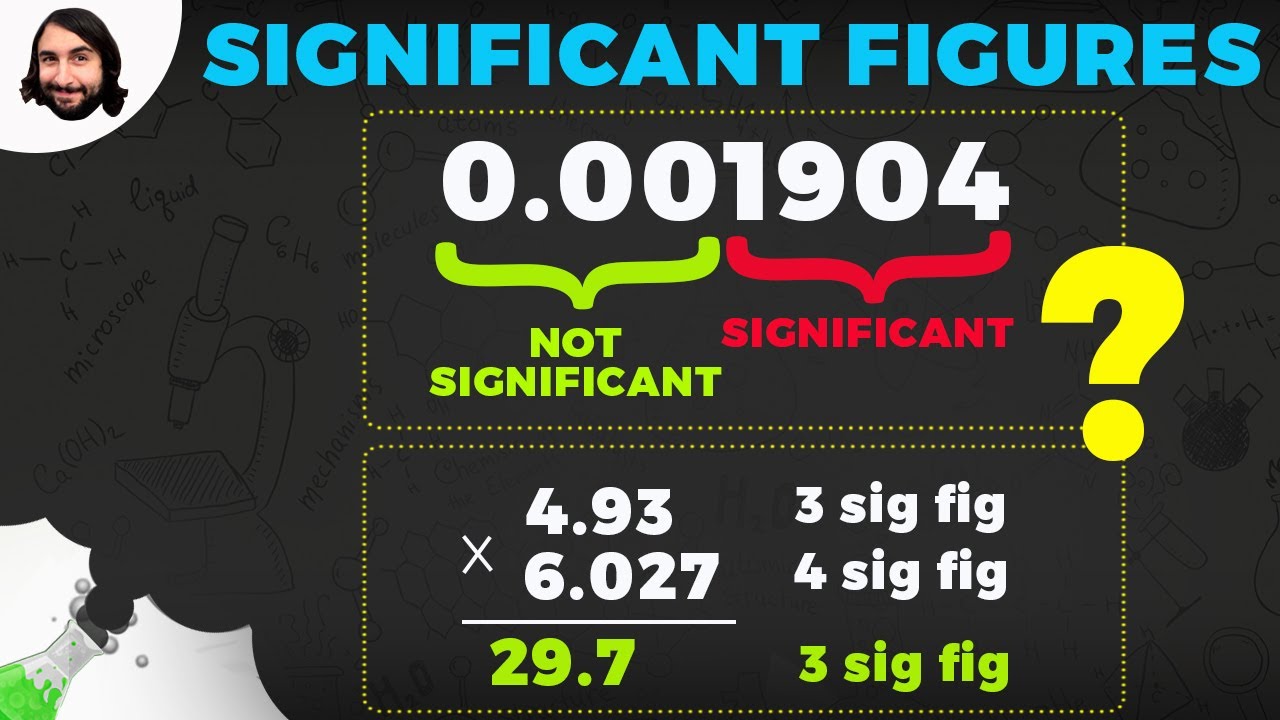
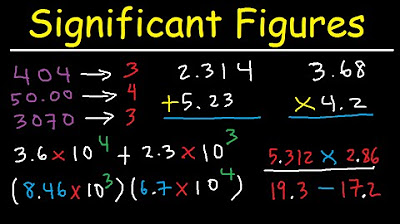
- 🔄 When performing multiplication and division, the answer should have the same number of significant figures as the number with the fewest significant figures in the problem.
- ➕➖ For addition and subtraction, the answer should have the same number of digits after the decimal point as the number with the least number of digits after the decimal in the problem.
- 🎓 Scientific notation is used to express very large or very small numbers and counts all digits, including the coefficient and the power of 10, as significant.
- 📝 When in doubt about the significance of trailing zeros in a whole number, it's best to express the number in scientific notation to avoid ambiguity.
- 📊 Significant figures are not applicable to counted quantities as they represent exact numbers without measurement uncertainty.
- 📈 Practice is essential for mastering the rules of significant figures, which will be consistently applied throughout chemistry calculations.
Q & A
What is the main topic of the script?
-The main topic of the script is the concept of significant figures or significant digits in chemistry calculations.
Why is it important to consider significant figures in calculations?
-It is important to consider significant figures in calculations because the final answer should reflect the accuracy of the measurements used in the problem, ensuring that the result is representative of the input data.
What is a real-world example given in the script to illustrate the importance of significant figures?
-A real-world example given in the script is the measurement of speed in a car or train, where the speed is measured as 10.82 meters per second, highlighting the implied accuracy of the measurement.
How does the accuracy of measured time affect the calculation of distance traveled?
-The accuracy of measured time directly affects the calculation of distance traveled because the distance is determined by multiplying the speed by the time. If the time is measured more accurately (more decimal places), it impacts the number of significant figures in the final distance calculation.
What is the rule for counting significant figures in a number with a leading zero before a decimal point?
-The rule for counting significant figures in a number with a leading zero before a decimal point is that the leading zero is not counted as it does not add any measurement accuracy to the number.
How are trailing zeros after a decimal point treated when counting significant figures?
-Trailing zeros after a decimal point are counted as significant figures because they indicate the level of precision of the measuring instrument or the accuracy of the measurement.
What is the rule for counting zeros that are sandwiched between other significant digits?
-Zeros that are sandwiched between other significant digits are counted as significant because they contribute to the magnitude of the number and indicate the accuracy of the measurement.
How does scientific notation affect the counting of significant figures?
-In scientific notation, only the digits before the power of 10 are counted for significant figures. The exponent part (the 10 to the power of n) does not affect the count of significant figures.
What is the rule for significant figures in counting quantities?
-Counting quantities, such as counting objects, are considered exact numbers and significant figures do not apply. The count is perfect and does not involve measurement uncertainty.
How does the script suggest handling situations where there is ambiguity in the number of significant figures?
-The script suggests using scientific notation in situations where there is ambiguity in the number of significant figures, as it removes any uncertainty by clearly indicating the precision of the measurement.
What is the general rule for calculating with significant figures in multiplication and division?
-In multiplication and division, the answer should have the same number of significant figures as the number with the fewest significant figures in the problem.
What is the general rule for calculating with significant figures in addition and subtraction?
-In addition and subtraction, the answer should have the same number of digits after the decimal point as the number with the least number of digits after the decimal point in the problem.
Outlines
📘 Introduction to Significant Figures
This paragraph introduces the concept of significant figures or digits, emphasizing their importance in calculations, especially in chemistry. It explains that the precision of the final answer in any calculation should depend on the precision of the numbers used in the problem. The paragraph uses a real-world example of measuring speed to illustrate the concept, highlighting the need to consider the accuracy of the measuring tools and the implications of including unnecessary decimal places in the final answer.
📙 Understanding Significant Figures in Calculations
This section delves deeper into the rules of significant figures, using examples to clarify when to count zeros and non-zero digits in a number. It explains that leading zeros before a decimal point are not counted, while trailing zeros after a decimal point are counted. The paragraph also addresses the treatment of zeros between other significant digits and provides guidance on how to handle scientific notation in the context of significant figures. The importance of accurate measurement representation is stressed, and the concept is related to practical applications in chemistry and real-life scenarios.
📙 Counting Significant Figures in Numbers
This paragraph focuses on the rules for counting significant figures in various types of numbers, including those with leading and trailing zeros. It explains that zeros before a decimal point do not count unless they are between significant figures. The paragraph also clarifies that trailing zeros after a decimal point are counted because they indicate the precision of the measuring instrument. Additionally, it highlights the significance of zeros between non-zero digits, which contribute to the accuracy of the number. The explanation is supported by examples that demonstrate how to correctly count significant figures in different scenarios.
📘 Applying Significant Figures in Arithmetic Operations
This section explains how to apply the rules of significant figures when performing arithmetic operations such as addition, subtraction, multiplication, and division. It provides specific examples to illustrate the process of determining the number of significant figures to keep in the final answer. The paragraph emphasizes that the answer should reflect the level of accuracy of the least precise number in the calculation. It also addresses the特殊情况 of scientific notation and counting significant figures in this format, reinforcing the importance of maintaining the correct number of significant figures to ensure accurate and meaningful results in calculations.
📙 Rounding and Expressing Final Answers
This paragraph discusses the challenges of rounding numbers to the appropriate number of significant figures, particularly when zeros are involved. It offers strategies for handling situations where the number of significant figures is ambiguous, such as when a whole number is followed by zeros. The paragraph suggests using scientific notation as a reliable method to express final answers unambiguously, ensuring that the intended number of significant figures is clear. The explanation includes examples that demonstrate how to convert numbers into scientific notation and the benefits of doing so in terms of clarity and accuracy.
Mindmap
Keywords
💡Significant Figures
💡Measurement Accuracy
💡Decimal Point
💡Trailing Zeros
💡Leading Zeros
💡Scientific Notation
💡Counting Significant Figures
💡Measurement Tools
💡Rounding
💡Zero Sandwiched Between Significant Figures
💡Real-World Example
Highlights
The importance of understanding significant figures in chemistry calculations is emphasized.
The concept of significant figures is introduced with a real-world example of measuring speed.
The rule that the final answer should reflect the accuracy of the least accurate number used in the calculation is explained.
The method of counting significant figures is outlined, including the treatment of zeros in different contexts.
An example is provided to illustrate how to calculate distance traveled based on speed and time, emphasizing the proper use of significant figures.
The significance of the decimal point in counting significant figures is discussed.
The treatment of leading zeros before a decimal point as non-significant is clarified.
The counting of trailing zeros after a decimal point as significant is explained.
The example of counting significant figures in scientific notation is provided.
The rule that significant figures only apply to measured quantities, not to counted quantities, is highlighted.
Guidance on how to handle ambiguity in significant figures with zeros is given, suggesting the use of scientific notation.
Instructions for multiplying and dividing numbers while considering significant figures are provided.
The method for calculating significant figures in addition and subtraction problems is detailed.
The impact of the least accurate number on the final answer in calculations is reiterated.
The importance of practice in mastering the use of significant figures in chemistry is emphasized.
The transcript concludes with an encouragement to review the section as needed to fully grasp the concept of significant figures.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: