STANDARD EQUATION OF A CIRCLE IN A GENERAL FORM

WOW MATH
9 Oct 202204:53
EducationalLearning
32 Likes 10 Comments

TLDRThe transcript from Mr. Rogan's class delves into the mathematical concept of a circle, defined as a set of points equidistant from a center with a constant radius. The lesson covers the expansion of binomial squares and the rearrangement of equations into a general form. It includes examples like converting the equation \((x+1)^2 + (y+3)^2 = 5\) into a standard circle equation, and other similar exercises, highlighting the process of simplifying and solving for constants to represent geometric shapes.

Takeaways
  • 📚 The script discusses the concept of a circle, emphasizing that it consists of all points equidistant from a central point, known as the center.
  • 📐 The constant distance from the center to any point on the circle is called the radius.
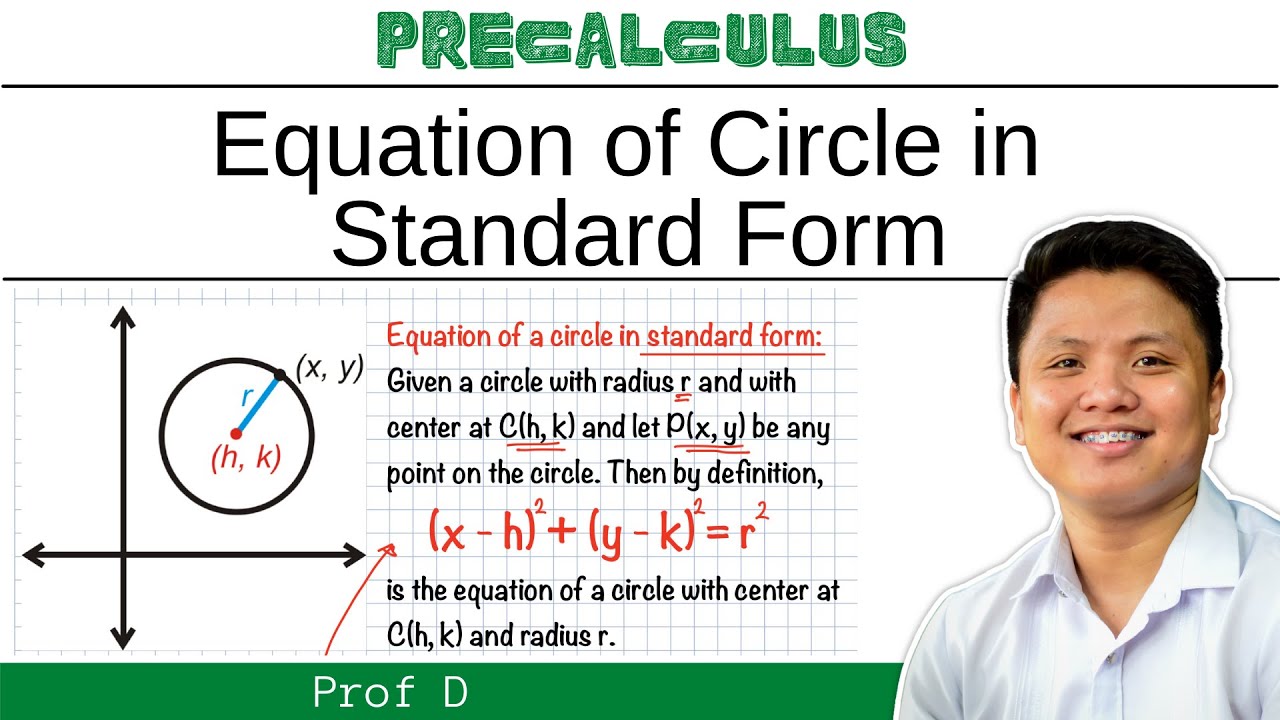
  • 🔍 The script provides a mathematical equation representing a circle: \((x + 1)^2 + (y + 3)^2 = 5\).
  • 📈 The process of expanding the binomial square is explained step by step in the script.
  • 🔢 The script demonstrates how to square the first and second terms and multiply them accordingly.
  • ✂️ The script shows the rearrangement of terms to form a general equation equated to zero.
  • 🧩 The script includes combining like terms to simplify the equation.
  • 📝 Another circle equation is presented: \(y^2 + 3y + 3^2 = 1\), with a similar process of expansion and simplification.
  • 📉 A third circle equation is given: \(x^2 + (y^2) = 36\), and the script explains how to expand and rearrange it.
  • 🔄 The final equation discussed is \(x^2 - 8x + y^2 + 4y + 16 + 4 = 10\), illustrating the process of combining and simplifying terms.
  • 📊 The script concludes with the simplified form of the last equation, highlighting the method of solving for a circle's equation.
Q & A

  • What is the definition of a circle?

    -A circle consists of all points on the plane equidistant from a fixed point, called the center. The distance from the center to any point on the circle is constant and is called the radius of the circle.

  • What is the equation of the circle given in the script?

    -The equation of the circle given is (x + 1)^2 + (y + 3)^2 = 5.

  • What is the first step to expand the equation (x + 1)^2 + (y + 3)^2 = 5?

    -The first step is to expand the square of the binomial. For (x + 1)^2, you square the first term (x^2), multiply the first and last term (x * 1 * 2 = 2x), and square the second term (1^2 = 1).

  • How do you expand (y + 3)^2?

    -To expand (y + 3)^2, you square the first term (y^2), multiply the first and last term (y * 3 * 2 = 6y), and square the second term (3^2 = 9).

  • How do you combine like terms after expanding the binomials?

    -Combine like terms by adding the x^2, 2x, y^2, 6y, and the constants from both binomials. This gives x^2 + 2x + y^2 + 6y + 10 = 5.

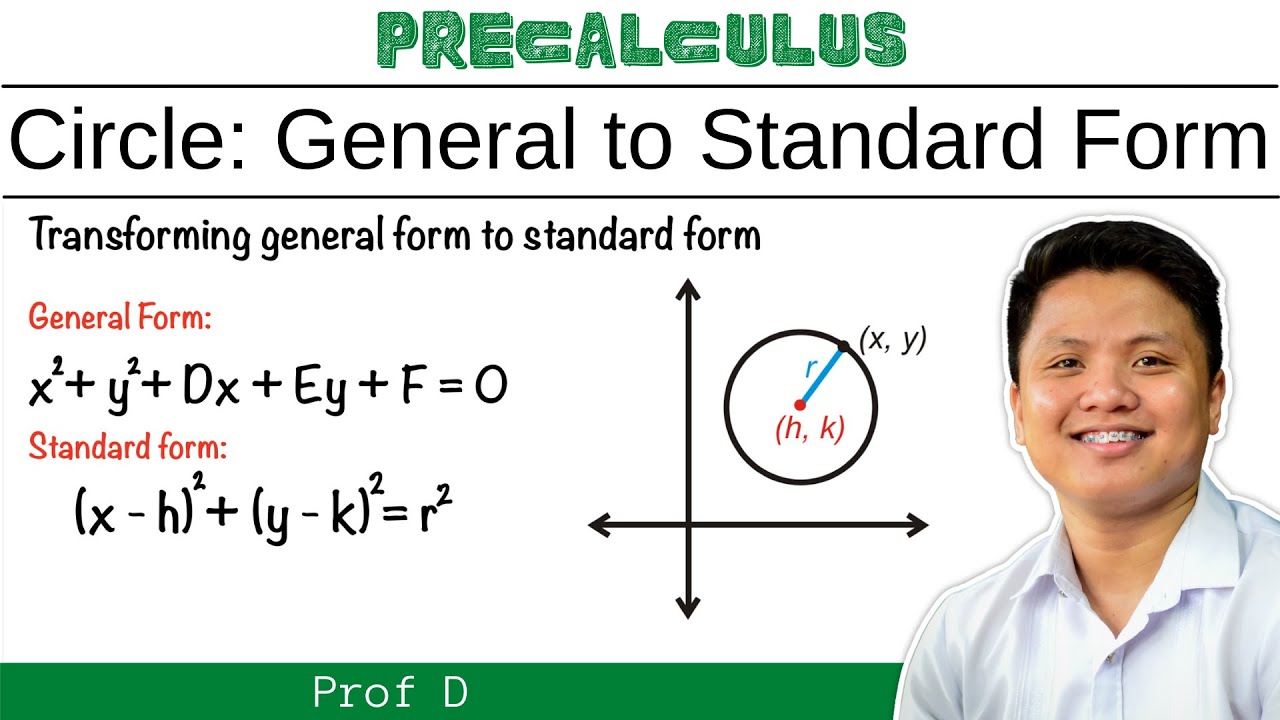
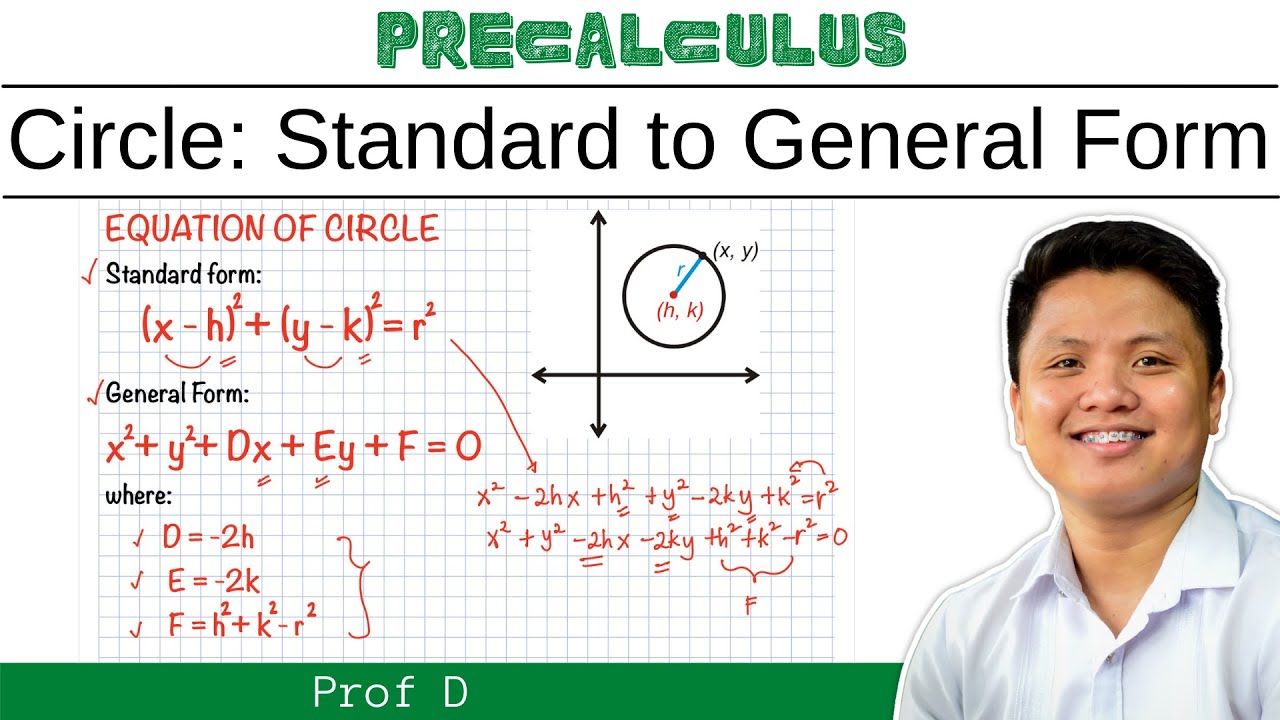
  • What is the general form of the equation after rearranging and combining like terms?

    -The general form of the equation after rearranging and combining like terms is x^2 + y^2 + 2x + 6y + 5 = 0.

  • What is the expanded form of (y + 3)^2 for the second example in the script?

    -The expanded form of (y + 3)^2 is y^2 + 6y + 9.

  • How do you simplify the equation (x + 1)^2 + y^2 = 36?

    -First, expand (x + 1)^2 to get x^2 + 2x + 1. Then rearrange to get x^2 + y^2 + 2x + 1 - 36 = 0, which simplifies to x^2 + y^2 + 2x - 35 = 0.

  • What is the expanded form of (x - 4)^2 in the script?

    -The expanded form of (x - 4)^2 is x^2 - 8x + 16.

  • How do you combine the terms in the equation (x - 4)^2 + (y + 2)^2 = 10?

    -Expand (x - 4)^2 to x^2 - 8x + 16 and (y + 2)^2 to y^2 + 4y + 4. Combine like terms to get x^2 + y^2 - 8x + 4y + 20 - 10 = 0, which simplifies to x^2 + y^2 - 8x + 4y + 10 = 0.

Outlines
00:00
📚 Understanding Circles and Equations

The first paragraph introduces the concept of a circle, explaining that it consists of all points on a plane equidistant from a fixed center point, with a constant distance known as the radius. The paragraph then delves into the mathematical representation of a circle, using the equation \((x+1)^2 + (y+3)^2 = 5\) as an example. It outlines the process of expanding the binomial, combining like terms, and rearranging the equation into a general form to solve for the circle's parameters.

Mindmap
Keywords
💡Circle
A circle is a two-dimensional shape consisting of all points on a plane that are equidistant from a fixed point known as the center. In the video, circles are discussed in the context of mathematical equations, which describe their boundaries and properties. The concept of a circle is central to the theme of the video as it is the primary shape being explored through algebraic expressions.
💡Center
The center of a circle is the fixed point from which all points on the circle are equidistant. It is a fundamental concept in defining a circle and is used in the video to explain the relationship between the points on the circle and the distance from the center, which is constant and known as the radius.
💡Radius
The radius of a circle is the constant distance from the center to any point on the circumference of the circle. It is a key parameter in circle equations and is used in the video to describe the size of the circle. The radius is squared in the equations to form part of the circle's equation, which is a common mathematical representation.
💡Equation
An equation in mathematics is a statement that expresses the equality of two expressions, which often include variables. In the context of the video, equations are used to represent the circle's geometric properties algebraically. The script involves expanding and rearranging these equations to understand the characteristics of the circles described.
💡Binomial
A binomial is a polynomial with two terms, which is relevant in the video when discussing the expansion of expressions like (x + 1)^2 or (y + 3)^2. The process of expanding a binomial is demonstrated in the script, showing how each term within the parentheses is squared and combined to form a complete equation representing a circle.
💡Expanding
Expanding in the context of the video refers to the process of breaking down a binomial raised to a power into its constituent parts. This is done by applying the distributive property (also known as FOIL - First, Outer, Inner, Last) to multiply each term within the parentheses by each other. The script demonstrates this process for several binomials to derive the equations of circles.
💡Combining Like Terms
Combining like terms is a mathematical process where terms in an equation that have the same variable and exponent are added or subtracted to simplify the equation. In the video, this process is used to simplify the expanded binomials and rearrange the circle equations into a more standard form, making them easier to interpret and analyze.
💡General Form
The general form of a circle's equation is a way of expressing the circle's properties algebraically, typically written as x^2 + y^2 + Dx + Ey + F = 0. In the video, the general form is used to represent the circle equation after expanding and simplifying the binomials. It helps in identifying the coefficients that define the circle's center and radius.
💡Constant
A constant in mathematics is a value that does not change. In the context of the video, constants are used in the circle equations to represent the fixed values that, when subtracted from the sum of the squared terms, result in the equation equaling zero. Constants help define the specific characteristics of the circle, such as its position and size.
💡Squared
Squaring a term in mathematics means multiplying the term by itself. In the video, squaring is used to expand binomials and to express the distance from the center to any point on the circle (the radius) squared. Squaring is a fundamental operation in forming the equations that describe circles.
💡Algebraic Expressions
Algebraic expressions are combinations of variables, constants, and operations (like addition, subtraction, multiplication, and exponentiation). In the video, algebraic expressions are used to represent and manipulate the mathematical properties of circles. They are the core of the discussion, as they allow for the exploration of geometric shapes through algebra.
Highlights

Introduction to the concept of a circle, defined as a set of points equidistant from a center point.

Explanation of the constant distance from the center to any point on the circle, known as the radius.

Equation of a circle in the form \( (x+1)^2 + (y+3)^2 = 5 \).

Step-by-step expansion of the binomial square for the circle equation.

Combining like terms to simplify the circle equation.

Rearranging the equation into the general form \( x^2 + y^2 + 2x + 6y + 10 = 5 \).

Subtracting 5 from both sides to set the equation to zero.

Second circle equation \( x^2 + (y+3)^2 = 1 \) and its expansion.

Rearranging the second equation into the general form and setting it to zero.

Third circle equation \( (x+1)^2 + y^2 = 36 \) and its detailed expansion.

Combining terms and subtracting 36 to set the third equation to zero.

Fourth circle equation \( (x-4)^2 + (y+2)^2 = 10 \) with binomial expansion.

Combining like terms for the fourth equation and adjusting it to the general form.

Final equation setup \( x^2 + y^2 - 8x + 4y + 20 - 10 = 0 \).

Emphasizing the process of expanding and simplifying binomials in circle equations.

Highlighting the importance of combining like terms for clarity and accuracy.

Demonstration of algebraic manipulation to achieve the general form of circle equations.

Clarification on setting circle equations to zero for standard form representation.

Transcripts

Browse More Related Video

EQUATION OF CIRCLE IN STANDARD FORM | PROF D

CIRCLES || PRE-CALCULUS

CIRCLE | TRANSFORMING GENERAL FORM TO STANDARD FORM | PROF D

PART 2: CIRCLES || PRE - CALCULUS

CIRCLE | TRANSFORMING STANDARD FORM TO GENERAL FORM | PROF D

Graphing Conic Sections Part 1: Circles

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Circle GeometryEquation ExpansionMathematicsBinomial TheoremRadius ConceptPlane GeometryConstant DistanceCenter PointSquared EquationsEducational Content