CIRCLE | TRANSFORMING GENERAL FORM TO STANDARD FORM | PROF D

Prof D
22 Mar 202113:14
EducationalLearning
32 Likes 10 Comments

TLDRThis educational video demonstrates the process of converting the equation of a circle from general form to standard form. The presenter begins by explaining the general form (x^2 + y^2 + Dx + Ey + F = 0) and the standard form ((x - h)^2 + (y - k)^2 = r^2). Two examples are provided, guiding viewers through the steps of completing the square to find the center (h, k) and radius (r) of the circle. The first example involves an equation with coefficients and constants, while the second simplifies the equation by dividing all terms by two before completing the square. The video concludes with the standard form equations for both circles, offering a clear understanding of the mathematical transformation.

Takeaways
  • 📚 The video is a tutorial on transforming the equation of a circle from general form to standard form.
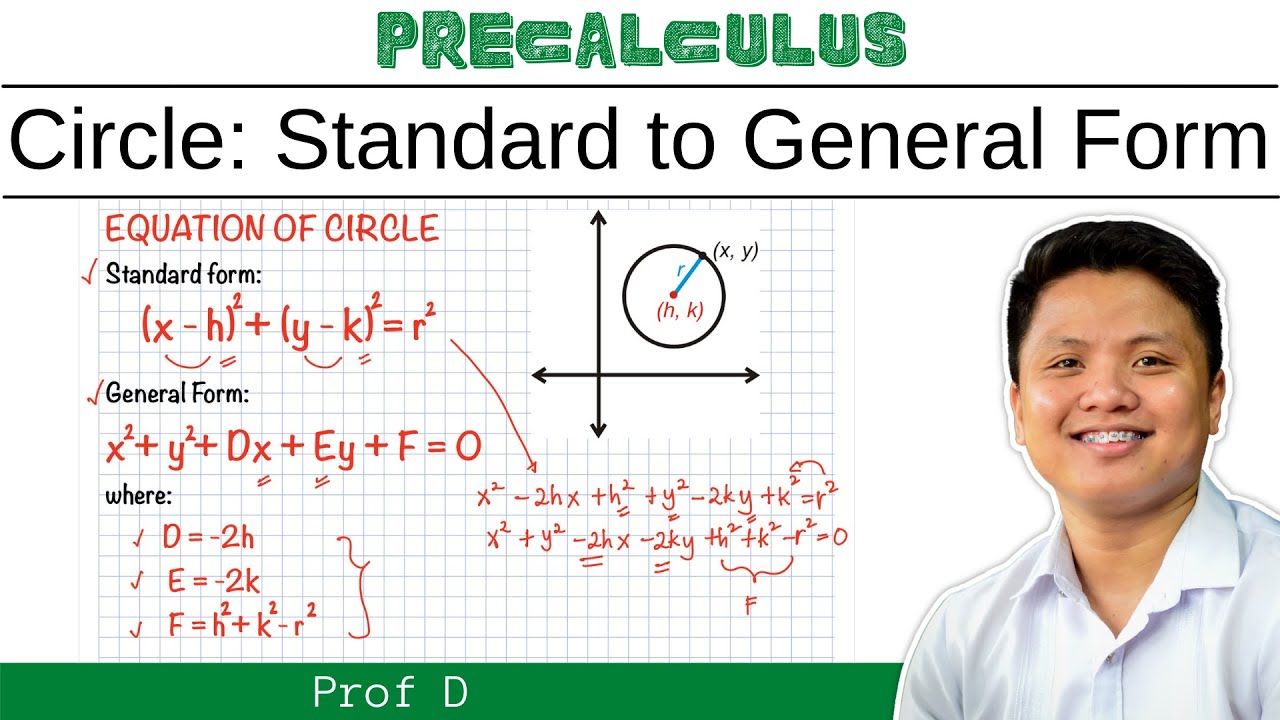
  • 🔍 The general form of a circle's equation is given as \( x^2 + y^2 + dx + ey + f = 0 \).
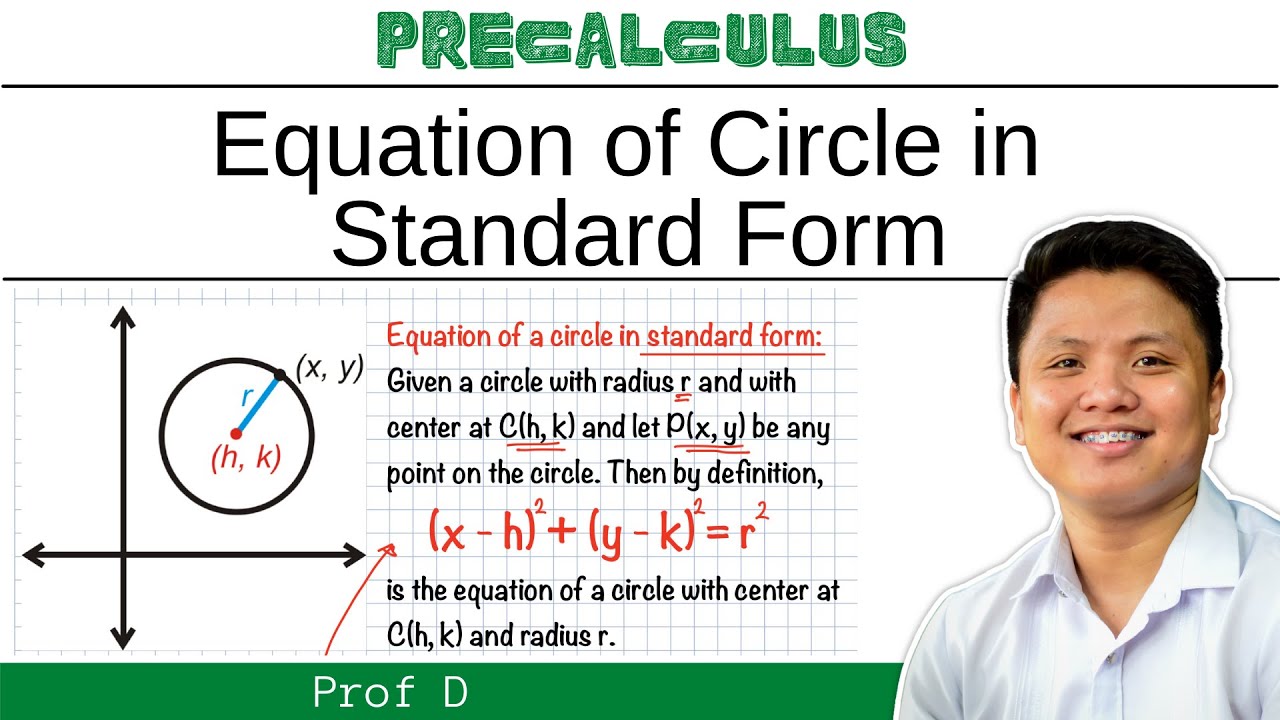
  • 📐 The standard form of a circle's equation is \( (x - h)^2 + (y - k)^2 = r^2 \), where \( (h, k) \) is the center and \( r \) is the radius.
  • 📘 The first example provided is the equation \( x^2 + y^2 + 10x - 6y - 2 = 0 \).
  • 🔢 The process involves completing the square for both \( x \) and \( y \) terms in the equation.
  • ✅ For the first example, completing the square results in the center at \( (-5, 3) \) and a radius of \( 6 \).
  • 📝 The second example provided is the equation \( 2x^2 + 2y^2 - x - 4y - 8 = 0 \).
  • 📉 The equation is simplified by dividing all terms by 2 before completing the square.
  • 🎯 After completing the square for the second example, the center is found to be at \( (2, 1) \) with a radius of \( 3 \).
  • 👨‍🏫 The presenter is Prof D, who encourages viewers to ask questions in the comments for further clarification.
  • 👋 The video concludes with a sign-off from Prof D, indicating the end of the tutorial.
Q & A

  • What is the general form of the equation of a circle?

    -The general form of the equation of a circle is x^2 + y^2 + dx + ey + f = 0.

  • What is the standard form of the equation of a circle?

    -The standard form of the equation of a circle is (x - h)^2 + (y - k)^2 = r^2, where (h, k) is the center and r is the radius.

  • What is the first step in transforming a general form equation to standard form?

    -The first step is to determine the center (h, k) and radius r of the circle by completing the square for both x and y terms.

  • How do you complete the square for the x terms in the equation?

    -You take the coefficient of x, divide it by 2, square it, and add it to both sides of the equation.

  • How do you complete the square for the y terms in the equation?

    -You take the coefficient of y, divide it by 2, square it, and add it to both sides of the equation.

  • What is the purpose of adding the square of half the coefficient of x to both sides of the equation?

    -This step ensures that the x terms form a perfect square trinomial, which is necessary for converting to the standard form of a circle's equation.

  • What is the purpose of adding the square of half the coefficient of y to both sides of the equation?

    -This step ensures that the y terms form a perfect square trinomial, which is necessary for converting to the standard form of a circle's equation.

  • How do you find the center (h, k) of the circle from the general form equation?

    -After completing the square, the center (h, k) can be found from the terms (x - h) and (y - k) in the standard form equation.

  • How do you find the radius r of the circle from the general form equation?

    -After completing the square and simplifying, the radius r can be found from the equation (x - h)^2 + (y - k)^2 = r^2, where r is the square root of the constant term on the right side of the equation.

  • What is the example given in the script for transforming the general form to standard form?

    -The example given is the equation x^2 + y^2 + 10x - 6y - 2 = 0, which is transformed into the standard form (x - (-5))^2 + (y - 3)^2 = 6^2.

  • What is the second example provided in the script for finding the standard form of a circle's equation?

    -The second example is the equation 2x^2 + 2y^2 - x - 4y - 8 = 0, which simplifies to the standard form (x - 2)^2 + (y - 1)^2 = 3^2.

Outlines
00:00
📚 Transforming Circle Equations: General to Standard Form

This paragraph introduces the video, explaining that the tutorial will cover how to convert the general form of a circle's equation to its standard form. The general form is given as x² + y² + Dx + Ey + F = 0, and the standard form as (x - h)² + (y - k)² = r². The first example involves finding the center and radius of the circle given by the equation x² + y² + 10x - 6y - 2 = 0. The process includes grouping x and y terms, moving the constant to the other side, and completing the square for both x and y terms.

05:03
🔢 Example 1: Completing the Square

In this paragraph, the first example is continued by completing the square. The terms x² + 10x and y² - 6y are transformed by adding and subtracting the necessary values to create perfect square trinomials. The equation x² + 10x + 25 + y² - 6y + 9 = 2 + 25 + 9 is then rewritten in its standard form (x + 5)² + (y - 3)² = 36, identifying the center at (-5, 3) and the radius as 6.

10:06
✏️ Example 2: Another Circle Conversion

This paragraph introduces a second example involving the circle equation 2x² + 2y² - x - 4y - 8 = 0. The terms are divided by 2 to simplify the equation, followed by grouping the x and y terms. Completing the square for x and y terms again, the equation is rewritten in its standard form (x - 1)² + (y - 1)² = 9, with the center at (1, 1) and the radius as 3. The paragraph concludes with an invitation to leave questions in the comments and a farewell from Prof D.

Mindmap
Keywords
💡General Form
The 'General Form' of an equation refers to a mathematical expression that can represent various geometric shapes, including circles. In the context of the video, the general form of a circle's equation is given as \(x^2 + y^2 + dx + ey + f = 0\). This form is less intuitive for identifying the circle's characteristics such as its center and radius, which is why the video focuses on transforming it into a more recognizable standard form.
💡Standard Form
The 'Standard Form' is a specific way of writing the equation of a circle that makes it easier to identify its center and radius. It is defined as \((x - h)^2 + (y - k)^2 = r^2\), where \((h, k)\) is the center of the circle and \(r\) is its radius. The video's main objective is to demonstrate how to convert the general form into this standard form, which is more straightforward for analysis and interpretation.
💡Completing the Square
Completing the square is a mathematical technique used to transform a quadratic equation into a perfect square trinomial plus or minus a constant. In the video, this method is applied to both the \(x\) and \(y\) terms of the circle's equation to transition from the general form to the standard form. For instance, the script mentions adding \((\frac{b}{2})^2\) to both sides of the equation to complete the square, where \(b\) is the coefficient of the \(x\) or \(y\) term.
💡Center of the Circle
The 'Center' of a circle is the point \((h, k)\) that is equidistant from all points on the circle's circumference. The video script provides a step-by-step process to determine the center from the general form of the circle's equation. For example, after completing the square, the values of \(h\) and \(k\) are identified as the coordinates where the squared terms \((x - h)^2\) and \((y - k)^2\) equal zero.
💡Radius
The 'Radius' (\(r\)) of a circle is the distance from the center to any point on the circle's edge. In the video, once the standard form of the equation is achieved, the radius can be directly read as the square root of the constant term on the right side of the equation (\(r = \sqrt{r^2}\)). The script illustrates this by showing that after completing the square and adjusting the equation, the radius is the square root of the constant term that results from the process.
💡Coefficient
A 'Coefficient' is a numerical factor that multiplies a variable in an algebraic expression. In the context of the video, the coefficients of \(x^2\) and \(y^2\) are implicitly 1, while the coefficients of \(x\) and \(y\) are represented by \(d\) and \(e\), respectively. The script discusses how to handle these coefficients during the process of transforming the general form to the standard form of a circle's equation.
💡Binomial
A 'Binomial' is an algebraic expression that consists of two terms, typically a squared term and a linear term, such as \(x^2 + bx\). In the video, binomials are used in the context of completing the square, where the script describes how to form a perfect square trinomial from a binomial by adding and subtracting the square of half the coefficient of the linear term.
💡Trinomial
A 'Trinomial' is an algebraic expression with three terms. In the video, the term 'trinomial' is used when discussing the process of completing the square, where the goal is to transform a binomial into a perfect square trinomial. For example, the script mentions adding \((\frac{b}{2})^2\) to form a trinomial that can be expressed as a square of a binomial.
💡Constant Term
The 'Constant Term' in an algebraic expression is the term that does not contain any variables. In the context of the video, the constant term \( f \) in the general form of the circle's equation is adjusted during the process of completing the square to ensure the equation remains balanced. The script illustrates how to move and adjust this constant term to achieve the standard form of the circle's equation.
💡Example
The term 'Example' in the video script refers to the practical demonstrations provided by the instructor to illustrate the process of converting the general form of a circle's equation to the standard form. Two examples are given in the script, each walking through the steps of identifying coefficients, completing the square, and determining the center and radius of the circle.
Highlights

Introduction of transforming the equation of a circle from general form to standard form.

General form of a circle equation is x^2 + y^2 + dx + ey + f = 0.

Standard form of a circle equation is (x - h)^2 + (y - k)^2 = r^2.

Example 1: Determine the center and radius of the circle x^2 + y^2 + 10x - 6y - 2 = 0.

Explanation of completing the square method to transform the equation.

Step-by-step process of completing the square for both x and y terms.

Adding 25 to both sides for x terms and 9 for y terms to complete the square.

Determining the center of the circle as (-5, 3).

Calculating the radius of the circle to be 6.

Example 2: Find the standard form of the circle 2x^2 + 2y^2 - x - 4y - 8 = 0.

Dividing all terms by 2 to simplify the equation.

Applying the completing the square method to the simplified equation.

Adding 4 to x terms and 1 to y terms for completing the square.

Finding the center of the circle in Example 2 as (2, 1).

Calculating the radius of the circle in Example 2 to be 3.

Conclusion of the video with an invitation for questions and comments.

Transcripts

Browse More Related Video

CIRCLE | TRANSFORMING STANDARD FORM TO GENERAL FORM | PROF D

EQUATION OF CIRCLE IN STANDARD FORM | PROF D

CIRCLES || PRE-CALCULUS

GENERAL EQUATION OF THE HYPERBOLA IN STANDARD FORM || PRE-CALCULUS

PART 2: CIRCLES || PRE - CALCULUS

DETERMINING IF AN EQUATION IS A CIRCLE, POINT, OR HAS NO GRAPH | PROF D

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
MathematicsCircle EquationGeneral FormStandard FormCompleting the SquareEducational ContentAlgebra TutorialMath ClassVideo LessonEquation Transformation