EQUATION OF CIRCLE IN STANDARD FORM | PROF D

Prof D
18 Mar 202115:35
EducationalLearning
32 Likes 10 Comments

TLDRThis educational video tutorial guides viewers on how to derive the equation of a circle in standard form. It begins by explaining the concept of a circle as a locus of points equidistant from a center point, defining the radius as this constant distance. The standard equation, \((x-h)^2 + (y-k)^2 = r^2\), is introduced, with 'h' and 'k' representing the center's coordinates and 'r' the radius. The video proceeds with three examples, illustrating how to find the equation given the circle's center and radius. It covers scenarios including a circle centered at the origin with a given radius, a circle with a specified center and radius, and a circle defined by the endpoints of its diameter. The presenter simplifies the equations step-by-step, making the process accessible for learners. The video concludes with an invitation for viewers to ask questions in the comments section.

Takeaways
  • 📚 A circle is defined as the set of points that are at a constant distance (the radius) from a fixed point (the center).
  • 📐 The standard form of a circle's equation is \((x - h)^2 + (y - k)^2 = r^2\), where \((h, k)\) is the center and \(r\) is the radius.
  • 🔍 The equation of a circle is derived from the distance formula, which is \(d = \sqrt{(x_2 - x_1)^2 + (y_2 - y_1)^2}\).
  • 📝 Example 1: A circle with center at (0,0) and radius 5 has the equation \(x^2 + y^2 = 25\).
  • 📝 Example 2: A circle with center at (-4,5) and radius 4 has the equation \((x + 4)^2 + (y - 5)^2 = 16\).
  • 📝 Example 3: A circle with a diameter between points (4,5) and (-2,3) has a center at (1,4) and a radius \(\sqrt{10}\), with the equation \((x - 1)^2 + (y - 4)^2 = 10\).
  • 🧭 To find the center of a circle given its diameter, use the midpoint formula \(\left(\frac{x_1 + x_2}{2}, \frac{y_1 + y_2}{2}\)\).
  • 📏 The radius of a circle can be found using the distance formula applied to the endpoints of its diameter.
  • 🔢 Simplifying the equation \((x - h)^2 + (y - k)^2 = r^2\) involves substituting the values of \(h\), \(k\), and \(r\) and then simplifying the algebraic expression.
  • 📚 The video provides a practical guide on how to derive and simplify the standard form equation of a circle, with step-by-step examples.
  • 👨‍🏫 The presenter, Prof D, encourages viewers to ask questions or seek clarifications in the comment section if needed.
Q & A

  • What is the definition of a circle according to the video?

    -A circle is defined as the path or locus of a point that moves at a constant distance from a fixed point, called the center.

  • What is the term for the constant distance of any point from the center of a circle?

    -The constant distance of any point from the center of a circle is called the radius.

  • What is the standard form of the equation of a circle?

    -The standard form of the equation of a circle is (x - h)² + (y - k)² = r², where (h, k) is the center of the circle and r is the radius.

  • How is the equation of a circle derived?

    -The equation of a circle is derived from the distance formula, where d = √((x₂ - x₁)² + (y₂ - y₁)²), with (h, k) representing (x₁, y₁) and the point on the circle being (x₂, y₂).

  • What are the coordinates of the center and the radius of the circle in example one?

    -In example one, the center of the circle is at (0, 0) and the radius is 5 units.

  • What is the equation of the circle in example one?

    -The equation of the circle in example one is x² + y² = 25.

  • What are the coordinates of the center and the radius of the circle in example two?

    -In example two, the center of the circle is at (-4, 5) and the radius is 4 units.

  • What is the equation of the circle in example two?

    -The equation of the circle in example two is (x + 4)² + (y - 5)² = 16.

  • How are the center coordinates and the radius of a circle determined from the diameter endpoints in example three?

    -In example three, the center coordinates (h, k) are found using the midpoint formula, and the radius is determined by applying the distance formula to the endpoints of the diameter.

  • What is the equation of the circle in example three?

    -The equation of the circle in example three is (x - 1)² + (y - 4)² = 10.

  • Who is the presenter of the video and what is the main topic discussed?

    -The presenter of the video is Prof D, and the main topic discussed is how to find the equation of a circle in standard form.

  • How can viewers ask questions or seek clarifications after watching the video?

    -Viewers can ask questions or seek clarifications by posting them in the comment section below the video.

Outlines
00:00
📚 Introduction to Circle Equations

In the first paragraph, the video introduces the concept of finding the equation of a circle in standard form. It explains that a circle is the path of a point moving at a constant distance from a fixed point, known as the center. The constant distance is called the radius. The standard form equation of a circle with center (h, k) and radius r is given by (x - h)^2 + (y - k)^2 = r^2. The video then proceeds to an example where the center is at (0, 0) and the radius is 5, showing how to derive the equation x^2 + y^2 = 25.

05:00
📐 Example 2: Circle with Center at (-4, 5) and Radius 4

The second paragraph presents a second example of finding a circle's equation. The center of the circle is given as (-4, 5) with a radius of 4. The video demonstrates the process of substituting the values of h, k, and r into the standard equation to derive the equation of the circle as (x + 4)^2 + (y - 5)^2 = 16. It visually describes how the circle would be positioned 4 units to the right and left of the center, and 4 units above and below it.

10:02
📏 Example 3: Circle with Diameter Endpoints at (4, 5) and (-2, 3)

The third paragraph discusses a more complex example where the circle's diameter endpoints are given at (4, 5) and (-2, 3). The video uses the midpoint formula to find the center of the circle, which is calculated as (1, 4). It then applies the distance formula to find the radius, which is the square root of the sum of the squares of the differences in x and y coordinates of the endpoints, resulting in a radius of √10. The final equation of the circle in standard form is derived as (x - 1)^2 + (y - 4)^2 = 10.

15:04
👋 Conclusion and Sign-off

In the concluding paragraph, the video wraps up with a sign-off message. The presenter expresses hope that the viewers found the lesson helpful and invites any questions or clarifications to be posted in the comment section. The video ends with a friendly farewell from the presenter, Prof D, and a musical outro.

Mindmap
Keywords
💡Circle
A circle is defined as the set of all points in a plane that are at a constant distance from a fixed point, known as the center. In the context of the video, the circle represents the geometric shape that the host is explaining how to find the equation for. The script uses the concept of a circle to introduce the standard form equation of a circle, which is essential for solving geometric problems.
💡Standard Form
The standard form of a circle's equation is a mathematical representation that describes the circle's properties in a specific format. In the video, the standard form is given as (x - h)² + (y - k)² = r², where (h, k) is the center of the circle and r is its radius. This form is crucial for the video's theme, as it is the basis for finding the equation of a circle given certain conditions.
💡Center
The center of a circle is the fixed point from which all points on the circle are equidistant. In the video, the center is denoted by the coordinates (h, k) and is used to establish the location of the circle in the coordinate plane. The script explains how to identify the center's coordinates when given the conditions to find the circle's equation.
💡Radius
The radius of a circle is the constant distance from the center to any point on the circumference of the circle. In the video, the radius is symbolized by 'r' and is a key component in the standard form equation of a circle. The script provides examples where the radius is given as part of the conditions to derive the circle's equation.
💡Equation
An equation in mathematics is a statement that asserts the equality of two expressions. In the context of the video, the term 'equation' specifically refers to the mathematical formula used to describe a circle. The script demonstrates how to derive the equation of a circle based on its center and radius.
💡Distance Formula
The distance formula is a mathematical formula used to calculate the distance between two points in a coordinate plane. In the video, the distance formula is mentioned as the basis for deriving the standard form equation of a circle, where 'd' equals the square root of (x₂ - x₁)² + (y₂ - y₁)². The script uses this formula to explain how the radius of a circle can be determined.
💡Example
Examples are used in the video to illustrate how to apply the concepts and formulas discussed. The script provides three examples, each with different conditions, to demonstrate the process of finding the equation of a circle. These examples help viewers understand how to use the standard form equation in various scenarios.
💡Midpoint Formula
The midpoint formula is used to find the middle point between two endpoints on a line segment. In the video, the midpoint formula is applied to determine the center of a circle when the endpoints of the diameter are given. The script explains how to use the formula to calculate the x and y coordinates of the center.
💡Diameter
The diameter of a circle is a line segment that passes through the center and connects two points on the circle. In the video, the term 'diameter' is used in the context of example three, where the endpoints of the diameter are provided to find the center and radius of the circle. The script shows how the diameter can be used to derive the circle's equation.
💡Square Root
The square root operation is used to find a number that, when multiplied by itself, gives a specified number. In the video, the square root is used in the context of the distance formula and the standard form equation of a circle. The script demonstrates how to calculate the radius using the square root and how it is incorporated into the equation of a circle.
💡Coordinate Plane
The coordinate plane is a two-dimensional plane that uses a grid of perpendicular and intersecting lines called axes to define the position of points. In the video, the coordinate plane is the setting where the circle's equation is derived and visualized. The script uses the coordinate plane to explain the geometric relationships and positions of circles.
Highlights

Introduction to the concept of a circle as the path of a point moving at a constant distance from a fixed point, called the center.

Explanation of the constant distance from any point on the circle to the center, known as the radius.

Presentation of the standard form equation of a circle: (x - h)^2 + (y - k)^2 = r^2.

Derivation of the circle's equation from the distance formula.

Example 1: Finding the equation of a circle with center at (0,0) and radius 5.

Step-by-step substitution of values into the standard equation for Example 1.

Simplification of the equation to x^2 + y^2 = 25 for a circle with a 5-unit radius.

Visual representation of the circle's extent 5 units to the right and left, and up and down from the center.

Example 2: Determining the equation for a circle with center at (-4,5) and radius 4.

Identification of h, k, and r values for the circle in Example 2.

Substitution of values into the standard equation and simplification for Example 2.

Final equation of the circle in Example 2: (x + 4)^2 + (y - 5)^2 = 16.

Example 3: Using the diameter's endpoints to find the circle's equation.

Application of the midpoint formula to find the center of the circle in Example 3.

Utilization of the distance formula to calculate the radius for Example 3.

Final equation of the circle in Example 3: (x - 1)^2 + (y - 4)^2 = 10.

Conclusion of the video with a summary of the process and an invitation for questions or clarifications.

Transcripts

Browse More Related Video

CIRCLES || PRE-CALCULUS

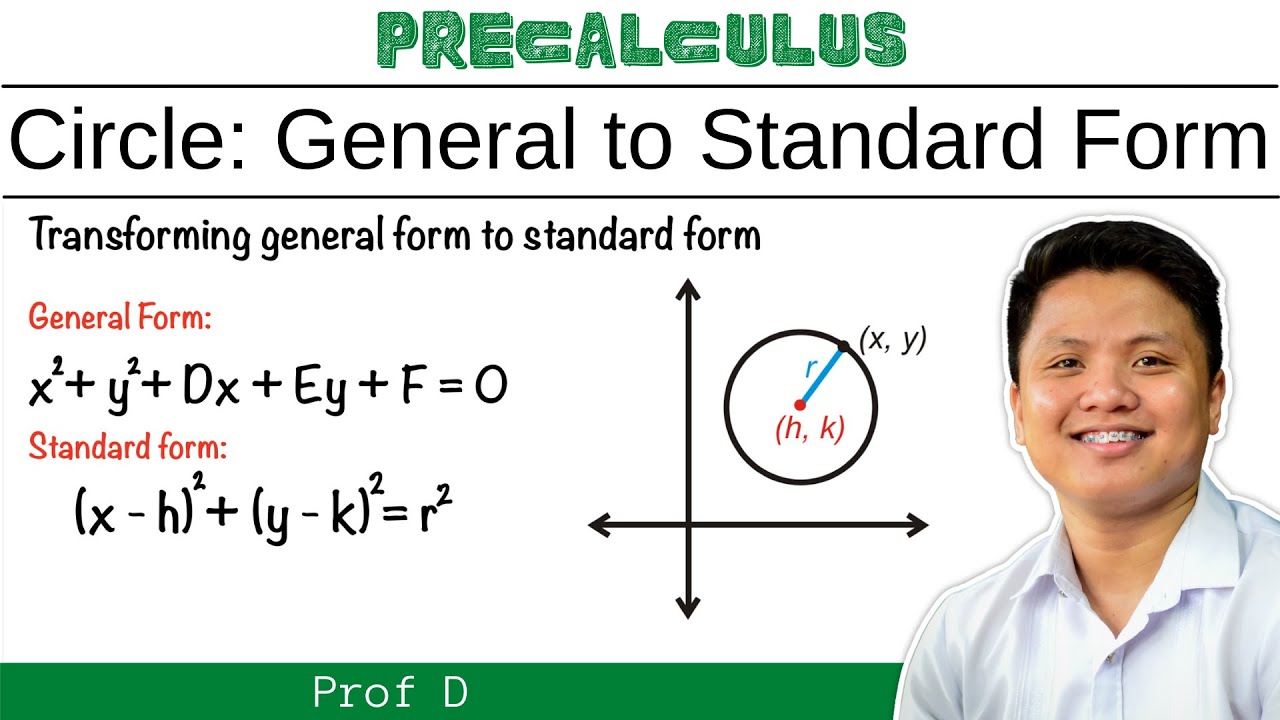
CIRCLE | TRANSFORMING GENERAL FORM TO STANDARD FORM | PROF D

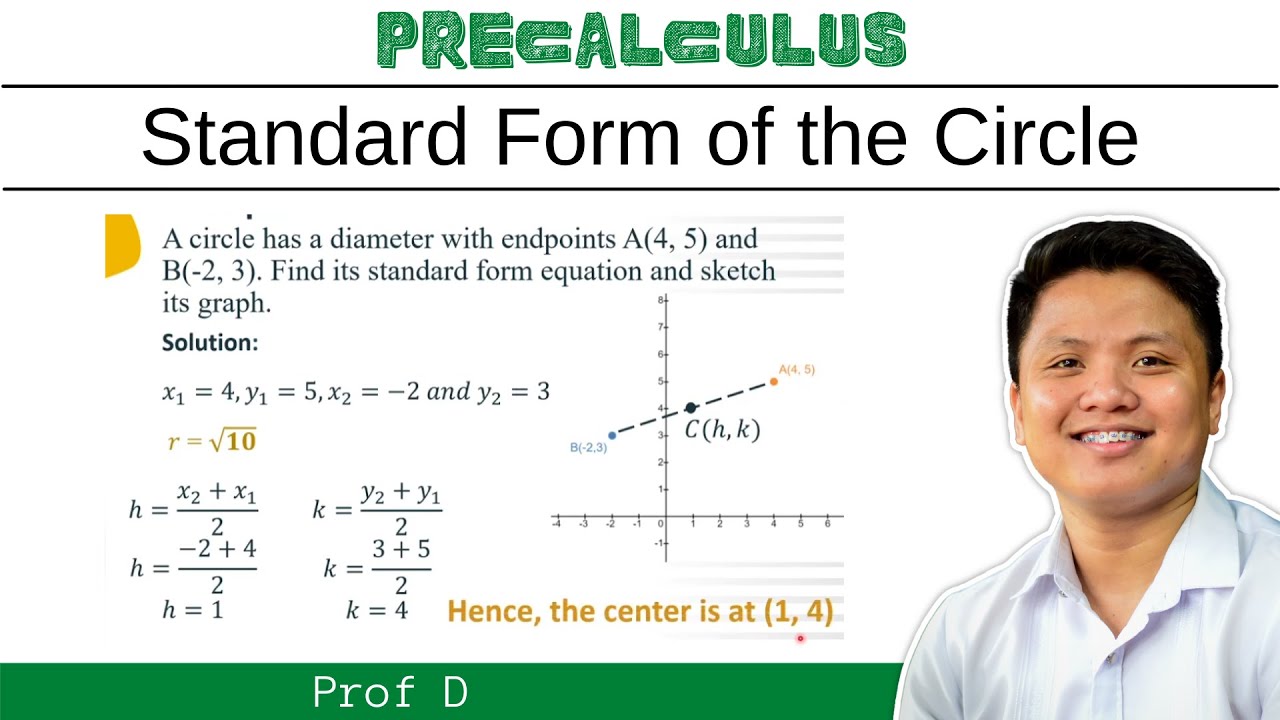
How to find the standard form of the circle | Circle | Conic Section | Pre-Calculus

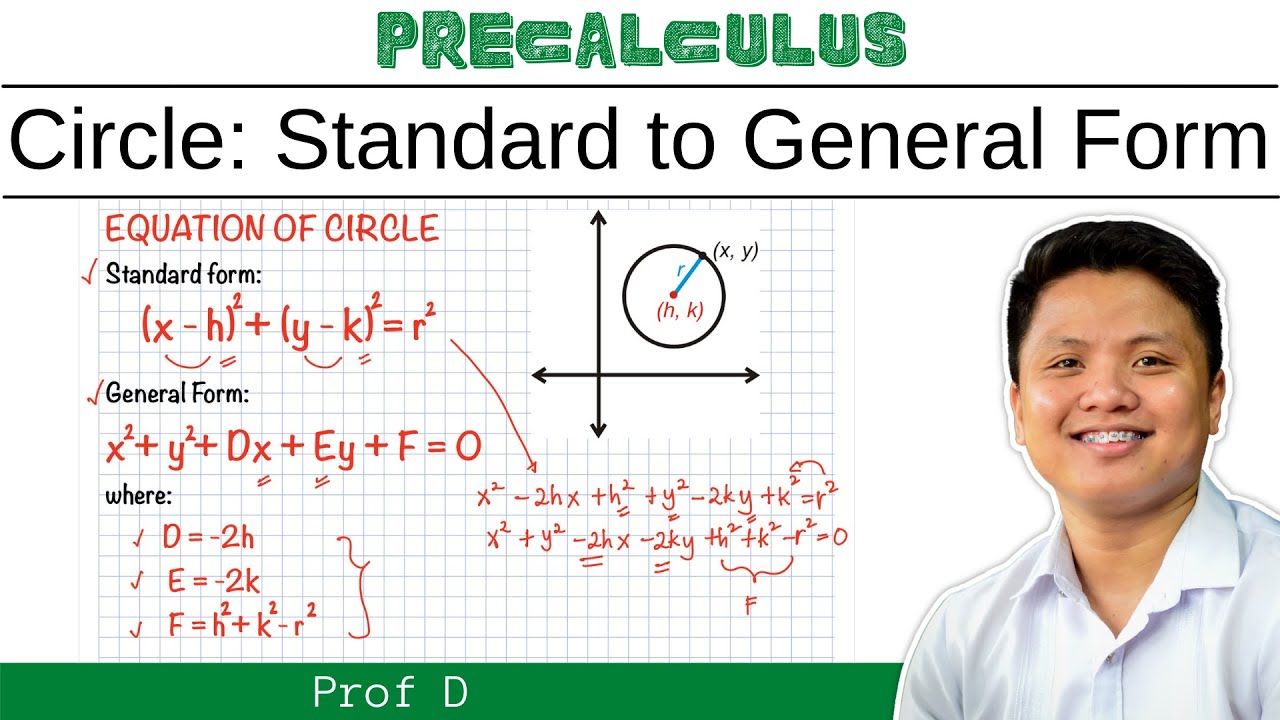
CIRCLE | TRANSFORMING STANDARD FORM TO GENERAL FORM | PROF D

Graphing Conic Sections Part 1: Circles

STANDARD EQUATION OF A CIRCLE IN A GENERAL FORM

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Circle EquationMath TutorialStandard FormGeometryDistance FormulaEducational ContentMathematicsOnline LearningCircle GeometryTutorial Series