CIRCLE | TRANSFORMING STANDARD FORM TO GENERAL FORM | PROF D
TLDRIn this video, the instructor explains how to transform the equation of a circle from standard form to general form. The standard form is \( (x - h)^2 + (y - k)^2 = r^2 \), and the general form is \( x^2 + y^2 + Dx + Ey + F = 0 \). The instructor goes through examples, demonstrating how to use the center and radius of a circle to rewrite the equation in general form. Detailed steps and calculations are provided to ensure a thorough understanding. This video is a helpful guide for students learning about circle equations.
Takeaways
- 📚 The video is a tutorial on converting the equation of a circle from standard form to general form.
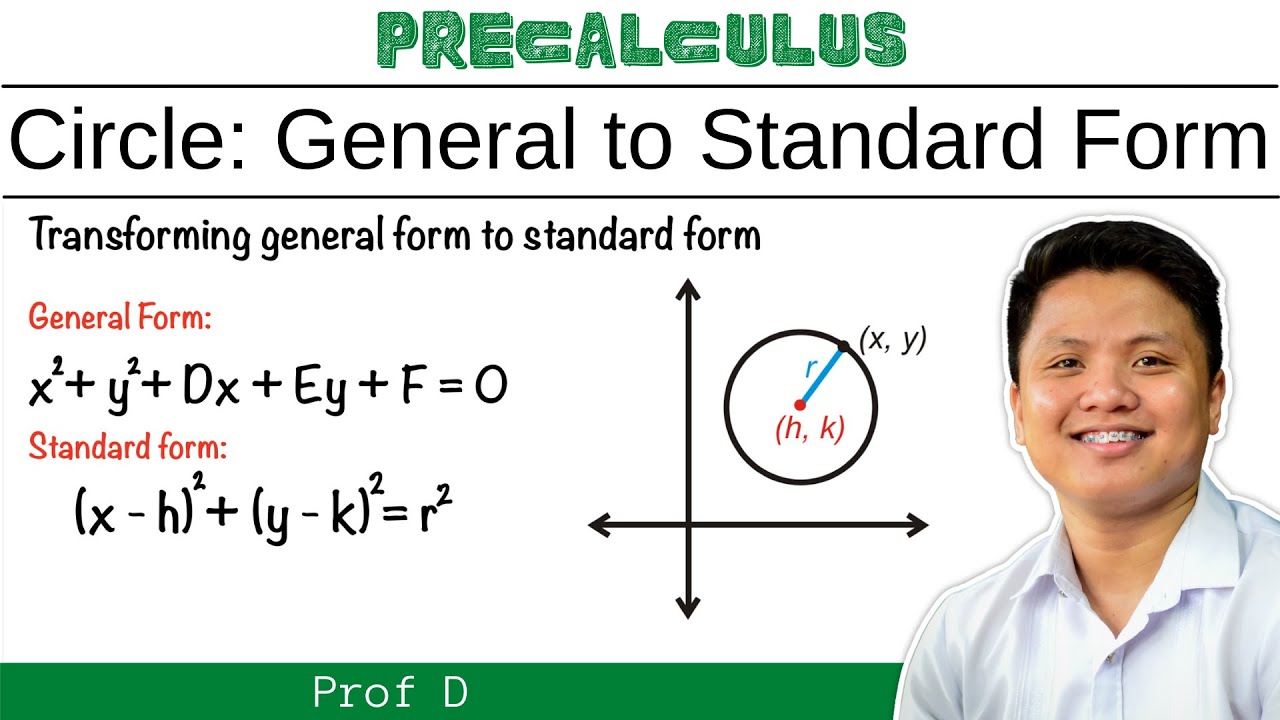
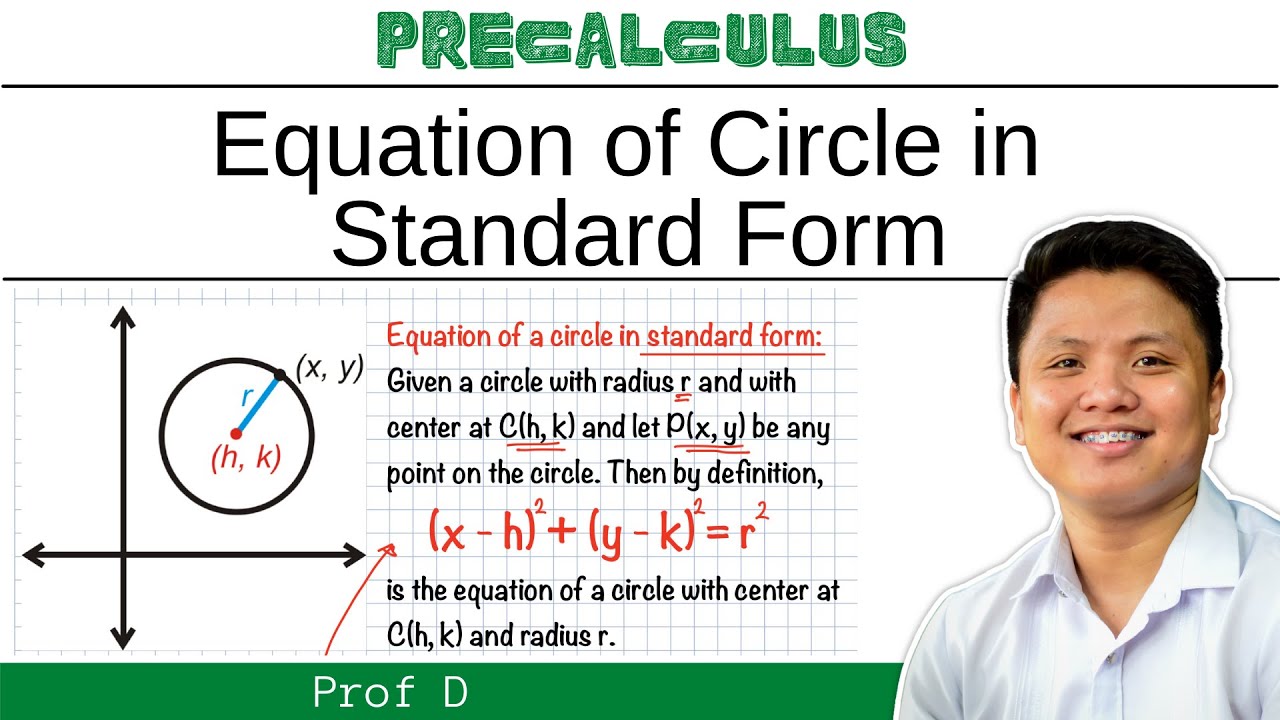
- 🔍 The standard form of a circle's equation is \( (x - h)^2 + (y - k)^2 = r^2 \), where \( (h, k) \) is the center and \( r \) is the radius.
- 📐 The general form of a circle's equation is \( x^2 + y^2 + dx + ey + f = 0 \), with \( d = -2h \), \( e = -2k \), and \( f = h^2 + k^2 - r^2 \).
- 📝 The first example demonstrates converting a circle with center at (-1, -6) and radius 5 into general form.
- 🧩 In the first example, the process involves substituting the values of \( h \), \( k \), and \( r \) into the standard form and then expanding and rearranging to get the general form.
- 🔢 The second example shows the conversion of the equation \( (x + 5)^2 + (y - 3)^2 = 36 \) into general form.
- 📉 The second example involves expanding the binomials, rearranging the terms, and simplifying to achieve the general form.
- 📌 The video explains that \( d \), \( e \), and \( f \) can be determined from the circle's center and radius for the general form equation.
- 🔄 The video provides two methods for converting the equation: one using the standard form and the other by directly calculating \( d \), \( e \), and \( f \).
- 🗣️ The instructor, ProfD, encourages viewers to ask questions or seek clarifications in the comments section.
- 👋 The video concludes with a sign-off from ProfD, indicating the end of the tutorial.
Q & A
What is the standard form of the equation of a circle?
-The standard form of the equation of a circle is (x - h)² + (y - k)² = r², where (h, k) is the center of the circle and r is the radius.
What is the general form of the equation of a circle?
-The general form of the equation of a circle is x² + y² + dx + ey + f = 0, where d = -2h, e = -2k, and f = h² + k² - r².
How do you convert the standard form of a circle's equation to the general form?
-To convert from standard form to general form, you substitute the values of h, k, and r into the general form equation and simplify.
What are the values of h, k, and r in the example with the center at (-1, -6) and radius -5?
-In the example, h = -1, k = -6, and r = 5.
How do you expand the binomials in the process of converting the equation to general form?
-You square the first term, multiply the outer and inner terms and double that product, and square the last term. Then you simplify and combine like terms.
What is the expanded equation of the circle with center (-1, -6) and radius 5 in standard form?
-The expanded equation in standard form is x² + y² + 2x - 12y + 37 = 25.
How do you rearrange the expanded equation to get the general form?
-You rearrange the equation by moving all terms to one side and setting it equal to zero, combining like terms as necessary.
What are the values of d, e, and f for the circle with center (-1, -6) and radius 5?
-For this circle, d = 2, e = 12, and f = 12.
What is the general form of the equation for the circle in the second example with x + 5 and y - 3 terms?
-The general form of the equation for the second example is x² + y² + 10x - 6y - 2 = 0.
What are the values of h, k, and r for the second example where the equation is x + 5 and y - 3 squared equals 36?
-In the second example, h = -5, k = 3, and r = 6.
What is the final step in converting the equation of the second example to general form?
-The final step is to substitute the values of h, k, and r into the general form equation and simplify to get x² + y² + 10x - 6y - 2 = 0.
Outlines
📚 Introduction to Transforming Circle Equations
The video begins with an introduction to converting the equation of a circle from standard form to general form. The standard form is given as \((x - h)^2 + (y - k)^2 = r^2\), where \((h, k)\) is the center and \(r\) is the radius. The general form is presented as \(x^2 + y^2 + dx + ey + f = 0\), with \(d = -2h\), \(e = -2k\), and \(f = h^2 + k^2 - r^2\). The instructor then proceeds to demonstrate the process with example one, which involves writing the equation of a circle with a specific center and radius in general form.
🔍 Detailed Example: Converting Standard to General Form
The instructor provides a detailed walkthrough of converting a circle's equation from standard to general form using the given center at (-1, -6) and radius 5. The process involves substituting the values of \(h\), \(k\), and \(r\) into the standard form equation, simplifying, and then expanding to get the general form. The instructor carefully explains each step, including squaring binomials and rearranging terms, to arrive at the final equation \(x^2 + y^2 + 2x + 12y + 37 = 0\).
📐 Second Example: Finding the General Form of a Given Circle
In the second example, the instructor tackles a different circle equation, \((x + 5)^2 + (y - 3)^2 = 36\), and converts it into the general form. The process includes expanding the binomials, rearranging the terms to match the general form structure, and solving for the constants \(d\), \(e\), and \(f\). The instructor shows that the center of the circle is at (-5, 3) and the radius is 6, leading to the final general form equation \(x^2 + y^2 + 10x - 6y - 2 = 0\).
👋 Conclusion and Invitation for Questions
The video concludes with the instructor summarizing the process and inviting viewers to ask questions or seek clarifications in the comments section. The instructor, profd, thanks the viewers for watching and signs off with a friendly 'bye, you', indicating the end of the educational content.
Mindmap
Keywords
💡Standard Form
💡General Form
💡Center of the Circle
💡Radius
💡Coefficients d, e, and f
💡Transformation
💡Example
💡Square of a Binomial
💡Simplifying the Equation
💡Combining Like Terms
💡Equation Expansion
💡Rearranging the Equation
Highlights
Introduction of transforming the equation of a circle from standard form to general form.
Explanation of the standard form of a circle equation: (x-h)^2 + (y-k)^2 = r^2.
Description of h and k as the center coordinates and r as the radius of the circle.
Presentation of the general form of a circle equation: x^2 + y^2 + dx + ey + f = 0.
Conversion of d, e, and f to -2h, -2k, and h^2 + k^2 - r^2 respectively.
Example 1: Writing the general form equation of a circle with center at (-1, -6) and radius -5.
Methodology for converting from standard to general form using substitution and squaring.
Simplification of the equation to standard form by substituting h, k, and r values.
Expansion and rearrangement of the equation to achieve the general form.
Combining like terms to simplify the equation to its final general form.
Calculation of d, e, and f values using the center and radius of the circle.
Final answer for the first example presented in the general form of the circle equation.
Introduction of a second method for transforming the equation of a circle.
Example 2: Finding the general form of the circle with equation x + 5 and y - 3 squared equals 36.
Step-by-step expansion of binomials to form the general equation of the circle.
Rearrangement of terms to match the general form x^2 + y^2 + dx + ey + f = 0.
Final answer for the second example presented in the general form of the circle equation.
Conclusion of the video with a summary of the methods and practical applications.
Invitation for questions or clarifications in the comment section.
Closing remarks and sign-off from the presenter.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: