PART 2: CIRCLES || PRE - CALCULUS

WOW MATH

28 Jul 202118:56

EducationalLearning

32 Likes 10 Comments

TLDRThis educational video lesson delves into the mathematical concept of circles, specifically focusing on the general form of the equation of a circle. The instructor guides viewers through the process of converting the general equation into the standard form, identifying the center and radius of a circle. Practical examples illustrate how to manipulate equations to find these characteristics, using techniques like completing the square. The video aims to clarify the transition from the general to the standard form and vice versa, providing a comprehensive understanding of circle equations for students.

Takeaways

📚 The video lesson discusses the general form of the equation of a circle and how to convert it into the standard form.
🔍 The general form of a circle's equation is given as \( x^2 + y^2 + dx + ey + f = 0 \).
📐 The standard form of a circle's equation is derived from the general form by completing the square for both \( x \) and \( y \) terms.
🔢 The process involves multiplying and rearranging terms to isolate \( x^2 \) and \( y^2 \), and then completing the square for each.
📈 The completed square form helps in identifying the circle's center and radius by comparing it to the standard form \( (x - h)^2 + (y - k)^2 = r^2 \).
📝 The video provides a step-by-step method to convert the general equation into the standard form by completing the square.
🌐 An example is given to demonstrate how to write the general equation of a circle with a center at (4, -1) and a radius of 7 units.
🔑 The center and radius of a circle can be found by completing the square and comparing the general form to the standard form.
📉 The video also explains how to convert a standard form equation back to the general form, using the method of completing the square.
📚 Additional examples are provided to illustrate the process of finding the center and radius from a given general form equation.
👨‍🏫 The tutorial concludes with a reminder to like, subscribe, and hit the bell button for more educational content.

Q & A

What is the general form of the equation of a circle?
-The general form of the equation of a circle is x^2 + y^2 + dx + ey + f = 0.
How can you derive the standard form of the equation of a circle from the general form?
-You can derive the standard form by completing the square for both x and y terms in the general equation, which results in (x - h)^2 + (y - k)^2 = r^2, where (h, k) is the center of the circle and r is the radius.
What are the values of d and e in terms of the center coordinates (h, k) of the circle?
-In the standard form of the equation, d is equal to -2h and e is equal to -2k.
What is the value of f in the general equation of a circle in terms of the center coordinates and radius?
-The value of f is h^2 + k^2 - r^2, where h and k are the coordinates of the center and r is the radius of the circle.
How do you find the center and radius of a circle given its general equation?
-You first rearrange the general equation into the standard form by completing the square for x and y. The center coordinates (h, k) can be found from the terms involving h and k, and the radius r can be determined from the constant term after setting the equation equal to r^2.
What is the standard form of the equation of a circle with center (4, -1) and radius 7 units?
-The standard form of the equation is (x - 4)^2 + (y + 1)^2 = 7^2, which simplifies to (x - 4)^2 + (y + 1)^2 = 49.
Can you provide an example of converting the standard form of a circle's equation to its general form?
-Yes, for the standard form equation x^2 + y^2 + 6x - 7 = 0, you would rearrange it to complete the square for x, resulting in the general form x^2 + 6x + y^2 - 7 = 0.
What is the process of converting the general form of a circle's equation to the standard form?
-The process involves isolating the x and y terms, completing the square for both x and y, and then rearranging the equation to isolate the constant term on one side, resulting in the standard form (x - h)^2 + (y - k)^2 = r^2.
How do you find the center and radius of the circle given by the general form x^2 + y^2 - 6x + 10y + 18 = 0?
-You would complete the square for x and y, resulting in (x - 3)^2 + (y - 5)^2 = 16. The center is at (3, 5) and the radius is the square root of 16, which is 4.
What is the significance of completing the square in finding the standard form of a circle's equation?
-Completing the square allows you to rearrange the general form of the equation into the standard form, which clearly shows the circle's center and radius, making it easier to visualize and understand the circle's properties.

Outlines

00:00

📚 Introduction to the Equation of a Circle

This introductory paragraph sets the stage for a lesson on the general form of the equation of a circle. The speaker invites viewers to like and subscribe to the channel before diving into the mathematical content. The general equation of a circle is presented as x squared plus y squared plus dx plus ey plus f equals zero. The paragraph emphasizes the importance of understanding this general form as a foundation for further exploration of circle equations.

05:01

🔍 Deriving the General Form from the Standard Form

The speaker explains the process of deriving the general form of the equation of a circle from its standard form. This involves expanding binomials and combining like terms. The process is illustrated with an example where the standard form equation is manipulated to reach the general form. The paragraph concludes with the identification of coefficients d, e, and f in terms of the circle's center (h, k) and radius r.

10:02

📘 Writing the General Equation with Given Parameters

This paragraph focuses on applying the general form of the circle's equation with specific parameters. The center of the circle and the radius are given, and the speaker demonstrates how to substitute these values into the general equation to obtain the standard form. The example provided shows the step-by-step process of completing the square to find the equation of a circle with a center at (4, -1) and a radius of 7 units.

15:03

🔄 Converting from General to Standard Form Using Completing the Square

The speaker discusses the method of converting a circle's equation from the general form to the standard form by completing the square. This involves isolating x and y terms, creating perfect square trinomials, and adjusting the constant term to maintain equality. The paragraph provides a detailed example, demonstrating the mathematical steps required to transform the equation and achieve the standard form.

📐 Finding the Center and Radius from the General Form

This paragraph delves into the process of determining the center and radius of a circle when given its equation in the general form. The speaker explains how to rearrange and complete the square for both x and y terms to reveal the circle's center coordinates and radius. Two examples are given, showcasing different scenarios where the general form of the equation is manipulated to extract the circle's properties.

🎓 Summary of the Process and Final Remarks

In the concluding paragraph, the speaker summarizes the process of working with the general form of the equation of a circle. The focus is on the steps required to convert the general form to the standard form and to extract the circle's center and radius. The speaker encourages viewers to take notes and to apply the concepts learned in the lesson. The video ends with a reminder to like, subscribe, and hit the bell button for more educational content.

Mindmap

Keywords

💡Circle

A circle is a geometric shape consisting of all points equidistant from a given center point. In the video, circles are the primary focus, with the discussion revolving around the mathematical representation and properties of circles, such as their equations and radii.

💡Equation of a Circle

The equation of a circle is a mathematical formula used to describe the set of all points that form a circle. The general form discussed in the video is 'x squared plus y squared plus dx plus ey plus f equals zero', which is essential for identifying the circle's center and radius.

💡Standard Form

Standard form of a circle's equation is '(x - h) squared plus (y - k) squared equals r squared', where (h, k) is the center of the circle and r is its radius. The video explains how to convert the general form of the equation into this more recognizable standard form.

💡Center of a Circle

The center of a circle is the point from which all points on the circle are equidistant. The video script explains how to find the center by rearranging the general form of the circle's equation into the standard form, revealing the coordinates (h, k).

💡Radius

The radius of a circle is the distance from the center to any point on the circumference. In the video, the radius is denoted by 'r' and is a key component in writing the standard form equation of a circle, as it determines the size of the circle.

💡Completing the Square

Completing the square is a mathematical technique used to solve quadratic equations and rewrite them in a form that makes the radius and center of a circle evident. The video demonstrates this process by manipulating the terms of the circle's equation.

💡General Form

The general form of the equation of a circle, as mentioned in the script, is 'x squared plus y squared plus dx plus ey plus f equals zero'. The video explains how to transform this into the standard form to make it easier to identify the circle's properties.

💡Binomial

A binomial is an algebraic expression with two terms, such as 'x squared' or 'y squared'. In the context of the video, binomials are used in the process of completing the square to rewrite the circle's equation into the standard form.

💡Quadratic Equation

A quadratic equation is a polynomial equation of degree two, typically in the form 'ax squared plus bx plus c equals zero'. The video script discusses how to solve for the center and radius of a circle by manipulating a quadratic equation representing the circle.

💡Range of a Circle

The range of a circle, in the context of the video, refers to the possible values that the radius can take. The video explains how to determine the radius from the standard form equation, which helps in understanding the size and extent of the circle.

Highlights

The general form of the equation of a circle is x squared plus y squared plus dx plus ey plus f equals zero.

The standard form of the equation of a circle can be derived from the general form.

To convert from standard to general form, expand and rearrange the terms.

The center of a circle can be found by completing the square for both x and y terms.

The radius of a circle is the square root of the constant term in the standard form equation.

The general equation of a circle with center (h, k) and radius r is x squared plus y squared plus dx plus ey plus f equals zero, where d equals -2h, e equals -2k, and f equals h squared plus k squared minus r squared.

An example is provided to write the general equation of a circle with a specific center and radius.

Completing the square involves dividing the coefficient of the x or y term by two and squaring it.

The process of converting from general to standard form is demonstrated with an example equation.

The center and radius of a circle given in general form can be determined by completing the square for both x and y terms.

An example demonstrates how to find the center and radius of a circle from its general form equation.

The radius of a circle is found by taking the square root of the constant term after completing the square.

The video provides a step-by-step guide on converting between general and standard form equations of a circle.

The importance of arranging terms and combining like terms is emphasized in the process of converting equation forms.

The video concludes with a reminder to like, subscribe, and hit the bell button for more video tutorials.

Practical applications of understanding circle equations are hinted at for further learning.

The video serves as a comprehensive guide for learners to understand the equations of circles in both general and standard forms.

Transcripts

Browse More Related Video

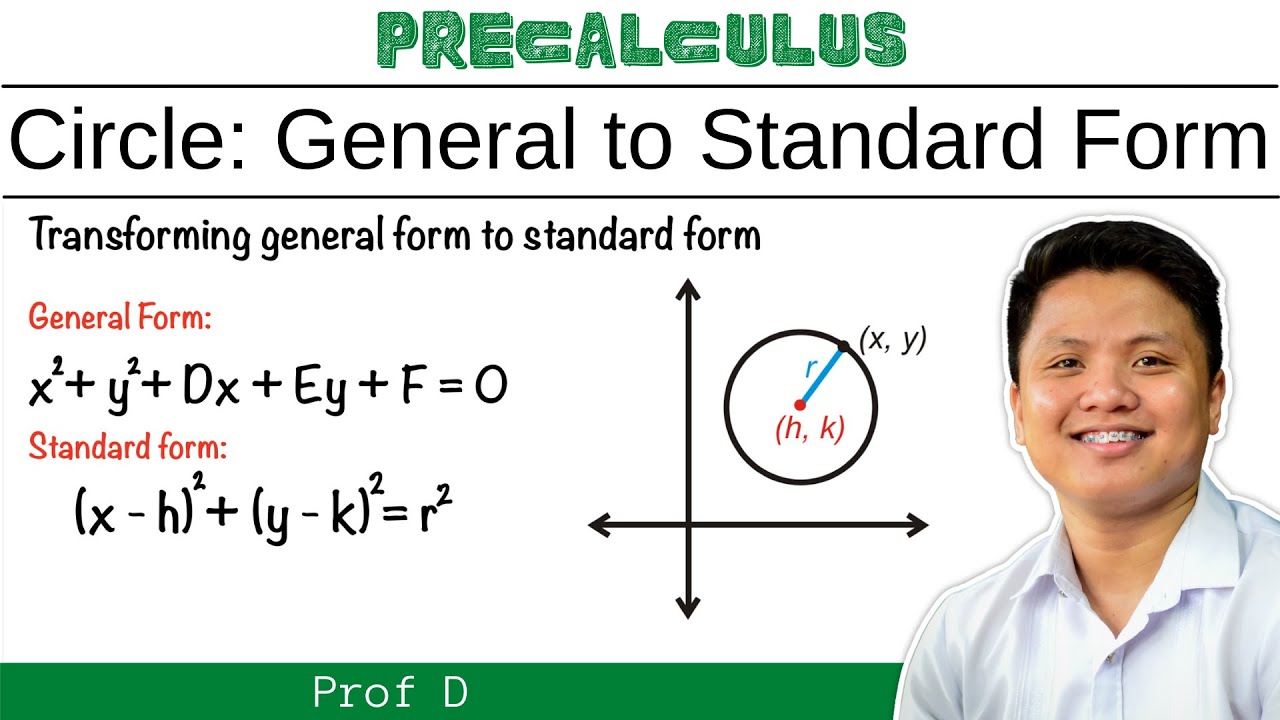
CIRCLE | TRANSFORMING GENERAL FORM TO STANDARD FORM | PROF D

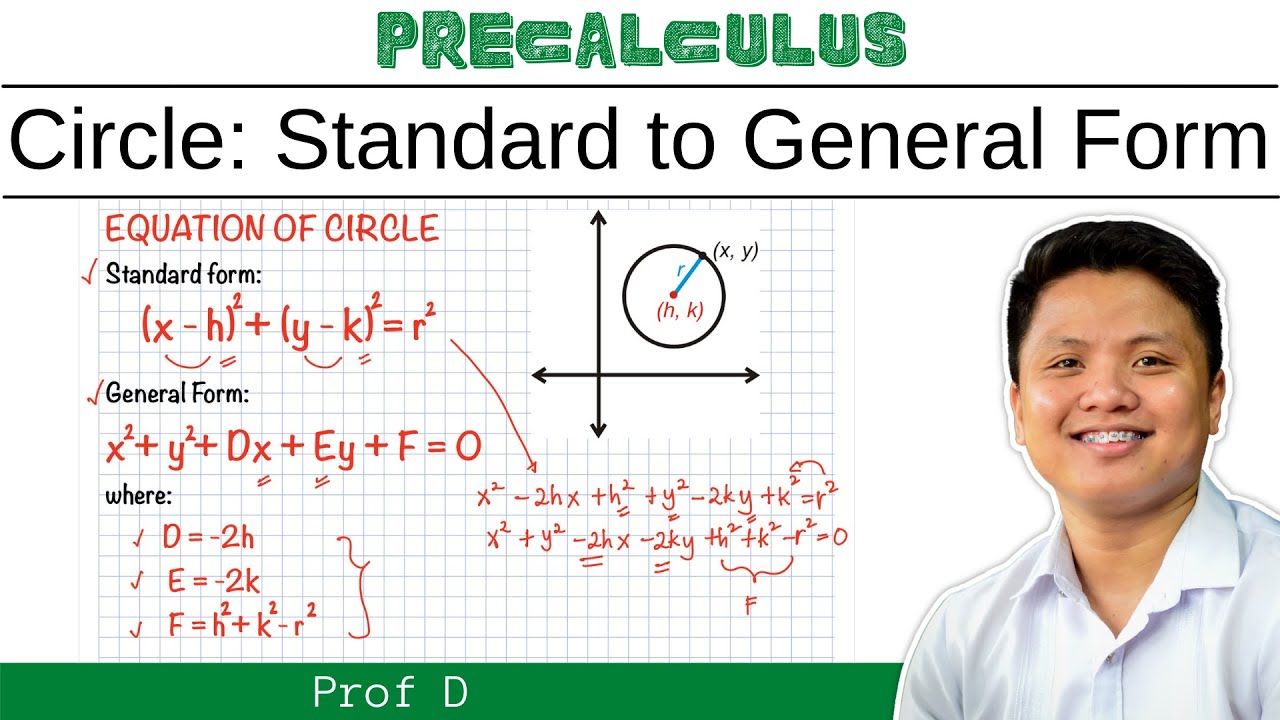
CIRCLE | TRANSFORMING STANDARD FORM TO GENERAL FORM | PROF D

STANDARD EQUATION OF A CIRCLE IN A GENERAL FORM

STANDARD EQUATION OF PARABOLA TO GENERAL FORM

CIRCLES || PRE-CALCULUS

GENERAL EQUATION OF THE HYPERBOLA IN STANDARD FORM || PRE-CALCULUS