PART 3 : CIRCLES || PRE - CALCULUS
TLDRThis educational video lesson delves into the fundamentals of circles, focusing on graph sketching, problem-solving, and applications. It begins with a step-by-step guide to graphing circles given their centers and radii, followed by solving equations to find the standard form of a circle. The video also covers concepts like slope of a line, distance formula, and midpoint, applying these to find the equation of a circle with a given diameter. It concludes with a real-world problem involving a seismological station and an earthquake's epicenter, demonstrating how to use a circle's equation to determine possible locations. The tutorial is designed to help viewers understand and apply mathematical concepts related to circles.
Takeaways
- 📚 The video lesson is focused on sketching the graph of a circle, problem-solving, and application involving circles.
- 📐 Example one demonstrates how to sketch a circle with a radius of 4 and a center at coordinates (0,0).
- ⭕ The equation of a circle with center (0,0) and radius 4 is \( x^2 + y^2 = 16 \).
- 📈 The second example involves sketching a circle given by the equation \( (x - 2)^2 + (y + 1)^2 = 36 \), with a center at (2, -1) and a radius of 6.
- 🔍 The third example shows completing the square to rewrite the equation \( x^2 + y^2 + 10x - 4y - 8 = 12 \) into the standard form of a circle's equation.
- 📏 Completing the square for the third example results in the circle's equation \( (x + 5)^2 + (y - 2)^2 = 49 \), with a center at (-5, 2) and a radius of 7.
- 🧭 The video discusses concepts such as slope of a line, distance between two points, and midpoint formula, which are essential for problem-solving with circles.
- 🔍 Problem-solving involves finding the standard equation of a circle given the endpoints of its diameter.
- 🌐 An example problem involves finding the possible location of an earthquake's epicenter using a circle with a known radius and center.
- 📍 The final example calculates possible coordinates for the epicenter of an earthquake, considering it to be one kilometer away from the shore, resulting in coordinates such as (3.31, 1) and (-3.31, 1).
- 👨🏫 The video concludes with an invitation to like, subscribe, and hit the bell for more educational content on the channel.
Q & A
What is the main topic of the video lesson?
-The main topic of the video lesson is about circles, focusing on sketching the graph of a circle, problem-solving, and applications.
What are the steps to sketch the graph of a circle with center at (0,0) and radius 4?
-To sketch the graph, first mark the center at (0,0). Then, measure 4 units outward from the center in all directions to mark the circumference of the circle. Draw a circle through these points.
What is the equation of a circle with center at (0,0) and radius 4?
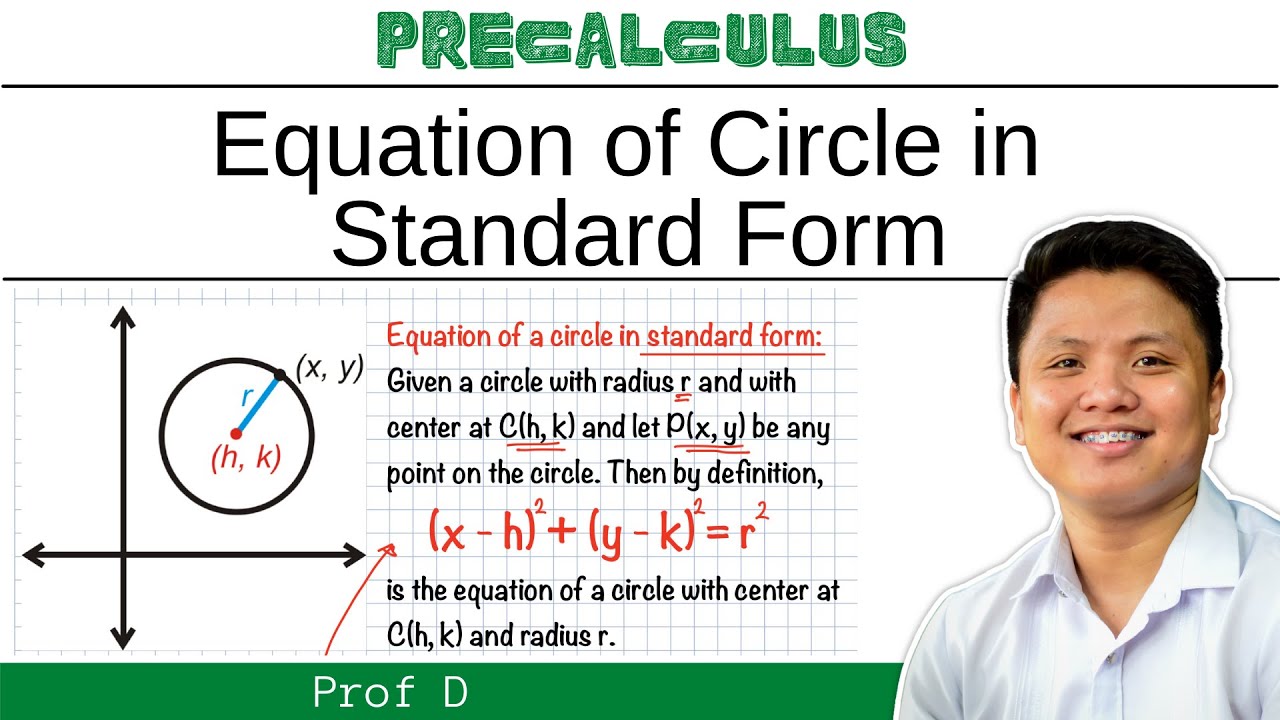
-The equation of the circle is \( x^2 + y^2 = 16 \), derived from the general equation \( (x - h)^2 + (y - k)^2 = r^2 \) where \( h = 0 \), \( k = 0 \), and \( r = 4 \).
How do you find the center and radius of the circle from the equation \( (x - 2)^2 + (y + 1)^2 = 36 \)?
-The center of the circle is at (2, -1), and the radius is the square root of 36, which is 6.
What is the process of completing the square for the equation \( x^2 + y^2 + 10x - 4y - 8 = 12 \)?
-Group the x terms and y terms, then complete the square by adding and subtracting the necessary values (in this case, 25 for x and 4 for y) to form perfect square trinomials. This results in the equation \( (x + 5)^2 + (y - 2)^2 = 49 \).
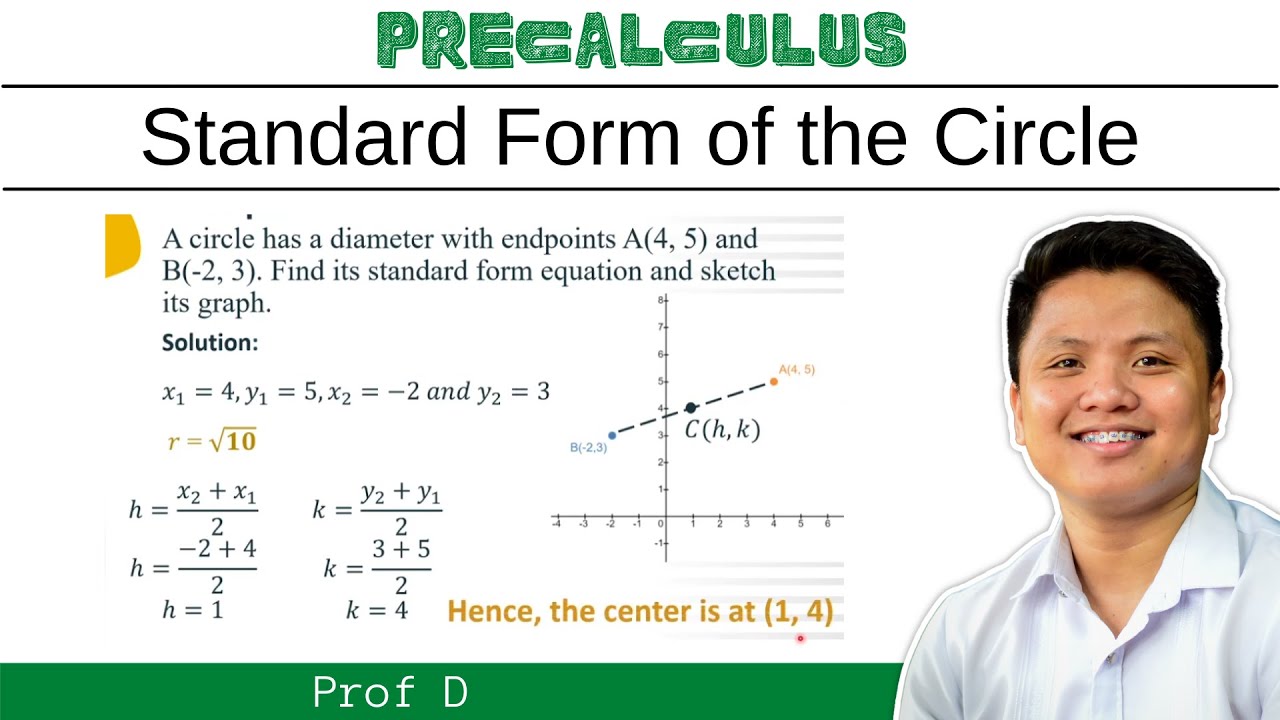
How do you determine the standard equation of a circle given the endpoints of its diameter?
-Use the midpoint formula to find the center of the circle, which is the midpoint of the diameter. Then use the distance formula to find the radius, which is half the length of the diameter.
What is the slope of a line given two distinct points (x1, y1) and (x2, y2)?
-The slope \( m \) of the line is calculated using the formula \( m = \frac{y2 - y1}{x2 - x1} \), provided \( x1 \neq x2 \).
What is the distance between two points (x1, y1) and (x2, y2)?
-The distance is found using the distance formula \( \sqrt{(x2 - x1)^2 + (y2 - y1)^2} \).
How do you find the midpoint of a segment with endpoints (x1, y1) and (x2, y2)?
-The midpoint \( (x, y) \) is found using the midpoint formula \( x = \frac{x2 + x1}{2} \) and \( y = \frac{y2 + y1}{2} \).
Given a seismological station located at (0, -4) and an earthquake epicenter 6 km away, how do you find the equation of the curve containing the possible locations of the epicenter?
-The curve is a circle with the station as the center and a radius of 6 km. The equation is \( x^2 + (y + 4)^2 = 36 \).
If the epicenter is also 1 km away from the shore, what are the possible coordinates of the epicenter?
-Solving the equation \( x^2 + (y + 1)^2 = 36 \) for x when y is 1 gives possible coordinates of the epicenter as (±3.31, 1) and (±5.20, -1), rounded to two decimal places.
Outlines
📐 Sketching the Graph of a Circle
This paragraph introduces the topic of circles and focuses on sketching the graph of a circle, problem-solving, and applications. It begins with an example of sketching a circle with a given radius and center. The equation of the circle is derived, and the process is demonstrated through visual steps. The video also covers sketching more complex circle equations, such as one with a shifted center.
🔍 Completing the Square and Circle Equations
The second paragraph delves into the process of completing the square for circle equations. It provides a step-by-step guide on how to transform a general equation into the standard form of a circle's equation. The example given walks through the algebraic manipulation required to find the center and radius of the circle from the equation, and then how to sketch the circle based on these parameters.
🧮 Finding the Center and Radius of a Circle
This section discusses how to find the center and radius of a circle when given the endpoints of a diameter. It uses the midpoint formula to determine the circle's center and the distance formula to calculate the radius. The process is illustrated with a specific example, showing how to substitute values into the formulas and solve for the circle's properties.
🌐 Standard Form of a Circle and Earthquake Epicenters
The fourth paragraph presents the standard form of a circle's equation and applies it to a real-world problem involving the location of an earthquake's epicenter. It explains how to use the given information about the distance from a seismic station to the shore and the epicenter to formulate the circle's equation. The video then solves for the possible coordinates of the epicenter under additional constraints.
📍 Determining Possible Coordinates of an Epicenter
The final paragraph continues the application of circle equations to the problem of locating an earthquake's epicenter. It provides a method to find the possible coordinates of the epicenter based on its distance from the shore and the seismic station. The calculations are shown in detail, with the final answer rounded to two decimal places, offering a clear solution to the problem.
Mindmap
Keywords
💡Circle
💡Graph
💡Equation
💡Radius
💡Center
💡Completing the Square
💡Slope
💡Distance Formula
💡Midpoint
💡Problem Solving
Highlights
Introduction to the video lesson focusing on sketching the graph of a circle, problem-solving, and application.
Explanation of how to sketch the graph of a circle with a given radius and center at (0,0).
Derivation of the equation of a circle using the formula x^2 + y^2 = r^2 where r is the radius.
Demonstration of sketching a circle with the equation (x-2)^2 + (y+1)^2 = 36.
Process of completing the square to rewrite the equation of a circle in standard form.
Example of sketching a circle with the equation x^2 + y^2 + 10x - 4y - 8 = 12.
Discussion on the slope of a line given two distinct points and the formula for calculating it.
Introduction of the distance formula between two points and its application.
Explanation of the distance from any point to the center of a circle using the distance formula.
Use of midpoint formula to find the center of a circle given the endpoints of a diameter.
Problem-solving example to find the standard equation of a circle with given diameter endpoints.
Application of the distance formula to determine the radius of a circle.
Derivation of the standard form equation of a circle given its center and radius.
Problem involving a seismological station and an earthquake epicenter to find the equation of a curve.
Solution to find the possible coordinates of the epicenter given additional distance information.
Final problem-solving example with calculations and rounding off to two decimal places.
Conclusion of the video with an invitation to like, subscribe, and hit the bell for more tutorials.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: