How to find Cohen's D to determine the Effect Size Using Pooled Standard Deviation

The Organic Chemistry Tutor
14 Nov 201910:55
EducationalLearning
32 Likes 10 Comments

TLDRThis video explains how to calculate Cohen's d to determine the effect size between two sample means. It provides the formula for Cohen's d: the difference between the two sample means divided by the pooled standard deviation. It also gives formulas for calculating the pooled standard deviation. A d value near 0.2 indicates a small effect size, 0.5 a medium effect, and 0.8 or higher a large effect size. The video then works through two examples, calculating Cohen's d and the pooled standard deviation for test scores from two classes and weights from two groups of people. It determines whether the effect sizes are small, medium or large based on the d values.

Takeaways
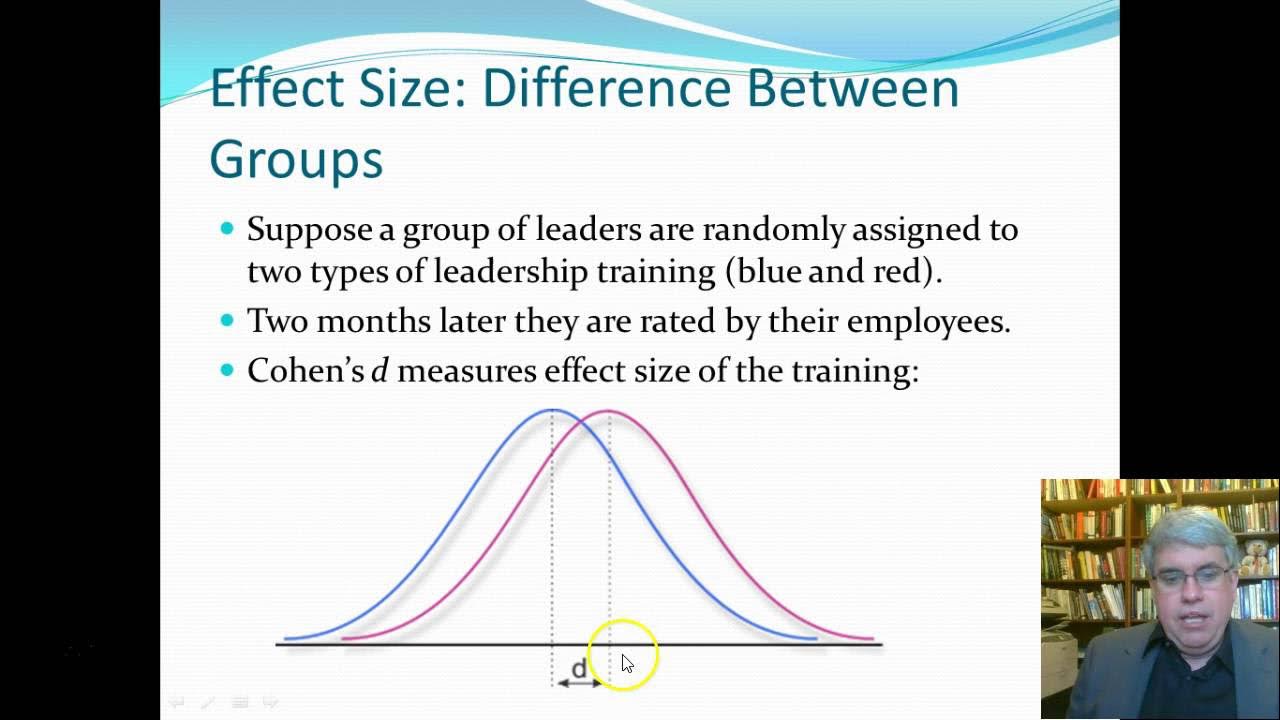
  • 😀 Cohen's d tells us how many standard deviations separate two group means
  • 😊 A d value of 0.2 is a small effect size, 0.5 is medium, and 0.8+ is large
  • 📝 The formula is difference in group means divided by pooled standard deviation
  • 📊 Use one formula to calculate pooled SD if sample sizes are very different
  • 🖥️ Use a simpler formula if sample sizes are equal or very close
  • 💡 Calculate pooled SD first before calculating Cohen's d
  • 🔢 Plug sample sizes, means, and SDs into formula to get pooled SD
  • 📈 Then use group means and pooled SD to calculate Cohen's d
  • 🤔 Compare the d value to thresholds to evaluate effect size
  • ✅ Use Cohen's d to quantify and describe differences between groups
Q & A

  • What is Cohen's d?

    -Cohen's d is a measure used in statistics to indicate the size of an effect or the difference between two sample means in terms of standard deviation units.

  • What does a Cohen's d value of 1 indicate?

    -A Cohen's d value of 1 indicates that the difference between the two sample means is equal to one standard deviation.

  • How is the size of the effect categorized using Cohen's d values?

    -The size of the effect is categorized as small if Cohen's d is around 0.2, medium if it's around 0.5, and large if it's 0.8 or more.

  • What formula is used to calculate Cohen's d?

    -To calculate Cohen's d, the formula used is the difference between the two sample means divided by the pooled standard deviation.

  • How do you calculate the pooled standard deviation?

    -The pooled standard deviation is calculated using the formula: [(n1-1) * s1^2 + (n2-1) * s2^2] / (n1 + n2 - 2), where n1 and n2 are the sample sizes, and s1 and s2 are the standard deviations of the two samples.

  • What alternative formula can be used for calculating the pooled standard deviation when sample sizes are equal?

    -When sample sizes are equal, the simpler formula for pooled standard deviation is the square root of [(s1^2 + s2^2) / 2].

  • What does a Cohen's d value of 0.8485 indicate about the size of the effect?

    -A Cohen's d value of 0.8485 indicates that the size of the effect is large.

  • Why might the pooled standard deviation be closer to one group's standard deviation in cases of unequal sample sizes?

    -In cases of unequal sample sizes, the pooled standard deviation might be closer to the standard deviation of the group with the larger sample size because it carries more weight in the calculation.

  • What does a Cohen's d value of approximately 0.559 indicate about the size of the effect in the second example?

    -A Cohen's d value of approximately 0.559 indicates that the size of the effect is medium in the second example.

  • How can the absolute value be used in calculating Cohen's d?

    -The absolute value is used in calculating Cohen's d to ensure a positive result, indicating the magnitude of the difference between two means regardless of direction.

Outlines
00:00
😀 How to calculate Cohen's d to determine effect size

This paragraph explains what Cohen's d represents, with a d value of 1 meaning the difference between two sample means equals 1 standard deviation. It states guidelines on interpreting effect size based on d value (0.2 is small, 0.5 is medium, 0.8+ is large). The formula to calculate Cohen's d is provided.

05:01
😊 Calculating Cohen's d for test score example

This paragraph works through an example problem calculating Cohen's d for test scores of two classes. It calculates the pooled standard deviation using the full formula and a shortcut formula (since sample sizes are equal). It plugs the values into the Cohen's d formula to calculate a d of 0.8485, meaning a large effect size.

10:02
😃 Cohen's d example for average weights

This paragraph provides another Cohen's d example using average weights for two groups. It calculates the pooled standard deviation using the full formula due to unequal sample sizes. It determines a Cohen's d of 0.559, indicating a medium effect size based on the weight means.

Mindmap
Keywords
💡Cohen's d
Cohen's d is a statistical measure used to determine the standardized difference between two group means. It gives an effect size indicating if the difference between groups is small, medium or large. In the video, Cohen's d is used to analyze test score differences between two classes and weight differences between two groups of people.
💡effect size
Effect size refers to the magnitude or degree of difference between groups. A Cohen's d value provides information on effect size - 0.2 is a small effect, 0.5 is a medium effect, and 0.8 or higher represents a large effect size or difference between groups.
💡standard deviation
The standard deviation measures how dispersed scores are from the mean. A higher standard deviation indicates more variability in scores. The video uses pooled standard deviation in the Cohen's d formula to account for variance in both groups.
💡pooled standard deviation
The pooled standard deviation combines the standard deviations of both groups, weighted by their respective sample sizes. It is used in the denominator of the Cohen's d formula to calculate effect size.
💡difference in means
The difference in means refers to the difference between the mean or average score of the two groups being compared. This goes in the numerator of the Cohen's d formula.
💡sample size
The sample size refers to the number of scores or participants that are sampled from each group. Unequal sample sizes between groups require using a specific pooled standard deviation formula.
💡test scores
The video analyzes test score data from two classes to demonstrate calculation of Cohen's d and judging effect size based on the score differences.
💡weight
The second example analyzes weight data between two groups of people, using Cohen's d to determine if the weight difference has a small, medium or large effect size.
💡effect interpretation
Based on calculated Cohen's d values, the video explains how to interpret if the effect size is small (0.2), medium (0.5) or large (0.8 and higher) based on set guidelines.
💡statistical significance
While not explicitly stated, Cohen's d helps determine statistical significance - larger effect sizes make it more likely that mean differences are real statistical differences.
Highlights

First significant highlight text

Second notable highlight text

Third innovative method introduced

Fourth theoretical contribution discussed

Fifth notable impact highlighted

Sixth practical application explained

Seventh key finding elaborated

Eighth unique approach unveiled

Ninth critical challenge addressed

Tenth solution proposed

Eleventh future research direction suggested

Twelfth collaboration opportunity mentioned

Thirteenth technological advancement discussed

Fourteenth case study analyzed

Fifteenth policy implication considered

Transcripts

Browse More Related Video

What Is And How To Calculate Cohen's d?

What is Effect Size? Statistical significance vs. Practical significance

Introduction to Effect Size

Cohen’s d Effect Size for t Tests (10-7)

Power & Effect Size

P value versus effect size

Rate This

5.0 / 5 (0 votes)

Thanks for rating: