PROBLEM SOLVING INVOLVING CIRCLES

WOW MATH
6 Oct 202207:00
EducationalLearning
32 Likes 10 Comments

TLDRThe video script presents a series of mathematical problems involving geometry. It begins with finding the equation of a circle given its center and a midpoint on the circle, leading to the use of the circle's standard equation. Next, it addresses the height of a semicircular tunnel at the edge of a 10-foot wide street, using the circle's equation to determine the height at a specific point. Lastly, the script explores the horizontal distance from the center of a Ferris wheel when a car is at a certain altitude, using the Pythagorean theorem to solve. The presenter solves the first two problems but decides to tackle the last one independently, showcasing a step-by-step approach to problem-solving in geometry.

Takeaways
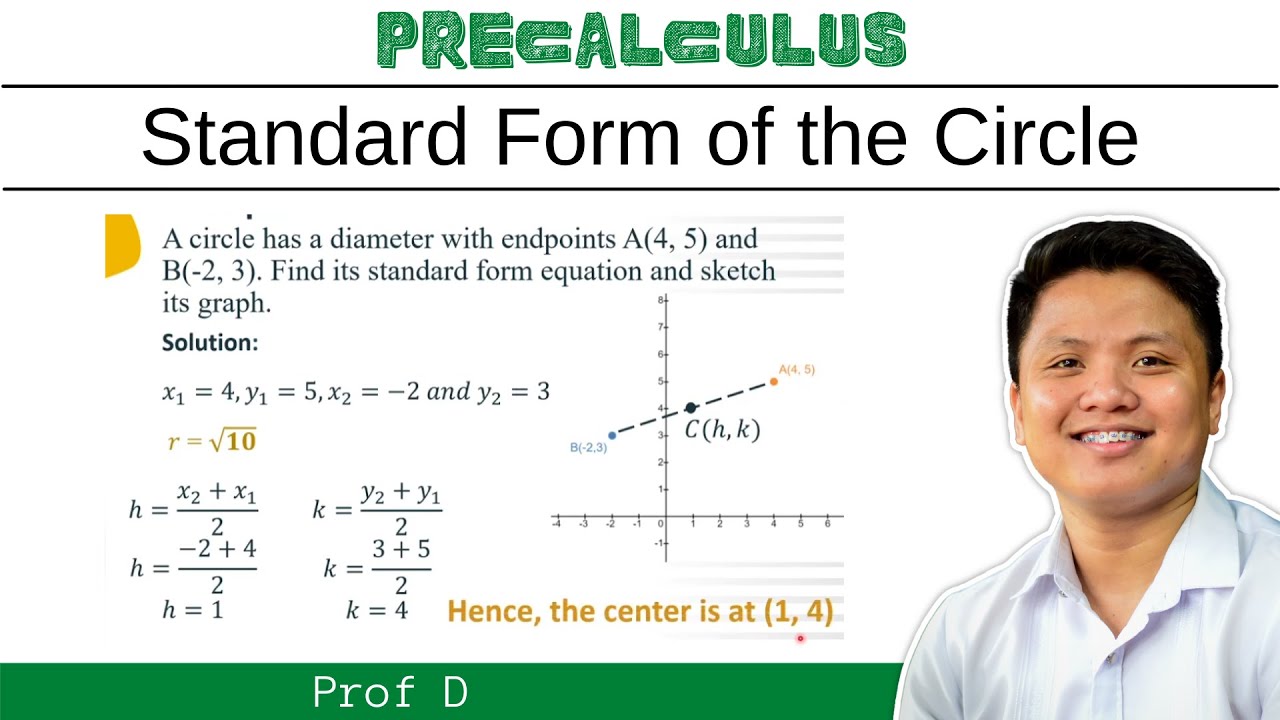
  • 📚 The first problem involves finding the equation of a circle given its center at (5, -2) and a midpoint of a segment joining the center and a point on the circle at (-2, 1).
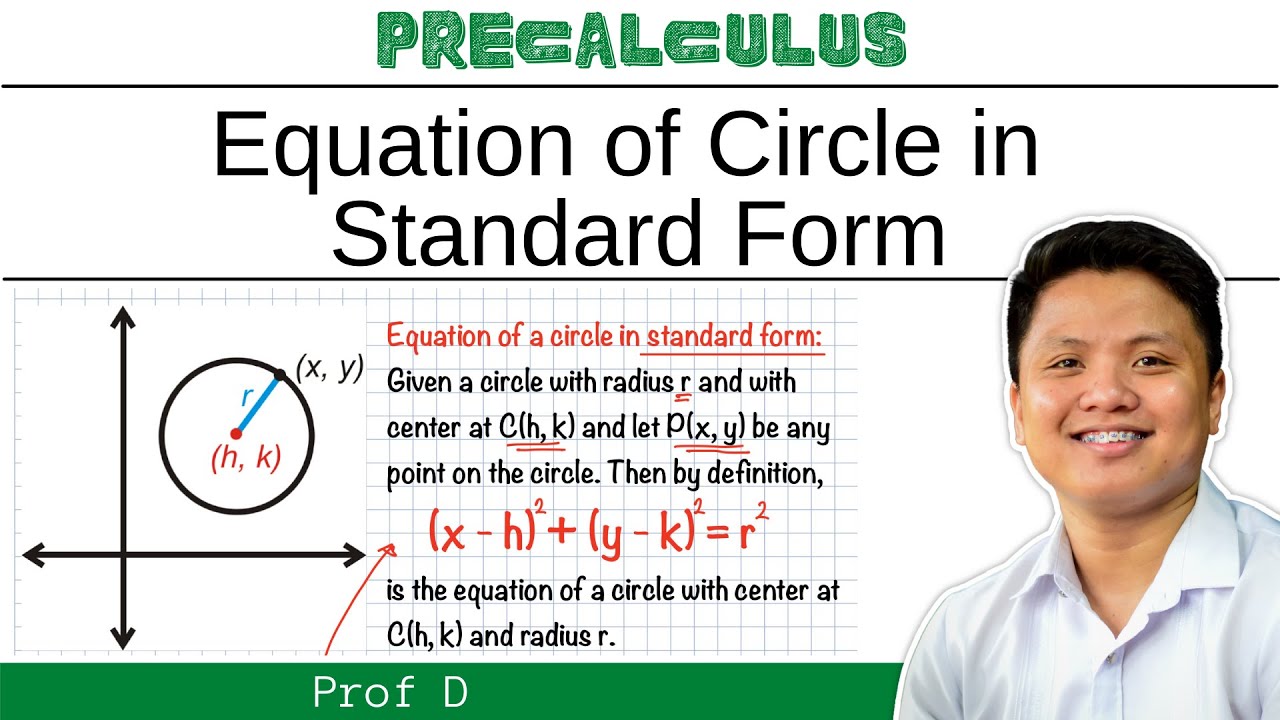
  • 🔍 The standard equation of a circle is used: \((x - h)^2 + (y - k)^2 = r^2\), where \(h\) and \(k\) are the coordinates of the center.
  • 📐 The midpoint formula is applied to find the radius of the circle: \(\sqrt{(x_2 - x_1)^2 + (y_2 - y_1)^2}\).
  • 🧩 The calculation for the radius leads to \(\sqrt{(-2 - 5)^2 + (1 - (-2))^2} = 2\sqrt{58}\).
  • 🚧 The second problem asks for the height of a semicircular tunnel with a 10-foot wide road at the edge of each lane.
  • 🌕 The tunnel's semicircle has a radius of 9 feet, and the center is at the origin (0,0).
  • 📏 The height of the tunnel at the edge of each lane is found using the circle's equation and the given coordinates.
  • 📉 The height calculation results in \(y = \sqrt{56} - 5 \approx 7.48\) feet, rounded to two decimal places.
  • 🎡 The third problem is about a Ferris wheel elevated 1 meter above the ground, with the car at the highest point 31 meters from the ground.
  • 🎢 To find the horizontal distance from the center when the car is at an altitude of 24 meters, the diameter of the Ferris wheel is calculated first.
  • 📐 The diameter is determined as 30 meters, and the radius as 15 meters, using the height of the Ferris wheel and the car's altitude.
  • 📈 The horizontal distance is calculated using the Pythagorean theorem, resulting in approximately 12.68 meters.
Q & A

  • What is the equation of a circle with center at (5, -2) and a midpoint of a segment joining the center and a point on the circle being (-2, 1)?

    -The equation of the circle is (x - 5)^2 + (y + 2)^2 = 232. This is derived from the standard circle equation (x - h)^2 + (y - k)^2 = r^2, where (h, k) is the center and r is the radius. The radius is calculated using the distance formula between the center and the midpoint.

  • How do you calculate the radius of the circle given in the transcript?

    -The radius is calculated using the distance formula: √[(x2 - x1)^2 + (y2 - y1)^2]. In this case, the center is (5, -2) and the midpoint is (-2, 1), so the radius squared (r^2) is 232.

  • What is the height of the tunnel at the edge of each lane of a single line street that goes through a semicircular tunnel with a radius of 9 feet?

    -The height of the tunnel at the edge of each lane is 7.48 feet. This is found by setting up the equation y^2 + (x - 5)^2 = 9^2 and solving for y when x is at the edge of the lane.

  • How do you determine the height of the tunnel at the edge of the lane if the street is 10 feet wide?

    -Since the street is 10 feet wide and the tunnel is semicircular with a radius of 9 feet, the street is divided equally on both sides of the center, 5 units to the left and 5 units to the right. The height is then calculated at the edge of the lane, which is 5 units from the center.

  • What is the diameter of a Ferris wheel if the car at the highest point is 31 meters above the ground and the wheel is elevated 1 meter above the ground?

    -The diameter of the Ferris wheel is 30 meters. This is calculated by subtracting the elevation of the wheel (1 meter) from the total height at the highest point (31 meters).

  • How do you find the horizontal distance from the center of the Ferris wheel when the car is at an altitude of 24 meters from the ground?

    -First, calculate the radius of the Ferris wheel, which is half of the diameter (15 meters). Then, knowing the car's altitude from the lowest point of the wheel is 23 meters (24 meters - 1 meter elevation), use the Pythagorean theorem to find the horizontal distance (x) where x^2 + 8^2 = 15^2.

  • What is the horizontal distance from the center of the Ferris wheel when a car is at an altitude of 24 meters from the ground?

    -The horizontal distance is 12.68 meters. This is found by solving the equation x^2 = 64 - 225, which simplifies to x = √161.

  • What is the significance of the midpoint in the first problem of finding the equation of the circle?

    -The midpoint is crucial as it helps determine the radius of the circle. It is used in conjunction with the center to apply the distance formula and find the radius squared, which is a key component of the circle's equation.

  • How does the problem involving the semicircular tunnel relate to the geometry of a circle?

    -The problem involves a semicircular tunnel, which is half of a circle. The geometry of a circle is used to determine the height of the tunnel at the edge of the lane by applying the circle's equation to find the y-coordinate.

  • What mathematical concepts are applied in the transcript to solve the problems?

    -The transcript applies concepts such as the standard equation of a circle, the distance formula, the Pythagorean theorem, and the concept of midpoint to solve the geometric problems.

  • Why is it necessary to round off the height of the tunnel to two decimal places in the second problem?

    -Rounding off to two decimal places is necessary to provide a practical and precise measurement for the height of the tunnel, which is typically required in real-world applications and engineering designs.

  • How does the elevation of the Ferris wheel affect the calculation of the horizontal distance from its center?

    -The elevation of the Ferris wheel is taken into account when calculating the total altitude of the car from the ground. This total altitude is then used to find the horizontal distance from the center using the Pythagorean theorem.

Outlines
00:00
📚 Solving the Equation of a Circle

The first paragraph introduces a problem-solving session focused on mathematical equations. The main issue discussed is finding the equation of a circle with a given center at (5, -2) and a midpoint of a segment joining the center to a point on the circle, which is (-2, 1). The process involves using the standard equation of a circle, \((x-h)^2 + (y-k)^2 = r^2\), and substituting the known values to find the radius. The calculation proceeds with determining the distance between the center and the midpoint using the distance formula and eventually leads to the circle's equation with a radius squared of 232.

05:01
🏗️ Calculating the Height of a Semi-circular Tunnel

The second paragraph presents a problem related to a semi-circular tunnel with a 10 feet wide street running through it. The task is to calculate the height of the tunnel at the edge of each lane. Using the equation of a circle, the center is assumed to be at the origin (0,0) with a radius of 9 feet. The street width is divided equally on both sides of the tunnel, with each lane being 5 feet from the center. The height calculation involves setting up an equation based on the circle's geometry and solving for the y-coordinate at the edge of the lane, which results in a height of 7.48 feet when rounded to two decimal places.

🎡 Determining the Horizontal Distance on a Ferris Wheel

The final paragraph deals with a Ferris wheel problem where the wheel is elevated 1 meter above the ground, and the car at its highest point is 31 meters from the ground level. The goal is to find the horizontal distance from the center of the wheel when the car is at an altitude of 24 meters. The solution starts by determining the diameter of the Ferris wheel, which is 30 meters, and then calculating the radius. The altitude of the car is adjusted for the wheel's elevation, resulting in a 23-meter vertical distance from the wheel's lowest point. Using the Pythagorean theorem, the horizontal distance is calculated to be 12.68 meters when the car is at the specified altitude.

Mindmap
Keywords
💡Equation of a Circle
The equation of a circle is a fundamental concept in geometry that defines a circle's shape using algebraic expressions. It is typically represented as \((x - h)^2 + (y - k)^2 = r^2\), where \((h, k)\) is the center of the circle and \(r\) is its radius. In the video, this equation is used to find the equation of a circle given its center and a point on the circle, which is crucial for solving the first problem presented.
💡Midpoint
The midpoint of a segment in geometry is the point that divides the segment into two equal parts. It is calculated as the average of the coordinates of the endpoints. In the context of the video, the midpoint is used to help determine the equation of the circle, as it provides a point on the circle that, along with the center, helps to define the circle's radius.
💡Semicircular Tunnel
A semicircular tunnel refers to a tunnel that has a half-circle shape. In the video, the problem involves calculating the height of such a tunnel at the edge of a lane. This concept is important for understanding how the geometry of a semicircle can be applied to real-world structures like tunnels.
💡Radius
The radius of a circle is the distance from the center of the circle to any point on its circumference. In the video, the radius is used to describe the size of the semicircular tunnel and the Ferris wheel, and it is a key component in solving the problems related to these structures.
💡Ferris Wheel
A Ferris wheel is a large amusement ride consisting of a rotating upright wheel with passenger cabins attached to the rim. In the video, a problem is solved involving the height and horizontal distance of a car on a Ferris wheel, demonstrating the application of geometric principles to understand the motion and position of objects on such a ride.
💡Altitude
Altitude in the context of the video refers to the height of an object above a reference point, such as the ground level. The script discusses the altitude of a car on a Ferris wheel and uses it to calculate the horizontal distance from the center of the wheel, showing how altitude can be used to describe the position of objects in three-dimensional space.
💡Horizontal Distance
Horizontal distance is the measurement of distance along a horizontal plane, as opposed to a vertical distance. In the video, the horizontal distance is calculated to determine how far a car on a Ferris wheel is from the center when it is at a certain altitude, illustrating the use of geometry to find positions in a two-dimensional plane.
💡Diameter
The diameter of a circle is any straight line segment that passes through the center of the circle and connects two points on the circle. It is twice the radius. In the video, the diameter of the Ferris wheel is calculated to help find the horizontal distance of the car at a given altitude, highlighting the relationship between the diameter and the circle's properties.
💡Pythagorean Theorem
The Pythagorean theorem is a fundamental principle in geometry that states that in a right-angled triangle, the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the lengths of the other two sides. In the video, this theorem is implicitly used to calculate distances and solve problems involving right triangles, such as finding the height of the semicircular tunnel.
💡Distance Formula
The distance formula is derived from the Pythagorean theorem and is used to calculate the distance between two points in a coordinate plane. It is expressed as \(\sqrt{(x_2 - x_1)^2 + (y_2 - y_1)^2}\). In the video, the distance formula is used to find the radius of the circle by calculating the distance between the center and a point on the circle.
💡Transposition
Transposition in mathematics refers to the process of rearranging terms in an equation or expression. In the context of the video, transposition is used when solving for the height of the semicircular tunnel, where terms are moved to the other side of the equation to isolate the variable of interest.
Highlights

Finding the equation of a circle with a given center and midpoint.

Using the standard equation of a circle to substitute known values.

Calculating the distance between points on a circle using the distance formula.

Solving for the radius squared in the circle's equation.

Determining the height of a semicircular tunnel at the edge of a lane.

Applying the circle equation to a semicircular tunnel scenario.

Using geometric properties to find the y-value at the edge of the lane.

Calculating the height of the tunnel with a given radius and lane position.

Rounding off the calculated height to two decimal places.

Exploring the altitude of a Ferris wheel car and its horizontal distance from the center.

Determining the diameter of the Ferris wheel based on the car's highest altitude.

Calculating the horizontal distance from the center at a specific altitude.

Using the Pythagorean theorem to find the horizontal distance.

Subtracting the altitude from the diameter to find the remaining distance.

Solving for the horizontal distance using algebraic manipulation.

Final calculation of the horizontal distance from the center of the Ferris wheel.

Encouraging self-discovery and problem-solving by attempting the last question independently.

Transcripts

Browse More Related Video

PROBLEM SOLVING INVOLVING CIRCLES

INTERESTING JEE Advanced Problem

How to find the standard form of the circle | Circle | Conic Section | Pre-Calculus

EQUATION OF CIRCLE IN STANDARD FORM | PROF D

PART 3 : CIRCLES || PRE - CALCULUS

PROBLEM SOLVING INVOLVING ELLIPSE

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
GeometryCircle EquationProblem SolvingSemicircular TunnelFerris WheelAltitude CalculationDistance FormulaRadius CalculationMidpointTrigonometry