How to find the standard form of the circle | Circle | Conic Section | Pre-Calculus

Prof D
3 Jul 202205:14
EducationalLearning
32 Likes 10 Comments

TLDRThe video script demonstrates the process of finding the standard form equation of a circle given the endpoints of its diameter. It begins by using the distance formula to calculate the diameter between points A(4,5) and B(-2,3), determining a radius of √10. The midpoint formula is then applied to find the circle's center at (1,4). The standard form equation of the circle is derived as (x-1)² + (y-4)² = 10. The script concludes with instructions on how to sketch the graph of the circle, emphasizing the importance of plotting the center and using the radius to draw the circle's outline.

Takeaways
  • 📚 The task involves finding the standard form equation of a circle and sketching its graph.
  • 📏 The diameter of the circle is defined by the endpoints A(4,5) and B(-2,3).
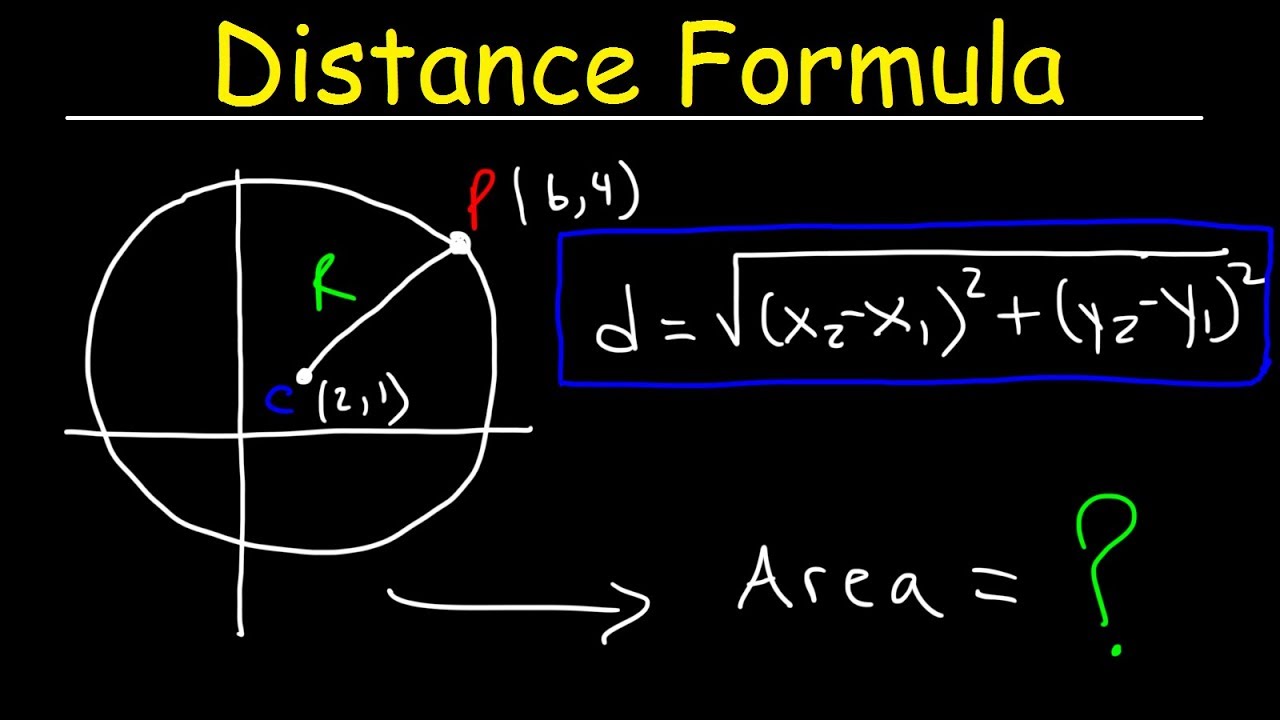
  • 🔍 The distance formula is used to calculate the length of the diameter: \( \sqrt{(x_2 - x_1)^2 + (y_2 - y_1)^2} \).
  • 📐 The midpoint of the line segment AB is the center of the circle, calculated using the midpoint formula.
  • 🔢 The radius (r) of the circle is half the diameter, which is \( \sqrt{10} \).
  • 📍 The center of the circle is found to be at (1,4) by applying the midpoint formula.
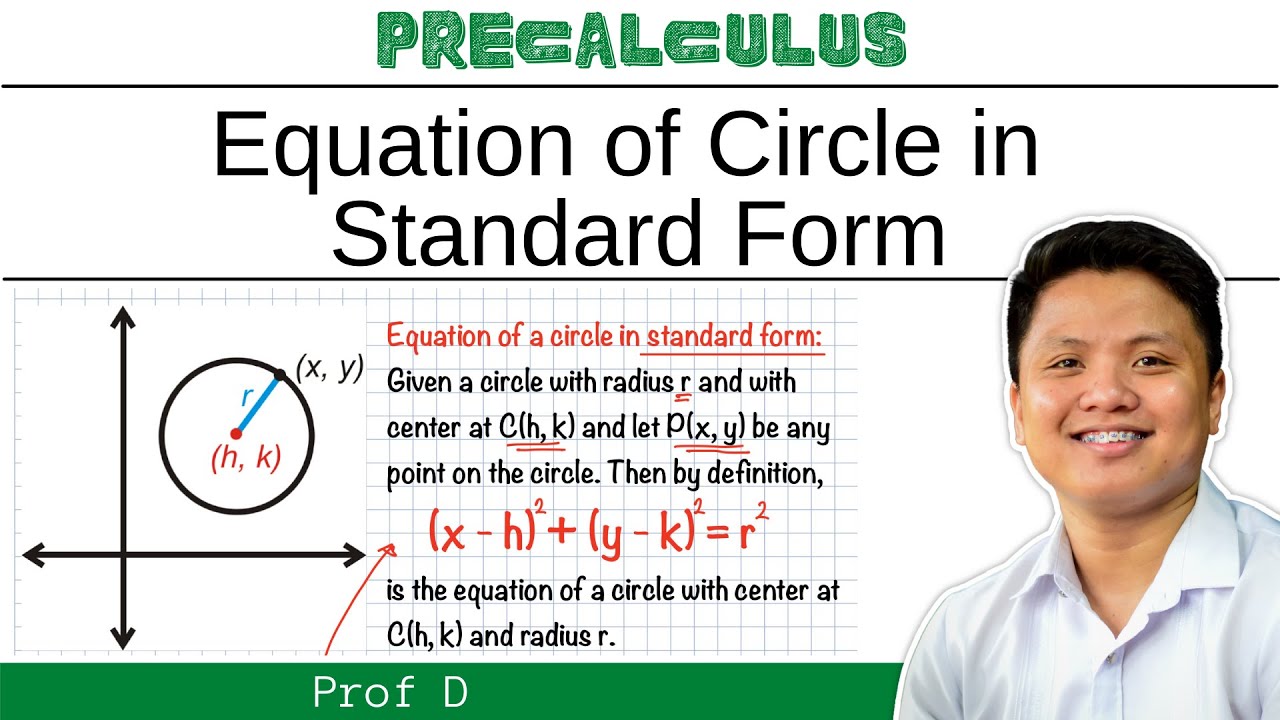
  • 🧩 The standard form equation of a circle is \( (x - h)^2 + (y - k)^2 = r^2 \).
  • 📝 The values for h, k, and r are substituted into the standard equation to get \( (x - 1)^2 + (y - 4)^2 = 10 \).
  • 🎨 To sketch the graph, the center (1,4) is plotted, and the radius \( \sqrt{10} \) is used to locate four points on the circle.
  • 🖌️ The four points are then connected to form the circle's graph.
Q & A

  • What are the coordinates of point A mentioned in the transcript?

    -Point A has coordinates (4, 5).

  • What are the coordinates of point B mentioned in the transcript?

    -Point B has coordinates (-2, 3).

  • What is the distance formula used to find the diameter of the circle?

    -The distance formula used is \( d = \sqrt{(x_2 - x_1)^2 + (y_2 - y_1)^2} \).

  • What is the length of the diameter of the circle?

    -The length of the diameter is \( 2\sqrt{10} \).

  • How is the radius of the circle calculated?

    -The radius of the circle is half the length of the diameter, which is \( \sqrt{10} \).

  • What is the midpoint formula used to find the center of the circle?

    -The midpoint formula used is \( (h, k) = \left(\frac{x_2 + x_1}{2}, \frac{y_2 + y_1}{2}\right) \).

  • What are the coordinates of the center of the circle?

    -The center of the circle is at (1, 4).

  • What is the standard form equation of the circle?

    -The standard form equation of the circle is \( (x - 1)^2 + (y - 4)^2 = 10 \).

  • How can you find the four points on the axis to sketch the circle?

    -Plot the center and then use the radius to find the points on the x and y axes where the circle intersects.

  • What is the quadrant in which the center of the circle is located?

    -The center of the circle is located in Quadrant I.

  • How do you sketch the graph of the circle after plotting the center?

    -After plotting the center, plot the four points of the circle using the given radius, then connect these points to form the circle.

  • What is the significance of the radius in the standard form equation of the circle?

    -The radius, when squared, determines the size of the circle and its distance from the center to any point on the circle.

  • How does the distance between point A and point B relate to the circle's diameter?

    -The distance between point A and point B is the length of the diameter of the circle, which is used to calculate the radius.

Outlines
00:00
📚 Finding the Standard Form Equation of a Circle

The script begins with an example of finding the standard form equation of a circle given the endpoints of its diameter. The endpoints are A(4,5) and B(-2,3). To find the equation, the first step is to calculate the diameter's length using the distance formula. The midpoint of the line segment AB is determined to be the center of the circle, which is calculated to be at (1,4). The radius is half the diameter, which simplifies to √10. The standard form equation of the circle is then derived using the formula (x-h)² + (y-k)² = r², where h, k, and r are the center coordinates and radius, respectively. Substituting the values, the equation becomes (x-1)² + (y-4)² = 10.

05:01
🎨 Sketching the Graph of the Circle

The second paragraph continues with the process of graphing the circle. It starts by plotting the center of the circle at coordinates (1,4). Then, using the radius, which is √10, four points on the circumference of the circle are plotted. These points are located at a distance of √10 units from the center along the x and y axes. Finally, these points are connected to form the complete circle. The paragraph concludes by describing the visual representation of the circle in the coordinate plane.

Mindmap
Keywords
💡Circle
A circle is a two-dimensional geometric shape consisting of all points that are equidistant from a central point, known as the center. In the video, the circle is the main subject, and the process of finding its equation and graphing it is the central theme. The video script uses the term to describe the shape whose equation and graph are being determined.
💡Diameter
The diameter of a circle is a line segment that passes through the center of the circle and connects two points on the circle. It is twice the length of the radius. In the context of the video, the diameter is determined by the endpoints of the line segment, A(4,5) and B(-2,3), and is used to find the radius of the circle.
💡Endpoint
An endpoint refers to one of the two points that define the limits of a line segment or interval. In the video, the endpoints are the coordinates of points A and B, which are used to calculate the diameter of the circle and subsequently the circle's properties.
💡Standard Form Equation
The standard form equation of a circle is given by the formula (x-h)² + (y-k)² = r², where (h,k) is the center of the circle and r is the radius. The video explains how to derive this equation for a specific circle by finding its center and radius.
💡Center
The center of a circle is the point equidistant from all points on the circle, and it is the point around which the circle is symmetric. In the video, the center is found using the midpoint formula applied to the endpoints of the diameter, resulting in the coordinates (1,4).
💡Radius
The radius is the distance from the center of the circle to any point on the circle. It is half the length of the diameter. The video demonstrates how to calculate the radius by halving the diameter's length, which is derived from the distance between points A and B.
💡Distance Formula
The distance formula is a mathematical formula used to find the distance between two points in a coordinate plane. It is given by √((x₂-x₁)² + (y₂-y₁)²). In the video, the distance formula is applied to find the length of the diameter of the circle.
💡Midpoint Formula
The midpoint formula is used to find the midpoint of a line segment given its endpoints. It is calculated as ((x₁+x₂)/2, (y₁+y₂)/2). The video uses this formula to determine the center of the circle by finding the midpoint of the diameter's endpoints.
💡Graph
In the context of mathematics, to graph means to represent a mathematical relationship on a coordinate plane. The video describes the process of graphing a circle by plotting its center and the points on the circle using the radius.
💡Quadrant
A quadrant refers to one of the four equal regions created by the intersection of the x-axis and y-axis on a coordinate plane. The video mentions plotting the center of the circle in quadrant one, which is the region where both x and y coordinates are positive.
Highlights

Introduction to example number two involving a circle with a specific diameter.

Identifying the end points of the circle's diameter: point A (4,5) and point B (-2,3).

Using the distance formula to calculate the length of the circle's diameter.

Substituting coordinates into the distance formula to find the diameter.

Simplifying the equation to find the diameter's length as 2√10.

Determining the radius of the circle by halving the diameter.

Calculating the radius to be √10.

Finding the midpoint of the line segment AB to locate the circle's center.

Applying the midpoint formula to determine the center's coordinates.

Calculating the center's x-coordinate (h) to be 1.

Calculating the center's y-coordinate (k) to be 4.

Identifying the center of the circle as the point (1,4).

Using the standard form equation for a circle to express the relationship between h, k, and r.

Substituting the values of h, k, and r into the standard equation.

Simplifying the equation to obtain the circle's standard form: (x-1)² + (y-4)² = 10.

Describing the process of sketching the graph of the circle.

Plotting the center of the circle on the coordinate plane.

Determining the four points on the circle using the radius.

Connecting the points to form the circle's graph.

Transcripts

Browse More Related Video

EQUATION OF CIRCLE IN STANDARD FORM | PROF D

PART 3 : CIRCLES || PRE - CALCULUS

PROBLEM SOLVING INVOLVING CIRCLES

Distance Formula

CIRCLES || PRE-CALCULUS

Graphing Conic Sections Part 1: Circles

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Circle GeometryDiameter CalculationMidpoint FormulaStandard EquationGraph SketchingDistance FormulaRadius DeterminationCenter CoordinatesMath TutorialEducational ContentGeometry Lesson