Relative and Absolute Maximums and Minimums | Part II
TLDRThe video script delves into the application of calculus in identifying extrema, such as maximums and minimums, on a graph. It clarifies that while a horizontal tangent line, indicating a derivative of zero, often signifies a maximum or minimum, this is not always the case. For instance, a cubic function can have a point where the derivative is zero but is neither a maximum nor a minimum. The concept of a critical number is introduced, which is a point where the derivative is zero or does not exist. These points are potential candidates for extrema but not all critical numbers guarantee a maximum or minimum. The script also discusses the importance of Fermat's Theorem, which states that all relative extrema occur at critical numbers. The process for finding maximums and minimums involves identifying all critical numbers within the domain, calculating the function's value at these points and the endpoints, and then analyzing which of these values represent absolute or relative extrema. The video concludes by suggesting that visual inspection of the graph can serve as a check for the computed extrema.
Takeaways
- 📈 Calculus is used to find exact locations of extrema (maxima and minima) on a graph, which can represent various quantities like energy, population size, or profit.
- 🔍 A horizontal tangent line on a graph at a point indicates that the derivative at that point is zero.
- 🤔 A zero derivative does not always signify a maximum or minimum; it could also be an inflection point.
- 🔑 The concept of a critical number is introduced, which is a point where the derivative is zero or does not exist.
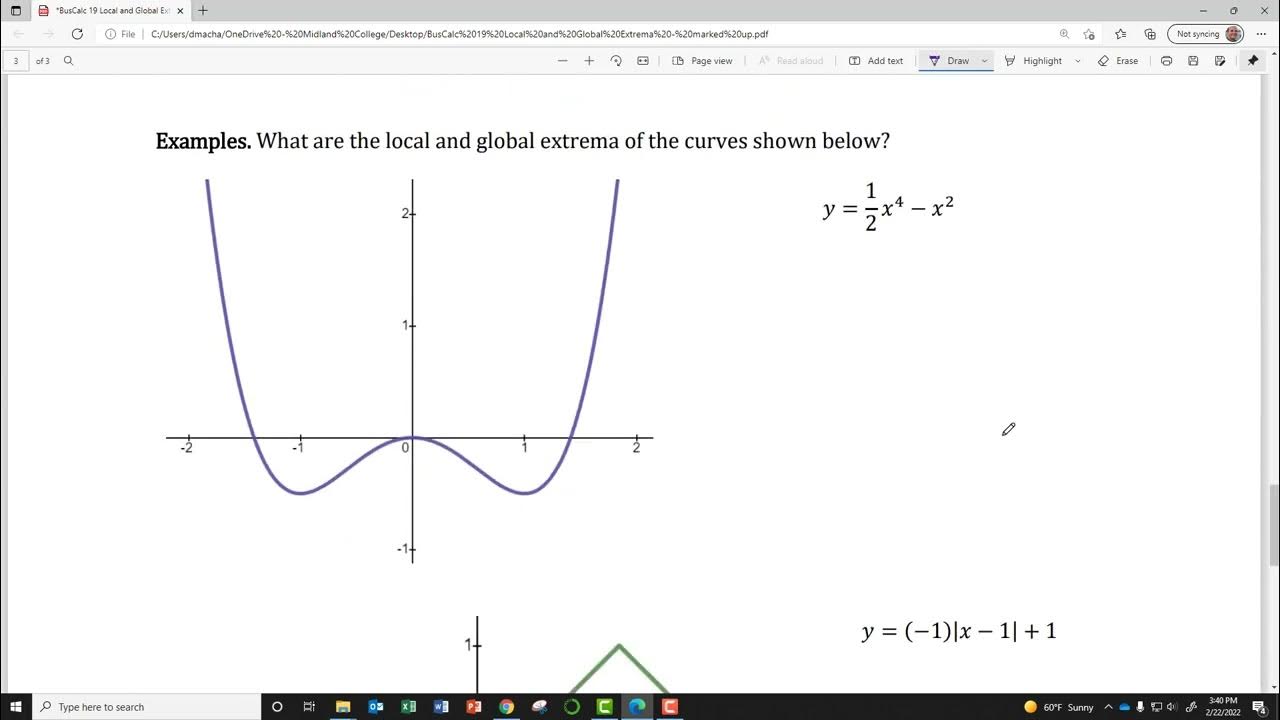
- 🚫 A derivative that doesn't exist at a point can still correspond to a maximum or minimum, as illustrated by a function with an absolute value.
- 📌 Rolle's theorem states that every relative maximum and minimum must occur at a critical number.
- 🎯 Critical numbers are potential candidates for relative maxima or minima, but not all critical numbers will result in extrema.
- 🔍 To find maxima and minima on an interval, first identify all critical numbers within the domain.
- ✅ Evaluate the function at each critical number and at the endpoints of the domain to determine potential extrema.
- 📊 Compare the function values to identify the absolute maximum and minimum from the list of evaluated points.
- 📐 Further analysis is required to determine which critical numbers correspond to relative maxima, minima, or neither.
- 🖌️ It's beneficial to visually inspect the graph as a check to see if the computed maxima and minima align with the graph's behavior.
Q & A
What is the significance of a horizontal tangent line on a graph?
-A horizontal tangent line on a graph indicates that the derivative at that point is equal to zero. This often corresponds to a relative maximum or minimum, but it's not always the case as there can be points where the tangent is horizontal but do not represent extrema.
Is it true that every time the derivative is zero, there is a relative maximum or minimum?
-No, it is not always true. While a zero derivative often suggests a potential maximum or minimum, there are instances, such as a cubic function at its inflection point, where the derivative is zero but it is neither a maximum nor a minimum.
Can a function have a maximum or minimum where the derivative does not exist?
-Yes, a function can have a maximum or minimum at a point where the derivative does not exist. An example given is a function involving an absolute value, which can have a maximum at a cusp where the derivative is undefined.
What is a critical number?
-A critical number is a value in the domain of a function where the derivative is either zero or does not exist. These points are of interest because they are potential locations for relative maxima or minima.
What is Fermat's Theorem and how does it relate to critical numbers?
-Fermat's Theorem states that every relative maximum or minimum must occur at a critical number. If there is no critical number, there is no maximum or minimum at that point.
Is it necessary for a function to have a critical number to have a maximum or minimum?
-Yes, according to Fermat's Theorem, a function must have a critical number for it to have a relative maximum or minimum. However, not all critical numbers result in maxima or minima; further analysis is needed to determine this.
What is the process for finding the maximums and minimums of a function on an interval?
-First, find all the critical numbers within the specified domain. Then, compute the function values for each critical number and the endpoints of the domain. Finally, compare these values to determine the absolute and relative maxima and minima.
Why is it important to also consider the endpoints of the domain when finding maxima and minima?
-Endpoints are important because the function's maximum or minimum can occur at these points. Even if an endpoint is not a critical number, it can still represent a maximum or minimum value for the function on the interval.
How can one determine whether a critical number corresponds to a maximum, minimum, or neither?
-After identifying the critical numbers and calculating the function values at these points and the endpoints, further analysis such as the second derivative test or comparing these values can help determine if a critical number is a maximum, minimum, or neither.
What is the second derivative test and how is it used?
-The second derivative test involves evaluating the second derivative of a function. If the second derivative at a critical point is positive, the point is a local minimum. If it's negative, the point is a local maximum. If the second derivative is zero or undefined, the test is inconclusive and other methods must be used.
Can one always rely on the graph of a function to confirm the maxima and minima calculated?
-While the graph can provide a visual confirmation, it is not always entirely reliable due to potential inaccuracies in graphing or limitations of the graph's resolution. It is always best to perform the mathematical analysis to confirm the maxima and minima.
Why is calculus useful in finding exact locations and values of extrema?
-Calculus, through the use of derivatives, allows for the precise identification of points where extrema occur. It provides a systematic method to analyze the behavior of a function and determine not just the approximate locations, but the exact points and corresponding function values of maxima and minima.
Outlines
📈 Understanding Maxima and Minima with Calculus
The paragraph discusses the use of calculus to find exact points of extrema (maxima and minima) on a graph, which could represent various quantities like energy, population size, or profit. It emphasizes the role of the derivative being equal to zero at maximum points, as indicated by a horizontal tangent line. However, it challenges the assumption that every zero derivative indicates a maximum or minimum by presenting a cubic function example where the derivative is zero but does not correspond to an extremum. The paragraph also explores the possibility of having a maximum or minimum where the derivative does not exist, using an absolute value function as an example. It concludes by introducing the concept of critical numbers, which are points where the derivative is either zero or does not exist, and highlights Fermat's Theorem, which states that all relative maxima and minima must occur at critical numbers.
🔍 Identifying and Analyzing Critical Numbers
This paragraph elaborates on the process of finding maxima and minima on an interval. It explains that critical numbers, where the derivative is zero or does not exist, are potential candidates for maxima or minima but not all critical numbers are guaranteed to be so. The method involves finding all critical numbers within the specified domain, calculating the function's value at these points and at the domain's endpoints, and then comparing these values to determine the absolute and relative maxima and minima. The paragraph also suggests a final step of verifying the computed maxima and minima against the graph for accuracy.
Mindmap
Keywords
💡Extrema
💡Derivative
💡Tangent Line
💡Relative Maximum/Minimum
💡Critical Number
💡
💡Fermat's Theorem
💡Absolute Maximum/Minimum
💡Cusp Point
💡Endpoint
💡Graph
💡Calculus
Highlights
Calculus is a useful tool for finding exact locations of extrema on a graph.
A horizontal tangent line on a graph indicates that the derivative at that point is zero.
Zero derivative does not always correspond to a maximum or minimum; it can also indicate an inflection point.
The concept of a critical number is introduced, which is a point where the derivative is either zero or does not exist.
Fermat's Theorem states that all relative maxima and minima must occur at critical numbers.
Not all critical numbers result in maxima or minima; they are merely candidates that require further analysis.
The process to find maxima and minima involves identifying critical numbers within the specified domain.
Endpoints of a domain are also considered as they can potentially represent maxima or minima even if not critical numbers.
After identifying critical numbers and endpoints, calculate the function's value at these points.
Compare the calculated values to determine the absolute maximum and minimum.
Further analysis is needed to distinguish between relative maxima, minima, and neither for intermediate values.
Graphical representation can be used as a check to confirm the calculated maxima and minima.
The derivative at a cusp point, such as with an absolute value function, does not exist but can still represent a maximum.
The importance of investigating both conditions where the derivative is zero and does not exist to understand the behavior of the function.
The method presented is a systematic approach to analyzing the extrema of a function on a given interval.
The process involves both algebraic manipulation and graphical analysis to ensure accuracy.
The transcript provides a clear, step-by-step guide to understanding and applying calculus to find extrema.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: