Calculus Review Maximum and Minimum Values of Functions
TLDRThis video script offers an in-depth exploration of maximums and minimums, crucial concepts for AP Calculus exams and college-level calculus courses. It distinguishes between relative (local) and absolute (global) maximums and minimums, emphasizing the importance of understanding their differences. The script delves into identifying these points on a function's graph, explaining that relative extrema occur within intervals, not at endpoints, and are found at critical points where the derivative is zero or undefined. The first and second derivative tests are introduced as methods to determine the nature of these points, with the first derivative test being the most common approach. The video also highlights the significance of the second derivative test in scenarios where the first derivative is not easily interpretable. The script further discusses the application of the Candidate's Test for finding absolute extrema and the use of accumulation functions in related problems. Additionally, it touches on the Extreme Value Theorem, which underpins the Candidate's Test, and the Lonely Critical Point Theorem, a useful concept when a function has only one critical point. Finally, the script connects these ideas to finding the range of a function by considering relative extrema, limits at infinity, and the function's behavior. The summary underscores the video's comprehensive approach to a complex topic, providing learners with a clear roadmap for understanding and applying these mathematical concepts.
Takeaways
- 📈 The concept of relative (local) maxima and minima versus absolute (global) maxima and minima is crucial for understanding calculus problems.
- 🚫 Relative maxima and minima cannot occur at endpoints of a function's domain; they must occur within an open interval.
- 🔑 Critical points are essential in identifying potential maxima and minima and are where the derivative is either zero or undefined.
- 🧐 Not all critical points are maxima or minima; further analysis is required to determine their nature.
- 📉 The first derivative test involves checking the sign change of the derivative around a critical point to determine if it's a relative maximum or minimum.
- 📚 The second derivative test uses the sign of the second derivative at a critical point where the first derivative is zero to determine the nature of the point.
- 📊 Sign charts or graphs of the first derivative can be used visually to apply the first derivative test.
- 🔍 The Candidates' test (also known as the Extreme Value Theorem) is used to find absolute maxima and minima by evaluating endpoints and critical points of a continuous function on a closed interval.
- ⚖️ The extreme value theorem underpins the Candidates' test, stating that a continuous function on a closed interval will have an absolute maximum and minimum.
- 🏔 The Lonely Critical Point theorem states that if a function has only one critical point on a closed interval and it's a relative extremum, then it's also the absolute extremum.
- 📋 When finding the range of a function, consider the values at relative extrema, and the limits as x approaches positive and negative infinity.
Q & A
What is the difference between a relative maximum and an absolute maximum?
-A relative maximum (or local maximum) is a point on a function where the function value is the highest within an open interval around that point. An absolute maximum (or global maximum) is the highest value that the function reaches over its entire domain, which could be at an endpoint or at a critical point within the domain.
What are critical points in the context of calculus?
-Critical points are points in the domain of a function where the first derivative is either zero or does not exist. These points are potential locations for relative maxima, minima, or points of inflection.
What is the first derivative test for relative maxima and minima?
-The first derivative test involves examining the sign of the first derivative on either side of a critical point. If the first derivative changes from positive to negative at a critical point, the function has a relative maximum at that point. Conversely, if the first derivative changes from negative to positive, the function has a relative minimum.
How does the second derivative test work for classifying critical points?
-The second derivative test states that if the first derivative is zero at a critical point and the second derivative is positive, the function has a relative minimum at that point. If the second derivative is negative, the function has a relative maximum.
What is the Candidates' Test, and when is it used?
-The Candidates' Test is used to find the absolute maximum or minimum of a function on a closed interval. It states that if a function is continuous on the interval, the absolute maximum or minimum will occur at an endpoint or at a critical point within the interval.
What is the significance of the Extreme Value Theorem in calculus?
-The Extreme Value Theorem states that if a function is continuous on a closed interval, it must have both an absolute maximum and an absolute minimum on that interval. This theorem underpins the Candidates' Test and the Lonely Critical Point Theorem.
What is the Lonely Critical Point Theorem, and how is it applied?
-The Lonely Critical Point Theorem is a concept that, if a continuous function has only one critical point on a closed interval and that point is a relative maximum or minimum, then the function has an absolute maximum or minimum at that point. It is used when there is only one critical point to determine the absolute extremum.
How can one determine the range of a function?
-The range of a function can be determined by considering the function's values at its relative maxima and minima, as well as the limits as the function approaches positive and negative infinity. This provides the smallest and largest values the function can attain, which defines the range.
What is the role of the second derivative in the second derivative test?
-The second derivative helps determine the concavity of the function at a critical point. If the second derivative is positive, the function is concave up, indicating a relative minimum. If it is negative, the function is concave down, indicating a relative maximum.
Why is it important to justify conclusions when identifying maxima and minima?
-Justification is crucial because it provides a clear explanation of how the conclusion was reached. It demonstrates understanding of the concepts and ensures that the identification of maxima and minima is based on correct application of calculus principles.
What is the relationship between the first derivative and the sign chart?
-The first derivative can be used to create a sign chart, which is a visual representation of where the derivative is positive or negative. This helps in identifying intervals of increase or decrease in the function, which in turn helps in locating relative maxima and minima.
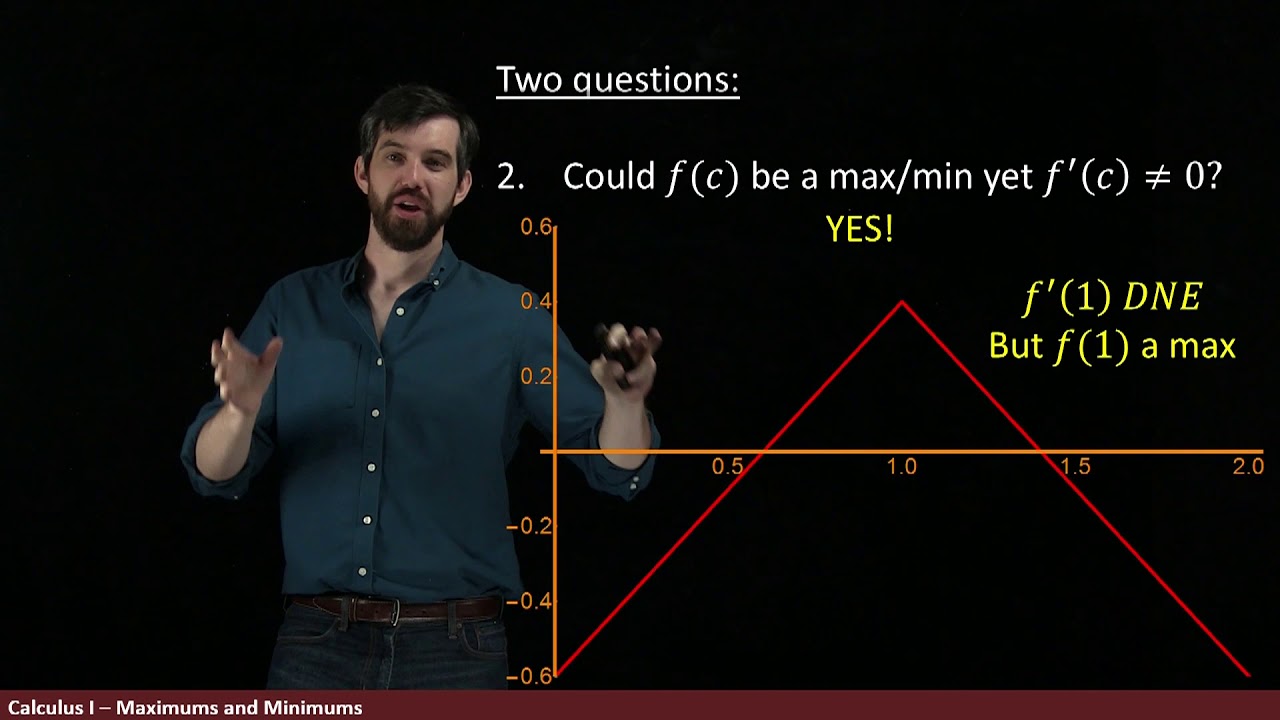
How can one determine if a function has neither a maximum nor a minimum at a critical point?
-By evaluating the first derivative at the critical point, if the derivative is neither zero nor undefined, the function does not have a relative maximum or minimum at that point. This is referred to as 'neither' in the context of classifying critical points.
Outlines
📚 Introduction to Maximums and Minimums
The video begins with an introduction to the concepts of maximums and minimums, focusing on the AP Calculus exam and offering a review for college-level calculus students. The presenter outlines the difference between relative/local and absolute/global maximums and minimums. The importance of critical points in identifying these extrema is emphasized, and the audience is introduced to the first derivative test as a method to determine relative maxima and minima.
🔍 First and Second Derivative Tests
The presenter explains the first derivative test for relative maximums and minimums, demonstrating how to use the sign change of the derivative to identify these points. The second derivative test is also introduced, providing a method to determine the nature of the extremum when the first derivative equals zero. The video includes examples and emphasizes the need for clear justification in exams, contrasting the use of the two tests and when each is appropriate.
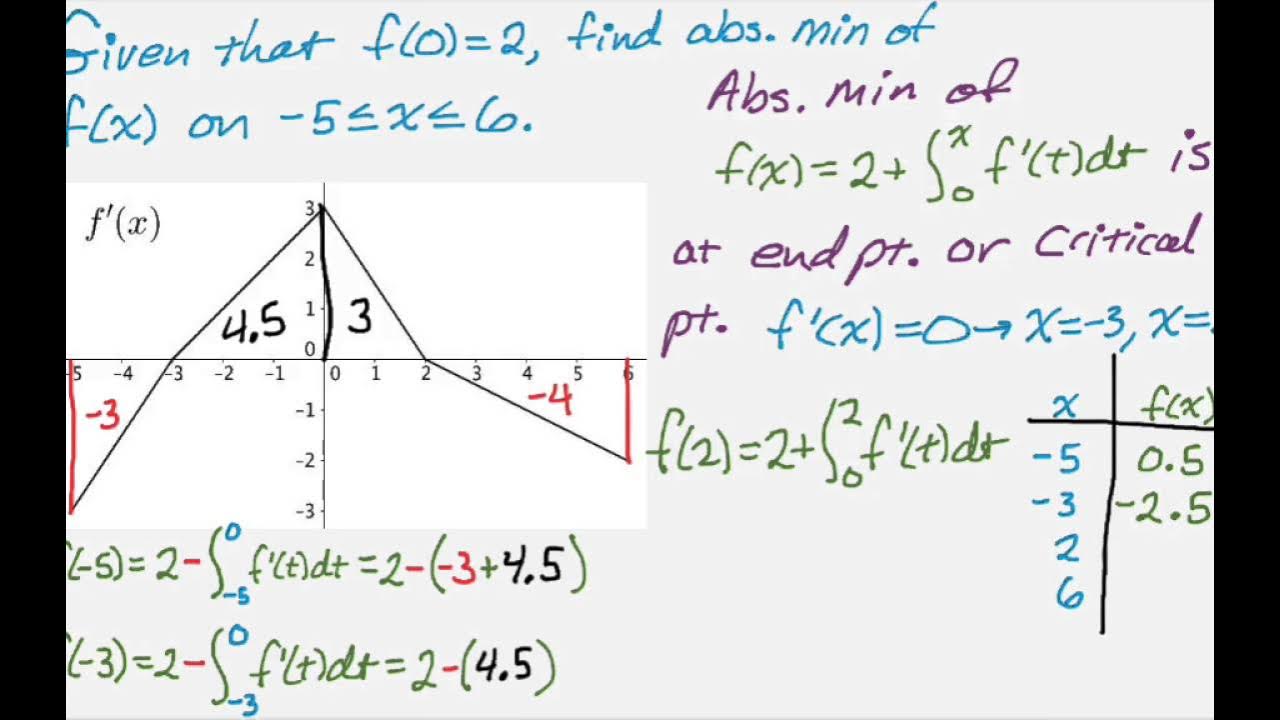
🔢 Absolute Maximum and Minimum
The discussion shifts to absolute maximum and minimum values, noting that these can occur at endpoints or critical points. The video explains that absolute extrema are often found using accumulation functions and emphasizes the importance of understanding what is being asked for in a given problem—whether it's the maximum or minimum value or the point at which it occurs. The candidate's test is introduced as a method to find absolute maxima and minima within a closed interval.
📈 The Extreme Value Theorem
The presenter discusses the Extreme Value Theorem, which underpins the candidate's test by ensuring that a continuous function on a closed interval will have an absolute maximum and minimum. The theorem is contrasted with cases where a function might not have a maximum or minimum due to discontinuities, such as vertical asymptotes or defined behavior that prevents a true maximum or minimum from occurring.
🏁 The Lonely Critical Point Theorem
The video introduces an informal concept called the 'Lonely Critical Point Theorem,' which is a practical approach when a function has only one critical point on a closed interval. The presenter explains that if this single critical point corresponds to a relative extremum, it must also be the absolute extremum. This theorem simplifies the process of finding absolute maxima and minima in certain scenarios.
🔄 Finding the Range of a Function
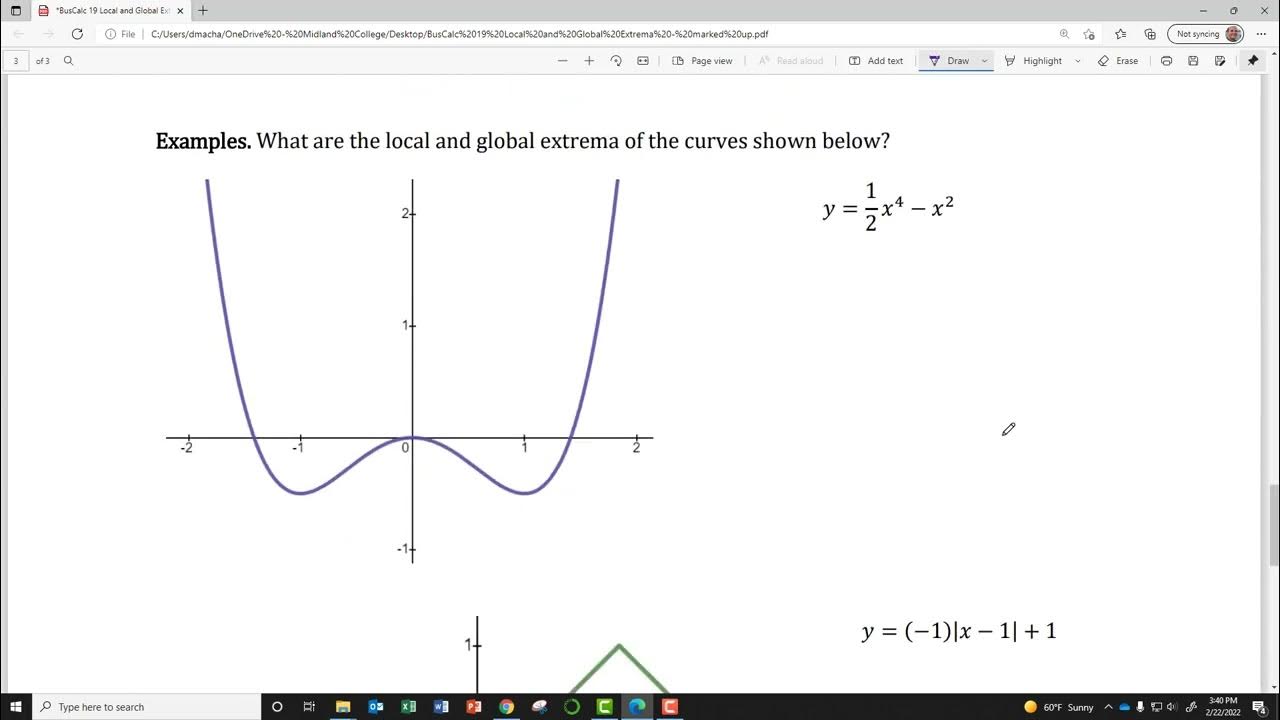
The final topic is finding the range of a function, which is tied to understanding maximum and minimum values. The presenter suggests that if a function is continuous, its range can be determined by considering the values at relative extrema and the limits as x approaches positive and negative infinity. A step-by-step approach is provided, including making a table of function values at points of interest and using this information to deduce the range of the function.
Mindmap
Keywords
💡Relative Maximums and Minimums
💡Absolute Maximum and Minimum
💡Critical Points
💡First Derivative Test
💡Second Derivative Test
💡Sign Chart
💡Endpoint Behavior
💡Accumulation Function
💡Extreme Value Theorem
💡Lonely Critical Point Theorem
💡Range of a Function
Highlights
The video discusses the concepts of relative and absolute maximums and minimums, essential for AP Calculus exams and college-level calculus courses.
Relative maximums and minimums occur within an open interval and cannot be at an endpoint.
Critical points are defined as points where the derivative is either zero or does not exist within the domain of the function.
Not all critical points are relative maxima or minima; further analysis is required to classify them.
The first derivative test is introduced as a common method to identify relative maxima and minima.
The second derivative test is presented as an alternative method for identifying relative maxima and minima when the first derivative is zero.
The importance of justifying conclusions when identifying maxima and minima is emphasized.
Absolute maximums and minimums can occur at endpoints or critical points and represent the highest or lowest value of a function on an interval.
The concept of accumulation functions is introduced, which are integrals representing the accumulation of a rate of change.
The Candidate's Test is explained as a method to find absolute maxima and minima by evaluating endpoints and critical points of a continuous function.
The Extreme Value Theorem is discussed, which states that a continuous function on a closed interval has an absolute maximum and minimum.
The Lonely Critical Point Theorem is described, which states that if a function has only one critical point and it's a relative extremum, then it's also the absolute extremum.
The process of finding the range of a function by considering relative extrema, and limits as x approaches positive and negative infinity is outlined.
The video provides a comprehensive overview of techniques to master for AP Calculus exams, including multiple methods for identifying and justifying maxima and minima.
The presenter uses visual aids like sign charts and derivative graphs to illustrate the concepts, making them more accessible for understanding.
The video emphasizes the practical applications of these concepts in calculus problems and how they are commonly tested in AP Calculus exams.
Different scenarios are explored where the first derivative test or the second derivative test would be more applicable, based on the information provided.
The importance of understanding the question's requirements, whether it's asking for the value or the x-coordinate of the extremum, is highlighted.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: