Calculus 1: Relative Extrema Examples

Allen Tsao The STEM Coach
5 Aug 201911:13
EducationalLearning
32 Likes 10 Comments

TLDRThis video script explores the application of derivatives to find absolute and relative minimums and maximums of a function using the first derivative test. It emphasizes the importance of identifying critical numbers where the derivative is zero and endpoints of an interval as potential candidates for extrema. The script provides step-by-step examples, including setting up a table to evaluate function values at critical points and endpoints, and demonstrates how to solve for critical points algebraically. It also explains why the first derivative test itself isn't always necessary when finding absolute extrema, as comparing values will ultimately determine the minimum and maximum. The tutorial concludes with a reminder to visit the instructor's website for more calculus examples and resources.

Takeaways
  • 📚 The video discusses applications of derivatives, specifically finding absolute and relative minimums and maximums using the first derivative test.
  • 🔍 To find a relative maximum, look for points where the first derivative is zero, as these are potential locations for extrema.
  • 📈 The first step in finding extrema is to identify critical numbers (where the derivative is zero) and endpoints of the interval.
  • 📝 The script provides an example of finding the absolute minimum value of a function within an interval by evaluating critical numbers and endpoints.
  • 🧮 It demonstrates how to set up a table to evaluate the function at critical numbers and endpoints, and then compare the values to find the minimum and maximum.
  • 🌐 The video explains that endpoints are not necessarily relative minimums or maximums, but they are always candidates for absolute extrema.
  • 📉 The process of finding extrema involves evaluating the function at critical points and endpoints, then determining which values are the smallest or largest.
  • 🤔 The speaker mentions that while the first derivative test could be used to classify relative minimums and maximums, it's not necessary for finding absolute extrema since a comparison is required anyway.
  • 📚 Another example given is finding the extrema of a function involving sine, where the derivative is set to zero to find critical points.
  • 🔢 The script walks through the process of finding critical numbers by setting the derivative equal to zero and solving for the variable.
  • 📘 The final example involves a function with a more complex derivative, demonstrating the use of power and product rules to find critical points.
Q & A

  • What is the primary application of derivatives discussed in the script?

    -The primary application of derivatives discussed in the script is to find absolute or relative minimums or maximums of a function using the first derivative test.

  • What is meant by 'critical numbers' in the context of finding minimums and maximums?

    -Critical numbers are the values of x where the first derivative of a function is zero or undefined. These are potential locations for relative minimums or maximums.

  • How does the first derivative test help in finding minimums and maximums?

    -The first derivative test helps by identifying critical numbers where the derivative is zero. Evaluating the function at these points and at the endpoints of the interval can help determine the minimums and maximums.

  • What is the process for finding the absolute minimum value of a function on a given interval?

    -The process involves identifying critical numbers by setting the derivative equal to zero, finding the endpoints of the interval, evaluating the function at these points, and then determining which value is the absolute minimum.

  • What is the significance of endpoints in finding minimums and maximums?

    -Endpoints are significant because they are always considered as candidates for minimums and maximums since the function's behavior at the boundaries of the interval can lead to extreme values.

  • Why does the script mention that the first derivative test is not necessary for classifying critical points in some cases?

    -The script mentions that the first derivative test is not necessary when the problem asks for both minimums and maximums because the comparison of values will inherently classify the critical points, making the explicit test redundant.

  • How does the script illustrate the process of finding minimums and maximums using a specific function?

    -The script uses the function f(x) = x^2 - x + 4 as an example, showing the steps of finding the derivative, solving for critical numbers, evaluating the function at endpoints and critical numbers, and comparing these values to find the minimum and maximum.

  • What is the role of the second derivative test in classifying critical points?

    -The second derivative test can be used to determine whether a critical point is a relative minimum, relative maximum, or neither by analyzing the concavity of the function at that point.

  • What is the difference between a local minimum and an absolute minimum?

    -A local minimum is the lowest point of a function within a certain neighborhood, whereas an absolute minimum is the lowest point of the function over the entire domain or a specified interval.

  • How does the script handle the derivative of a trigonometric function, specifically sine?

    -The script explains that the derivative of sine is cosine, and when dealing with a constant multiplied by sine, the derivative is simply the constant times cosine. The critical points are found where the cosine is zero.

  • What is the importance of considering the domain when finding minimums and maximums?

    -Considering the domain is crucial because it restricts the set of possible values that x can take, which in turn affects the endpoints and critical points used to find minimums and maximums.

Outlines
00:00
📚 Finding Absolute Extrema Using Derivatives

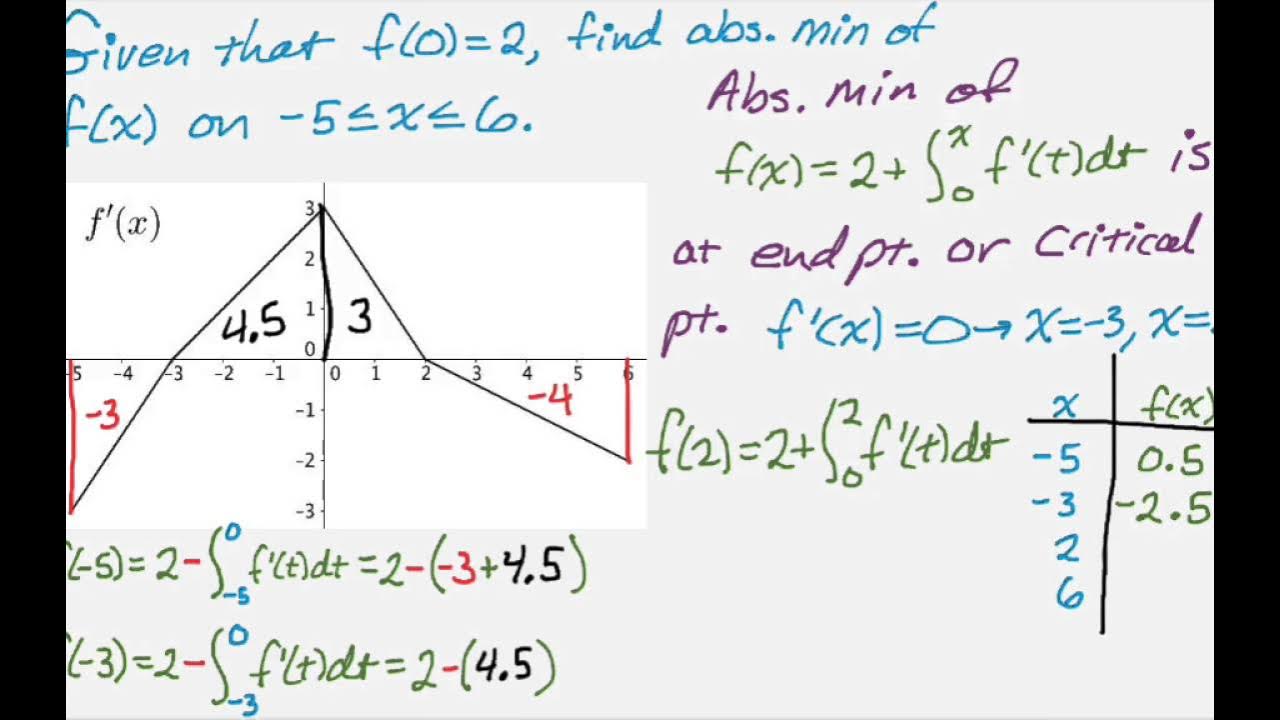
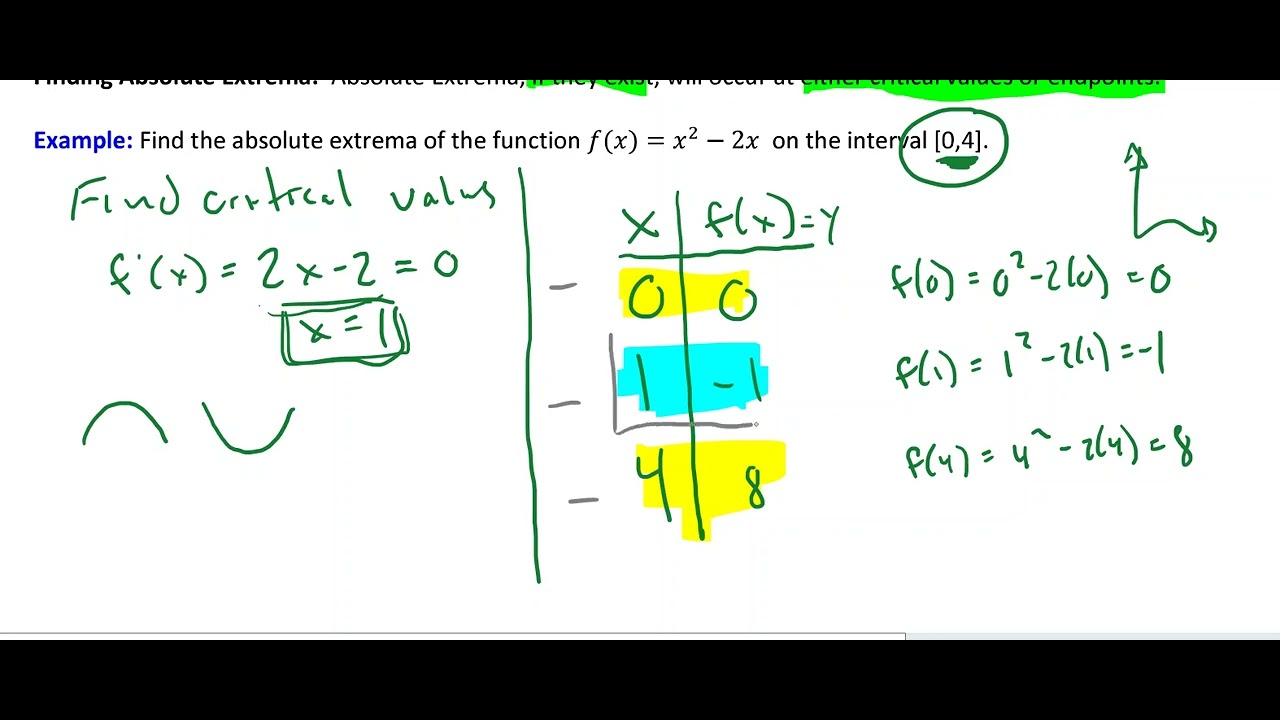
This paragraph discusses the application of derivatives in finding absolute minimum and maximum values of a function within a given interval. The process involves identifying critical numbers where the derivative is zero and evaluating the function at these points as well as at the endpoints of the interval. The speaker demonstrates this by finding the absolute minimum and maximum values of a quadratic function, setting the derivative equal to zero to solve for critical points, and evaluating the function at these points and the interval endpoints. The minimum and maximum values are then determined by comparing these evaluations.

05:00
🔍 Derivative Test for Critical Points and Absolute Extrema

The speaker explains the process of finding absolute extrema without necessarily classifying critical points as relative minima or maxima using the first derivative test. Instead, the focus is on evaluating the function at endpoints and where the derivative equals zero. The example involves a function with a product and chain rule derivative, which is set to zero to find critical points. The speaker simplifies the equation to find potential values for x, evaluates the function at these points and the interval endpoints, and determines the absolute minimum and maximum values. The paragraph also touches on the limitations of using the first derivative test when the problem requires finding both minimum and maximum values.

10:03
📉 Evaluating Functions at Critical Points for Extrema

In this paragraph, the speaker continues the discussion on finding absolute extrema by evaluating a function at critical points and endpoints. The example given is a function involving a square root and a squared term. The derivative is computed and set to zero to find critical points. The equation is manipulated to isolate terms and solve for x, yielding potential values. The function is then evaluated at these critical points and the interval endpoints to determine the minimum and maximum values. The speaker emphasizes the importance of considering the domain restrictions when identifying endpoints and critical points.

Mindmap
Keywords
💡Derivatives
Derivatives in calculus are a measure of how a function changes as its input changes. They are used to find the rate of change of a quantity and are central to the theme of the video, which focuses on applications of derivatives. In the script, derivatives are used to find absolute and relative extrema (minimums and maximums) of functions, which is a key application in calculus.
💡First Derivative Test
The First Derivative Test is a method used to determine the local extrema of a function by analyzing the sign changes of its first derivative. The video script explains that this test is used to find where the first derivative is zero, which can indicate potential maximum or minimum points of a function.
💡Absolute Minimum/Maximum
An absolute minimum or maximum refers to the lowest or highest value that a function attains over its entire domain. The script discusses finding the absolute minimum and maximum values of a function within a given interval, which involves evaluating the function at critical points and endpoints.
💡Relative Minimum/Maximum
A relative minimum or maximum is a point where the function's value is less than or greater than the values of the function in its immediate vicinity. The script mentions these terms in the context of finding local extrema, although it does not delve into the formal first derivative test to classify them.
💡Critical Numbers
Critical numbers are the values of the independent variable (usually x) that make the derivative of a function equal to zero or undefined. In the script, the presenter explains that to find extrema, one must look for critical numbers and evaluate the function at these points, as well as at the endpoints of the interval.
💡Endpoints
Endpoints refer to the starting and ending values of an interval on the x-axis. The script emphasizes the importance of evaluating the function at the endpoints of an interval when searching for absolute extrema, as these can sometimes be the points of minimum or maximum values.
💡Interval
An interval is a continuous subset of numbers on the real number line. In the context of the video, the interval defines the range over which the extrema of a function are sought. The script discusses finding minimum and maximum values within specific intervals.
💡Sine Function
The sine function is a trigonometric function that describes a smooth, periodic oscillation. The script uses the sine function as an example to illustrate how to find the extrema of a function involving trigonometric expressions.
💡Cosine Function
The cosine function, like the sine function, is a trigonometric function that represents a periodic wave. In the script, the derivative of the sine function (which is the cosine function) is used to find critical numbers where the derivative is zero.
💡Power Rule
The Power Rule is a basic differentiation rule that states the derivative of x^n (where n is a constant) is n*x^(n-1). The script applies the power rule when finding the derivative of a function that involves x squared, as part of the process to find its extrema.
💡Product and Chain Rules
The Product Rule and Chain Rule are differentiation techniques used when dealing with the derivatives of products of functions and compositions of functions, respectively. The script mentions applying these rules when finding the derivative of a function that is a product of x squared and another function, to determine its critical points.
Highlights

Applications of derivatives are discussed, focusing on finding absolute or relative minimums and maximums.

The First Derivative Test is introduced as a method to find minimums and maximums.

Critical numbers where the first derivative is zero are important for finding minimums and maximums.

Endpoints of an interval are also considered when looking for minimums and maximums.

Absolute minimum and maximum values of a function within an interval are calculated using critical numbers and endpoints.

An example function is given to demonstrate finding the absolute minimum and maximum values.

The process of evaluating the function at critical numbers and endpoints is explained.

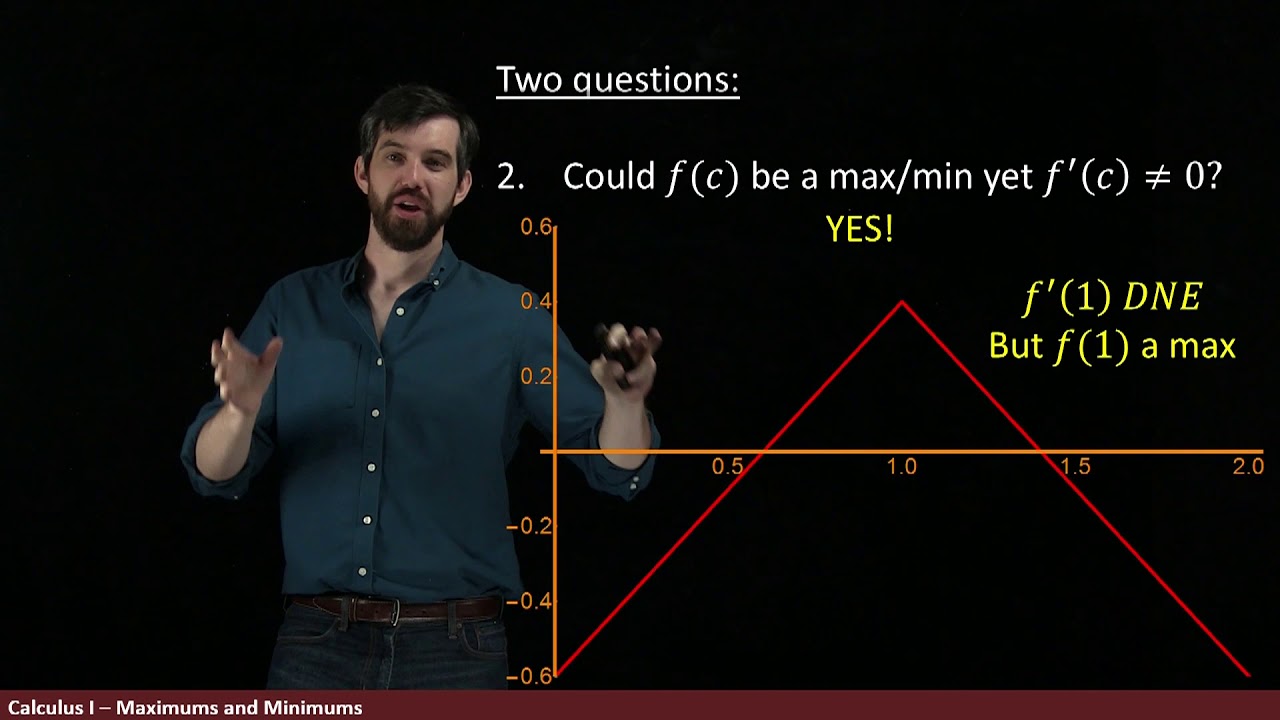
The concept of local minimum and maximum is discussed with an example.

Endpoints are not necessarily relative minimums or maximums unless specified.

A step-by-step approach to finding absolute minimums and maximums is provided.

The derivative of sine function is explained, and its application in finding critical numbers is shown.

The importance of considering the interval when finding critical numbers is highlighted.

An example of finding the maximum and minimum values of a trigonometric function is given.

The First Derivative Test is mentioned as an alternative method to classify relative minimums and maximums.

The process of finding critical numbers by setting the derivative equal to zero is demonstrated.

The importance of understanding the domain restrictions when finding critical numbers is discussed.

An example of finding absolute maximums and minimums for a function with a complex derivative is provided.

The transcript concludes with an invitation to visit the speaker's website for more examples and resources.

Transcripts

Browse More Related Video

3.4 - Using Derivatives to Find Absolute Max and Min Values

Candidates Test with Definite Integrals

Relative and Absolute Maximums and Minimums | Part II

Finding Absolute Extrema (Max/Min) on a Closed Interval [a, b]

Calculus Review Maximum and Minimum Values of Functions

Finding Absolute Extrema

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
DerivativesFirst Derivative TestAbsolute ExtremaRelative ExtremaCritical NumbersInterval AnalysisCalculus TutorialMaxima MinimaFunction AnalysisMathematics Education