AP Calc AB & BC Practice MC Review Problems #6
TLDRThis video script is a comprehensive review session focusing on multiple-choice questions, presumably for an AP Calculus exam. The presenter covers a range of topics, starting with the differentiability and continuity of functions, moving on to the application of formulas for equilateral triangles, and discussing rates of change. The script continues with the analysis of horizontal and vertical asymptotes, Riemann sums, average values of functions, related rates, limits, and solving differential equations. Each concept is methodically explained with step-by-step solutions, making it an invaluable resource for students preparing for AP Calculus exams. The presenter emphasizes the importance of understanding the underlying mathematical principles and applying them to solve a variety of problems. The summary of the video aims to provide a clear and concise overview of the topics covered, highlighting the educational value of the content for those studying calculus.
Takeaways
- 📚 The video is a review session for AP calculus multiple-choice questions, not from actual AP exams but based on previous sections.
- 🔗 The problems covered are relevant for AP AB, BC, and the review sets are linked in a playlist below the video.
- 📈 For the first question, understanding the graph of the derivative allows determining that the function F is differentiable on the interval (-2, 4).
- 🧮 Memorizing formulas, such as the area of an equilateral triangle, can be beneficial for solving related rate problems.
- 🏞️ The concept of asymptotes and domain are crucial for functions, especially when dealing with rational functions.
- 🤔 The video emphasizes the importance of limits and how they can differ when approaching positive or negative infinity.
- 🏛️ Riemann sums are used to approximate definite integrals, and the limit as the number of intervals approaches infinity can define the integral's value.
- 🚧 When dealing with related rates, it's essential to differentiate every term in the equation with respect to time.
- 🔑 The average value of a function over an interval can be found by dividing the integral of the function over the interval by the length of the interval.
- ⚖️ For inverse functions, the value of one function at a point can be used to find the derivative of the inverse at the corresponding point.
- 🧬 Solving differential equations often involves separating variables and integrating both sides of the equation.
- 📉 Finding the area between curves involves setting up integrals that account for where the curves intersect and which function is above the other over a given interval.
Q & A
What is the significance of the derivative graph in the first question of the video?
-The derivative graph in the first question indicates the slope of the function f at various points. From the graph, it can be determined that the function f is differentiable on the interval from negative 2 to 4, as the derivative exists and is continuous over this interval.
Why is it important to consider the domain when discussing vertical asymptotes?
-The domain of a function determines the set of all possible input values (x-values) for which the function is defined. A vertical asymptote occurs where the function is not defined, typically where the denominator of a rational function is zero. However, if a value that would make the denominator zero is not included in the domain, then there is no vertical asymptote at that point.
How does the concept of limits apply to finding horizontal asymptotes?
-Horizontal asymptotes are horizontal lines that a function approaches as the input (x-value) approaches positive or negative infinity. To find the equation of a horizontal asymptote, one can take the limit of the function as x approaches positive or negative infinity. If the limit results in a constant, this constant represents the y-value of the horizontal asymptote.
What is the relationship between the average value of a function and its integral over an interval?
-The average value of a function F on an interval [a, b] is given by the definite integral of F over the interval divided by the length of the interval (b - a). This represents the mean value of the function over the interval and can be found by integrating the function and then dividing by the interval length.
How does one determine the rate at which the height of an equilateral triangle increases given the rate of change of its area?
-To determine the rate at which the height of an equilateral triangle increases, one must first know the relationship between the area, base, and height of the triangle. The rate of change of the height (dh/dt) can be found by differentiating the area formula with respect to time and then using the given rate of change of the area (da/dt) and the base length to solve for dh/dt.
What is the role of L'Hôpital's Rule in evaluating limits of the form 0/0 or ∞/∞?
-L'Hôpital's Rule provides a method for finding the limit of a function when it results in an indeterminate form such as 0/0 or ∞/∞. The rule states that if the limit of the ratio of two functions is indeterminate, one can take the limit of the ratio of their derivatives instead. This process may need to be repeated multiple times until a determinate limit is found.
How can one solve a related rates problem involving two changing variables?
-To solve a related rates problem, one must establish an equation relating the two variables and their rates of change. Then, using given information (such as one of the rates), the equation can be solved for the unknown rate. This often involves applying the chain rule to differentiate the equation with respect to time.
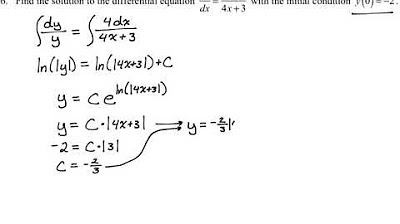
What is the general approach to solving separable differential equations?
-A separable differential equation can be solved by separating the variables, meaning each variable is isolated on one side of the equation. After separating the variables, both sides of the equation are integrated with respect to their respective variables, which often leads to the solution in terms of a function of the independent variable.
How does one find the total area between two curves on a given interval?
-To find the total area between two curves, one must first determine where the curves intersect within the interval, as this may affect the order of the curves (which one is above the other). Then, the area is found by integrating the upper curve minus the lower curve from one intersection point to the other. If the curves switch positions within the interval, separate integrals are calculated for each region and then summed to find the total area.
What is the significance of the initial condition in a differential equation?
-The initial condition provides a starting point or a specific value that the solution of a differential equation must satisfy. It is crucial for determining the unique solution to the differential equation, as without it, there may be multiple solutions that fit the equation.
How does one find the inverse function of a given function and its derivative at a specific point?
-If an inverse function G of a function F is given, along with a point on G, one can find the corresponding point on F and then calculate the derivative of F at that point. The derivative of G at the given point will be the reciprocal of the derivative of F, due to the properties of inverse functions.
Outlines
😀 AP Calculus BC Review: Set 6 Introduction
The video begins with an introduction to another set of multiple-choice review problems for AP Calculus BC, which are not from actual AP exams but are based on previous sections. The presenter emphasizes that the content is relevant for both AB and BC exams and provides a link to other sets in a playlist. The first question involves the graph of a derivative, F prime, and the presenter discusses the implications of the graph on the differentiability and continuity of the function F.
📐 Equilateral Triangle Area and Rate of Change
The second paragraph focuses on a problem involving an equilateral triangle with a given area formula. The presenter calculates the rate at which the height of the triangle is increasing per second when the area is 36√3 cm². By using the area formula and the rate of change of the area, the presenter determines the height of the triangle and its rate of change, resulting in a rate of 4/3 cm per second.
📈 Horizontal and Vertical Asymptotes of a Rational Function
The third paragraph deals with determining the number of horizontal and vertical asymptotes of a given function. The presenter clarifies that the function is not a rational function and discusses the domain of the function. By analyzing the behavior of the function as x approaches infinity and negative infinity, the presenter identifies two horizontal asymptotes and confirms the presence of a vertical asymptote at x = -2.
🧮 Riemann Sums and Integral Approximations
In the fourth paragraph, the presenter tackles a problem involving the right Riemann sum approximation of an integral. The presenter uses the limit as n approaches infinity to find the value of the integral, simplifying the expression by comparing the degrees of the numerator and denominator and then taking the ratio of the coefficients to find the final answer.
🔢 Average Value of a Function and Related Rates
The fifth paragraph discusses the calculation of the average value of a function F(x) on the interval from 1 to 5. The presenter also solves a related rates problem involving variables M and R changing with respect to time T. By using the given relationship between M and R and the given rate of change of M, the presenter finds the rate of change of R, dr/dt.
📉 Limits and Differential Equations
The sixth paragraph covers solving a limit as x approaches negative infinity involving exponential functions and a differential equation of the form dy/dx = 4xy/(x² - 1). The presenter uses separation of variables to solve the differential equation and finds the particular solution that passes through the point (0, 3). The solution involves integrating both sides of the equation and applying initial conditions to find the constant of integration.
🧩 Area Between Curves and Final Thoughts
The final paragraph addresses the problem of finding the total area between two curves, y = 3x² - 6x and y = 6x, from x = 2 to x = 5. The presenter identifies the points of intersection and determines that the curves switch positions within the interval. By calculating two separate integrals for the regions where each curve is on top and adding the results, the presenter finds the total area to be 23. The video concludes with a wish for the viewers to find the problems helpful and good luck.
Mindmap
Keywords
💡Multiple-choice review
💡Derivative
💡Equilateral triangle
💡Asymptote
💡Riemann sum
💡Average value
💡Related rates
💡Limit
💡Inverse functions
💡Separable differential equation
💡Definite integral
Highlights
The video provides a review of multiple-choice questions based on previous AP exams, not actual AP exam problems.
The problems are relevant for AP Calculus AB, BC, and those sections are available in a linked playlist.
The first question discusses the differentiability of a function based on its derivative's graph.
It is established that if a function is differentiable, it is also continuous.
An equilateral triangle's area formula is given, and a related rate problem is solved involving the triangle's height.
The rate of change of the triangle's height is calculated using the area formula and given rate of area change.
A function's horizontal and vertical asymptotes are determined by examining its form and limits.
The domain of the function is identified to exclude values that would result in division by zero.
The Riemann sum approximation of an integral is discussed, with a focus on taking the limit as the number of intervals approaches infinity.
The average value of a function over an interval is calculated using integration and the interval length.
A related rates problem involves finding the rate of change of one variable given the rate of change of another and their relationship.
The limit of a function as x approaches negative infinity is found by analyzing the behavior of exponential functions.
The value of an inverse function's derivative is determined using the derivative of the original function and the relationship between inverse functions.
A separable differential equation is solved using integration and considering the initial conditions to find the particular solution.
The total area between two curves is calculated by finding where the curves intersect and integrating over the respective intervals.
The domain of the solution to a differential equation is identified based on the initial condition and the function's behavior.
The video concludes with a reminder that understanding the progression of difficulty in AP exam questions can vary based on individual strengths.
Transcripts
Browse More Related Video
AP Calculus BC Practice Exam 2012 - Calculator Multiple Choice questions 76-92
AP Calculus AB 2008 Multiple Choice (Calculator) - Questions 76-92
AP Calculus Practice Exam Part 3 (MC #21-30)
AP Calculus AB Review

AP Calculus BC 2003 Multiple Choice (calculator) - Questions 76 - 92
AP Calc AB & BC Practice MC Review Problems #5
5.0 / 5 (0 votes)
Thanks for rating: