AP Calc AB & BC Practice MC Review Problems #5
TLDRThe video script is an educational walkthrough for AP Calculus students, focusing on multiple-choice questions. It covers a variety of calculus topics including slope fields, integrals, discontinuities, differential equations, and limits. The speaker uses a methodical approach to solve problems, often eliminating incorrect answer choices through logical deduction and mathematical reasoning. The script emphasizes the importance of understanding the underlying concepts rather than relying on memorization of formulas. It also provides strategies for tackling different types of calculus problems, such as using substitution for integrals and applying L'Hôpital's rule for indeterminate forms. The speaker encourages students to practice solving problems on their own before comparing their solutions with the ones provided in the video. The summary serves as a concise and engaging overview of the script's content, aimed at sparking interest in viewers and highlighting the educational value of the video.
Takeaways
- 😀 The video covers multiple-choice review for AP Calculus AB and BC, focusing on slope fields, integrals, differential equations, and limits.
- 😎 Slope fields are matched to differential equations, with a systematic approach of identifying zero slopes and testing points in different quadrants.
- 🤓 Integrals are tackled using substitution, with attention to detail on replacing variables and adjusting bounds.
- 🧠 Factoring is used to identify removable discontinuities in functions.
- 📊 Analysis of differential equations involves confirming solutions through inspection of derivatives.
- 📈 Understanding critical points in functions helps determine their behavior and characteristics.
- 📚 Continuous functions are analyzed for critical points and average values to solve related problems.
- 🔍 Volume calculations of solids with known cross-sections involve sketching, determining bounds, and setting up integrals.
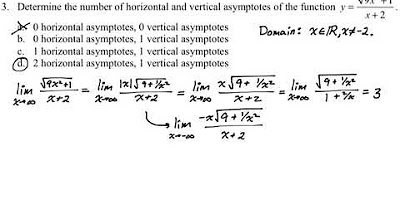
- 👨🏫 L'Hopital's rule is employed to evaluate limits of functions as X approaches infinity, with attention to detail on the application of the rule.
- 💡 Simplification techniques are used to expedite limit calculations, leveraging properties of logarithms and exponential functions.
Q & A
What is the main focus of the video?
-The video focuses on reviewing multiple-choice questions for AP Calculus AB & BC, providing solutions and explanations for each problem.
Why are the problems in the video not actual AP exam questions?
-The problems are not actual AP exam questions because they are based on questions that have appeared on past multiple-choice exams, and the presenter created them for practice purposes.
What is the strategy for solving the first differential equation problem in the video?
-The strategy involves looking at zero slopes first, then eliminating options that do not match the given slope field, and finally focusing on a quadrant to determine the correct differential equation.
How is the integral involving the substitution u = 2x - 3 transformed?
-The integral is transformed by substituting u = 2x - 3, which means du/2 = dx. The limits of integration are changed from x values to u values, and the integrand is adjusted accordingly.
What is a removable discontinuity?
-A removable discontinuity, also known as a hole, is a type of discontinuity where the function is not defined at a certain point, but the point can be defined in such a way that the function becomes continuous.
How does the presenter approach the problem involving the function f(x) with a removable discontinuity?
-The presenter factors the function to find the values of x that could potentially cause a removable discontinuity, and identifies that x = -4 is the value where the discontinuity occurs.
What is a separable differential equation?
-A separable differential equation is a type of ordinary differential equation that can be written in the form dy/dx = f(y)/g(x), which can be rearranged so that all the y terms are on one side and all the x terms are on the other, allowing for easy integration.
How does the presenter solve the differential equation with the initial condition y(0) = -2?
-The presenter separates the variables, integrates both sides, applies the initial condition to find the constant C, and then determines the domain of the solution based on the behavior of the differential equation.
What is the method used to find the volume of the solid with known cross-sections?
-The method involves setting up an integral that represents the volume of the solid, using the cross-sectional areas perpendicular to the y-axis and the height of each cross-section, and then integrating over the given interval.
How does the presenter approach the problem of finding the average value of a continuous function on an interval?
-The presenter uses the average value formula, which is the integral of the function over the interval divided by the length of the interval, and applies it to find the integral needed for the given problem.
What is the quotient rule used for in the video?
-The quotient rule is used to find the derivative of a function that is the ratio of two other functions, as seen when calculating H'(3) where H is the quotient of functions F and G.
How does the presenter handle the limit problem as x approaches infinity?
-The presenter uses L'Hôpital's rule multiple times to evaluate the limit, as the direct substitution results in an indeterminate form of infinity over infinity.
Outlines
📚 AP Calculus AB & BC Review: Slope Fields and Differential Equations
The video begins with an introduction to a review session for AP Calculus AB & BC, emphasizing the importance of understanding both AB and BC material for the BC exam. The presenter discusses that the problems are not actual AP questions but are inspired by past exam questions. The first problem involves identifying a differential equation from a given slope field, using zero slopes and test points to systematically eliminate incorrect answer choices.
🔍 Substitution and Integrals: U-Substitution Technique
The second paragraph delves into the technique of u-substitution for evaluating integrals. The presenter uses the example of integrating x over the square root of (2x - 3) from 2 to 4. The process involves substituting u = 2x - 3, adjusting the integral's bounds, and transforming the integrand to involve u, ultimately simplifying the integral for easier computation.
🔺 Discontinuities and Factoring: Removable Discontinuities in Functions
The third paragraph addresses the concept of removable discontinuities in the context of a given function. The presenter explains how to identify points of discontinuity by factoring the function's expression and looking for factors that can be canceled out, which would indicate a removable discontinuity or 'hole' in the function.
📈 Critical Points and Function Behavior: Determining Function Characteristics
In the fourth paragraph, the discussion shifts to the behavior of a continuous function with two critical points on a specified interval. The presenter navigates through various assertions about the function, leveraging the information that the function is continuous but not necessarily differentiable. The goal is to deduce which statements must be true based on the function's critical points.
🧮 Differential Equations: Solving Separable Equations with Initial Conditions
The fifth paragraph focuses on solving a separable differential equation given an initial condition. The presenter transforms the differential equation and applies integration to find the general solution. Then, using the initial condition, a specific solution is determined. The importance of considering the domain of the solution is highlighted, particularly when dealing with discontinuities.
🏗 Volume Calculations: Solids of Known Cross-Sections
The sixth paragraph introduces a method for calculating the volume of a solid with known cross-sectional shapes. The presenter outlines the process of setting up an integral that represents the volume of the solid formed by the region between the curves y = x^4 and y = 8x. The approach involves finding the intersection points of the curves and expressing the volume as an integral that multiplies the base and height of the cross-sections.
🔢 Average Value and Definite Integrals: Applying Average Value to Find an Integral
The seventh paragraph discusses the concept of the average value of a function and how it can be used to find a definite integral. The presenter uses the average value from a given interval to find the integral over a different interval, employing a u-substitution technique to simplify the calculation.
📉 Quotient Rule and Derivatives: Finding the Derivative of a Ratio of Functions
The eighth paragraph applies the quotient rule for derivatives to find H'(3), where H is the ratio of two functions F and G. The presenter uses the graph of the functions to identify the necessary values and applies the quotient rule formula to compute the derivative at the specified point.
🏞 L'Hôpital's Rule and Limits: Evaluating Limits as X Approaches Infinity
The final paragraph deals with the application of L'Hôpital's rule to evaluate a limit as X approaches infinity. The presenter demonstrates the correct notation and method for applying L'Hôpital's rule iteratively to resolve an indeterminate form of infinity over infinity. The video concludes with a reflection on the challenges of the problems and well wishes for the viewers.
Mindmap
Keywords
💡Multiple-choice review
💡Slope field
💡Substitution
💡Removable discontinuity
💡Differential equation
💡Critical points
💡Separable differential equation
💡Volume with known cross-sections
💡Average value
💡Quotient rule
💡L'Hôpital's rule
Highlights
The video provides a multiple-choice review for AP Calculus AB & BC, covering all topics from AB plus additional BC material.
The problems are not actual AP questions but are based on past exam questions.
Answer choices are not provided for most problems to encourage solving them step-by-step.
Links to the problems and other problem sets are provided in the video description.
The first question involves matching a given slope field to the correct differential equation.
An organized approach is demonstrated by first looking at points with zero slopes.
The next problem involves a u-substitution technique for evaluating a definite integral.
The integral is transformed by substituting u = 2x - 3 and adjusting the bounds accordingly.
The third question examines identifying a removable discontinuity in a rational function.
Factoring is used to find the x-value that results in a removable discontinuity.
The fourth problem deals with verifying a proposed solution to a second-order homogeneous differential equation.
By analyzing the derivatives of the proposed solutions, the correct answer is determined to be C.
The fifth question asks about the properties of a continuous function with two critical points on a given interval.
The function's behavior between selected data points is considered to deduce what must be true.
The sixth problem involves solving a separable differential equation with an initial condition.
The domain of the solution is restricted based on the original differential equation's discontinuity.
The seventh question is about finding the volume of a solid with known cross-sectional shapes.
The volume is calculated by integrating the product of the base and height of the rectangular cross-sections.
The eighth problem involves finding the average value of a function on a given interval.
The average value is used to evaluate an integral over a different interval using substitution.
The final question examines finding the derivative of a quotient of two functions using the quotient rule.
Values of the functions and their derivatives at a specific point are used to calculate the derivative.
The video concludes with a limit problem involving L'Hopital's rule, which is solved by repeated differentiation.
An alternative approach is suggested by approximating the expression using properties of logarithms.
Transcripts
Browse More Related Video

AP Calculus BC 2008 Multiple Choice (no calculator) - questions 1 - 28
AP Calc AB & BC Practice MC Review Problems #6
AP Calculus AB 2008 Multiple Choice (No Calculator)
AP Calc AB & BC Practice MC Review Problems #3
AP Calculus AB/BC Multiple Choice Practice Test (2012 AP CED Problems)

AP Calculus Multiple Choice Practice Test (2020 AP CED Problems)
5.0 / 5 (0 votes)
Thanks for rating: