AP Calculus AB 2008 Multiple Choice (Calculator) - Questions 76-92
TLDRThe video script is a comprehensive review of the AP Calculus AB 2008 multiple-choice calculator section, guiding viewers through various calculus problems. The presenter, Vincent, demonstrates how to approach each question efficiently, emphasizing the strategic use of a calculator to save time. The review covers topics such as identifying intervals where a function is increasing by analyzing the derivative, evaluating limits, applying the Fundamental Theorem of Calculus to find antiderivatives and definite integrals, and using related rates for real-world problems. Vincent also addresses the concept of points of inflection, average function values, and population density as a function of distance from a river. The script is an invaluable resource for students preparing for the AP Calculus exam, providing clear explanations and step-by-step solutions to complex problems.
Takeaways
- 📈 When analyzing a graph of a derivative to determine where a function is increasing, look for intervals where the graph is above the x-axis, indicating f'(x) > 0.
- 🔍 For piecewise functions, evaluate the limits from both the left and right to determine the existence and value of the function at certain points.
- 🧮 Use a calculator to find the derivative of a function and adjust the viewing window to better identify intervals where the derivative is positive or negative.
- 🏞️ To find the area between two curves, determine the points of intersection and then integrate the top function minus the bottom function over the specified intervals.
- 📌 Points of inflection can be found by taking the second derivative of a function and looking for sign changes, which can be quickly graphed using a calculator.
- ∫ For definite integrals, use the Fundamental Theorem of Calculus, which relates the integral of a function to its antiderivative.
- 🚀 When dealing with motion or rate problems, integrate the velocity function over the given time interval to find the position function.
- 🔄 Related rates problems require setting up an equation using the given rates and solving for the unknown rate of change.
- 📉 If a function's second derivative is always negative, the function is concave down, and its slope is decreasing.
- 🏗️ For population density problems, integrate the density function over the area to find the total population.
- 🤔 Always consider the implications of limits, derivatives, and integrals in the context of the problem to avoid common mistakes.
- 🧐 When a question seems tricky, carefully analyze the given information and use it to eliminate incorrect answer choices.
Q & A
What is the main focus of the video?
-The video is a review of the AP Calculus AB 2008 multiple-choice calculator section, guiding viewers through solving various calculus problems using a calculator.
Why might using a calculator for every question in the calculator section slow you down?
-Some questions can be solved mentally or with simpler methods, and using a calculator for every question might consume more time than necessary, thus slowing down the overall process.
What is the significance of the derivative being greater than zero in the context of the video?
-When the derivative of a function is greater than zero, it indicates that the function is increasing on that interval. This is important for determining where a function has a positive slope.
What is the purpose of the tracing feature on a calculator?
-The tracing feature allows for the identification of exact points where a function intersects with another function or the x-axis, which is useful for finding roots or points of intersection.
How does the video approach the question involving the integral from negative 5 to 5?
-The video suggests finding the area of the function from negative 5 to 2 and then adding it to the area from 2 to 5, using the given integrals to simplify the process.
What is the concept used to find the value of G of 4 in the context of the antiderivative?
-The video uses the Fundamental Theorem of Calculus, which allows for the evaluation of the definite integral by finding the antiderivative of the function and then applying the limits of integration.
Why is it important to find the second derivative to determine points of inflection?
-Points of inflection occur where the concavity of a function changes. The second derivative, being the derivative of the first derivative, indicates changes in concavity, thus helping to identify points of inflection.
How does the video approach the problem of finding the acceleration of a particle at a specific time?
-The video uses the concept that acceleration is the derivative of velocity. By finding the derivative of the given velocity function and evaluating it at the specific time, the acceleration is determined.
What is the method used in the video to find the area between two curves?
-The video demonstrates how to find the points of intersection between the two curves and then evaluates the integral of the top function minus the bottom function over the given intervals to find the area between the curves.
Why is it suggested to not use the calculator for every question in the calculator section of the AP Calculus exam?
-The suggestion is to avoid over-reliance on the calculator for simpler questions to save time and focus on problems where the calculator's capabilities can significantly streamline the solution process.
How does the video determine the value of x where the function F has a relative maximum?
-The video uses the first derivative test, looking for where the first derivative changes from positive to negative, which indicates a relative maximum.
What is the key to solving the related rates problem involving the radius of a sphere and its surface area?
-The key is to apply the chain rule to the formula for the surface area of a sphere (4πr^2) and then evaluate the expression at the given radius using the given rate of change of the radius.
Outlines
📈 AP Calculus Multiple-Choice: Understanding Increasing Functions and Graphs
Vincent introduces the AP Calculus AB 2008 multiple-choice section, focusing on question 76. He explains how to determine where a function F is increasing by looking at the derivative f'(x) and identifying where it's greater than zero. The discussion covers interpreting the graph of f'(x) to find intervals of increase, and the importance of not using a calculator for every question to avoid slowing down. A detailed analysis of a sloppy question and its answer choice B is provided, along with a general approach to tackling such problems efficiently.
🧮 Limits, Piecewise Functions, and Derivatives in AP Calculus
The summary delves into question 77, which involves a piecewise function and the evaluation of true statements about its behavior. Vincent explains the concept of limits from the left and right, and how they relate to the existence of a limit at a certain point. The importance of the limit being equal on both sides for its existence is highlighted. The summary also covers questions 78 and 80, where the focus is on finding intervals where a function is increasing using derivatives and a graphing calculator, and determining points of inflection by analyzing the second derivative.
🏛 FTC and Motion Problems: AP Calculus Solutions
Vincent tackles questions 81 and 82, which involve the Fundamental Theorem of Calculus (FTC) and a motion problem, respectively. For question 81, he demonstrates how to use FTC to find the value of an antiderivative at a specific point. In question 82, the focus is on finding the acceleration of a particle at a given time by taking the derivative of the velocity function. The use of a calculator to efficiently solve these problems is emphasized.
📉 Integrating Areas and Analyzing Tangent Lines in AP Calculus
The summary explains how to find the area between two curves for question 83 by identifying points of intersection and setting up integrals. For question 84, Vincent discusses the use of the first derivative test to find relative maxima and minima, highlighting how to interpret the graph of the derivative. The concept of horizontal tangent lines is clarified as a distraction in this context.
🧵 Rolle's Theorem and Continuous Functions in AP Calculus
Vincent explores question 85, which involves using Rolle's theorem to deduce that a function must not be differentiable at some point within a given interval if certain conditions are met. The explanation covers the three conditions of Rolle's theorem and how the non-existence of a value C, where the derivative equals zero, leads to the conclusion that the function is not differentiable at some point K within the interval.
📊 Analyzing Slope and Position Functions in AP Calculus
The summary for question 86 discusses how to determine which graph could represent a position function based on given velocity values. Vincent explains the process of elimination using the slope and function values at specific points. For question 87, he demonstrates how to find the position of an object at a given time using the integral of the velocity function and the initial position.
🌐 Related Rates and Average Function Values in AP Calculus
Vincent addresses a related rates problem in question 88, where the rate of change of the surface area of a sphere is calculated given the rate of change of the radius. The use of the chain rule and the importance of including the rate of change of the radius (dr/dt) are highlighted. For question 90, he explains how to analyze a table of values for a function that is twice differentiable, with a focus on the slope and concavity of the function.
🏙 Population Density and Definite Integrals in AP Calculus
The final summary covers question 92, which involves calculating the total population within a rectangular boundary of a city with a river on one side. Vincent explains how to use the concept of population density and definite integrals to find the total population by summing up the population slices along the river's edge.
Mindmap
Keywords
💡AP Calculus
💡Derivative
💡Limit
💡Integral
💡Fundamental Theorem of Calculus
💡Points of Inflection
💡Velocity and Position
💡
💡Related Rates
💡Rolle's Theorem
💡Average Rate of Change
💡Average Function Value
Highlights
Vincent guides through the AP Calculus AB 2008 multiple-choice calculator section starting with question 76.
For question 76, the focus is on identifying where the function F is increasing by analyzing the graph of its derivative f'(x).
It is emphasized not to use a calculator for every question to avoid slowing down, especially in the calculator section.
Question 77 involves a piecewise function, and the task is to determine which statements about its limits are true.
In question 78, the derivative of sin(x^3) - x is analyzed to find intervals where the function f(x) is increasing.
For question 79, the integral of f(x) from -5 to 5 is calculated using given integrals and the fundamental theorem of calculus.
Question 80 deals with finding the number of points of inflection for the function F by examining the second derivative.
In question 81, the value of G(4) is determined using the antiderivative G(x) and the fundamental theorem of calculus.
Question 82 requires finding the acceleration of a particle at time T=3 by taking the derivative of the velocity function.
For question 83, the area between two curves is calculated using the points of intersection and definite integrals.
Question 84 applies the first derivative test to find the value of x where F has a relative maximum, based on the graph of F'.
In question 85, the value of a definite integral is found using the continuity of f' and the fundamental theorem of calculus.
Question 86 involves identifying which graph could represent the position function based on a table of velocity values.
For question 88, related rates are used to determine the rate of change of the surface area of a sphere given the rate of change of its radius.
Question 89 explores Rolle's theorem and its conditions, leading to the conclusion that the function is not differentiable at some point within the interval.
In question 90, the table of values for a continuous and twice differentiable function is analyzed to determine which could represent the function F, considering the concavity and slope conditions.
Question 91 calculates the average function value of a given function on the interval from -1 to 3 using the average value formula.
Question 92 deals with a population density problem, where the population within a certain strip distance from a river is integrated to find the total population.
Transcripts
Browse More Related Video

AP Calculus BC 2003 Multiple Choice (calculator) - Questions 76 - 92
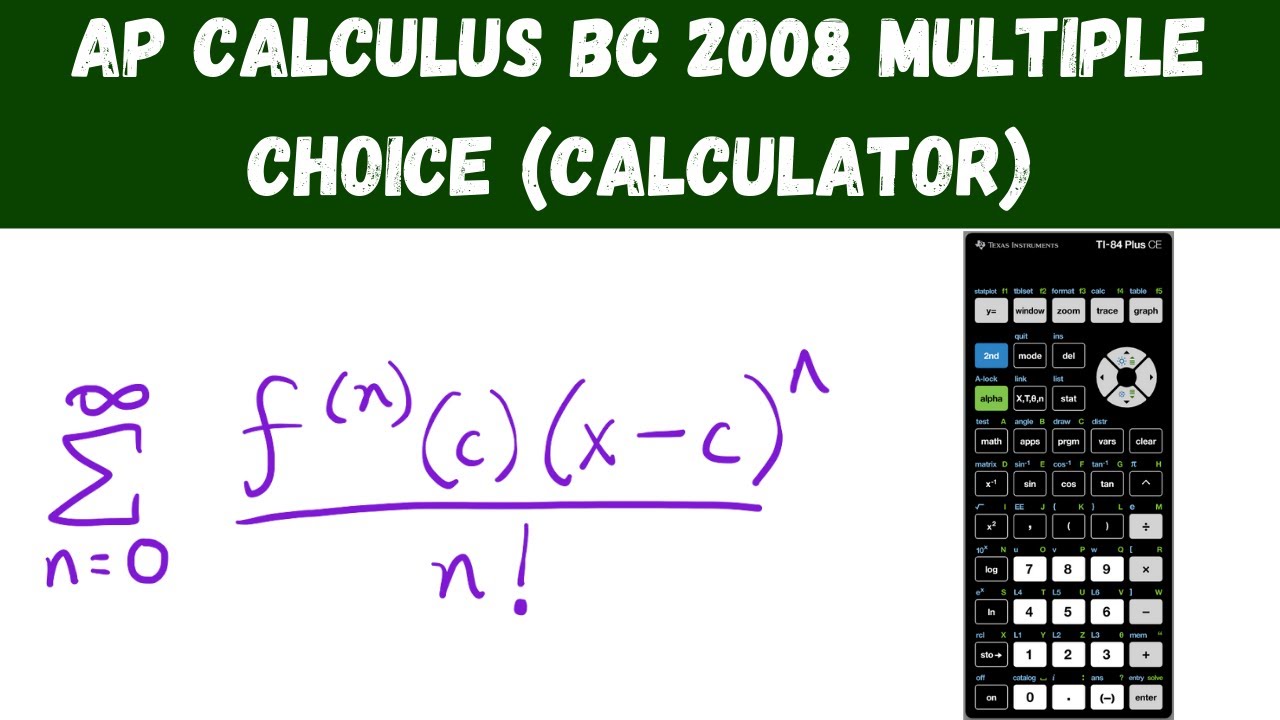
AP Calculus BC 2008 Multiple Choice (calculator) - Questions 76 - 92
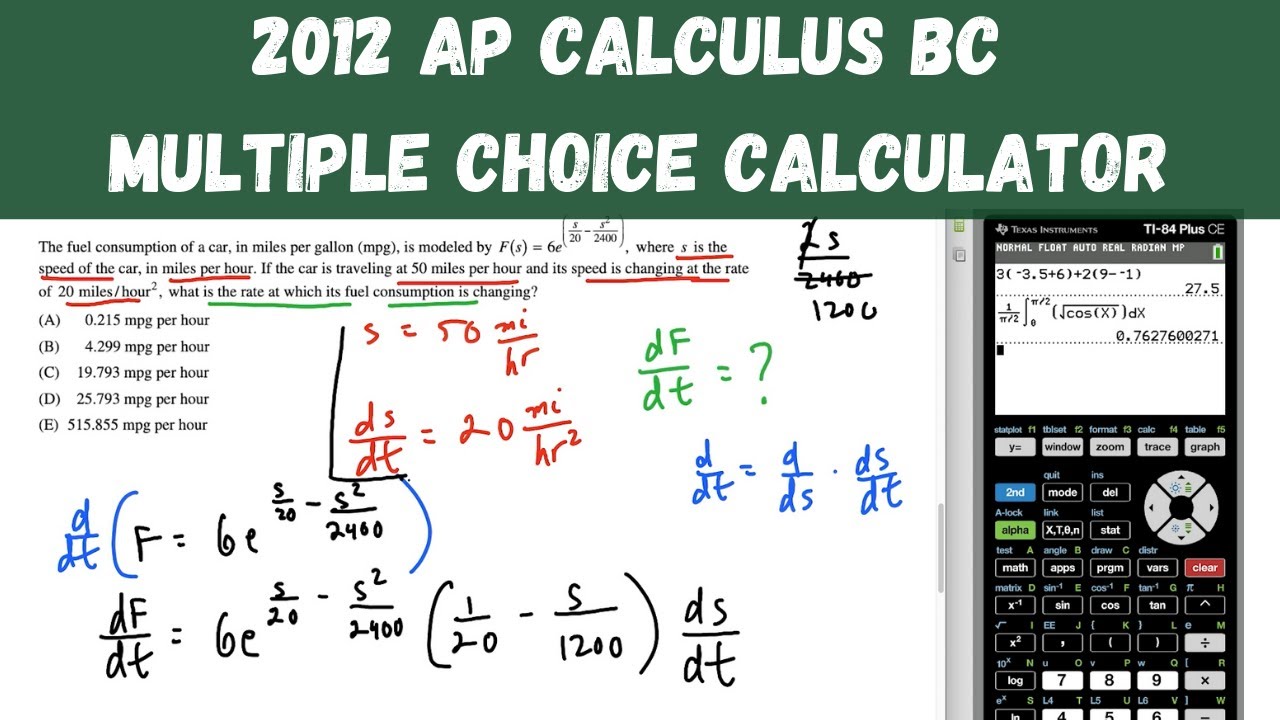
AP Calculus BC Practice Exam 2012 - Calculator Multiple Choice questions 76-92

AP Calculus AB 2012 Multiple Choice (calculator) - Questions 76 - 92
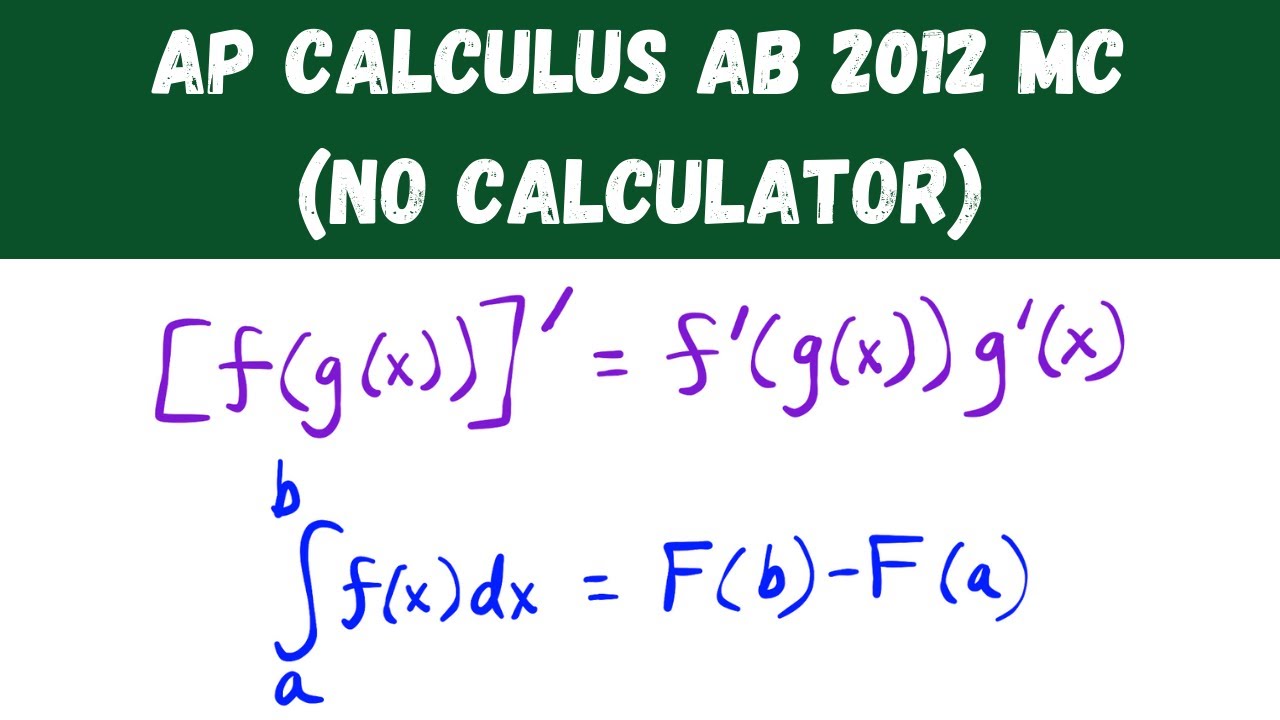
AP Calculus AB 2012 Multiple Choice (no calculator) - Questions 1-28
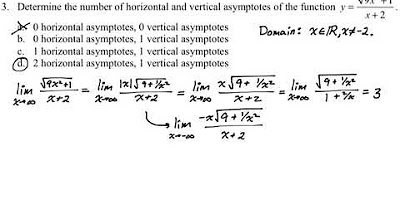
AP Calc AB & BC Practice MC Review Problems #6
5.0 / 5 (0 votes)
Thanks for rating: