8. Circuits and Magnetism I
TLDRThe video script is a comprehensive lecture on electromagnetism, delivered by a professor to his class. It begins with an exploration of electric circuits, explaining the role of electrostatic forces and the concept of electromotive force (EMF) through an analogy of a ski lift. The lecture progresses to the dynamics of resistors and capacitors in series and parallel, highlighting their combined effects on current and voltage. A key focus is on the behavior of capacitors charging and discharging, with an emphasis on the differential equations governing these processes. The script also delves into the energetics of the system, detailing the conservation of energy between the battery, capacitor, and resistor. Towards the end, the professor introduces magnetism, contrasting it with electrostatics by emphasizing its dependence on moving charges. The script concludes with a discussion on the fundamental principles of magnetostatics, including the Lorentz force and its implications for particle motion, as well as the magnetic effects of current-carrying wires. The lecture is rich with analogies, mathematical explanations, and experimental insights, aiming to provide a deep understanding of the principles of electromagnetism.
Takeaways
- 🔋 The concept of electromotive force (emf) is introduced as the work done by non-electrostatic forces, like the force inside a battery, which is not entirely explained by electrostatic force alone.
- ⚙️ An analogy of a ski lift is used to explain emf, where the ski lift represents the non-conservative force that does work against gravity, similar to how a battery does work in a circuit.
- 🔊 The importance of electrostatic forces is emphasized, as they are still crucial in circuits, even though they are conservative and do not do work when charges move in a closed loop.
- 🔌 The behavior of resistors and capacitors in series and parallel is discussed, highlighting that resistors add up in series and combine as the sum of their reciprocals in parallel, whereas capacitors combine as the sum of their values in both scenarios.
- ⚡ The force on a moving charge in a magnetic field, known as the Lorentz force, is introduced and explained to be the cause of magnetic effects, which are not covered by electrostatics.
- 🧲 The magnetic field produced by an electric current is described, and it is noted that a current-carrying coil behaves like a magnet with north and south poles, even in the absence of actual magnets.
- 🔵 The force on a current-carrying wire in a magnetic field is explained as the sum of the Lorentz forces on the individual charges moving within the wire.
- 💡 The cross product relationship v x B is used to determine the direction and magnitude of the force on a moving charge in a magnetic field, where v is the velocity of the charge and B is the magnetic field.
- 🔁 The work done by the magnetic field on a moving charge is always zero because the force is always perpendicular to the velocity, meaning the magnetic field does no work and does not change the kinetic energy of the charge.
- ⏫ The cyclotron, a device for accelerating charged particles using an alternating voltage and a magnetic field, is explained, demonstrating how particles can be accelerated to high energies without the need for a high voltage source.
- 📐 The force on a current-carrying loop in a magnetic field is calculated, showing that the forces on different segments of the loop can result in a net torque, causing the loop to align with the magnetic field.
Q & A
What is the primary difference between electrostatic force and the force inside a battery that causes current to flow in a circuit?
-The electrostatic force is due to charges on the plate of a capacitor and cannot do work for a charge that goes around in a full loop in an electrostatic field, where the net work done is zero. The force inside a battery, on the other hand, is non-conservative and is responsible for doing work as the charges go around the circuit, leading to heating in a resistor.
How does the ski lift analogy help explain the concept of emf (electromotive force) in a circuit?
-The ski lift analogy compares the work done by the ski lift against gravity to the work done by the emf in a circuit. Just as the ski lift carries skiers to the top of the slope against gravity, the emf in a circuit drives the current around the circuit against the electrostatic force, providing the energy needed to do work, such as heating a resistor.
Why is the line integral of the total force around a closed loop in a circuit not zero, even though electrostatics is conservative?
-The line integral of the total force is not zero because, in addition to the conservative electrostatic force, there is a non-conservative force (like the chemical force inside a battery) that does work on the charges as they move around the circuit. This non-conservative force allows the charges to continually move and do work, such as heating a resistor.
What is the relationship between the emf of a battery and the voltage difference between two points in a circuit?
-The emf of a battery is equal to the voltage difference between two points in a circuit when the circuit is operating under steady-state conditions. This voltage difference is the result of the line integral of the electrostatic force around a closed loop, minus the work done by the non-conservative force (like the chemical force in the battery).
How does the behavior of charges in a magnetic field differ from their behavior in an electrostatic field?
-In an electrostatic field, a stationary charge experiences no force, and like charges repel each other while opposite charges attract. In a magnetic field, however, a stationary charge experiences no force, but a moving charge experiences a force perpendicular to both its velocity and the magnetic field. This force can cause a charged particle to move in a circular path if the magnetic field is uniform.
What is the fundamental principle behind the operation of a cyclotron?
-A cyclotron operates on the principle that charged particles can be accelerated by an electric field while they are also constrained to a circular path by a magnetic field. The magnetic field's role is to keep the particles in a spiral path without doing work on them, while the electric field provides the force for acceleration. The frequency of the alternating current used to create the electric field is synchronized with the frequency of the particles' circular motion, allowing continuous acceleration.
How does the force on a current-carrying wire in a magnetic field relate to the force on individual charges within the wire?
-The force on a current-carrying wire in a magnetic field is the result of the collective forces on the individual charges moving within the wire. Each charge experiences a force due to the magnetic field, and when these forces are summed over a small segment of the wire (considering the current density and the magnetic field), they result in a net force on that segment, which can be calculated as the cross product of the current element and the magnetic field.
What is the significance of the direction of the magnetic field in determining the direction of the force on a moving charge?
-The direction of the magnetic field is crucial because it determines the direction of the force experienced by a moving charge according to the right-hand rule (or left-hand rule for negative charges). The force is perpendicular to both the velocity of the charge and the magnetic field, resulting in a circular or helical path for the charge, depending on the orientation of the field and the charge's motion.
Why does the magnetic field not do any work on a moving charge?
-The magnetic field does not do any work on a moving charge because the force exerted by the magnetic field on the charge is always perpendicular to the direction of the charge's velocity. Work is defined as the force along the direction of displacement, and since there is no component of the magnetic force in the direction of the charge's motion, no work is done, and thus the kinetic energy of the charge remains unchanged.
What is the role of the alternating current (AC) in the operation of a cyclotron?
-In a cyclotron, the alternating current (AC) is used to reverse the polarity of the electric field plates at the precise moment when the charged particle crosses the gap between them. This reversal ensures that the particle always experiences an accelerating force, allowing it to continuously gain speed as it spirals outward in a larger and larger circle within the magnetic field.
How does the magnetic moment of a current loop relate to the torque experienced by the loop in a magnetic field?
-The magnetic moment of a current loop, which is proportional to the product of the current and the area enclosed by the loop, acts like a dipole moment in a magnetic field. The torque experienced by the loop is given by the cross product of this magnetic moment (μ) and the magnetic field (B). The torque tends to align the magnetic moment of the loop with the direction of the external magnetic field.
Outlines
🔋 Understanding Circuits and EMF
The professor begins by revisiting circuits, emphasizing the complexity beneath the surface of a simple battery and resistor setup. They explain that while electrostatic force is involved, it's not the sole player in the circuit's operation. The work done in the circuit, such as heating a resistor, suggests another force at work within the battery. An analogy is drawn between a ski lift and a circuit, illustrating how the electromotive force (EMF) is akin to the mechanical work done against gravity by the ski lift. The concept of EMF is introduced as the line integral of forces around a loop, which, unlike electrostatic force, is non-zero due to non-conservative forces within the battery.
🔍 Analyzing Circuits with Resistors and EMF
The discussion continues with the exploration of simple circuits involving a single resistor and an electromotive force (EMF) source. The professor outlines the fundamental principles that govern these circuits, such as the conservation of current and the relationship between voltage, current, and resistance (Ohm's Law). They then extend the analysis to more complex circuits with multiple resistors in series and parallel, explaining how to calculate the equivalent resistance and the current flow through each resistor. The concept of emf as the voltage difference around a closed loop is also clarified.
🔌 Capacitors in Series and Parallel
The professor moves on to capacitors, explaining how they behave when placed in series and parallel. They describe the calculation of equivalent capacitance in both configurations, highlighting the difference in behavior compared to resistors. The analogy of a ski slope is used again to illustrate the concept of potential difference and energy storage in capacitors. The lecture also touches on the idea of energy conservation in a circuit involving a battery, resistor, and capacitor, setting the stage for a homework problem.
⚡ Exploring the Dynamics of Charging Capacitors
The focus shifts to the behavior of capacitors when they are charged in a circuit. The professor describes the process of charging a capacitor with a resistor and a battery, detailing the current flow and the resulting charge on the capacitor over time. A differential equation is derived to describe the charge as a function of time, leading to an exponential growth model for the charge on the capacitor. The final charge on the capacitor is shown to be dependent on the capacitance and the EMF of the battery. The lecture concludes with a student's question about the negative sign in the equation, which the professor clarifies.
🧮 The Energetics of Capacitors and Resistors
The professor delves into the energetics of the circuit involving a capacitor, a resistor, and a battery. They ask students to consider the initial and final states of the system to understand the energy transfer. The work done by the battery is shown to be equal to the energy stored in the capacitor and the energy dissipated in the resistor. The current's exponential decay with time is derived from the charge function, and the homework assignment is outlined, which involves calculating the energy given by the battery and ensuring it equals the sum of the energy stored and dissipated.
⚖️ Balancing Forces in Circuits with Resistors
The lecture concludes with a discussion on analyzing more complex circuits with multiple branches and resistors. The professor emphasizes the importance of identifying the number of unknowns and setting up the correct number of independent equations to solve the circuit. The technique of using loop equations based on voltage drops and Ohm's law is introduced. The professor also touches on the concept of reference frames and their relation to the observed phenomena in the circuit, hinting at the principles of relativity.
🧲 Introducing Magnetism and its Phenomena
The professor introduces magnetism as the next topic, starting with historical discoveries and moving on to describe new phenomena not covered by electrostatics. They discuss the forces observed around moving charges and magnets, such as the attraction or repulsion between a moving charge and a neutral wire. The concept of reference frames in relation to magnetism is also mentioned, with a promise to delve deeper into relativistic effects in future lectures.
🚄 Relativity and the Observer's Perspective on Magnetism
The professor explores the concept of magnetism from the perspective of a moving observer. They discuss the scenario where a charge in a moving train is attracted to a wire and use it to illustrate the principles of relativity. The key takeaway is that electromagnetism works for all observers in uniform motion, and the laws of physics remain the same. The lecture ends with a teaser for the fundamental equations of magnetostatics that will be covered in subsequent classes.
📐 Fundamental Equations of Magnetostatics
The professor presents the fundamental equations of magnetostatics, which describe the force on a moving charge in a magnetic field and how electric currents produce magnetic fields. The Lorentz force, given by the cross product of velocity and magnetic field, is introduced as a postulate based on experimental evidence. The professor also discusses the concept of work done by the magnetic field, highlighting that it always perpendicular to the motion of the particle and thus does no work. The lecture includes simple problems to illustrate the application of the v x B force.
🚀 Velocity Filtering and Particle Trapping
The lecture focuses on applications of magnetic fields in particle physics, specifically velocity filtering and particle trapping. The professor explains how a combination of electric and magnetic fields can be used to select particles with a specific velocity, creating a velocity filter. They also describe how a uniform magnetic field can be used to trap particles in a circular orbit, with the frequency of the orbit dependent only on the charge-to-mass ratio of the particle and the magnetic field's strength. The historical use of this principle in the invention of the cyclotron by Lawrence is also mentioned.
🔗 Force on a Current-Carrying Wire in a Magnetic Field
The professor discusses the force experienced by a small segment of a wire carrying current in a magnetic field. They derive the force as the product of current, the length of the wire segment, and the magnetic field, taking the cross product into account. The lecture explores scenarios where this force can be used to balance the weight of the wire or to distort a loop of current-carrying wire. The concept of torque on a current loop in a magnetic field is introduced, with the magnetic moment of the loop being analogous to a magnetic dipole moment.
🟩 Torque on a Magnetic Loop
The final topic of the lecture is the calculation of torque on a rectangular current loop in a magnetic field. The professor explains how the forces on different segments of the loop result in a net torque, which is equivalent to the magnetic moment of the loop (current times the area) cross the magnetic field. The magnetic moment is a vector quantity, and its interaction with the magnetic field is similar to that of a magnetic dipole in a field. The lecture concludes with a setup for the next class, where further exploration of this topic is expected.
Mindmap
Keywords
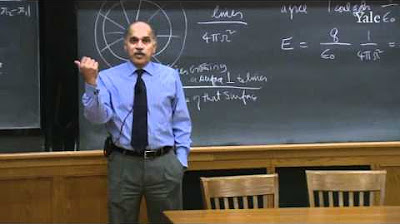
💡Electrostatic Force
💡Electromotive Force (EMF)
💡Circuit
💡Resistor
💡Voltage
💡Current
💡Capacitor
💡Magnetic Field
💡Lorentz Force
💡Coulomb's Law
💡Relativity
Highlights
The professor introduces the concept of a circuit with a battery and a resistor, emphasizing that the work done in the circuit is not solely due to electrostatic force.
An analogy of a ski slope is used to explain the non-conservative force inside a battery, which is likened to a ski lift carrying skiers to the top of the slope.
The line integral of the electrostatic field around a closed loop is zero, indicating that the work done by electrostatic force in a complete circuit is zero.
The concept of emf (electromotive force) is introduced as the work done per unit charge by non-electrostatic forces, which is analogous to the work done against gravity by a ski lift.
The voltage difference between two points in a circuit is equated to the emf, providing a simplified way to understand and calculate emf.
The professor discusses the behavior of resistors in series and parallel, highlighting that their combined effect can be modeled as a single equivalent resistor.
Capacitors in parallel and series are also discussed, showing that their combined behavior is the sum of their individual capacitances and the inverse of their combined inverses, respectively.
An interesting problem involving a capacitor, a resistor, and a switch is presented to illustrate the dynamic behavior of charging a capacitor.
The current through a charging capacitor is described as initially high and exponentially decreasing over time, reaching zero when the capacitor is fully charged.
The professor emphasizes the importance of solving differential equations to understand the behavior of physical systems, such as the charging of a capacitor.
The energy transfer during the charging process is discussed, ensuring that the work done by the battery equals the energy stored in the capacitor and the energy dissipated in the resistor.
The concept of magnetism is introduced, highlighting that it is a force caused by and felt by moving charges, which is a new phenomenon not covered by electrostatics.
The Lorentz force, which is the force experienced by a moving charge in a magnetic field, is introduced and explained through its cross product relationship with velocity and magnetic field.
The professor demonstrates that a magnetic field does no work on a moving charge, as the force is always perpendicular to the velocity.
The concept of a velocity filter using electric and magnetic fields is explained, showing how to select particles with a specific velocity from a beam.
The cyclotron, a particle accelerator, is described, which uses an alternating current and a magnetic field to accelerate particles to high energies without the need for a high voltage source.
The force on a current-carrying wire in a magnetic field is calculated, showing how the force can be used to balance the weight of the wire or cause it to move.
The magnetic moment of a current loop is introduced, which is a vector quantity that describes the force and torque experienced by the loop in a magnetic field.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: