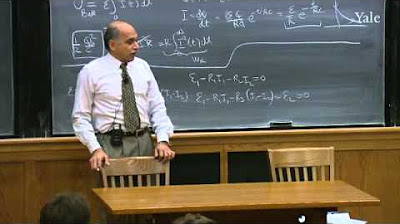7. Resistance
TLDRThe transcript is a detailed lecture on electrostatics and electrical circuits, delivered by a professor to his students. It covers the concept of image charges and how they can be used to solve problems involving conductors, such as a grounded infinite plane or a conducting sphere, in the presence of external charges. The professor explains the distribution of charges and the resulting electric fields, emphasizing the uniqueness theorem that guarantees a single solution for the potential function within a region bounded by conductors at known potentials. The lecture also delves into the nature of capacitance, the energy stored in a capacitor, and the calculation of energy density in an electric field. Furthermore, it explores the microscopic perspective of electric current in a conductor, highlighting the role of resistance and collisions in maintaining a steady current. Ohm's law is derived from the relationship between current density, the number of charge carriers, and the electric field. The discussion concludes with an examination of the behavior of capacitors in a circuit, including discharging through a resistor and the calculation of energy transfer. The lecture is rich with analogies and provides a comprehensive understanding of fundamental electrical principles.
Takeaways
- 👨🏫 Professor's Perspective: The professor acknowledges the challenge of knowing students in a large class and the asymmetry of familiarity.
- 🧵 Conductors and Image Charges: The concept of image charges is introduced to simplify problems involving conductors, where the conductor adjusts its charge distribution to maintain an equipotential surface.
- ⚡ Electric Field and Potential: The electric field lines must be perpendicular to the surface of a conductor because any tangential component would imply a change in potential along the conductor's surface.
- 🌐 Grounded Conductors: A grounded conductor, such as an infinite plane or a large sphere, is maintained at zero potential, which influences how charges are induced on its surface.
- 📐 Capacitance Calculation: The capacitance of a system can be calculated using the geometry of the conductors and the principle that the potential difference is proportional to the charge.
- 🔋 Energy Stored in a Capacitor: The energy stored in a capacitor is given by ½CV^2, which can be derived from the work done in moving charge through the potential difference.
- 🔌 Ohm's Law: Ohm's law (V = IR) is derived from the relationship between current density, the electric field, and the material's conductivity.
- 🔵 Current in Conductors: The current in a conductor is due to the motion of charge carriers (usually electrons) which are driven by an electric field and are subject to collisions within the material.
- 🔵🔵 Resistivity and Conductivity: The resistivity and conductivity of a material are determined by the charge carrier's density, their charge, the average time between collisions, and the mass of the carriers.
- ⏲️ Time Constant: The time constant (RC) of an RC circuit determines how long it takes for the capacitor to discharge or charge through a resistor.
- 🔋 Capacitor Discharge: A capacitor discharges through a resistor with the driving force being the charge on the capacitor itself, leading to an exponential decay in charge and voltage.
Q & A
What is the main challenge the professor identifies in teaching a large class?
-The professor acknowledges that in a large class, it's difficult to know what most of the students look like, which makes it hard to form a personal connection or to recognize them outside of the classroom.
Why does the professor mention the asymmetry in a future scenario where a student might become his doctor?
-The professor illustrates the point that there is an asymmetry in recognition because the students will know what he looks like, but he won't know them, which could be disconcerting in a situation like a medical procedure.
What is the significance of the 'image charge' concept in the context of an infinite conducting plane?
-The 'image charge' is a hypothetical charge used to simplify the calculation of electric fields and potentials in the presence of a conductor. It represents the induced charge distribution on the conductor in response to a given charge in the vicinity.
How does the professor describe the process of a conductor achieving a constant potential?
-The conductor achieves a constant potential by inducing charges on its surface that counteract the potential created by external charges. In the case of an infinite conducting plane, it draws negative charges from the ground to maintain a zero potential.
What is the role of the Earth in the context of grounding an object?
-The Earth acts as a large reservoir with nearly zero potential. When an object is grounded, it is connected to the Earth, which allows it to maintain a constant potential, typically zero, by exchanging charges with the Earth.
Why does the professor suggest that the charge distribution on an infinite grounded plane can be calculated?
-The charge distribution on an infinite grounded plane can be calculated because the problem has a simple and symmetrical geometry. This allows for the use of mathematical techniques to determine the distribution of induced charges in response to an external charge.
What is the relationship between the charge on the plane and the image charge in the context of the infinite plane problem?
-The charge on the infinite plane is equal and opposite to the image charge. This means that if the image charge is -q, the total charge induced on the plane must also be -q to maintain the equipotential condition on the plane.
How does the professor explain the distribution of the induced charge on the infinite plane?
-The induced charge on the infinite plane is not evenly distributed. It is concentrated more in front of the external charge and decreases as one moves away from the charge, maintaining the equipotential nature of the plane.
What is the force of attraction between the external charge and the infinite plane?
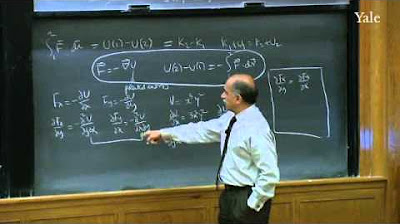
-The force of attraction is calculated based on the Coulomb's law between the external charge q and the image charge -q. The force can be determined by the formula q * (-q) / (4πε₀ * d), where d is the distance between the charges.
What is the potential energy stored in a capacitor?
-The potential energy stored in a capacitor is given by the formula Q²/(2C) or ½CV², where Q is the charge on the capacitor, C is the capacitance, and V is the voltage across the capacitor.
Why does the professor emphasize the uniqueness theorem in the context of conductors and charges?
-The uniqueness theorem is emphasized because it guarantees that if a solution for the potential function is found that satisfies the boundary conditions and charge distributions, it is the only solution. This is crucial for validating the use of image charges and other approximation methods in electrostatics problems.
Outlines
😀 Introduction to Image Charges and Conductors
The professor begins by addressing the anonymity in a large class and humorously remarks on the asymmetry of not recognizing his students, but potentially being operated on by one in the future. He then delves into the topic of image charges, particularly in the context of a perfectly conducting object like a metal potato. He explains the concept of potential and fields generated by charges and how these change when a conductor is introduced. The professor also revisits the question of image charges and emphasizes the importance of understanding the underlying principles.
🧲 Electric Fields and Conductors
The discussion continues with the behavior of electric fields around conductors. The professor explains how a grounded conducting plane, representing an equipotential surface, will induce charges to distribute in a way that maintains a constant potential, which is zero in this case due to grounding. He uses the concept of an 'image charge' to simplify the problem of a charge near a grounded conducting plane and shows that the electric field lines must terminate perpendicularly on the conductor. The professor also explores the distribution of the induced charge on the plane and the force of attraction between the charge and the plane.
📚 Calculation of Charge Distribution on a Conducting Plane
The professor elaborates on how to calculate the charge distribution on an infinite grounded conducting plane when a point charge is brought near it. He uses the concept of a mirror charge to illustrate the distribution and shows that the charge density on the plane can be determined by understanding the electric field. The professor also calculates the force of attraction between the point charge and the plane, as well as the potential energy involved in assembling the charge distribution. He highlights the difference in energy calculations for real and hypothetical scenarios.
💡 Potential Energy and Non-Grounded Conductors
The professor explores what happens when a conducting plane is not grounded. He explains that the conductor cannot acquire a net negative charge and must split into positive and negative charges, distributing them in a way that maintains a constant potential. The positive charges are spread uniformly, while the negative charges arrange themselves to counteract the external charge. This discussion leads to a comparison with a finite conducting sphere and how it would respond to an external charge, introducing the concept of an image charge within the sphere.
🔋 Capacitors and Energy Storage
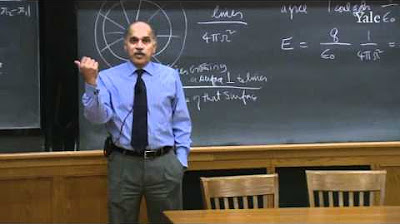
The professor transitions to the topic of capacitors, explaining how they store energy by separating positive and negative charges. He defines capacitance as the system's ability to hold charge and derives the formula for capacitance in a parallel plate capacitor. The professor also discusses the energy stored in a capacitor, presenting two ways to express this energy and highlighting the energy density of an electric field. He emphasizes the importance of considering the energy stored in a capacitor when it is charged.
🏗️ Conductors and Electric Fields
The professor concludes the lecture by discussing the presence of an electric field inside a conductor, which is necessary for current flow. He explains that the current density is uniform across a wire and is due to the motion of carriers, such as electrons, which are accelerated by the electric field but constantly collide with impurities in the material. These collisions cause the electrons to change direction randomly, resulting in a net current that is proportional to the applied electric field. The professor also introduces the concept of resistivity and relates it to the conductivity of materials.
Mindmap
Keywords
💡Equipotential
💡Image Charge
💡Electric Field
💡Conductor
💡Grounding
💡Capacitance
💡Resistivity
💡Ohm's Law
💡Electromotive Force (emf)
💡Energy Storage in a Capacitor
💡Electric Current
Highlights
The professor acknowledges the challenge of knowing students in a large class and the resulting asymmetry in familiarity.
An analogy is made between a doctor wearing a mask and the professor's inability to recognize students due to the large class size.
The concept of image charges is introduced to simplify the calculation of electric fields in the presence of conductors.
The potential and electric field of a point charge near an infinite grounded conducting plane are discussed.
The distribution of charge on an infinite plane and the force of attraction between a charge and the plane are calculated.
The energy required to assemble a charge distribution on a grounded conducting plane is derived.
The scenario of an ungrounded, isolated conducting plane and its behavior upon approaching a charge is explored.
The image charge method is applied to a grounded conducting sphere and the resulting charge distribution is calculated.
The potential energy of a charge near a grounded conducting sphere is determined using the image charge concept.
The behavior of a charge near an isolated (ungrounded) conducting sphere is analyzed, leading to a uniform positive charge distribution.
The professor explains the principle of a capacitor, including how it stores charge and the calculation of capacitance.
The energy stored in a capacitor is calculated, and the concept of energy per unit volume in an electric field is introduced.
The definition of electric current and its relation to the microscopic motion of charge carriers is discussed.
An explanation of current density and its calculation using the number of carriers, their charge, and velocity is provided.
Ohm's law is derived from the microscopic perspective of current carriers in a conductor.
The resistivity of a material and its inverse relationship with conductivity are explained.
The concept of electromotive force (emf) is introduced as the work done per unit charge by non-conservative chemical forces inside a battery.
The balance of energy within a circuit, from the energy stored in a capacitor to the heat produced in a resistor, is demonstrated.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: