11. Lenz's and Faraday's Laws
TLDRThe transcript is a detailed lecture on electromagnetism, focusing on Faraday's law of electromagnetic induction and its applications. The professor begins by summarizing the concept of electromotive force (EMF) in a circuit, explaining how a changing magnetic flux can induce an electric field. The discussion then delves into Lenz's law, which describes the direction of the induced current, aiming to oppose the change causing the EMF. Practical applications, such as betatrons and power generators, are explored to illustrate the conversion between mechanical and electrical energy. The lecture also covers the principles of transformers, which utilize mutual inductance to either step up or step down voltages in AC circuits. Finally, the concept of self-inductance is introduced, highlighting how the energy stored in an inductor is proportional to the square of the current flowing through it, and the importance of understanding inductor behavior in circuit analysis.
Takeaways
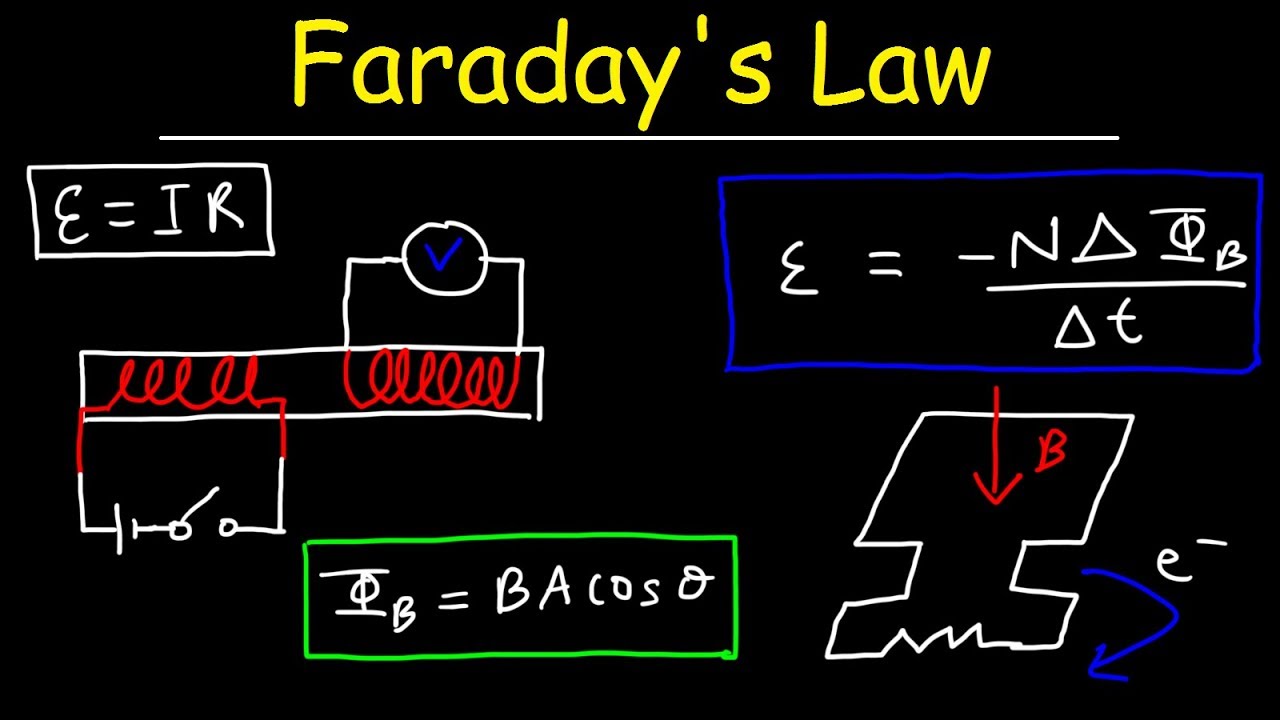
- 📚 The principle of electromotive force (EMF) is explained by Lenz's and Faraday's law, which states that the integrated electric and magnetic force around a closed loop equals the negative rate of change of magnetic flux through that loop.
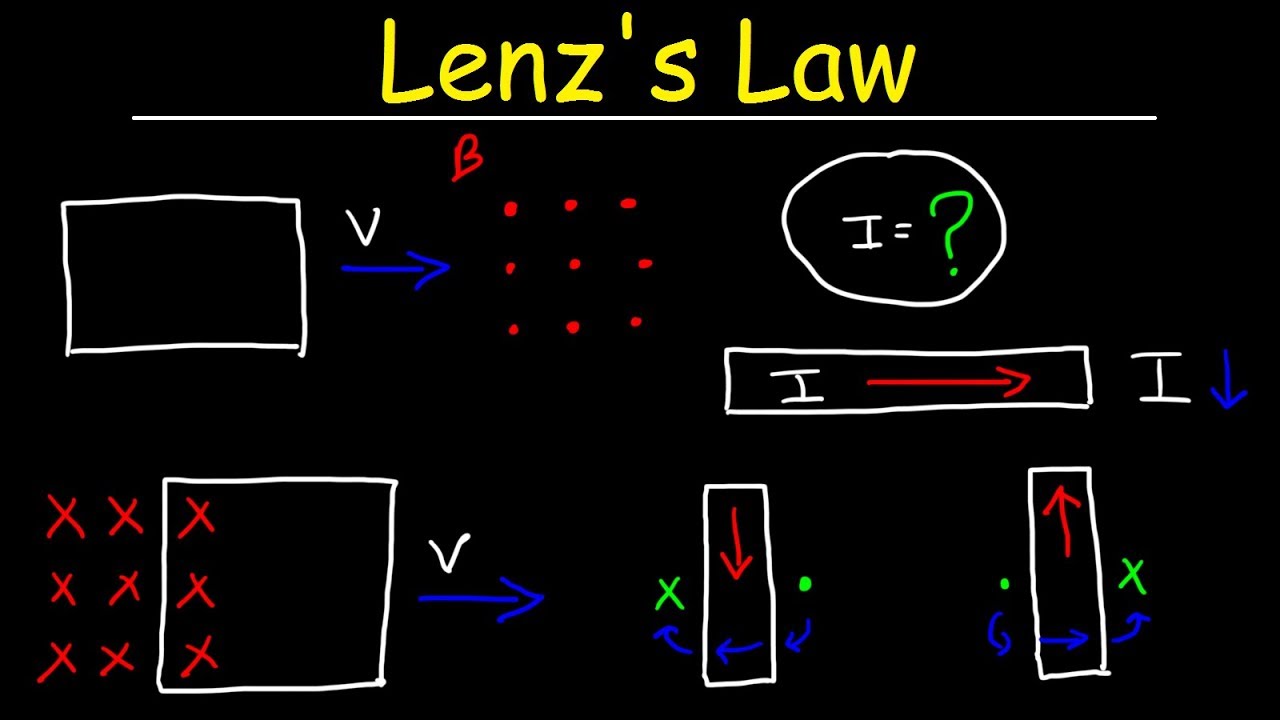
- 🧲 Flux can change due to a time-varying magnetic field (dB/dt) or a spatially-varying field when the loop is in motion, and this change induces an EMF that opposes the change according to Lenz's law.
- 🔋 The concept of self-inductance (L) is introduced as the ratio of the flux linkage to the current, which quantifies the amount of flux produced by a current in a coil and is measured in henries.
- 🔌 Transformers utilize the principle of mutual inductance, where a changing current in one coil induces a voltage in a second coil, allowing for stepping up or stepping down of voltages.
- 🔵 The energy stored in an inductor is proportional to the square of the current (½LI²), which is similar to the energy stored in a capacitor (Q²/2C), and represents the work done against the back EMF to increase the current.
- ⚡ The voltage across an inductor in a circuit is given by LdI/dt, where dI/dt is the rate of change of current, indicating that an inductor opposes changes in current, not the current itself.
- 🔧 The force of a magnetic field on a moving charge in a wire is given by q(v x B)⋅dl, where q is the charge, v is the velocity of the charge, B is the magnetic field, and dl is an element of the path.
- ⏱️ Inductors and capacitors cannot abruptly change the current or charge they carry due to the finite rate of energy change, which is a fundamental principle rooted in the conservation of energy.
- 🔗 The magnetic field energy density is given by B²/(2μ₀), which is analogous to the electric field energy density ε₀E²/2, with μ₀ and ε₀ being the permeability and permittivity of free space, respectively.
- 🔵 The operation of a betatron, a device for accelerating charged particles to relativistic speeds, is explained using the principles of changing magnetic fields and induced electric fields.
- 🌀 The back EMF generated in an inductor is such that it fights the change in current, which is a consequence of Faraday's law of electromagnetic induction and Lenz's law of electromagnetic induction.
Q & A
What is the fundamental principle described by Lenz's and Faraday's law?
-Lenz's and Faraday's law states that the electromotive force (EMF) around a closed loop is equal to the negative rate of change of magnetic flux through that loop. This principle is fundamental in understanding how changing magnetic fields can induce electric fields and generate EMFs.
How does the rate of change of magnetic flux through a loop result in an electromotive force (EMF)?
-The rate of change of magnetic flux through a loop results in an EMF because the total force on a unit charge moving around the loop is given by the rate of change of flux. This can occur due to the explicit time dependence of the magnetic field or due to the motion of the loop in a spatially varying magnetic field.
What is the significance of the negative sign in Faraday's law?
-The negative sign in Faraday's law indicates that the induced EMF will act to oppose the change in magnetic flux that produced it. This is known as Lenz's law, which is a manifestation of the conservation of energy and implies that the induced effects always work to resist changes in the system.
How does the right-hand rule apply to the direction of current induced in a loop by a changing magnetic field?
-The right-hand rule is used to determine the direction of the current induced in a loop by a changing magnetic field. If you point your thumb in the direction of the motion of the loop or the direction in which the magnetic field lines are moving (and your fingers in the direction of the magnetic field), the direction in which your palm faces indicates the direction of the induced current.
What is the relationship between the electromotive force (EMF) and the energy balance in a system where a current is generated?
-The energy balance is maintained as the work done on the wire (or light bulb in the example) is paid for by the person pulling the loop. When a current is generated, it experiences a force (B*I) due to the magnetic field, and overcoming this force with mechanical force converts mechanical energy into electrical energy.
How does the betatron work to accelerate particles even when their motion becomes relativistic?
-The betatron accelerates particles by changing the magnetic field in a manner that the flux through a loop of particles changes, inducing an electric field that circulates and accelerates the particles along a circle. The magnetic field is designed to be stronger near the center and weaker near the edges, and it increases in strength over time to keep the particle in a fixed-radius orbit despite the increasing speed.
What is the role of mutual inductance in a transformer?
-Mutual inductance is the property that allows an alternating current in one coil to induce an electromotive force in a nearby coil due to the shared magnetic flux. In a transformer, this principle is used to either step up or step down the voltage, depending on the ratio of turns in the primary and secondary coils.
Why can't transformers be used to convert DC to AC or vice versa in a simple manner?
-Transformers rely on the principle of mutual inductance and require a changing magnetic flux to operate. Since DC does not produce a changing magnetic flux, transformers cannot be used to convert DC to AC or vice versa in a simple manner. A changing current, such as AC, is necessary to create the varying magnetic field needed for the transformer to function.
How is the self-inductance (L) of a solenoid calculated?
-The self-inductance (L) of a solenoid is calculated using the formula L = μ₀ * n^(2) * A * N / l, where μ₀ is the permeability of free space, n is the number of turns per unit length, A is the cross-sectional area of the solenoid, N is the total number of turns, and l is the length of the solenoid.
What is the energy stored in an inductor when current flows through it?
-The energy stored in an inductor when current flows through it is given by the formula E = ½LI^(2), where L is the inductance, and I is the current flowing through the inductor.
Why can't the current through an inductor change instantaneously when a circuit is closed?
-The current through an inductor cannot change instantaneously due to the back electromotive force (EMF) that opposes rapid changes in current. According to the formula LdI/dt, if the current were to change instantaneously (implying an infinite rate of change, dI/dt), the back EMF would also become infinite, which is not physically possible.
Outlines
🔋 Introduction to Electromotive Force and Lenz's Law
The professor begins by summarizing key concepts discussed previously, focusing on Lenz's and Faraday's laws. These laws describe the electromotive force (EMF) generated in a circuit due to a changing magnetic field. The EMF is defined as the integral of electric and magnetic forces around a closed loop, which equals the negative rate of change of magnetic flux through that loop. Flux is the integral of the magnetic field over an area, and its change can be due to the field's variation in time or space. The professor emphasizes the importance of understanding these principles, which underpin a variety of physical phenomena.
🧲 Faraday's Law and the Generation of Electric Fields
The discussion shifts to Faraday's law, which is a fundamental principle relating time-varying magnetic fields to the generation of electric fields. The professor clarifies that Faraday's law applies even in the absence of a physical loop, highlighting its significance in understanding the interaction between electric and magnetic fields. The emphasis is on the minus sign in Faraday's law, which indicates the direction of the induced EMF opposes the change in magnetic flux. This is illustrated with the example of a moving magnet and a loop, demonstrating how the current generated will always fight to restore the original condition.
🏋️♂️ Energy Conversion in Electromagnetic Systems
The professor delves into the subtleties of energy conversion within electromagnetic systems. Using the example of a wire carrying a current in a magnetic field, the force on the charges within the wire is explained. The distinction between the velocity of the wire (v) and the velocity of charges along the wire (u) is clarified, leading to the conclusion that the net force on a charge is the cross product of the resultant velocity and the magnetic field. The professor reassures students that despite the complexity, the principles governing EMF calculations remain straightforward.
🤹♂️ The Betatron and Particle Acceleration
The professor introduces the betatron, a device capable of accelerating particles even as their motion becomes relativistic. The operation of the betatron is explained through the changing magnetic field, which generates an electric field that accelerates the particles in a circular path. The importance of the magnetic field's distribution is highlighted, with the field at the particle's orbital radius being half the average field to maintain a constant radius despite increasing speed. The limitations of the betatron due to energy radiation at high speeds are also discussed.
⚙️ Power Generation and Electromagnetic Induction
The focus turns to practical applications, specifically power generation through electromagnetic induction. The professor describes the process of generating electricity by rotating a coil within a magnetic field, resulting in a time-dependent voltage. The work done by the person rotating the coil is questioned, and it is concluded that no work is required to maintain a constant rotation in an open circuit. The concept of voltage as akin to potential is introduced, with the voltage across the coil's terminals being time-dependent.
🔌 Circuit Analysis and the Role of Inductors
The professor discusses the analysis of LR (inductor-resistor) circuits, emphasizing the importance of understanding the behavior of inductors in a circuit. The self-inductance of a coil is defined and related to the energy stored within the inductor. The work done in building up the current through an inductor is shown to be related to the magnetic energy stored. The professor also touches on the calculation of self-inductance (L) for a solenoid and relates it to the magnetic field energy within the solenoid.
⚡ The Characteristics of Inductors in Circuits
The professor concludes with the characteristics of inductors in circuits, noting that inductors oppose changes in current rather than current itself. The self-inductance is shown to result in a back EMF that opposes the change in current. The concept of energy storage in an inductor is reiterated, drawing parallels with capacitors. The professor also clarifies misconceptions about the instantaneous change in current or charge, explaining that such abrupt changes are impossible due to the finite rate of energy transfer. The lecture ends with a preview of upcoming topics, including LR, LC, and LCR circuits.
Mindmap
Keywords
💡Electromotive Force (EMF)
💡Faraday's Law
💡Magnetic Flux
💡Lenz's Law
💡Mutual Inductance
💡Self-Inductance
💡Inductor
💡Transformer
💡Energy Storage in Inductors
💡Coulomb's Law
💡AC and DC
Highlights
Introduction to Lenz's and Faraday's law, highlighting the relationship between electromotive force (EMF) and the rate of change of magnetic flux through a loop.
Explanation of how a changing magnetic field can induce an electric field that opposes the change in flux, as described by Lenz's law.
Discussion on the two reasons for flux change: time-varying magnetic fields and spatial changes due to a moving loop.
Derivation of the integral form of Faraday's law, illustrating how the EMF in a loop is related to the surface integral of the rate of change of magnetic field.
The significance of the negative sign in Faraday's law, indicating the direction of induced EMF and current flow.
Practical example of how a betatron uses a changing magnetic field to accelerate particles even at relativistic speeds.
Clarification of the difference between the force on a moving charge in a magnetic field and the EMF generated in a circuit.
Explanation of the energy balance in a circuit, where the work done on a wire or light bulb is paid for by the mechanical force overcoming the magnetic force.
Description of how mutual inductance allows for voltage transformation in devices like transformers, stepping up or down voltage levels.
Calculation of self-inductance (L) for a solenoid, relating it to the magnetic field energy stored within the inductor.
Discussion on the energy stored in an inductor, which is proportional to the square of the current and is responsible for the opposition to current change.
Introduction of the concept of inductor in an LR circuit, and how it behaves differently from a resistor in response to current changes.
Explanation of why the current through an inductor cannot jump instantaneously when a switch is closed in a circuit.
The importance of understanding the behavior of inductors and capacitors in circuits, as they are fundamental to many electrical and electronic systems.
Overview of the topics to be covered in subsequent lectures, including LR circuits, LC circuits, and LCR circuits, and their practical applications.
Emphasis on the practical importance of understanding Faraday's law and inductor behavior for designing and analyzing electrical circuits.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: