AP Physics 2 Circuits Review
TLDRThis video script offers a comprehensive review of circuits for AP Physics 2, explaining fundamental concepts like current, electromotive force, and resistance. It delves into ideal and non-ideal batteries, Ohm's law, and the characteristics of ohmic and non-ohmic materials. The script further explores series and parallel circuits, Kirchhoff's rules, and the dynamic behavior of capacitors, including charging, discharging, and energy storage. It concludes with the significance of power in relation to brightness and the equations governing capacitors, providing a solid foundation for understanding electrical circuits.
Takeaways
- 🔋 Current in a circuit is the rate of flow of charge, analogous to the flow of water, and is determined by the circuit's elements and the electromotive force (EMF) provided by a source like a battery.
- 🔌 A closed loop of conducting material is necessary for a complete circuit, allowing the current to flow from the source through the circuit and back to the source.
- 🔋 Batteries provide an EMF, creating a potential difference and an electric field that drives the flow of charges within the circuit.
- 🔌 Ideal batteries have no internal resistance, but non-ideal batteries do, which affects the actual voltage measured at the battery's terminals compared to the EMF they are designed to produce.
- ⚡ Resistance is a key quantity in circuits, determined by the material's resistivity, its length, and cross-sectional area, and it can be calculated for cylindrical materials using the formula involving π and the radius squared.
- 🌡 Resistivity is a material constant that can change with temperature, and it defines a material's ability to resist the flow of electric current.
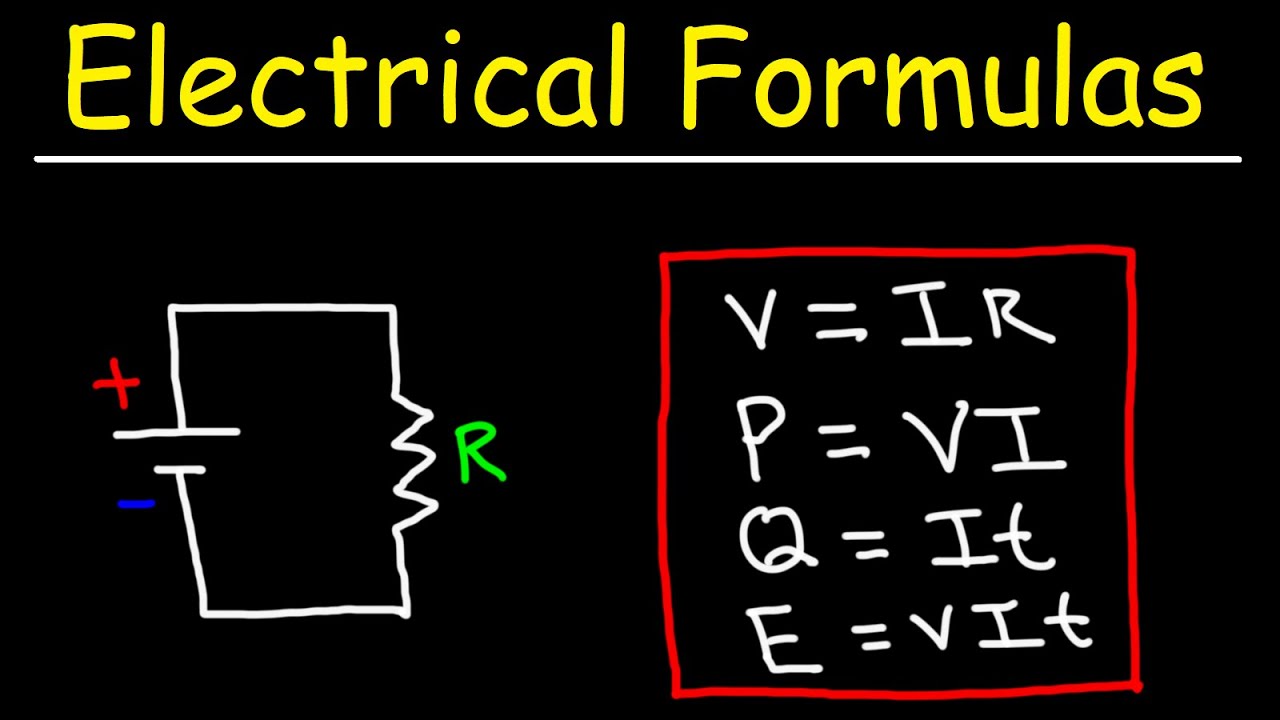
- 🔗 Ohm's law is a fundamental tool for circuit analysis, relating current, voltage, and resistance in ohmic materials, and it can be used to calculate unknown values in a circuit.
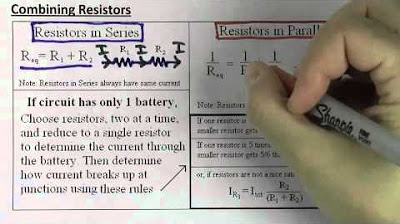
- 🔄 Series circuits have the same current throughout but divide the voltage across components, while parallel circuits have the same voltage across each branch but divide the current.
- 🔄 The equivalent resistance in a series circuit is the sum of individual resistances, increasing the overall resistance, while in a parallel circuit, it is found by taking the reciprocal of the sum of the reciprocals of individual resistances, effectively decreasing the overall resistance.
- 🔄 Kirchhoff's rules, the junction rule and the loop rule, are essential for analyzing complex circuits, ensuring the conservation of current at junctions and the sum of voltage rises and drops in a loop equal to zero.
- 💡 Power in a circuit is related to current and potential difference, and it can be manipulated using Ohm's law to understand the relationship between current, voltage, and resistance in terms of power dissipation and brightness in devices like light bulbs.
Q & A
What is the definition of current in a circuit?
-Current is the rate of flow of charge in a circuit, which can be thought of as the change in charge (ΔQ) divided by the change in time (ΔT).
What is the role of a battery in a circuit?
-A battery provides an electro-motive force (EMF) that creates a potential difference, which in turn generates an electric field to drive the flow of charges within the circuit.
What is the difference between an ideal and a non-ideal battery?
-An ideal battery has zero internal resistance, whereas a non-ideal battery has some internal resistance, which causes a difference between the EMF it is designed to produce and the actual voltage measured across its terminals.
How is the voltage across the terminals of a non-ideal battery calculated?
-The actual voltage across the terminals of a non-ideal battery is calculated by subtracting the voltage drop due to internal resistance (I times R) from the battery's EMF (Epsilon).
What is the formula for calculating resistance based on material properties?
-Resistance (R) can be calculated using the formula R = ρ (L/A), where ρ (Rho) is the resistivity of the material, L is the length, and A is the cross-sectional area.
How does temperature affect resistivity?
-As a material heats up, its resistivity generally increases, which can affect the resistance of a material in a circuit.
What is Ohm's law and how is it used in circuit analysis?
-Ohm's law states that the current (I) in a circuit is equal to the voltage (V) divided by the resistance (R), or I = V/R. It is used to analyze circuits and calculate unknown values of current, voltage, or resistance in an ohmic material.
What is the difference between series and parallel circuits?
-In a series circuit, there is only one path for the current to flow, and the current is the same at all points in the circuit. In a parallel circuit, there are multiple paths for the current, and the voltage across each branch is the same, but the current divides among the branches.
How do you calculate the equivalent resistance of a series circuit?
-The equivalent resistance of a series circuit is found by adding the resistances of all the individual resistors in the circuit.
How is the equivalent resistance of a parallel circuit calculated?
-The equivalent resistance (1/R_eq) of a parallel circuit is calculated by adding the reciprocals of the individual resistances (1/R1 + 1/R2 + ...), and then taking the reciprocal of the result.
What are Kirchhoff's rules and how are they applied in circuit analysis?
-Kirchhoff's rules include the junction rule, which states that the total current entering a junction is equal to the total current leaving it, and the loop rule, which states that the sum of the voltage rises and drops in a closed loop is equal to zero. These rules are used to solve for unknown currents and voltages in complex circuits.
How does a capacitor store charge in a circuit?
-A capacitor stores charge by having two plates that accumulate opposite charges as current flows. When connected to a battery, charge flows to the plates until the capacitor reaches the same voltage as the battery, at which point the current stops flowing.
What is the relationship between capacitance, voltage, and stored charge in a capacitor?
-The relationship is given by the formula Q = C * V, where Q is the charge stored, C is the capacitance, and V is the voltage across the capacitor.
How does the energy stored in a capacitor relate to its capacitance and voltage?
-The energy stored in a capacitor is given by the formula E = 1/2 * C * V^2, where E is the energy, C is the capacitance, and V is the voltage across the plates.
What happens to the current in a series circuit with a capacitor and a resistor when the capacitor is fully charged?
-When the capacitor is fully charged, the voltage across it equals the battery voltage, and no more current flows through the circuit, causing the current to become zero.
What is the effect of adding a dielectric material between the plates of a capacitor?
-Adding a dielectric material between the plates of a capacitor increases the capacitance, allowing it to store more charge before reaching the breakdown voltage of the dielectric.
Outlines
🔋 Basics of Circuits and EMF
This paragraph introduces fundamental concepts of circuits, emphasizing the definition of current as the rate of flow of charge, symbolized as ΔQ/ΔT. It explains the necessity of a closed loop for current flow and the role of a battery in providing electro-motive force (EMF) to drive the charges. The script also touches on the concept of potential difference and electric fields created by the battery, and how these influence the movement of charged particles like electrons. The distinction between ideal and non-ideal batteries is made, with non-ideal batteries having internal resistance that affects the voltage measured across its terminals, which is less than the EMF due to the voltage drop caused by this internal resistance (Epsilon - I*R).
🌐 Understanding Resistance and Ohm's Law
The second paragraph delves into the concept of resistance, detailing how it can be calculated for a cylindrical material using its length and cross-sectional area, and the constant resistivity (Rho) for a given material. It discusses the temperature dependence of resistivity and the method to experimentally determine a material's resistivity by plotting voltage/current ratio against length/area to find the slope, which equals resistivity. Ohm's law is highlighted as a critical tool for circuit analysis, relating current, voltage, and resistance, and noting that while many materials are non-ohmic, they may behave ohmically within certain limits of current and voltage.
🔗 Series and Parallel Circuits Dynamics
This section explains the differences between series and parallel circuits. In a series circuit, the current remains constant throughout, and the total resistance is the sum of individual resistances, leading to a voltage split across components. In contrast, parallel circuits have multiple paths with the same voltage across each branch, resulting in a total current that is the sum of the branch currents and an equivalent resistance that is lower than any individual branch. The paragraph also uses the metaphor of traffic lanes to illustrate how adding paths in a parallel circuit reduces the overall resistance, increasing the total current.
⚡ Kirchhoff's Rules for Circuit Analysis
The fourth paragraph introduces Kirchhoff's current and loop rules for analyzing complex circuits. The junction rule, equating the sum of incoming and outgoing currents at a node, is likened to cars at a four-way stop. The loop rule states that the sum of voltage rises and drops in a closed loop equals zero, allowing for the calculation of unknown currents or voltages within the circuit. These rules are essential for solving for unknowns in circuits that are not straightforward.
💡 Power, Brightness, and Capacitors in Circuits
This paragraph discusses the concept of power in circuits, defined as the product of current and potential difference, and its relationship with brightness, especially in light bulbs. It also introduces capacitors as components that store charge over time, affecting the dynamics of a circuit. When a capacitor is connected to a battery, it charges until it reaches the battery's voltage, after which the current flow stops. The discharge of a charged capacitor through a light bulb results in a brief illumination, demonstrating the stored energy in the capacitor.
🔄 Charging and Discharging of Capacitors
The script explains the process of charging and discharging capacitors in a circuit. Initially, when a switch is closed, the current prefers to flow through an uncharged capacitor due to its lower resistance compared to a light bulb. As the capacitor charges, the current gradually shifts towards the light bulb, causing it to brighten. Once the capacitor is fully charged, all current flows through the light bulb. The paragraph also contrasts this behavior with a series circuit, where the light bulb would gradually dim as the capacitor charges. It introduces the concept of equivalent capacitance in series and parallel capacitor arrangements, with the rules being the inverse of those for resistors.
🔌 Capacitance, Charge, Voltage, and Energy Storage
The final paragraph focuses on the properties and equations related to capacitors. It discusses how capacitance is directly related to the area of the plates and the distance between them, and inversely related to the dielectric constant of the material between the plates. The energy stored in a capacitor is highlighted, with the formula for potential energy given as 1/2 Q ΔV, where Q is the charge on one plate and ΔV is the voltage across the plates. The capacitance, measured in farads, is also explained in terms of its relationship with the charge, voltage, and the physical dimensions of the capacitor.
Mindmap
Keywords
💡Current
💡Electro-Motive Force (EMF)
💡Internal Resistance
💡Ohm's Law
💡Resistivity
💡Series Circuit
💡Parallel Circuit
💡Kirchhoff's Rules
💡Capacitor
💡Energy Storage
Highlights
Current is defined as the rate of flow of charge in a circuit, analogous to the flow of water.
Circuits require a closed loop of conducting material to allow current flow, typically provided by a battery.
Electro-motive force (EMF) is the force provided by a battery that initiates charge movement within a circuit.
EMF creates an electric field within a circuit, influencing the direction of charge movement.
Non-ideal batteries are modeled with internal resistance, affecting the voltage measured at the terminals.
The voltage drop across a non-ideal battery can be calculated using the formula EMF - I*R.
Resistance in a circuit is determined by material properties, length, and cross-sectional area.
Resistivity is a material constant that varies with temperature and defines the material's resistance to current flow.
Ohm's law (V = IR) is fundamental for analyzing circuits and calculating voltage, current, or resistance.
Series circuits have the same current throughout and their resistances can be summed for equivalent resistance.
Parallel circuits have the same voltage across each branch and their resistances combine to decrease equivalent resistance.
Kirchoff's rules (junction and loop rules) are essential for analyzing complex circuits.
Power in a circuit is calculated as current times potential difference, with implications for brightness in devices like light bulbs.
Capacitors store charge over time, affecting circuit dynamics and introducing time-dependent behavior.
The charging process of a capacitor involves an initial rush of current that gradually decreases as the capacitor charges.
The equivalent capacitance of capacitors in series is calculated using the reciprocal of the sum of their individual capacitances.
Capacitors in parallel increase the total capacitance, calculated by adding the values of individual capacitors.
The relationship between capacitance, voltage, and stored charge is given by the formula Q = C*V.
Energy stored in a capacitor is calculated using the formula 1/2 * C * V^2, highlighting the importance of capacitance and voltage.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: