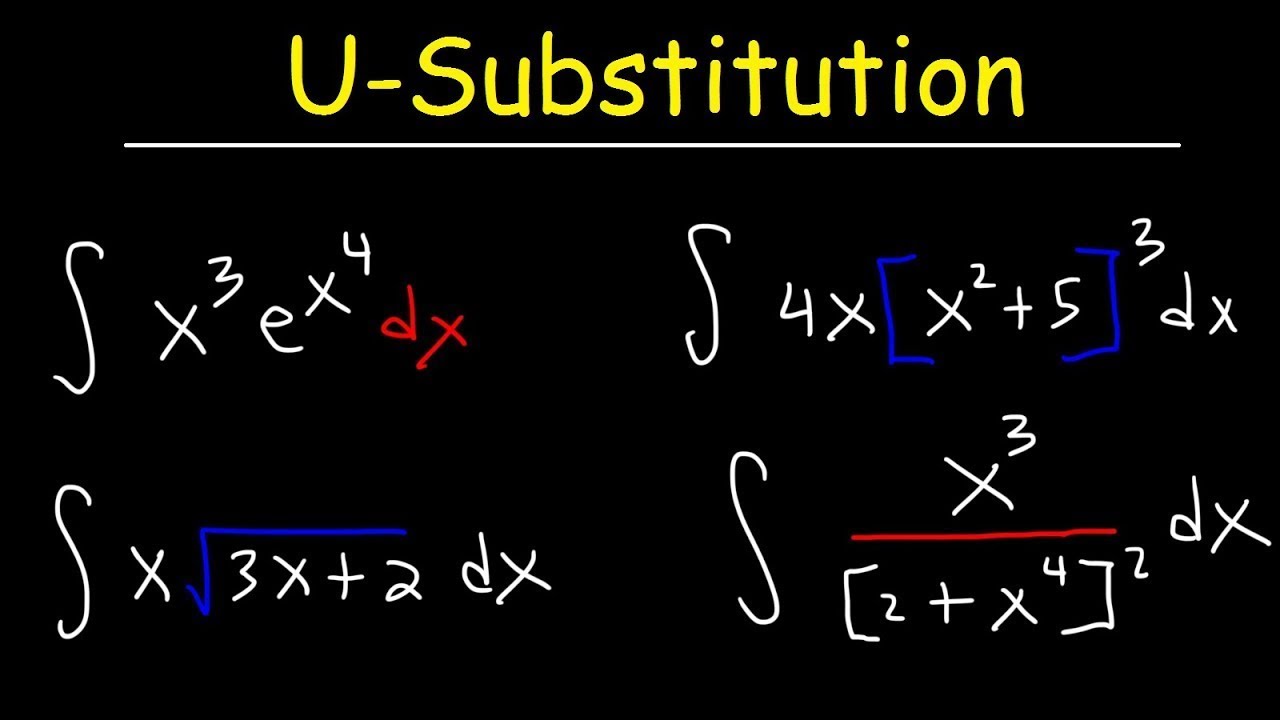
U-Substitution 5-sample video
TLDRThis video script is an educational walkthrough on the technique of U-substitution, a method used in calculus for integrating complex functions. The presenter explains that U-substitution is particularly useful when the power, chain, product, or quotient rules do not apply directly. The script outlines several examples where U-substitution simplifies the integration process, such as when dealing with products under a square root or when the derivative of one part of the product can be used to express another. The presenter also emphasizes the importance of 'side work' or algebraic manipulation to set up the substitution correctly. Throughout the script, the process is illustrated step by step, including finding the differential 'du', replacing 'dx' with the appropriate expression in terms of 'du', and then integrating with respect to 'u'. The video concludes with a reminder that not all functions can be integrated using elementary functions and encourages viewers to practice and explore different integration techniques.
Takeaways
- 📚 The video discusses the concept of integration techniques, emphasizing the use of substitution when the power, chain, product, or quotient rules don't apply directly.
- 🔑 Substitution is a method to simplify complicated integrals by replacing a part of the integral with a new variable, often denoted as 'U'.
- 🔍 To apply U-substitution, find a part of the integral that, when differentiated, produces another part of the integral.
- 🌟 The derivative of the chosen U should be easily integrable and should help simplify the integral into a more manageable form.
- 📐 When using U-substitution, it's crucial to find 'dU' and replace 'dx' with an equivalent expression involving 'dU'.
- 🧮 Perform algebraic manipulations to express all terms in the integral in terms of U, which simplifies the integration process.
- ✅ After integrating with respect to U, remember to substitute back the original variable to complete the solution.
- 🤔 The video provides examples of how to choose U and perform the substitution for different types of integrals, such as those involving square roots or trigonometric functions.
- 📉 The process involves rewriting the integral in terms of U, integrating, and then substituting back to the original variable to find the final answer.
- 📈 The video emphasizes the importance of 'side work' or rough work, which is the practice of solving parts of the problem step by step to reach the final solution.
- ➗ It's important to include the constant of integration 'C' after performing the integral to account for any constant solutions that might exist.
- 🚫 Not all functions can be integrated using elementary functions, and sometimes alternative methods or accepting the integral as it is may be necessary.
Q & A
What is the main technique discussed in the video for solving complex integrals?
-The main technique discussed in the video is U-substitution, which is used to simplify the process of integration when the power rule alone is not sufficient.
How does the video suggest determining whether U-substitution is applicable?
-The video suggests checking if the derivative of one part of the integrand can produce another part. If this is the case, U-substitution is likely to work.
What is the first step in performing U-substitution?
-The first step in performing U-substitution is to identify a suitable function to be represented by U and then find its differential, du, by differentiating the chosen function.
How can you tell if the power of the product in an integral is less, equal to, or greater than the power to which the derivative is raised?
-You can determine this by examining the powers in the integrand. If the power of the product is less than the power to which the derivative is raised, additional algebraic manipulation may be needed. If equal, U-substitution is straightforward. If greater, the integral might not be solvable using elementary functions.
What does the video suggest doing with the differential du when setting up U-substitution?
-The video suggests rewriting the differential du as a multiple of dx (for example, 1/2 du = dx) to simplify the substitution into the integral.
How does the video demonstrate the process of U-substitution?
-The video demonstrates the process of U-substitution by working through several examples, showing how to choose U, rewrite the integral in terms of U, and then integrate using the power rule or other appropriate methods.
What is the purpose of the 'side work' or 'rough work' mentioned in the video?
-The purpose of 'side work' or 'rough work' is to perform the necessary algebraic manipulations and calculations that lead to the simplification of the integral, making it easier to apply U-substitution.
Why is it important to add the constant of integration (C) after performing the integration?
-The constant of integration (C) is important to add because it accounts for any constant solutions that may have been lost during the integration process. It ensures the generality of the solution.
What is the general rule for integrating products of trigonometric functions as mentioned in the video?
-The general rule mentioned in the video for integrating products of trigonometric functions is to look for a substitution that eliminates one of the trigonometric functions in the product, such as using the derivative of tangent to eliminate secant squared.
What does the video suggest if U-substitution does not work for a particular integral?
-If U-substitution does not work, the video suggests trying other methods such as trigonometric substitution, integration by parts, or partial fraction decomposition.
Why is it important to practice making up questions about the material, as suggested in the video?
-Practicing by creating questions helps to reinforce understanding and retention of the material. It also helps to identify any gaps in knowledge or areas that may require further study.
Outlines
📚 Introduction to Integration Techniques
The video begins by contrasting differentiation, which often involves the chain rule, product rule, or quotient rule, with integration, which requires different techniques. The focus is on U substitution, a method for simplifying complex integrals. The speaker explains that U substitution is particularly useful when the derivative of one function can be used to express another. The example given involves a product under a square root, which is simplified by substituting U for a part of the expression and using the derivative to transform the integral into a more manageable form.
🔍 Applying U Substitution to Integration
The second paragraph delves deeper into the U substitution method, illustrating the process with a specific integral involving a power of a binomial. The speaker demonstrates how to identify a suitable U variable, calculate its differential (dU), and then rewrite the integral in terms of U. The process includes algebraic manipulation to express the integral in a form that can be easily integrated, resulting in a simplified expression that can be integrated term by term, followed by the application of the power rule for integration.
🧮 Advanced U Substitution with Algebra
In the third paragraph, the speaker addresses a more complex integral that requires additional algebraic steps to apply U substitution effectively. The example involves an integral with a square root and a power that is not immediately integrable using basic rules. The speaker shows how to choose an appropriate U variable, perform the necessary algebra to express the integral in terms of U, and then integrate. The process concludes with the integration of U to various powers and the final expression of the integral in terms of the original variable, x.
📈 Integration by Manipulation of Trigonometric Functions
The fourth paragraph discusses the application of U substitution to integrals involving trigonometric functions. The speaker explains that when faced with products of trigonometric functions, it's often beneficial to choose an appropriate U that simplifies the expression. The example provided involves secant and tangent functions, and the speaker demonstrates how to manipulate the integral to match the derivative of the chosen U variable. This allows for a straightforward integration, showcasing the power of U substitution in handling complex integrals.
🎓 Final Thoughts on U Substitution and Alternative Integration Methods
The final paragraph wraps up the discussion on U substitution by emphasizing the importance of practice and the existence of other integration methods such as trigonometric substitution, integration by parts, and partial fraction decomposition. The speaker acknowledges that not all functions can be integrated using elementary functions and encourages viewers to explore different techniques. The video concludes with a call to action for viewers to engage with the content, subscribe, and continue learning.
Mindmap
Keywords
💡Differentiation
💡Chain Rule
💡Product Rule
💡Quotient Rule
💡Substitution
💡Power Rule
💡Integral
💡Derivative
💡Trigonometric Functions
💡Constant of Integration
💡Partial Fraction Decomposition
💡Integration by Parts
Highlights
Introduction to the concept of U-substitution in integration, a technique for simplifying complex integrals.
Explanation of when U-substitution is applicable, specifically when the derivative of one part of the integral can produce another part.
Demonstration of U-substitution with an example involving a square root and a power of 8, showcasing a faster way to integrate than using foiling.
The importance of 'side work' or rough work for simplifying the integral before applying U-substitution.
How to find the differential 'du' and rewrite the integral in terms of 'u' and 'du' for easier integration.
Strategy for replacing 'DX' with an expression involving 'du' to simplify the integral.
Use of algebra to express 'x' in terms of 'u' for integration, which is crucial when the power of the product is complex.
Integration techniques when the power of the product is less than, equal to, or greater than the power of 'x'.
Step-by-step guide on performing U-substitution, including finding the antiderivative and adding the constant of integration.
Differentiating between when to use U-substitution and when to use other integration techniques like trigonometric substitution or partial fraction decomposition.
An example of U-substitution with a trigonometric function, illustrating the process of replacing 'secant x' with 'u' and integrating.
The general rule for integrating products of trigonometric functions and when it's appropriate to use U-substitution with trig functions.
Advice on checking the derivative of the chosen 'u' to ensure that U-substitution will be effective for the integral.
Emphasizing the need for practice in U-substitution and other integration techniques to master them.
Encouragement to explore different methods of integration if U-substitution does not seem to work for a particular problem.
A reminder that not all functions can be integrated using elementary functions, and it's important to recognize when U-substitution is not applicable.
The presenter's call to action for viewers to engage with the content by liking, sharing, subscribing, and leaving comments.
Closing thoughts on the importance of continuous learning in mathematics and the significance of perseverance.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: