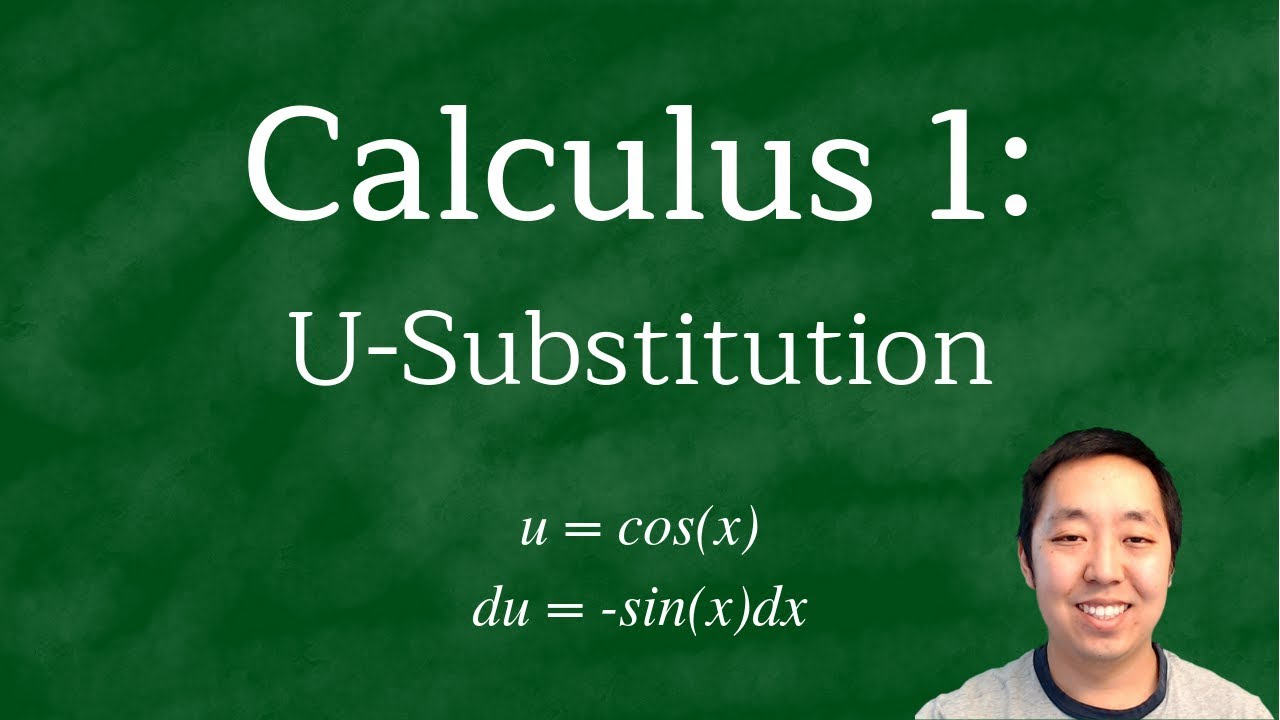
U Substitution!
TLDRThe video script delves into the concept of u-substitution, an integral technique used to simplify complex integration problems that cannot be resolved with basic power rules. The presenter illustrates the method by first discussing the limitations of the power rule and the need for additional strategies. Through a step-by-step process, the video guides viewers on how to identify a suitable u-variable, often a function or expression within the integral that simplifies upon differentiation. The script then demonstrates the u-substitution process with several examples, emphasizing the importance of selecting an appropriate u-variable and the algebraic cancellation that follows. The method is likened to a translation tool, converting a complex problem into a more manageable form, and then translating it back to the original variable. The video concludes with a reminder to look for u-variables whose derivatives are present in the integral, as this often leads to a more straightforward solution. The engaging narrative and practical examples make the concept of u-substitution accessible and highlight its utility in advanced calculus.
Takeaways
- 📚 U-Substitution is a technique used in integration to deal with functions that cannot be integrated using the power rule alone.
- 🔑 The chain rule in differentiation has a corresponding method in integration, which is U-Substitution, allowing us to 'undo' the chain rule.
- ⚙️ The process of U-Substitution involves four steps: defining a new variable 'u', substituting 'du/dx' for the appropriate part of the integral, simplifying, and then integrating with respect to 'u'.
- 🔍 When choosing 'u', it's often beneficial to select something that is trapped by a power, the denominator of a fraction, or something whose derivative appears in the integral.
- 🧙♂️ U-Substitution can be thought of as a 'translator' that converts a complex integral into a more manageable form that we can solve.
- ⛓ The chain rule in differentiation states that the derivative of a composite function is the derivative of the outer function times the derivative of the inner function.
- 🧬 An example given in the script is the integral of 60x \cdot (5x^2 + 7)^5 with respect to 'x', which is solved by setting 'u' to 5x^2 + 7.
- 📉 If the chosen 'u' does not simplify the integral, it may indicate that the wrong 'u' was chosen or that the integral may not be suitable for U-Substitution.
- 🔗 After integrating with respect to 'u', it's essential to substitute back to get the final answer in terms of the original variable 'x'.
- 📌 The script provides several examples to illustrate the process, emphasizing the importance of recognizing patterns and choosing an appropriate 'u' for the substitution.
- ✅ Practicing U-Substitution with various problems will enhance the understanding and application of this technique, making it feel 'like magic' once the user gets the hang of it.
Q & A
What is the main topic being discussed in the transcript?
-The main topic discussed is the method of integration known as u-substitution, which is used to integrate more complex functions that cannot be integrated using the power rule alone.
What is the chain rule in the context of differentiation?
-The chain rule is a fundamental rule used in calculus for finding the derivatives of composite functions. It states that the derivative of a composite function is the derivative of the outer function evaluated at the inner function, times the derivative of the inner function.
How does u-substitution help in integration?
-U-substitution helps in integration by transforming the integral into a simpler form that can be more easily computed. It is particularly useful for integrals that involve functions raised to a power or functions within a power, effectively 'undoing' the chain rule in the integration process.
What is the first step in performing u-substitution?
-The first step in performing u-substitution is to define something to be 'u', typically a function or an expression that is trapped by a power or within a power.
How do you find the expression for 'du/dx' in u-substitution?
-To find the expression for 'du/dx', you take the derivative of the defined 'u' with respect to 'x'. This gives you the relationship between 'u' and 'x', which is necessary to substitute 'dx' in terms of 'du'.
What is the purpose of substituting 'dx' with 'du/du' in the integral?
-Substituting 'dx' with 'du/du' allows the integral to be expressed entirely in terms of 'u', simplifying the integral to a form that can be solved using standard integration techniques such as the power rule.
What is the final step in u-substitution after integrating with respect to 'u'?
-The final step is to substitute back in for 'u' to express the integral in terms of the original variable 'x', thus providing the solution to the original integration problem.
Why might you choose the denominator of a fraction as 'u' when performing u-substitution?
-The denominator of a fraction is often a good choice for 'u' because it usually simplifies the integral when its derivative appears in the original integral, allowing for easier cancellation and simplification.
How does the concept of a 'translator' or 'Rosetta Stone' help in understanding u-substitution?
-The 'translator' or 'Rosetta Stone' analogy helps in understanding u-substitution by viewing it as a process of converting a problem from a complex form into a simpler, more understandable form ('u' terms), and then translating it back to the original form ('x' terms) after integration.
What is the role of the constant 'C' in the context of integration?
-The constant 'C' represents the constant of integration, which is added to the result of an indefinite integral to account for the possibility of an infinite number of antiderivatives differing by a constant.
Why is it important to check if the integral can be solved using the power rule before resorting to u-substitution?
-Checking if the integral can be solved using the power rule is important because it is a simpler and more straightforward method if applicable. U-substitution is a more complex method used when the power rule or other simpler methods are not applicable.
Outlines
😀 Understanding u-Substitution in Integration
The video introduces the concept of u-substitution, a technique used in integration to handle functions that cannot be integrated using the power rule alone. It explains that just as there are multiple methods to derive functions, integration also requires various techniques. The video uses the example of the function f(X) = (5x^2 + 7)^6 to illustrate the need for u-substitution. It shows how to find the derivative using the chain rule and then suggests that integration can be thought of as 'undoing' the derivative. The process involves defining a new variable 'u' to simplify the integral, substituting 'du/dx' for 'dx', and then integrating with respect to 'u'. Finally, the original variable 'x' is substituted back in to find the integral in terms of 'x'.
🔍 The Process of u-Substitution
This paragraph breaks down the u-substitution process into clear steps. It starts by identifying a suitable function to define as 'u', typically a power or a root. Then, it involves substituting 'du/dx' for 'dx' and performing algebraic manipulations to simplify the integral. The integral is then solved with respect to 'u' using the power rule or other integration techniques. Finally, the original variable 'x' is substituted back in to express the integral in terms of 'x'. The video uses the example of integrating 8x * sqrt(4x^2 + 10) to demonstrate these steps, emphasizing the importance of choosing the right 'u' for successful substitution.
📚 Applying u-Substitution to Complex Integrals
The video continues with more examples to illustrate the application of u-substitution. It emphasizes the need to choose the right function for 'u', which is often a power or a root within the integral. The examples include integrating 60x^2 * e^(5x^3 + 1) and handling cases where the initial choice of 'u' does not lead to simplification. The video demonstrates how to correct the process by choosing a different function for 'u', resulting in a simpler integral that can be solved using basic integration rules. It also highlights the importance of looking for patterns in the integral that can be simplified through u-substitution.
🚫 Consequences of Choosing the Wrong 'u'
This paragraph discusses what happens when an incorrect 'u' is chosen during u-substitution. It uses the integral of (21x^2 + 14x) / (x^3 + x^2) as an example, showing that choosing the wrong function for 'u' can lead to a complex integral that does not simplify. The video corrects the mistake by choosing a different function for 'u', which allows for successful simplification and integration. It emphasizes the importance of recognizing when u-substitution is not applicable or when a different choice of 'u' is necessary for simplification.
🔁 Correcting u-Substitution with the Right Choice
The video demonstrates how to correct the process of u-substitution by choosing the right function for 'u'. It shows that by factoring and simplifying the integral, a more suitable 'u' can be identified. The example of integrating 1/(x * ln(x)) is used to illustrate this, where 'u' is chosen as the natural log of 'x', leading to a simpler integral. The video emphasizes the importance of recognizing when a function within the integral is the derivative of the chosen 'u', as this often leads to successful simplification through u-substitution.
🎓 Summary of u-Substitution Technique
The video concludes with a summary of the u-substitution technique, emphasizing its role in 'undoing' the chain rule in integration. It reiterates the steps of the process: checking if the power rule applies, converting the integral into terms of 'u', integrating with respect to 'u', and then converting back to terms of 'x'. The video advises viewers to practice the technique, as it can feel like 'magic' once mastered. It also provides general advice on choosing 'u', suggesting that it is often the bottom of a fraction, the inside of a power, or a function whose derivative appears in the integral.
Mindmap
Keywords
💡Integration
💡u-Substitution
💡Chain Rule
💡Power Rule
💡Derivative
💡Algebraic Manipulation
💡Constant of Integration (C)
💡Definite Integral
💡Natural Logarithm
💡Exponential Function
💡Integration by Parts
Highlights
Introduction to u-substitution as a method for integrating complex functions that do not conform to the power rule.
Derivation of f(x) = (5x^2 + 7)^6 using the chain rule as a precursor to discussing integration.
The integral of 60x * (5x^2 + 7)^5 with respect to x is shown to not follow the power rule, necessitating u-substitution.
U-substitution is introduced as a technique to 'undo' the chain rule in integration.
The process of u-substitution involves defining u in terms of x, substituting du/dx for dx, and simplifying the integral.
Integration of 60x * (5x^2 + 7)^5 with respect to x is demonstrated using u = 5x^2 + 7, resulting in a simpler integral.
The constant 60x is simplified through algebraic manipulation to make the integral easier to solve.
The integral is solved in terms of u and then translated back to terms of x to find the final answer.
The step-by-step process of u-substitution is outlined for clarity and ease of understanding.
A second example problem is solved using u-substitution, emphasizing the method's versatility.
The importance of choosing the correct u to ensure cancellation and simplification in the integral is discussed.
An example of an incorrect u-substitution is shown, illustrating the consequences of an improper choice.
The correct choice of u for the given fraction is the denominator, which simplifies the integral significantly.
Integration of more complex functions, such as exponential and logarithmic, are demonstrated using u-substitution.
The use of u-substitution to integrate 1/x * ln(x) dx is shown, highlighting the method's application to a wide range of integrals.
The concept of u-substitution is likened to a translator or a Rosetta Stone, converting complex integrals into a more understandable form.
The importance of recognizing patterns in the integral that correspond to derivatives of the proposed u is emphasized for successful u-substitution.
A summary of the u-substitution method and its application to undo the chain rule in integration is provided.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: