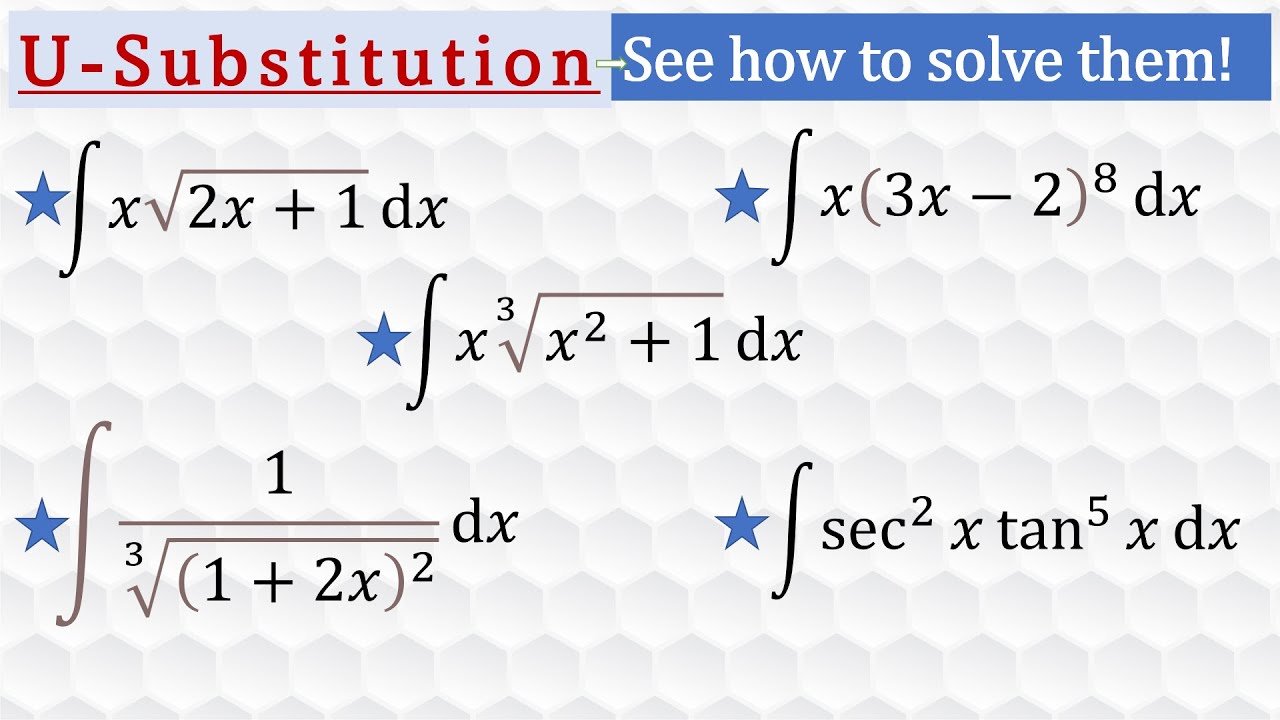
How To Integrate Using U-Substitution

The Organic Chemistry Tutor
19 Apr 202121:35
EducationalLearning
32 Likes 10 Comments

TLDRThis video tutorial demonstrates the method of u-substitution for integrating complex functions. The instructor walks through several examples, showing how to identify the u variable and du, then substituting and integrating to find the antiderivative. The examples cover a range of functions, including polynomials, trigonometric functions, and those involving square roots and exponents. The key takeaway is that with practice, u-substitution can become a straightforward technique for integration.

Takeaways
  • 📚 U-substitution is a method used in calculus to simplify the process of finding definite and indefinite integrals.
  • 🔍 Identifying the appropriate 'u' and its derivative 'du' is crucial for successful u-substitution.
  • 🎯 The choice of 'u' should simplify the integral by transforming all x variables into u variables.
  • 📈 Common strategies involve setting 'u' to an inner function or a more complex part of the integral expression.
  • 🔢 Once 'u' is determined, 'du' is calculated as the derivative of 'u', and dx is isolated to facilitate substitution.
  • 🧮 Substituting 'u' and 'du' into the integral simplifies the equation, often leading to easier integration.
  • ✅ After integration, it's important to substitute back the original variables to find the final answer.
  • 📐 The video covers a variety of examples, including polynomial, trigonometric, and exponential functions, to demonstrate the versatility of u-substitution.
  • 📉 U-substitution can handle a range of scenarios, including functions within square roots or higher degree polynomials.
  • 👨‍🏫 Practice and familiarity with different types of functions and their derivatives are key to mastering u-substitution.
Q & A

  • What is the main topic of the video?

    -The main topic of the video is how to integrate using u-substitution, focusing on definite integrals.

  • What are the two key components needed to perform u-substitution?

    -To perform u-substitution, you need to identify the u variable and du (the derivative with respect to u).

  • How does the video demonstrate the process of u-substitution?

    -The video demonstrates the process of u-substitution by first identifying the appropriate u variable and du, then replacing x with u and dx with du/dx, and finally solving for the antiderivative in terms of u before substituting back the original variable.

  • What is the first example problem solved in the video?

    -The first example problem solved in the video is finding the integral of 4x times (x^2 + 5)^3.

  • How is the u-substitution applied in the example of integrating 8 cosine(4x) dx?

    -In the example of integrating 8 cosine(4x) dx, u is set to 4x, du is 4 dx, and dx becomes du/4. The integral is then solved in terms of u, and finally, u is replaced back with 4x to get the answer.

  • What is the significance of the u-substitution method?

    -The u-substitution method is significant because it simplifies the process of finding antiderivatives for complex integrands, making the integration process more manageable.

  • How does the video handle the example of integrating x^3e^(x^4)?

    -For the example of integrating x^3e^(x^4), u is set to x^4, du is 4x^3 dx, and dx becomes du/4x^3. The integral is then solved in terms of u and finally, u is replaced back with x^4 to get the answer.

  • What is the role of the power rule in u-substitution?

    -The power rule is used in u-substitution to find the antiderivative of u to a certain power. It simplifies the process by applying the rule to the expression in terms of u before substituting back the original variable.

  • How does the video address situations where x and u have the same degree?

    -When x and u have the same degree, the video demonstrates that it's necessary to solve for x in terms of u to eliminate the x variable from the integrand before performing u-substitution.

  • What is the final example problem in the video, and how is it solved?

    -The final example problem is integrating (3x + 2) with respect to x. The video shows that since the x and u have the same degree, it's necessary to solve for x in terms of u, and then perform the u-substitution to find the integral.

  • What is the general advice given in the video for selecting the u variable?

    -The general advice given in the video for selecting the u variable is to choose the part of the integrand that is more complicated or has the higher exponent, as this will simplify the process of integration.

Outlines
00:00
📚 Introduction to U-Substitution

This paragraph introduces the method of U-Substitution for solving definite integrals. It explains the process of identifying the U variable and dU, and how to transform the original integral into a form that cancels out unwanted terms. The example given involves integrating a function of x, which is 4x times the cube of (x^2 + 5). The explanation walks through the steps of substituting x with u, solving for dx, and simplifying the integral to find the antiderivative. The paragraph emphasizes the importance of correctly selecting the U variable to cancel out terms and the process of solving for dx to avoid errors.

05:01
🔍 Applying U-Substitution to Different Functions

This paragraph continues the discussion on U-Substitution by applying it to various functions. It covers the integration of trigonometric functions, exponential functions, and radical expressions. The examples include integrating 8 cosine of 4x dx, x cube e to the x to the fourth, and 8x times the square root of (40 - 2x) squared dx. The explanation highlights the process of selecting the appropriate U variable based on the complexity of the expression inside the integral and demonstrates how to simplify the integral using the power rule and other calculus techniques. The paragraph also emphasizes the importance of solving for dx to ensure the correct substitution and the final step of replacing U with its original expression.

Mindmap
Keywords
💡u-substitution
u-substitution is a method used in calculus to evaluate integrals by transforming them into a more manageable form. It involves selecting a new variable, u, and its derivative with respect to x (du/dx or du), and then rewriting the integral in terms of u. This technique is particularly useful when the integrand is complicated or involves a product of variables. In the video, u-substitution is used to integrate expressions like 4x * (x^2 + 5)^3 and 8cos(4x)dx, where the choice of u simplifies the integral for easier evaluation.
💡antiderivative
The antiderivative of a function is a function whose derivative is the original function. In the context of integration, finding the antiderivative is the process of determining a function whose rate of change matches the function being integrated. The antiderivative is denoted by the integral sign followed by the function and a 'plus c' at the end, indicating that the result can vary by a constant. The video focuses on finding antiderivatives using u-substitution.
💡definite integral
A definite integral represents the net area under a curve between two points on the x-axis. It is defined by the limit of a sum of rectangles approximating the area under the curve. The value of a definite integral is a constant, and it is denoted by the integral symbol with the function, the lower limit of integration, and the upper limit of integration. The video discusses the process of evaluating definite integrals using u-substitution.
💡power rule
The power rule is a fundamental rule in calculus that states if a function y = x^n, where n is a constant, then the derivative of y with respect to x is n*x^(n-1). Conversely, when finding antiderivatives, the power rule states that the integral of x^n dx is (1/(n+1))*x^(n+1) + c, where c is the constant of integration. The video uses the power rule to evaluate antiderivatives after applying u-substitution.
💡trigonometric functions
Trigonometric functions are mathematical functions that relate angles to the ratios of the sides in a right triangle. The most common trigonometric functions are sine (sin), cosine (cos), tangent (tan), and their reciprocals. In the context of the video, trigonometric functions are used in the integrands, and u-substitution is applied to simplify their integration.
💡integration by parts
Integration by parts is a technique used to evaluate integrals of the product of two functions. It is based on the product rule for differentiation and involves reversing the process. The formula for integration by parts is ∫u dv = uv - ∫v du. The video does not explicitly mention integration by parts, but it is another method for integrating more complex functions, and u-substitution is one of the alternatives when integration by parts is not applicable.
💡chain rule
The chain rule is a fundamental principle in calculus used to find the derivative of a composite function. It states that the derivative of a function f(g(x)) is f'(g(x)) * g'(x). The chain rule is used when the function being integrated is a function of another function, and it helps to break down the process of differentiation into simpler steps. Although not explicitly mentioned in the video, the concept is related to the process of u-substitution, where the derivative of u (du/dx) is used to find the derivative of the integrand.
💡constant of integration
The constant of integration, denoted by 'c', is added to the antiderivative of a function to account for the infinite number of possible antiderivatives that exist for a given function. Since the derivative of a constant is zero, the antiderivative process is not unique, and adding 'c' allows for the inclusion of all possible antiderivatives. The constant 'c' is a crucial part of the integral notation and is included in every indefinite integral.
💡derivative
A derivative is a mathematical concept that represents the rate of change or the slope of a function at a particular point. It is a fundamental concept in calculus and is used to analyze the behavior of functions, such as their increase or decrease, maximum or minimum values, and the shape of their graphs. In the context of u-substitution, the derivative of the chosen u variable is used to transform the integral into a more manageable form.
💡integration
Integration is the process of finding the antiderivative of a function, which gives the function whose derivative is the original function. It is the reverse operation of differentiation and is used to calculate areas, volumes, and other quantities that involve accumulation. The video focuses on the method of u-substitution as a technique for integration, particularly for more complex integrands.
💡variable
In mathematics, a variable is a symbol that represents a number or an unknown quantity. Variables are used in expressions and equations to denote values that can change. In the context of u-substitution, a new variable 'u' is introduced to simplify the process of integration by transforming the original variable 'x' into 'u', and its derivative 'du/dx' is used to facilitate the calculation.
Highlights

The video demonstrates how to integrate using u-substitution, focusing on definite integrals.

The first example involves finding the anti-derivative of 4x times x squared plus five to the third power.

To apply u-substitution, one must identify the u variable and du (the derivative of u).

The u variable is chosen to be x squared plus 5, and du is 2x dx, which allows for the cancellation of x terms.

The process involves solving for dx in terms of du, and then replacing x with u and dx with du/2x in the integral.

The anti-derivative of the given function is found to be one half u to the fourth plus a constant, where u is x squared plus five.

Another example is the integration of 8 cosine 4x dx, where u is 4x and du is 4 dx.

The anti-derivative of cosine is sine, and the final answer is 2 sine 4x plus a constant.

The video also covers the integration of x cube e raised to the x to the fourth, using u substitution.

For the function x cube e raised to the x to the fourth, u is chosen to be x to the fourth, and du is 4x cubed dx.

The antiderivative in this case is one fourth e to the u plus a constant, and the final answer is one fourth e to the x to the fourth plus a constant.

The video provides a method for integrating 8x times the square root of 40 minus 2x squared dx using u substitution.

In this example, u is set to 40 minus 2x squared, and the final answer is negative four over three times the square root of 40 minus 2x squared raised to the 3/2 plus a constant.

The video explains how to integrate x cubed divided by two plus x to the fourth squared using u substitution, with u set to two plus x to the fourth.

The final answer for this integration is negative one over four times u plus a constant, where u is two plus x to the fourth.

The video demonstrates the integration of the trigonometric function sine to the fourth of x times cosine of x dx using u substitution.

The final answer for the trigonometric integration is one-fifth sine raised to the fifth power of x plus a constant.

The video also addresses the integration of the square root of 5x plus 4, with u set to 5x plus 4.

The final answer for this integration is two over fifteen times the square root of 5x plus 4 raised to the three halves plus a constant.

The video concludes with a more complex example involving the integration of three x plus two, where u is set to three x plus two and x is solved for in terms of u.

The final answer for this complex integration is two over forty-five times three x plus two raised to the five over two, minus four over twenty-seven times three x plus two raised to the three over two, plus a constant.

Transcripts

Browse More Related Video

How to Integrate Using U-Substitution (NancyPi)

U-substitution With Definite Integrals

Integration of Rational Functions By Completing The Square - Calculus

❖ Integration using U-Substitution ❖

U-Substitution 5-sample video

BusCalc 13.2 Integration by Substitution

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
u-substitutionintegralsmathematicsantiderivativetrigonometricalgebraiceducationalstep-by-stepproblem-solvingmath-tutorial