How to Integrate Using U-Substitution (NancyPi)
TLDRIn this informative video, Nancy demonstrates the method of U-substitution for integrating complex functions. She explains that U-substitution is a technique used when direct integration is challenging, by introducing a new variable U to simplify the integral. Nancy walks through several examples, showing how to select the appropriate U expression, calculate the differential DU, and perform the substitution. She also addresses how to handle negative signs and rearrange expressions when the initial DU does not match the integral's requirements. The video concludes with an example involving trigonometric functions, emphasizing the power rule and back-substitution to find the final answer. Nancy's approach is clear and methodical, making U-substitution accessible for those tackling calculus.
Takeaways
- 📚 U-substitution is a technique used in calculus to simplify integrals that are difficult to integrate directly.
- 🔍 To choose the U expression, look for the longer expression involving x that is inside another function, like a power, root, or fraction.
- 📌 The U expression is usually the part of the integral that will become the derivative of the function you are integrating.
- 🧩 After selecting U, find DU by taking the derivative of U with respect to x and including DX in the expression.
- 🔄 When substituting, replace the U expression and DX with DU in the integral, rearranging as needed for easier integration.
- 🎯 The goal of U-substitution is to transform the integral into a form that can be easily integrated using standard rules.
- 📐 If the DU expression does not match the remaining part of the integral, manipulate the U or DU to make it match.
- 🌟 When integrating, remember to apply the power rule, which involves raising the power by 1 and dividing by the new power.
- 🔖 After integration, replace U with its original expression and include the constant 'C' for indefinite integrals.
- 📊 For trigonometric functions, rewrite the expression with parentheses to clarify the power applied to the function before applying U-substitution.
- 👍 The video provides several examples to illustrate the process of U-substitution, including those with fractions, square roots, and trig functions.
Q & A
What is U-substitution in integration?
-U-substitution is a technique used in integration to simplify the integral by changing the variable of integration to make the integral easier to evaluate.
How do you choose the U expression in U-substitution?
-The U expression is usually the longer expression involving x that is inside another function, such as inside a power, under a square root, or at the bottom of a fraction.
What is the first step in U-substitution?
-The first step in U-substitution is to pick the U expression, which is typically the part of the integral that is inside a function.
How do you find the differential DU?
-To find the differential DU, you take the derivative of the U expression with respect to x and include DX, which represents the differential of x.
What happens to DU when you integrate?
-When you integrate, DU disappears because it represents the differential of x, which is not present in the final integrated form.
Why is back substitution necessary in U-substitution?
-Back substitution is necessary to replace the U expression with its original x-dependent form, as the integral was initially expressed in terms of x, not U.
How do you handle a U expression that doesn't give you exactly what you need for integration?
-If the U expression doesn't match what's needed for integration, you may have to manipulate or rearrange the U expression or the DU to get the correct form for substitution.
What is a common scenario where U-substitution is useful?
-U-substitution is particularly useful when the integral involves a function of x that is inside a power, square root, or at the bottom of a fraction, as these are the forms that typically lead to the need for U-substitution.
Can you give an example of a U-substitution problem with a trigonometric function?
-An example of a U-substitution problem with a trigonometric function is integrating a function like (cos(x))^3. Here, you would choose U as cos(x) and find DU as -sin(x)DX, then proceed with the substitution and integration.
What is the final step in U-substitution?
-The final step in U-substitution is to back substitute the original x expression for U in the integrated form to express the result in terms of the original variable.
Why is it important to include 'plus C' in the final answer of an indefinite integral using U-substitution?
-It is important to include 'plus C' in the final answer of an indefinite integral because it represents the constant of integration, which is necessary when there are no limits specified for the integral.
Outlines
📚 Introduction to U-Substitution
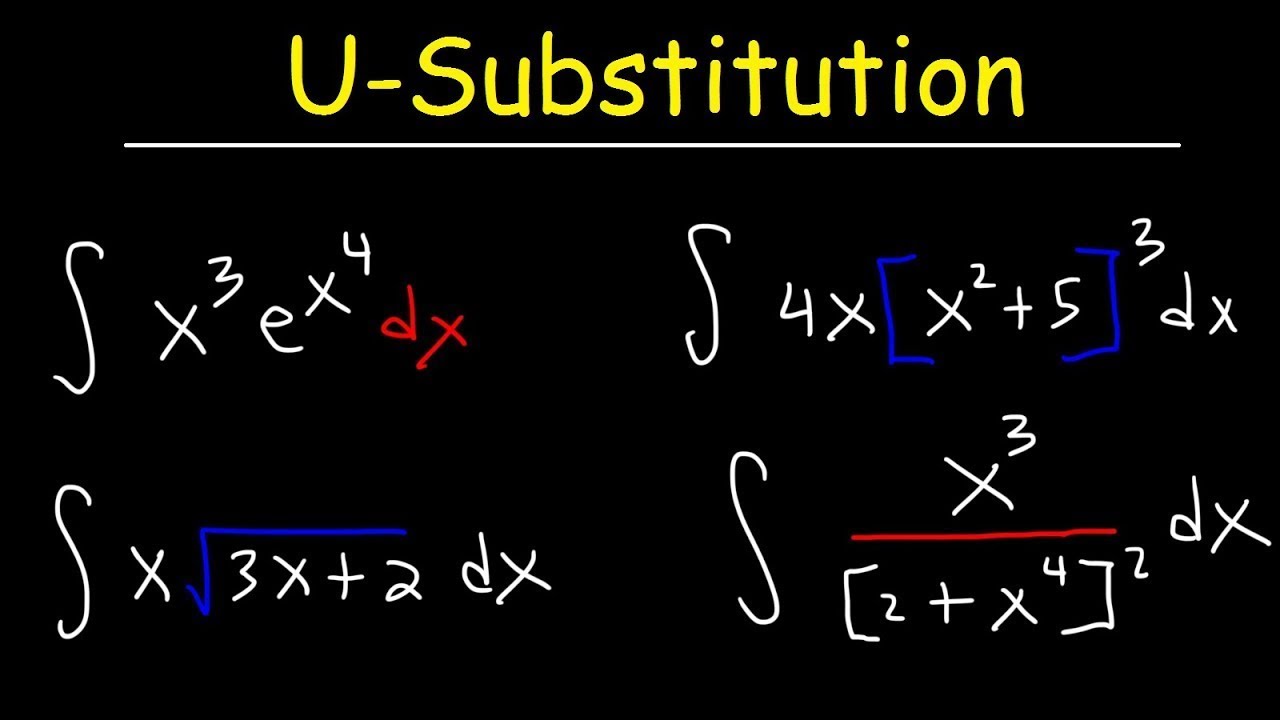
This paragraph introduces the concept of U-substitution, a technique used in calculus to simplify integrals that are not immediately integrable. Nancy explains that U-substitution involves changing the integral by substituting a complex expression with a new variable, U, to make the integration process easier. She outlines the types of problems that will be covered, including those with power rules, fractions, square roots, and trigonometric functions. The first example provided is a basic U-substitution problem involving a power rule.
🧠 Choosing the U Expression and Finding DU
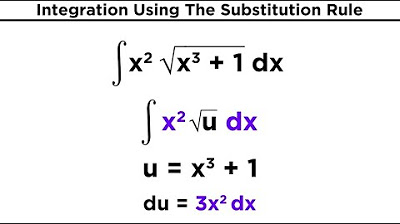
In this paragraph, Nancy discusses the process of selecting an appropriate U expression, which is typically the longer expression within the integral that is part of another function, such as inside a power, square root, or fraction. She emphasizes that the goal of U-substitution is to have the integral divided into two parts: a function and its derivative. The paragraph then illustrates how to find the differential DU by taking the derivative of the U expression with respect to X. The example given involves integrating a function where the U expression is x^3 + 5, and the resulting DU is 3x^2 dx.
🔄 Substituting U and Integrating
This section explains the substitution process, where the integral is rewritten in terms of U and DU. Nancy demonstrates how to replace the original expression with U and the differential with DU, resulting in a simpler integral that can be easily solved using the power rule. She also discusses the disappearance of DU upon integration and the need to back-substitute to replace U with its original expression in X. The example provided involves integrating a function where the U expression is 1 - x^4, and the resulting DU is -4x^3 dx, which is then manipulated to match the integral's remaining expression.
📈 Manipulating U and DU for Integration
Nancy addresses situations where the DU obtained does not directly match the integral's remaining expression, requiring manipulation of U or DU. She shows how to adjust the expressions to make them compatible for substitution. The paragraph includes an example where the U expression is x + 2, and the integral involves a square root. Nancy demonstrates how to rearrange the U expression to isolate X and how to substitute everything in terms of U and DU for easier integration. The process results in an integral that can be solved using the power rule, leading to the final answer involving U.
🌐 Handling Trigonometric Functions with U-Substitution
This paragraph focuses on applying U-substitution to integrals involving trigonometric functions. Nancy explains that when dealing with powers of trigonometric functions, it's helpful to rewrite the expression with parentheses to clarify the U expression. She then demonstrates the process of selecting U, finding DU, and substituting these into the integral. An example is provided where the U expression is cos(x), and the integral involves a cube of the cosine function. The paragraph shows how to simplify the integral by removing a negative sign and using the power rule to integrate, resulting in a final answer expressed in terms of U, which is then back-substituted to X.
🎓 Conclusion and Encouragement for Understanding U-Substitution
Nancy concludes the video by summarizing the process of U-substitution and encouraging viewers to grasp the concept. She acknowledges that not everyone has to like math, but hopes that the explanation provided helps in understanding U-substitution. She invites viewers to like the video or subscribe to her channel if they found the content helpful, emphasizing the goal of making calculus more approachable.
Mindmap
Keywords
💡Integration
💡U-substitution
💡Variable
💡Derivative
💡Power Rule
💡Chain Rule
💡Trigonometric Functions
💡Rearrangement
💡Indefinite Integral
💡Differential
💡Back Substitution
Highlights
Introduction to U-substitution as a method for simplifying complex integrals.
Explanation of when U-substitution is most useful: when direct integration is difficult.
Rule of thumb for choosing U: it's usually the longer expression inside another function.
The integral form for U-substitution must have a function and its derivative.
Example of choosing U for the first problem: U = X^3 + 5.
Demonstration of finding DU by taking the derivative of U with respect to X.
Substitution of U and DU into the integral, resulting in a simpler form.
Use of the power rule to integrate the simplified form of the integral.
Explanation of the disappearance of DU upon integration.
Process of back-substituting U to return the integral to its original variable.
Second example with a fraction and square root, requiring rearrangement of DU.
Strategy for choosing U when the expression is inside a power function.
Manipulation of DU to match the remaining part of the integral.
Integration of the simplified integral using the power rule and back-substitution.
Third example involving a square root and rearrangement of the U expression.
Final example with trigonometric functions and the process of U-substitution.
Rewriting trigonometric expressions with parentheses for clarity in U-substitution.
Solution of the trigonometric integral using U-substitution and power rule.
Summary and encouragement for understanding U-substitution in calculus.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: