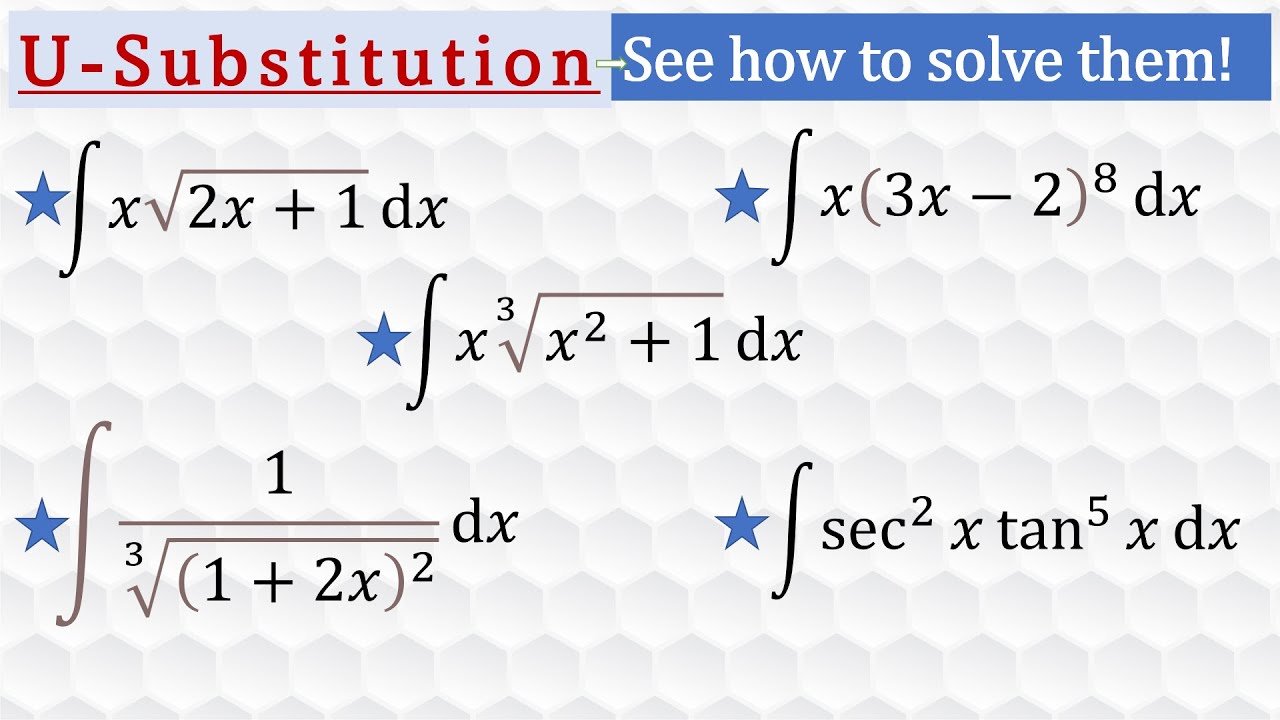
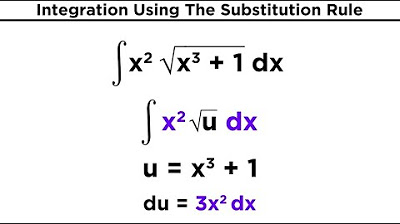
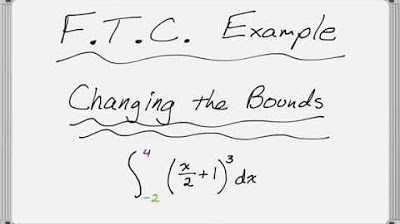Find The Definite Integral Of x3 Into 3 Plus x Power 4 All Power -2 [Evaluate The Definite Integral]
TLDRThe video script is an instructional piece focusing on the substitution technique in calculus, specifically when dealing with integrals of the form involving an exponent. The speaker emphasizes the importance of recognizing the substitution method, which involves two key steps: deriving to identify the variable to substitute (in this case 'u') and then integrating (anti-deriving) to solve the problem. The process is illustrated through an example where the integral of '4x^3 dx' is solved by first substituting 'u' for 'x^3', then finding 'du' in terms of 'dx', and finally integrating 'u' to the power of '-2'. The script also clarifies common confusions regarding the 'du' part of the substitution and the application of the power rule (add and divide). The speaker encourages practice to master the technique and mentions that it is the only method taught in their course for students of science, highlighting its significance in solving integral calculus problems.
Takeaways
- 🔍 The video explains the substitution method in calculus, focusing on selecting 'u' as the base of the exponent and deriving its differential.
- 📝 It details the process of finding 'du' and the steps to replace 'x cube dx' with 'du/4' to simplify the integral calculation.
- 🤔 The instructor clarifies the confusion around how the differential 'du' corresponds to '4x^3 dx' and how it simplifies to 'du over four'.
- 📊 The integral simplifies further by isolating 'u' in the equation and applying basic integral rules ('add one, divide by new power') to solve it.
- 📐 Discussion includes practical tips for handling integrals in exams, emphasizing the need to understand the derivation and substitution thoroughly to avoid confusion.
- 👨🏫 The tutorial uses a specific example to illustrate the substitution technique, showing step-by-step how to replace terms with 'u' and simplify the expression.
- 💡 Emphasizes the importance of practice in mastering the technique, suggesting that first-time exposure might require additional exercises to gain confidence.
- 🔢 The video also covers how to handle definite integrals using the substitution method, including setting up limits of integration.
- 📑 Explains the basic rule of integration ('add one to the power and divide by the new power') through an example, showing how to go from 'u^-2' to 'u^-1'.
- 🎓 The instructor points out that this is the only technique (substitution) taught in this specific course, compared to multiple techniques in more advanced science courses.
Q & A
What is the substitution technique in calculus?
-The substitution technique is a method used in calculus to simplify and evaluate integrals by replacing a complicated expression within the integral with a simpler variable, often denoted as 'u'.
How do you determine the base 'u' for the substitution technique?
-The base 'u' is typically chosen as an expression within the integral that can be differentiated to produce a term that is already present in the integral.
What is the process of finding 'du' in the substitution technique?
-To find 'du', you differentiate the chosen base 'u' with respect to 'x', and then solve for 'du' by isolating it on one side of the equation.
Why do we need to isolate 'du' in the substitution technique?
-Isolating 'du' allows us to express the integral in terms of 'u' and 'du', simplifying the integral and making it easier to evaluate.
What is the purpose of the substitution technique in evaluating definite integrals?
-In definite integrals, the substitution technique helps to simplify the integral, evaluate it, and then apply the limits of integration to find the definite integral's value.
How does the substitution technique relate to the process of anti-derivation?
-The substitution technique involves both differentiation to find the substitution expression and anti-derivation (integration) to find the integral's value after the substitution has been made.
What is the rule number one mentioned in the transcript?
-Rule number one refers to the power rule of integration, where you add the exponent and then divide by the new exponent when integrating a term of the form x^n.
How do you handle the constant factor in the integral during the substitution technique?
-The constant factor is treated separately from the substitution process. It is factored out of the integral and then multiplied back in after the integral of the substitution expression is evaluated.
What is the significance of recognizing standard forms in the substitution technique?
-Recognizing standard forms allows you to quickly apply the substitution technique by identifying suitable expressions to replace with 'u' and their corresponding 'du' expressions.
How does the substitution technique help in evaluating integrals that are not in standard form?
-The substitution technique helps by transforming non-standard integrals into a form that can be easily integrated by recognizing and replacing complex expressions with simpler variables.
What is the final step after evaluating the integral using the substitution technique?
-The final step is to substitute back the original variable 'x' for 'u' in the result obtained from the integral of the substituted expression.
Why is practice important when using the substitution technique?
-Practice is important to become familiar with the technique, recognize suitable expressions for substitution, and efficiently evaluate integrals, especially during exams or time-constrained situations.
Outlines
🧮 Understanding the Substitution Technique in Integration
The first paragraph introduces the concept of the substitution technique in calculus. It explains the process of choosing a base 'u' for the exponent and deriving to obtain a simpler form, such as '4x', which can be replaced with 'du/4'. The paragraph emphasizes the importance of recognizing and replacing terms to simplify the integral. It also discusses the confusion that might arise when dealing with 'du' and how to address it by using the relation between 'du' and 'dx'. The speaker provides a step-by-step guide on how to apply the substitution technique, including the integration process after deriving, and the use of a calculator for evaluating definite integrals. The paragraph concludes with a reminder of the two-step nature of the technique: deriving to replace and then integrating to find the answer.
📚 Mastering the Substitution Technique with Rules and Forms
The second paragraph focuses on simplifying the integration process using the substitution technique by recognizing certain forms and applying specific rules. It mentions that students in science typically learn one main substitution technique, which involves three forms: 'x^a', 'e^x', and '1/x'. The paragraph explains the rules for handling these forms: adding and dividing for 'x^a', and recognizing 'e' and '1/x' as constants in the integral. The speaker stresses the importance of practicing the technique to overcome initial confusion and to become proficient in recognizing and applying these forms. The paragraph ends by encouraging students to refer back to these rules and forms as a reference throughout their studies.
Mindmap
Keywords
💡Substitution technique
💡Derivative
💡Integral
💡Exponent
💡Definite integral
💡Rule number one
💡Isolation
💡Anti-derivation
💡Constants
💡Power rule
💡Integration by substitution
Highlights
The practice involves the substitution technique in integration, where 'u' is chosen as the base of the exponent.
The derivative of the substitution is taken to get the expression to replace.
The integral becomes simpler after substitution, allowing for easier anti-derivation.
The technique involves two steps: deriving to get the expression for substitution, then anti-deriving to integrate.
The integral of x^n can be solved by adding the exponent and dividing by the coefficient.
The integral of 1/x is a known form that can be directly integrated.
The technique requires recognizing and using specific forms like x^a, e^x, and 1/x.
The integral of x^3 dx is used as an example to demonstrate the substitution method.
The integral is simplified by replacing x^3 with u and dx with du/4.
The integral of u^(-2) is solved by recognizing the power rule and integrating.
The constant of integration is added after solving the integral.
Definite integrals involve plugging in the limits of integration into the antiderivative.
A calculator can be used to evaluate definite integrals with numerical limits.
The technique is a fundamental method in calculus with practical applications.
Students are encouraged to practice the technique to gain proficiency.
The substitution technique is the only integration method covered in the course.
The technique involves recognizing and manipulating specific forms to simplify the integral.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: