Completing the Square Made Easy (Precalculus - College Algebra 19)

Professor Leonard

19 Feb 202061:26

EducationalLearning

32 Likes 10 Comments

TLDRIn this educational video, the presenter delves into the concept of completing the square, a method for solving quadratic equations. The video aims to demystify the process by not only guiding viewers through the steps but also by explaining the underlying principles. The host emphasizes the importance of understanding why completing the square works, rather than just memorizing the procedure. This knowledge is highlighted as essential for more advanced mathematics courses, such as calculus, where the method is occasionally required. The video provides step-by-step examples, starting with simpler quadratics and progressing to more complex ones involving fractions. It also touches on the use of the quadratic formula as an alternative solution method when completing the square is not feasible. The presenter's approach focuses on engaging the audience with a clear, logical explanation, making the concept of completing the square more accessible and less intimidating.

Takeaways

📚 Completing the square is a mathematical technique used to solve quadratic equations by transforming them into a perfect square trinomial, which can then be solved using the square root method.
🔍 The process involves understanding why completing the square works and how it can be a powerful tool in advanced math subjects like calculus.
🧩 The key to completing the square is to take a quadratic equation and force it to factor into a perfect square, which allows for the use of the square root method to find solutions.
⚖️ When modifying one side of the equation by adding or subtracting, it's crucial to balance the equation by performing the inverse operation on the other side to maintain equality.
🔑 Finding the correct numbers to add to the quadratic equation to form a perfect square trinomial involves identifying numbers that both add up to the coefficient of the linear term (B) and multiply to the constant term (C).
💡 The principle of completing the square is particularly useful in scenarios where the quadratic equation does not factor easily or where the square root method is not directly applicable.
📐 The technique can be applied even when the quadratic equation has fractions or complex coefficients, although it may require additional steps such as finding a common denominator or adjusting for the coefficients.
🚫 While completing the square is a valuable method, it is not always the most efficient for finding x-intercepts, especially when dealing with equations that have fractions or complex coefficients. In such cases, the quadratic formula may be more practical.
📉 Completing the square can also be used to find the vertex of a parabola, which is helpful in understanding the graph of the quadratic function and its properties.
📝 It is important to practice and understand the process of completing the square not just for its immediate application in solving equations but also because it builds a foundation for more advanced mathematical concepts.
✨ The video script emphasizes the importance of understanding the 'why' behind mathematical processes rather than just memorizing the steps, which is a valuable approach to learning mathematics.

Q & A

What is the main concept of completing the square?
-The main concept of completing the square is to transform a quadratic equation into a perfect square trinomial, which allows for the application of the square root method to solve the equation.
Why is understanding the process behind completing the square important?
-Understanding the process is important because it helps in grasping the mathematical reasoning behind the technique, which can be applied in higher-level mathematics courses like calculus.
What are the two principles involved in completing the square?
-The two principles are: (1) transforming a quadratic into a perfect square trinomial to use the square root method, and (2) maintaining the balance of the equation by adding and subtracting the same value to one side of the equation.
How does the method of completing the square relate to the quadratic formula?
-Completing the square is one way to derive the quadratic formula. It demonstrates how the formula's structure emerges from the process of creating a perfect square trinomial and solving for the variable.
What is the purpose of adding and then subtracting the same value in the process?
-The purpose is to maintain the equality of the equation. When you add a value to one part of the equation, you must subtract the same value to keep the equation balanced and unchanged, except for the manipulation needed to complete the square.
When is it appropriate to use completing the square instead of the quadratic formula?
-Completing the square is typically used when the quadratic equation is easily transformed into a perfect square trinomial, especially when the coefficient of the x² term is 1. The quadratic formula is more versatile and is generally preferred for equations that are not easily factorable or when dealing with more complex quadratics.
How does one find the numbers that need to be added and subtracted to complete the square?
-To find the numbers, you need to determine what values, when added to the middle term of the quadratic and then squared, will result in a perfect square trinomial. These numbers are typically half of the coefficient of the linear term (x term).
What is the significance of the coefficient 'a' in the context of completing the square?
-The coefficient 'a' is significant because if 'a' is 1, it simplifies the process of completing the square. When 'a' is not 1, you often need to factor it out first, which can make the process more complex.
Why might one choose to get rid of fractions when solving a quadratic equation?
-Getting rid of fractions simplifies the equation and makes it easier to apply algebraic techniques such as factoring or applying the square root method. It also avoids the complexity of dealing with fractional coefficients or terms.
How does the process of completing the square help in understanding the structure of quadratic equations?
-Completing the square helps in understanding that a quadratic equation can be rewritten in the form of a perfect square trinomial plus or minus a constant. This insight is valuable for recognizing patterns and structures in quadratic equations, which is a fundamental skill in algebra.
What is the role of the constant term in the process of completing the square?
-The constant term often needs to be 'held off' or separated from the rest of the equation during the process. This allows you to focus on creating a perfect square trinomial with the x terms, which is then combined with the constant term to maintain the equality of the equation.

Outlines

00:00

📚 Introduction to Completing the Square

The video begins with an introduction to the concept of completing the square, emphasizing that understanding the method is crucial for its application in more advanced mathematics such as calculus. The presenter aims to explain not just the process but also the reasoning behind it, highlighting the importance of this technique in solving quadratic equations and its connection to the square root method and trigonometric substitution.

05:02

🔍 Understanding the Principles of Completing the Square

The second paragraph delves into the principles behind completing the square. It discusses the idea of transforming a quadratic equation into a perfect square trinomial to apply the square root method. The presenter also covers the concept of balance in equations, explaining that any operation performed on one side of the equation must be counterbalanced to maintain equality.

10:03

🧩 Demonstrating the Process with an Example

The third paragraph presents a step-by-step example of completing the square using the equation x^2 - 6x + 9 = 0. It illustrates how to identify the coefficients that will create a perfect square trinomial and how to adjust the equation to make it factorable. The process of adding and then subtracting the same value to maintain equality in the equation is also demonstrated.

15:08

🔢 Applying the Method to Non-Factorable Quadratics

In the fourth paragraph, the presenter discusses how to apply completing the square to quadratic equations that are not factorable using the square root method. It emphasizes the need to identify the correct numbers that, when added and multiplied, will create a perfect square trinomial. The paragraph also touches on the decision-making process regarding whether to use the quadratic formula or to complete the square.

20:10

📝 Completing the Square with Fractions

The fifth paragraph addresses the challenge of completing the square when the quadratic equation involves fractions. It demonstrates how to adjust the process to find the correct numbers that will lead to a perfect square trinomial, even when dealing with fractional coefficients. The presenter also shows how to handle the distribution and combination of terms in the equation.

25:13

📐 Using the Square Root Method After Completing the Square

The sixth paragraph explains how to use the square root method after successfully completing the square. It details the steps to isolate the perfect square trinomial, take the square root of both sides, and solve for x. The presenter also discusses the implications of the solutions, such as whether they are real or complex, and how to interpret these in the context of the quadratic equation.

30:14

🎓 Conclusion and Further Applications

The final paragraph concludes the lesson on completing the square, reinforcing its utility in mathematics beyond just finding x-intercepts. It mentions that while the quadratic formula is often more efficient for practical problems, understanding and being able to complete the square is valuable for more advanced mathematical concepts that the viewer will encounter in the future.

Mindmap

Keywords

💡Completing the Square

Completing the square is a mathematical method used to solve quadratic equations. It involves manipulating the equation to express it in the form of a perfect square trinomial plus or minus a constant. In the video, this technique is explained as a way to make the square root method applicable to equations that are not easily factorable. The process is demonstrated with various examples, emphasizing the importance of understanding the underlying principles rather than just following a procedural approach.

💡Quadratic Equation

A quadratic equation is a polynomial equation of the second degree, typically written in the form ax^2 + bx + c = 0, where a, b, and c are constants. The video focuses on solving these types of equations using different methods, including completing the square, which is particularly useful when the equation cannot be easily factored.

💡Square Root Method

The square root method is a technique for solving quadratic equations when the equation can be simplified to the form of a squared term set equal to a positive constant. This allows for the direct application of the square root to both sides of the equation to find the solution. The video explains that this method is not always applicable, leading to the use of completing the square in more complex cases.

💡Factoring

Factoring is the process of breaking down a polynomial into its factors, which are simpler polynomials that when multiplied together give the original polynomial. In the context of the video, factoring is initially attempted for quadratic equations, but when this is not possible, completing the square is introduced as an alternative method to solve for the roots of the equation.

💡Perfect Square Trinomial

A perfect square trinomial is a special type of quadratic polynomial that can be factored into the square of a binomial. It is of the form (a + b)^2 = a^2 + 2ab + b^2. The video demonstrates how to manipulate a non-factorable quadratic equation into a perfect square trinomial, which allows for the application of the square root method to find its solutions.

💡Quadratic Formula

The quadratic formula is a general method for solving quadratic equations and finding the roots of a quadratic function. It is given by x = (-b ± √(b^2 - 4ac)) / (2a), where a, b, and c are coefficients of the quadratic equation ax^2 + bx + c = 0. The video mentions the quadratic formula as an alternative to completing the square, especially when dealing with more complex or time-consuming equations.

💡Precalculus

Precalculus is a branch of mathematics that typically precedes the study of calculus. It covers various algebraic topics, including the solving of quadratic equations through methods like completing the square, which is a subject of the video. The video emphasizes the importance of understanding completing the square not just for its immediate application in precalculus but also for its utility in more advanced mathematics.

💡Trigonometric Substitution

Trigonometric substitution is a technique used in calculus to solve integrals that involve quadratic functions under a square root. The video script mentions that the concept of completing the square, which is the focus of the video, is foundational for understanding more advanced topics like trigonometric substitution.

💡Distributive Property

The distributive property is a fundamental algebraic principle that states that the product of a number and a sum is equal to the sum of the products of the number and each addend. In the context of the video, this property is used when completing the square, particularly when factoring out terms and distributing constants across parentheses.

💡X-intercepts

X-intercepts, also known as zeros or roots, are the points where a function or curve intersects the x-axis. They are the solutions to the equation f(x) = 0. The video discusses finding x-intercepts using different methods, with a focus on when and why completing the square is an appropriate choice.

💡Parabolas

Parabolas are U-shaped curves that are defined by quadratic equations. The video script discusses the properties of parabolas in relation to the solutions of quadratic equations. When completing the square, the process can help identify the vertex of the parabola and determine whether it will intersect the x-axis at real points, which are the x-intercepts.

Highlights

The video explains the concept of completing the square, a method for solving quadratic equations.

Completing the square is not always easy, but understanding the process makes it simpler.

The video emphasizes the importance of understanding the math behind completing the square rather than just memorizing the steps.

Completing the square can be a powerful tool in higher-level math courses, such as calculus and trigonometry.

The process involves turning a quadratic equation into a perfect square trinomial to use the square root method.

The video introduces two key principles for completing the square: understanding the process of creating a perfect square and balancing the equation.

The first principle is to transform the quadratic into a factored perfect square trinomial to apply the square root method.

The second principle is about equation balance: if you add to one side of the equation, you must subtract the same amount on the other side.

The video provides a detailed example of completing the square with the equation x^2 - 6x + 9 = 0.

The process involves identifying the missing number that would make the quadratic factorable.

The video explains how to find the missing number by setting up and solving two equations based on the coefficients of the quadratic.

The video demonstrates that completing the square can be used to solve quadratics that are not easily factorable.

The process of completing the square is shown with the equation x^2 + 4x - 3 = 0, which is not easily factorable.

The video shows how to find the number to add to the quadratic to make it a perfect square trinomial, using the equation x^2 - 3x + 1.

Completing the square can lead to real solutions, complex solutions, or no real solutions, depending on the quadratic equation.

The video concludes by emphasizing the value of understanding the 'why' behind completing the square for future mathematical studies.

Transcripts

Browse More Related Video

Proving the Quadratic Formula - Twice (Precalculus - College Algebra 20)

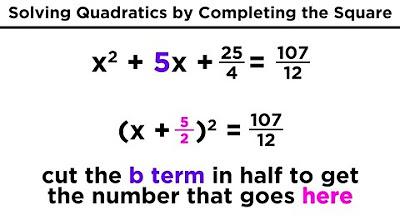
Solving Quadratics by Completing the Square

Calculus Complete the Square and Integrate

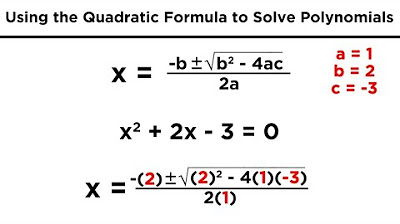
Solving Quadratics by Using the Quadratic Formula

How to Solve By Completing the Square (NancyPi)

How to Solve Quadratic Equations using Three Methods - When Leading Coefficient is Not One

Related Tags

Completing the Square Algebra Method Mathematical Technique Quadratic Equations Educational Content Precalculus Intermediate Algebra Math Tricks Root Finding Square Root Method