Deriving Kinematic Equations - Kinematics - Physics
TLDRThis video script offers a comprehensive guide on deriving the four fundamental kinematic equations, which are essential for analyzing motion with constant acceleration. It begins with explaining how the slope of a velocity-time graph represents acceleration, leading to the first equation. The script then delves into calculating displacement by examining the area under the curve, resulting in the second equation. The third equation is derived from understanding the concept of average velocity, and the fourth equation is elegantly derived by manipulating the previously established equations. The video emphasizes the applicability of these equations in scenarios involving constant acceleration and promises to present a kinematics chart in a subsequent video to assist in selecting the appropriate equation for various problems.
Takeaways
- 📈 The slope of a velocity versus time graph represents acceleration, calculated as the change in velocity over the change in time (∆V/∆T).
- 🚀 The first kinematic equation is derived from the slope of the velocity-time graph: V_final = V_initial + a*t.
- 📊 The area under the curve in a velocity-time graph corresponds to displacement, consisting of both triangular and rectangular components.
- 🔺 The second kinematic equation is derived from the area under the curve: ∆X = V_initial*t + 0.5*(V_final - V_initial)*t^2.
- 🏃♂️ The third kinematic equation relates average velocity to displacement and time: ∆X = (V_final + V_initial)/2 * t, assuming constant acceleration.
- 🔄 The fourth kinematic equation is derived from the relationship between final and initial velocities, acceleration, and displacement: 2A∆X = V_final^2 - V_initial^2.
- 🔢 The kinematic equations are particularly useful for solving problems involving constant acceleration.
- 📝 When using the kinematic equations, it's important to identify which equation is most suitable for the given problem.
- 📚 The script provides a foundation for understanding the kinematic equations, which will be further elaborated in subsequent educational content.
- 🛠️ The kinematic equations are a set of tools that, when applied correctly, can help solve a variety of motion-related physics problems.
- 📈 The script emphasizes the importance of understanding the relationships between velocity, acceleration, time, and displacement in kinematic analysis.
Q & A
What are the four kinematic equations derived in the video?
-The four kinematic equations derived in the video are: 1) a = (Vf - Vi) / t, 2) x = (Vi * t) + (1/2) * a * t^2, 3) x = (Vf + Vi) / 2 * t, and 4) Vf^2 = Vi^2 + 2*a*x.
How is acceleration represented on a velocity versus time graph?
-On a velocity versus time graph, acceleration is represented by the slope of the line. The slope is calculated as the change in the velocity (y variable) divided by the change in time (x variable).
What does the area under the curve in a velocity-time graph represent?
-The area under the curve in a velocity-time graph represents the displacement of the object. It can be calculated by summing the areas of the geometric shapes (like triangles and rectangles) under the curve.
How can you calculate the displacement when the motion involves a triangle and a rectangle under the velocity-time graph?
-To calculate the displacement in such a case, you find the area of the rectangle by multiplying the base (Vi * t) by the height (T), and the area of the triangle by using the formula (1/2) * (Vf - Vi) * t. The total displacement is the sum of these two areas.
What is the significance of the average velocity in kinematics?
-The average velocity is significant in kinematics because it represents the change in position (displacement) divided by the change in time. It is useful for calculating the displacement when dealing with constant acceleration motion.
What is the assumption made when using the kinematic equations?
-The assumption made when using the kinematic equations is that the motion involves constant acceleration. If the acceleration is not constant, these equations will not yield accurate results.
How can you determine which kinematic equation to use for a particular problem?
-To determine which kinematic equation to use, one must analyze the given information in the problem, such as whether the acceleration is constant, if initial and final velocities are known, and if the displacement or time is given. A kinematics chart can be a helpful tool for making this decision.
What is the relationship between the final and initial velocities, and the displacement in the third kinematic equation?
-The third kinematic equation shows that the displacement (x) is equal to the average velocity (which is half the sum of the initial and final velocities) multiplied by the time (t). It can also be expressed as Vf^2 = Vi^2 + 2*a*x, indicating that the final velocity is the square root of the sum of the square of the initial velocity and twice the product of acceleration and displacement.
What is the purpose of the next video mentioned in the script?
-The next video will provide a kinematics chart to help viewers decide which kinematic equation to use for solving a particular problem. This chart will serve as a guide to understanding the relationships between the given and unknown variables in kinematics problems.
How does the video script help students overcome a common issue with kinematic equations?
-The video script helps students by providing a step-by-step derivation of the kinematic equations, explaining the assumptions behind them, and offering insights into how to identify which equation is suitable for a given problem. The upcoming kinematics chart in the next video will further assist in this decision-making process.
What is the mathematical representation of the first kinematic equation?
-The first kinematic equation is mathematically represented as a = (Vf - Vi) / t, where 'a' is the acceleration, 'Vf' is the final velocity, 'Vi' is the initial velocity, and 't' is the time.
What are the variables represented by the symbols in the kinematic equations?
-In the kinematic equations, 'Delta X' represents displacement, 'V' represents velocity, 'a' represents acceleration, 't' represents time, and 'A' represents acceleration in the context of the third and fourth equations.
Outlines
📚 Introduction to Kinematic Equations
This paragraph introduces the concept of deriving the four kinematic equations, starting with an analysis of a velocity versus time graph. It explains how the slope of a straight line on this graph represents acceleration and uses the slope equation to define acceleration as the change in velocity over the change in time. The paragraph then proceeds to derive the first kinematic equation, which relates final velocity, initial velocity, and time. It also discusses the significance of the area under the curve in representing displacement and derives the second kinematic equation by calculating the area of a triangle and a rectangle within the graph. The explanation is clear, focusing on the relationship between velocity, acceleration, time, and displacement, and sets the stage for further exploration of the remaining kinematic equations.
📈 Derivation of Remaining Kinematic Equations
The second paragraph continues the derivation of the kinematic equations by focusing on the relationship between average velocity, displacement, and time. It introduces the third kinematic equation, which is derived from the concept that average velocity times time equals displacement. The explanation then leads to the fourth kinematic equation, which is derived by substituting previously established equations and performing algebraic manipulations. The paragraph emphasizes the importance of constant acceleration in the application of these equations and concludes with a brief recap of all four kinematic equations, highlighting their significance in solving motion problems. The summary also notes the common challenge students face in selecting the appropriate equation for a given problem and promises to address this in a subsequent video.
Mindmap
Keywords
💡Kinematic Equations
💡Velocity vs. Time Graph
💡Acceleration
💡Displacement
💡Average Velocity
💡Constant Acceleration
💡Final Velocity
💡Initial Velocity
💡Time
💡Slope
💡Area Under the Curve
Highlights
The video teaches the derivation of the four kinematic equations, which are fundamental in understanding motion.
The velocity versus time graph is introduced as a starting point for deriving the kinematic equations.
The slope of the line in the velocity-time graph represents acceleration, which is a key concept in kinematics.
The first kinematic equation is derived from the slope equation, relating acceleration to the change in velocity over time.
The area under the curve in a velocity-time graph represents displacement, which is crucial for understanding the motion of an object.
The second kinematic equation is derived by calculating the area of a triangle and a rectangle under the curve.
The third kinematic equation is based on the concept that average velocity is equal to the displacement divided by the change in time.
The fourth kinematic equation is derived by substituting the average velocity expression into the displacement equation.
An important assumption made in these derivations is that the motion being analyzed is under constant acceleration.
The third kinematic equation is often seen in the form of VF squared equals VI squared plus 2A Delta X.
The video emphasizes the importance of choosing the right kinematic equation for a particular problem.
The next video will introduce a kinematics chart to help decide which equation to use for solving a problem.
The kinematic equations are widely used in problems dealing with constant acceleration.
The video provides a clear and detailed explanation of each step in deriving the kinematic equations.
The derivation process involves algebraic manipulation and understanding of basic principles of physics.
The video is educational and beneficial for students learning about motion and its mathematical representation.
Transcripts
Browse More Related Video

Deriving Kinematic Equations (UPDATED) - Kinematics - Physics
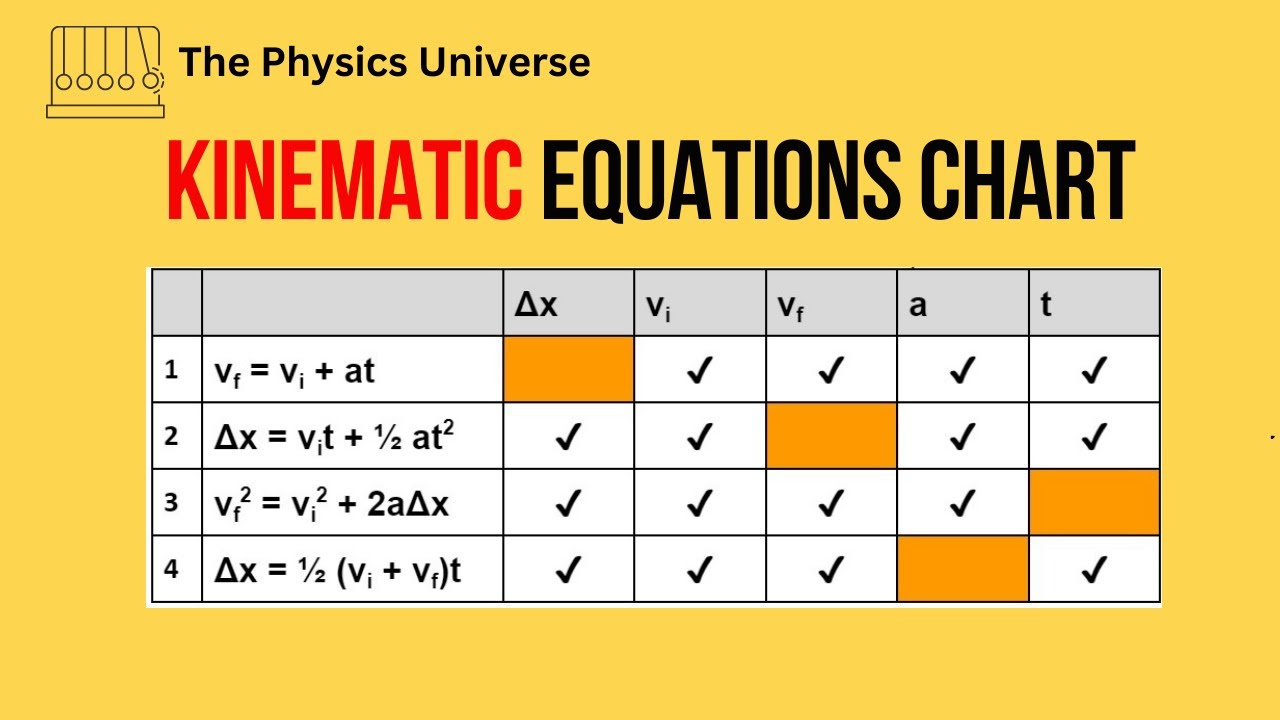
Creating And Using Kinematic Equations Chart - Kinematics - Physics
01 - Motion with Constant Acceleration in Physics (Constant Acceleration Equations)

How to Derive the Equations of Motion (Derivation)
Equation of Motion : How to Select the Right Equation?
motion graphs explained
5.0 / 5 (0 votes)
Thanks for rating: