motion graphs explained

PhysicsHigh
3 Sept 201507:12
EducationalLearning
32 Likes 10 Comments

TLDRThis educational video utilizes PhET Interactive Simulations from the University of Colorado's physics department to explain motion graphs. It demonstrates two scenarios: uniform motion with constant velocity and accelerated motion with constant acceleration. In the first case, a man moves at a steady 5 meters per second, resulting in a horizontal velocity-time graph and a straight displacement-time graph with a constant slope. The second scenario introduces acceleration, showing the man's velocity increasing over time, which is reflected in a sloped displacement-time graph. The video uses these graphs to illustrate concepts like velocity as the slope of the displacement graph and displacement as the area under the velocity-time graph, providing a clear understanding of motion dynamics.

Takeaways
  • 📚 The video uses animation from PhET at the University of Colorado to explain motion graphs.
  • 🚶‍♂️ Situation one involves an object moving at a constant velocity, which is demonstrated with a man moving at 5 meters per second.
  • 🔄 Constant velocity means the object moves at a uniform rate, resulting in a straight line graph with a constant slope.
  • 📊 The velocity-time graph for constant velocity is a horizontal line, indicating no change in speed.
  • 📐 The slope of the displacement-time graph represents velocity, calculated as the change in displacement over time.
  • 📏 From the velocity-time graph, displacement can be determined by calculating the area under the line.
  • 🚀 Situation two involves an object with a constant acceleration, starting from zero velocity and increasing.
  • ⏱ Acceleration is the rate of change of velocity, which in the second scenario, is constant at 1 meter per second squared.
  • 📈 The velocity increases linearly over time in the velocity-time graph, showing a straight line with a positive slope.
  • 🛤 The displacement-time graph for constant acceleration shows an increasing slope, as the object speeds up.
  • 🔢 Displacement can also be found from the area under the velocity-time graph, which in the case of constant acceleration forms a triangle.
Q & A

  • What is the main topic of the video?

    -The main topic of the video is to explain motion graphs using animation from PhET at the University of Colorado's physics department, focusing on constant velocity and constant acceleration scenarios.

  • What is the first scenario demonstrated in the video?

    -The first scenario is where a person or an object is moving at a constant velocity.

  • What is the initial setup for the constant velocity scenario in the video?

    -In the constant velocity scenario, the person (little man) is set to move at a velocity of five meters per second with an acceleration of zero.

  • How does the video represent constant velocity on a graph?

    -The video represents constant velocity on a graph as a straight line with a constant slope, indicating that the displacement changes at the same value over equal intervals of time.

  • What does a horizontal line on a velocity-time graph indicate?

    -A horizontal line on a velocity-time graph indicates that the object is moving at a constant velocity, meaning there is no acceleration or deceleration.

  • How can you determine the velocity from a displacement-time graph?

    -You can determine the velocity from a displacement-time graph by measuring the slope of the line, which is the rise over run, representing the change in displacement over the change in time.

  • What is the second scenario demonstrated in the video?

    -The second scenario is where the object is moving at a constant acceleration.

  • What initial conditions are set for the constant acceleration scenario in the video?

    -In the constant acceleration scenario, the object starts with a velocity of zero and an acceleration of one meter per second squared.

  • How does the video represent constant acceleration on a velocity-time graph?

    -The video represents constant acceleration on a velocity-time graph as a straight line with a constant slope, where the velocity increases at a constant rate over time.

  • How does the displacement-time graph differ between constant velocity and constant acceleration?

    -In the displacement-time graph, constant velocity results in a straight line with a constant slope, while constant acceleration results in a curve with an increasing slope, as the velocity and thus the displacement increase over time.

  • How can you calculate the displacement from a velocity-time graph in the constant acceleration scenario?

    -In the constant acceleration scenario, you can calculate the displacement from the area under the velocity-time graph, which forms a triangle. The area of the triangle represents the total displacement.

Outlines
00:00
📈 Understanding Constant Velocity and Acceleration Graphs

This paragraph introduces the use of animation from PhET, the University of Colorado's physics department, to explain motion graphs. It focuses on two scenarios: constant velocity and constant acceleration. In the first scenario, a man moves at a constant velocity of 5 meters per second across a screen until he hits a wall. The constant velocity is depicted as a straight line with a constant slope in a displacement-time graph, representing uniform motion. The velocity-time graph shows a horizontal line, indicating no change in speed. From the graphs, one can extract information such as velocity, calculated as the slope of the displacement-time graph, and displacement, calculated as the area under the velocity-time graph. The man's displacement is determined to be 20 meters after 4 seconds of travel at a constant velocity.

05:00
🚀 Analyzing Motion with Constant Acceleration

The second paragraph delves into the concept of constant acceleration. The man starts with zero velocity but is given an acceleration of 1 meter per second squared. As the video progresses, it's evident that the man's velocity increases at a constant rate, as acceleration is the rate of change of velocity. The velocity-time graph shows a straight line with a slope of 1, indicating the constant increase in velocity. The displacement-time graph, in this case, shows an increasing slope as the velocity increases, which is consistent with the concept that the slope represents velocity. The area under the displacement-time graph, which is a triangle in this scenario, gives the displacement. By calculating the area of the triangle and considering the time, the man's displacement is determined to be approximately 20 meters after about 6.5 seconds of acceleration.

Mindmap
Keywords
💡Constant Velocity
Constant velocity refers to the motion of an object where the speed and direction remain unchanged over time. In the context of the video, the little man moves at a constant velocity of five meters per second, which is represented by a straight line in the velocity-time graph. This line is horizontal, indicating no change in speed, and the slope of the displacement-time graph is constant, illustrating a uniform rate of movement.
💡Acceleration
Acceleration is the rate at which an object's velocity changes over time. In the video, when the little man's acceleration is set to zero, he moves at a constant velocity, and when it is set to one meter per second squared, his velocity increases uniformly. The script explains that acceleration is zero when velocity does not change, and it is constant when velocity increases at a steady rate.
💡Displacement
Displacement is the change in position of an object. It is a vector quantity that considers both magnitude and direction. In the video, the script describes how the little man's displacement can be determined from the area under the velocity-time graph, which represents the distance traveled over time. The concept is crucial in understanding motion graphs as it directly relates to the object's movement.
💡Velocity-Time Graph
A velocity-time graph is a graphical representation of an object's velocity plotted against time. It helps visualize how the speed of an object changes over time. In the video, the script uses this graph to illustrate constant velocity (a horizontal line) and constant acceleration (an increasing slope). The graph is essential for understanding the relationship between velocity, time, and acceleration.
💡Displacement-Time Graph
A displacement-time graph shows the relationship between an object's displacement and time. It is a useful tool for analyzing motion, especially when the object's velocity is not constant. In the video, the script explains that the slope of this graph gives the velocity, and the area under the graph represents the total displacement, as seen when the little man's velocity increases due to acceleration.
💡Rate of Change
The rate of change is a measure of how quickly a quantity changes with respect to another quantity. In the video, the script uses this concept to explain acceleration as the rate of change of velocity. It is fundamental to understanding how the velocity of an object increases or decreases over time.
💡Slope
Slope, in the context of graphs, is a measure of the steepness of a line and represents the ratio of the change in the dependent variable to the change in the independent variable. The video script mentions that the slope of the displacement-time graph gives the velocity, and as the velocity increases with constant acceleration, so does the slope.
💡Area Under the Graph
The area under a graph, specifically in the context of motion graphs, can represent quantities such as displacement or work done. In the video, the script explains that the area under the velocity-time graph can be used to calculate displacement when the object's velocity is constant, and the area under the displacement-time graph can be used to find displacement when the velocity is changing.
💡Constant Acceleration
Constant acceleration occurs when an object's velocity changes at a steady rate over time. In the video, the script describes a scenario where the little man starts with zero velocity but is given a constant acceleration of one meter per second squared, resulting in an increase in velocity at a constant rate.
💡Phet
Phet is a reference to the educational resource from the University of Colorado's physics department. In the video, the script mentions using Phet's animation to explain motion graphs, indicating that Phet provides interactive simulations and visualizations that help in understanding complex physics concepts like motion and acceleration.
Highlights

Introduction to using PhET animation from the University of Colorado's physics department to explain motion graphs.

Explanation of two motion situations: constant velocity and constant acceleration.

Demonstration of a person moving at a constant velocity with a set speed of 5 meters per second and zero acceleration.

Description of constant velocity as a straight line with a constant rate on a displacement-time graph.

Explanation of how to graph constant velocity resulting in a straight line with a constant slope.

Illustration of a velocity-time graph for constant velocity showing a horizontal line.

Calculation of velocity from the slope of the displacement-time graph.

Derivation of displacement from the area under the velocity-time graph.

Reset to a new situation with zero initial velocity and constant acceleration.

Introduction of a scenario with an object starting from rest and accelerating at 1 meter per second squared.

Observation of increasing velocity over time with constant acceleration.

Analysis of a displacement-time graph showing an increasing slope as velocity increases.

Calculation of displacement from the area under the velocity-time graph as a triangle.

Explanation of how the area of the triangle in the graph represents the object's displacement.

Final demonstration of the object's displacement calculation using the area under the graph.

Conclusion summarizing the understanding of motion graphs for both constant velocity and acceleration.

Transcripts

Browse More Related Video

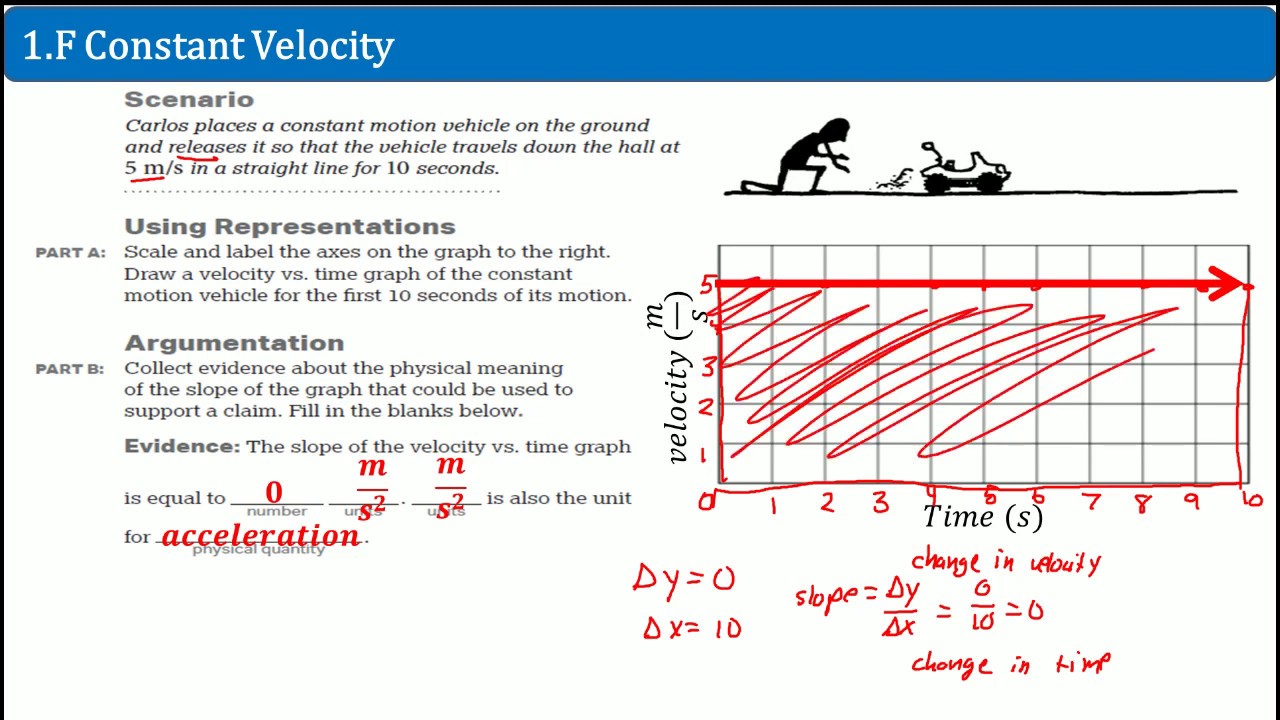
AP Physics Workbook 1.F Constant Velocity

Velocity Time Graphs, Acceleration & Position Time Graphs - Physics

College Physics 1: Lecture 7 - Instantaneous Velocity

01 - Motion with Constant Acceleration in Physics (Constant Acceleration Equations)

Distance (position) to Velocity Time Graph Physics Help

Visualizing Motion Using Tape Charts and Motion Graphs | SCIENCE 7 QUARTER 3 MODULE 2 WEEK 3

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Motion GraphsPhysicsSimulationConstant VelocityConstant AccelerationEducationalPhetUniversity of ColoradoDisplacementVelocity-Time Graph