2022 Live Review 7 | AP Physics 1 | Understanding Torque and Rotational Motion
TLDRIn this educational video, Kristen Gonzalez-Vega delves into the concepts of torque, rotational motion, and energy. She explains how torque is the basis for rotational dynamics, leading to angular acceleration when a net torque acts on an object. The video covers key equations, the significance of rotational inertia, and how it's influenced by mass distribution. Through practical examples and graphical representations, Kristen illustrates how to apply these principles to real-world scenarios, such as balancing forces on a bar, comparing angular accelerations of different shapes, and calculating changes in rotational kinetic energy. The video also touches on the conservation of angular momentum and its relationship with energy in rotational systems, providing a comprehensive understanding of rotational physics.
Takeaways
- 📚 Kristen Gonzalez-Vega teaches AP Physics and discusses rotational motion concepts.
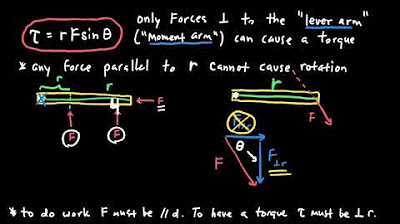
- 🔩 Torque is the rotational equivalent of force and is caused by a force acting perpendicular to a pivot point.
- ⚙️ Objects with net torque experience angular acceleration, similar to how net force causes linear acceleration.
- 📐 The magnitude of angular acceleration depends on the net torque and the object's rotational inertia.
- 📈 Key rotational motion equations include angular acceleration (α), torque (τ), angular momentum (L), and rotational kinetic energy (K).
- 📊 Graphs in rotational motion, such as angular displacement vs. time, can help visualize concepts like angular velocity and acceleration.
- 🤸♀️ Rotational inertia is the rotational equivalent of mass in linear motion and depends on the object's mass and how it's distributed.
- 🏓 The conservation of angular momentum applies when no net torque acts on a system, similar to how linear momentum is conserved with no net force.
- 🔄 Both rotating and translating objects can have angular momentum, and it remains constant if no net torque acts on the object.
- 🌐 The script includes examples and practice problems to apply the concepts of torque, rotational inertia, and angular momentum in various scenarios.
- 🎥 The video also covers the dynamics of pulleys with mass and how they affect the system's acceleration and tension in the strings.
Q & A
What is the definition of torque?
-Torque is the tendency of a force to cause or prevent the rotation of an object. It is calculated as the product of the force applied and the perpendicular distance from the pivot or axis of rotation.
How does torque relate to angular acceleration?
-Torque is directly related to angular acceleration. When a net torque acts on an object, it causes the object to experience an angular acceleration. The magnitude of the angular acceleration depends on the net torque and the rotational inertia of the object.
What is rotational inertia?
-Rotational inertia, also known as moment of inertia, is a measure of an object's resistance to changes in its rotational motion. It depends on the mass of the object and how that mass is distributed relative to the axis of rotation.
How does the distribution of mass affect rotational inertia?
-The distribution of mass affects rotational inertia in that the more mass is located at a greater distance from the axis of rotation, the higher the rotational inertia. This means it will be more difficult to change the object's rotational motion.
What is the relationship between linear motion and rotational motion in physics?
-Linear and rotational motions are different applications of the same fundamental principles of physics. For example, linear acceleration (a = F/m) has a rotational counterpart (alpha = τ/I), linear momentum (p = mv) is similar to angular momentum (L = Iω), and translational kinetic energy (KE = 1/2 mv^2) is analogous to rotational kinetic energy (KE = 1/2 Iω^2).
How can you determine the rotational inertia of an object?
-The rotational inertia (I) of an object can be determined using the formula I = k * m * r^2, where k is a shape constant that varies depending on the shape and mass distribution of the object, m is the mass, and r is the radius of rotation.
What is the significance of angular momentum in physics?
-Angular momentum is a measure of the rotational motion of an object and is important because it is conserved in the absence of an external torque. This conservation principle is crucial in solving problems involving collisions and the transfer of energy and momentum.
How does the conservation of angular momentum apply to a spinning figure skater pulling their arms in?
-When a figure skater pulls their arms in, they decrease their moment of inertia (I) by bringing the mass closer to their body. Since angular momentum (L) is conserved, if I decreases, the angular velocity (ω) must increase to maintain the same L (L = Iω).
What happens to the rotational kinetic energy when the radius of rotation decreases?
-When the radius of rotation decreases, the rotational kinetic energy, which is given by the formula KE = 1/2 Iω^2, increases by the square of the factor by which the radius decreases. This is because the moment of inertia (I) is proportional to the square of the radius (I ∝ r^2).
How can you calculate the change in angular momentum during a collision?
-The change in angular momentum during a collision can be calculated using the impulse-momentum theorem, which states that the change in angular momentum (ΔL) is equal to the net torque (τ) times the change in time (Δt), or ΔL = τΔt.
What is the role of energy conservation in rotational motion?
-While angular momentum is conserved in the absence of an external torque, energy conservation is not always guaranteed. For example, in a perfectly inelastic collision, linear momentum may be conserved, but kinetic energy is not, as some energy is transformed into other forms, such as heat or sound.
Outlines
📚 Introduction to Rotational Concepts
Kristen Gonzalez-Vega introduces the video by stating her credentials and the topic of discussion, which is torque and rotational motion. She outlines the learning objectives, which include reviewing rotational concepts such as rotational dynamics, energy, and momentum, and understanding their application through equations and graphs. Kristen emphasizes that rotation is not a separate topic but an application of forces in a different context, and she encourages viewers to apply the concepts through practice problems.
📐 Understanding Torque and Angular Acceleration
The video delves into the concept of torque, defining it as a force exerted perpendicular to a pivot point, and its relationship with angular acceleration. Kristen explains that the magnitude of angular acceleration depends on the net torque and the rotational inertia of an object, drawing parallels with linear motion. She introduces several equations related to torque, angular momentum, and rotational kinetic energy, and discusses their similarities with linear motion equations. Kristen also emphasizes the importance of understanding graphs in physics, particularly how slopes and areas represent different physical quantities in rotational motion.
🌐 Rotational Inertia and Its Impact on Motion
Kristen defines rotational inertia as the resistance to changes in angular velocity, analogous to mass in linear motion. She explains that rotational inertia depends on the mass distribution of an object, and introduces the formula for rotational inertia, though she notes it's not necessary to memorize it for exams. The video includes a demonstration to visually explain how the distribution of mass affects rotational inertia. Kristen also discusses the relationship between rotational inertia, angular momentum, and rotational kinetic energy, and how these quantities can be used in various scenarios, such as collisions and rolling objects.
📊 Analyzing Graphs for Rotational Motion
The video continues with an analysis of graphs relevant to rotational motion, including angular displacement vs. time, angular velocity vs. time, and angular acceleration vs. time. Kristen explains how to interpret the slopes and areas of these graphs to determine angular velocity, angular acceleration, and changes in angular momentum. She compares these graphs to those from the kinematics unit, highlighting the similarities and differences in their interpretation for linear vs. rotational motion.
🔧 Balancing Torques with Practical Examples
Kristen presents a practical example of balancing torques using a bar with masses hanging from either end. She explains how to draw a free-body diagram for rotational scenarios and how to calculate the unknown mass to balance the bar. The video then moves on to compare the angular acceleration of a hoop and a disk rolling down a ramp, emphasizing the role of rotational inertia in determining which object will reach the bottom first. Kristen uses this example to illustrate the application of torque and rotational inertia concepts in real-world situations.
🌪️ Conservation of Angular Momentum and Energy
The video discusses the conservation of angular momentum and energy in rotational motion. Kristen explains that while angular momentum is conserved when there is no net torque, energy may not be conserved due to factors like friction. She uses the example of a figure skater pulling in their arms to illustrate how angular momentum remains constant while energy changes due to work done by external forces. Kristen also touches on the calculation of angular momentum and impulse, and how these concepts apply to objects both translating and rotating.
🔄 Collisions and Rotational Inertia
Kristen introduces a method to determine the rotational inertia of a bike wheel using collisions with a hoop of known rotational inertia. She explains the procedure of the experiment, which involves measuring the angular velocities before and after the collision and using the conservation of angular momentum to calculate the wheel's rotational inertia. The video demonstrates the concept through a practical example, showing the wheel and hoop sticking together after the collision and rotating at the same angular velocity.
🔧 Pulleys, Tensions, and Rotational Dynamics
The video concludes with a discussion on pulleys, specifically non-ideal pulleys with mass. Kristen explains how the presence of mass on a pulley affects the system's dynamics, requiring the consideration of the pulley's rotational inertia in addition to the linear motion equations. She guides the viewer through drawing free-body diagrams for the pulleys and setting up net force and torque equations. Kristen emphasizes the importance of identifying which forces contribute to torque and how these forces affect the system's acceleration and rotational motion.
🎓 Summary and Additional Resources
In the final part of the video, Kristen summarizes the key takeaways, reminding viewers to draw free-body diagrams for rotational scenarios and to understand when angular momentum and energy are conserved or changing. She encourages viewers to rank forces and understand their impact on rotational motion. Kristen also mentions additional resources, such as AP Daily videos, for further study on the topic of rotation and torque.
Mindmap
Keywords
💡Torque
💡Rotational Motion
💡Rotational Energy
💡Rotational Momentum
💡Angular Acceleration
💡Rotational Inertia
💡Graphs
💡Collisions
💡P pulleys
💡Free Body Diagrams
Highlights
Kristen Gonzalez-Vega teaches AP Physics at Centennial High School in Frisco, Texas.
The lesson focuses on torque, rotational motion, rotational dynamics, and rotational energy.
Torque is the basis of rotational dynamics and is a force exerted perpendicular to a point of action or pivot.
An object experiencing net torque will undergo angular acceleration, similar to how net force causes linear acceleration.
The magnitude of angular acceleration depends on the net torque and the rotational inertia of the object.
Important equations for rotational concepts include angular acceleration, torque, angular momentum, and rotational kinetic energy.
Graphs in physics, such as angular displacement vs. time, can provide insights into the behavior of rotational motion.
Rotational inertia is the equivalent of mass in linear motion and resists changes in rotational velocity.
The rotational inertia of an object depends on its mass and how that mass is distributed.
Objects in rotation possess both angular momentum and rotational kinetic energy.
Angular momentum remains constant if no net torque acts on the system, while linear momentum remains constant if no net force acts on the system.
Torque can be calculated as the product of the distance from the pivot (r) and the force (f) perpendicular to it.
In a balanced torque situation, clockwise torques must be balanced by counterclockwise torques.
The conservation of angular momentum is demonstrated when a figure skater spins with arms out and then pulls them in, increasing angular velocity while keeping angular momentum constant.
In a rotational system with a non-ideal pulley, the pulley's rotational inertia affects the acceleration of falling masses.
The lesson emphasizes the importance of applying linear motion equations to rotational motion, with a focus on understanding the underlying principles.
Transcripts
Browse More Related Video
AP Physics 1 Torque and Rotational Motion Review

AP Physics 1 review of Torque and Angular momentum | Physics | Khan Academy

AP Physics 1 - Rotational Dynamics

Rotational Motion
Rotation Free Response Paragraph Questions (AP Physics 1)

2021 Live Review 7 | AP Physics 1 | Rotational Kinematics, Torque, and Angular Acceleration
5.0 / 5 (0 votes)
Thanks for rating: