Rotational Motion

Bozeman Science
25 Sept 201410:40
EducationalLearning
32 Likes 10 Comments

TLDRIn this informative video, Mr. Andersen explores the principles of rotational motion, emphasizing concepts such as angular displacement, velocity, and acceleration. He explains how rotational inertia and the radian, the fundamental unit for angular measurements, play crucial roles in understanding and calculating rotational dynamics. Through practical examples, including an album spinning demonstration and a comparison of objects with varying rotational inertia, he illustrates how torque and mass distribution affect rotational acceleration. The video also introduces a phet simulation for hands-on learning, encouraging viewers to make predictions and analyze the impact of forces on rotating objects.

Takeaways
  • ๐ŸŒ€ Rotational motion is the turning or spinning motion of an object, which can be dangerous activities like slacklining in Yosemite.
  • ๐Ÿ”ด Applying a net torque to an object results in rotational motion, which can be measured by angular displacement, angular velocity, and angular acceleration.
  • ๐Ÿ“ Angular velocity (ฯ‰) is the rate of change of the angle with respect to time and is measured in radians per second.
  • ๐Ÿ”„ All points on a rotating object have the same angular velocity, but their linear (tangential) velocities differ based on their distance from the axis of rotation.
  • ๐Ÿ“ Linear velocity (v) is calculated by multiplying the angular velocity by the radius (r) from the center of rotation.
  • ๐ŸฅŠ Rotational inertia is a measure of an object's resistance to changes in rotational motion, similar to how mass affects linear motion.
  • ๐Ÿ“‰ The equation for rotational motion is net torque (ฯ„) equals rotational inertia (I) times angular acceleration (ฮฑ), or ฯ„ = Iฮฑ.
  • ๐ŸŒŸ The radian is the base unit for measuring angular displacement and velocity, with 1 radian being approximately 57 degrees.
  • ๐ŸŽต In the example of spinning an album, the angular velocity can be calculated from the number of revolutions per minute, and the linear velocity can be determined at different points on the album.
  • ๐Ÿš€ Centripetal acceleration is the acceleration towards the center experienced by an object in circular motion, and it changes the direction of the velocity even if the speed is constant.
  • ๐Ÿงช Experiments with different shapes and mass distributions demonstrate that objects with lower rotational inertia (more mass concentrated towards the center) have higher angular accelerations under the same torque.
Q & A

  • What is rotational motion?

    -Rotational motion, also known as turning motion, is when an object moves in a circular path around a fixed axis.

  • How does a slack liner in Yosemite apply force to counteract rotation?

    -A slack liner applies a force or net torque on one side of their body, which causes rotation. To counteract this, they must apply an equal and opposite net torque on the other side.

  • What are the three key measures of rotational motion in physics?

    -The three key measures of rotational motion are angular displacement (the angle through which an object rotates), angular velocity (the speed at which an object rotates), and angular acceleration (the rate at which the angular velocity changes over time).

  • How is linear velocity related to angular velocity in rotational motion?

    -Linear velocity is directly related to angular velocity by the equation linear velocity = angular velocity ร— radius. This means that the speed at which a point on a rotating object moves in a straight line is proportional to how fast it is rotating (angular velocity) and its distance from the center of rotation (radius).

  • What is rotational inertia and how is it calculated?

    -Rotational inertia, also known as the moment of inertia, is a measure of an object's resistance to rotational motion. It is calculated by the formula I = mr^2, where m is the mass of the object and r is the distance from the axis of rotation.

  • Why is understanding radians important in the context of rotational motion?

    -Radians are important because they are the base unit for measuring angles in rotational motion. Understanding radians allows for accurate calculations of angular velocity, angular acceleration, and angular displacement.

  • How many radians are in one full revolution?

    -There are 2 pi radians in one full revolution, which is equivalent to 360 degrees.

  • What is the relationship between force, torque, and rotational inertia in rotational motion?

    -In rotational motion, the force or torque applied to an object is equal to the product of the object's rotational inertia (I) and its angular acceleration (ฮฑ). This relationship is similar to Newton's second law in linear motion, where force equals mass times acceleration (F = ma).

  • How does the mass distribution of an object affect its rotational inertia?

    -The mass distribution of an object greatly affects its rotational inertia. An object with more mass concentrated closer to the axis of rotation (like a solid sphere) has a lower rotational inertia compared to an object with the same mass distributed further from the axis (like a hollow sphere).

  • What is the centripetal acceleration experienced by an object in circular motion?

    -Centripetal acceleration is the acceleration experienced by an object in circular motion, always directed towards the center of the circle. It is responsible for keeping the object moving in a circular path and is calculated by the formula ac = v^2 / r, where v is the linear velocity and r is the radius of the circular path.

  • How can you calculate the angular acceleration of an object?

    -To calculate angular acceleration (ฮฑ), you find the change in angular velocity (ฯ‰) over the change in time (t). The formula is ฮฑ = ฮ”ฯ‰ / ฮ”t, where ฮ”ฯ‰ is the difference in angular velocity from the initial to the final state, and ฮ”t is the time interval over which this change occurs.

Outlines
00:00
๐ŸŽฅ Introduction to Rotational Motion

This paragraph introduces the concept of rotational motion, comparing it to a slackliner in Yosemite who must counteract forces with opposite torques. It explains the physics of rotational motion, including angular displacement, velocity, and acceleration, and emphasizes the importance of understanding rotational inertia and the radian unit for these measurements. The paragraph also discusses how objects with different radii from a central axis will have varying linear velocities despite the same angular velocity.

05:02
๐Ÿ“Š Calculating Angular Velocity and Acceleration

The second paragraph delves into the specifics of calculating angular velocity and acceleration using examples. It explains how angular velocity is the rate of change of angle over time and provides a practical example using an album spinning. The paragraph then discusses linear velocity as a function of angular velocity and radius, and how it varies across different points on a rotating object. It also touches on the concept of centripetal acceleration and the effects of applying torque to an object to create motion.

10:03
๐Ÿงฎ Predicting Velocities and Understanding Rotational Inertia

The final paragraph focuses on predicting velocities around an object and understanding the concept of rotational inertia. It explains how rotational inertia affects the acceleration of an object when a torque is applied. The paragraph uses demonstrations with PVC pipes and cylinders of different shapes to illustrate how rotational inertia influences the rate of fall and acceleration. It concludes with a mention of a phet simulation for further exploration of these concepts.

Mindmap
Keywords
๐Ÿ’กRotational Motion
Rotational motion, also known as turning motion, is the movement of an object where every point maintains a constant distance from a common point, which may or may not be within the object itself. In the context of the video, this is exemplified by the slack liner in Yosemite who must counteract the rotation with a net torque in the opposite direction. The concept is central to the video's theme, as it is the basis for understanding the various aspects of rotational physics discussed throughout the transcript.
๐Ÿ’กAngular Displacement
Angular displacement refers to the angle through which an object has rotated or turned around a fixed axis. It is a measure of the extent of rotational motion and is typically measured in radians or degrees. In the video, angular displacement is used to describe the turning of objects, such as the album spinning, and is a fundamental concept in calculating rotational motion, as it is directly related to angular velocity and acceleration.
๐Ÿ’กAngular Velocity
Angular velocity is the rate at which an object rotates around an axis, measured in radians per second or degrees per second. It describes how fast an object is turning. In the video, angular velocity is used to calculate the speed at which the album is spinning, with the example given that the album spins at 33.3 revolutions per minute, which translates to approximately 3.49 radians per second.
๐Ÿ’กAngular Acceleration
Angular acceleration is the rate of change of angular velocity over time, indicating how quickly the rotational speed of an object is changing. It is measured in radians per second squared. In the video, angular acceleration is demonstrated by the time it takes for the album to reach its spinning speed, starting from rest and accelerating to a certain angular velocity over a period of 2.8 seconds, resulting in an angular acceleration of 1.25 radians per second squared.
๐Ÿ’กRotational Inertia
Rotational inertia, also known as moment of inertia in physics, is a measure of an object's resistance to changes in its rotational motion. It depends on the mass of the object and the distribution of that mass relative to the axis of rotation. In the video, rotational inertia is explained as the factor that determines how much torque is needed to cause a given angular acceleration, with examples provided using PVC pipes of the same mass but different shapes to illustrate the concept.
๐Ÿ’กNet Torque
Net torque is the overall torque acting on an object, which results from the vector sum of all the individual torques acting on it. Torque is a measure of the force's tendency to cause rotation. In the video, net torque is used to explain the cause of rotational motion, such as the force applied by the slack liner to maintain balance or the push and pull forces applied in the phet simulation to cause the object to accelerate or decelerate in its rotation.
๐Ÿ’กCentripetal Acceleration
Centripetal acceleration is the acceleration that is directed towards the center of the circular path of an object in motion. It is responsible for keeping the object moving in a circular path and is a result of the net force acting towards the center of rotation. In the video, centripetal acceleration is discussed in the context of the album spinning, where even though the angular velocity is constant, the direction of the linear velocity is changing, leading to centripetal acceleration towards the center.
๐Ÿ’กLinear Velocity
Linear velocity is the speed at which an object is moving in a straight line. In the context of rotational motion, linear velocity is related to angular velocity and the radius of rotation. The linear velocity of a point on a rotating object depends on its distance from the axis of rotation, with points further from the axis moving faster in a circular path. In the video, linear velocity is calculated for a point on the spinning album, demonstrating that it varies with distance from the center.
๐Ÿ’กRadian
A radian is a unit of angular measure used in mathematics and physics. It is the natural unit of angular measure in the International System of Units (SI). One radian is the angle subtended at the center of a circle by an arc whose length is equal to the radius of the circle. In the video, the concept of the radian is introduced as the base unit for measuring angular displacement, velocity, and acceleration, with examples provided to illustrate its use in calculating the album's angular velocity and the angle of one full revolution.
๐Ÿ’กPVC Pipe Demonstration
The PVC pipe demonstration in the video is a practical example used to illustrate the concept of rotational inertia. Two PVC pipes, one solid and one with a weight taped to it, are dropped to show that the one with less rotational inertia (the solid pipe) reaches the ground first. This demonstrates that objects with greater rotational inertia require more torque to achieve the same angular acceleration, thus taking longer to fall when acted upon by gravity.
๐Ÿ’กPhET Simulation
The PhET simulation mentioned in the video is an interactive tool used to visually demonstrate and explore the principles of rotational motion. It allows users to apply forces and observe the resulting linear and angular velocities and accelerations, reinforcing the concepts discussed in the video. The simulation provides a hands-on learning experience that helps viewers understand how forces and torques affect rotational motion in a more dynamic and engaging way.
Highlights

Rotational motion is the subject of the video, which is turning motion and can be dangerous, as exemplified by a slack liner in Yosemite.

To counteract rotation, one must apply a net torque in the opposite direction, which is why the slack liner is attached with a rope.

Angular displacement, velocity, and acceleration are key concepts in understanding rotational motion.

All points on an object rotating have the same angular velocity.

Linear velocity is calculated by multiplying angular velocity by the radius or distance from the center of rotation.

Rotational inertia is crucial in understanding how objects resist changes in rotational motion, and it's calculated by multiplying the net torque by angular acceleration.

The radian is the fundamental unit for measuring angular velocity and acceleration, with 1 radian being approximately 57 degrees.

Angular velocity is the change in angle over time and can be represented by the symbol omega.

Angular acceleration is the change in angular velocity over time, which describes how quickly the angular velocity is increasing or decreasing.

An album spinning serves as a practical example to calculate angular velocity and acceleration, with the album spinning at 33.3 revolutions per minute.

Linear velocity varies with distance from the center of rotation; it's zero at the center and increases with radius.

Centripetal acceleration is the acceleration towards the center experienced by an object in circular motion.

Applying a net torque results in significant changes in an object's angular acceleration, as demonstrated in the phet simulation.

Rotational inertia is the resistance to changes in rotation, and it's affected by the distribution of mass in an object.

Objects with greater rotational inertia require more torque to achieve the same angular acceleration.

The video includes a demonstration showing that an object with less rotational inertia will accelerate more under the influence of gravity.

Different shapes and mass distributions result in different rotational inertias, affecting the rate of descent in a free-fall test.

The solid sphere, with its mass closer to the center, reaches the bottom first in a race, demonstrating the impact of rotational inertia on acceleration.

The video encourages viewers to make predictions about velocity around an object and explore the effects of torque on rotation through a phet simulation.

Transcripts

Browse More Related Video

AP Physics 1 - Rotational Dynamics

2022 Live Review 7 | AP Physics 1 | Understanding Torque and Rotational Motion

College Physics 1: Lecture 25 - Moment of Inertia and Rotational Dynamics

AP Physics 1 review of Torque and Angular momentum | Physics | Khan Academy

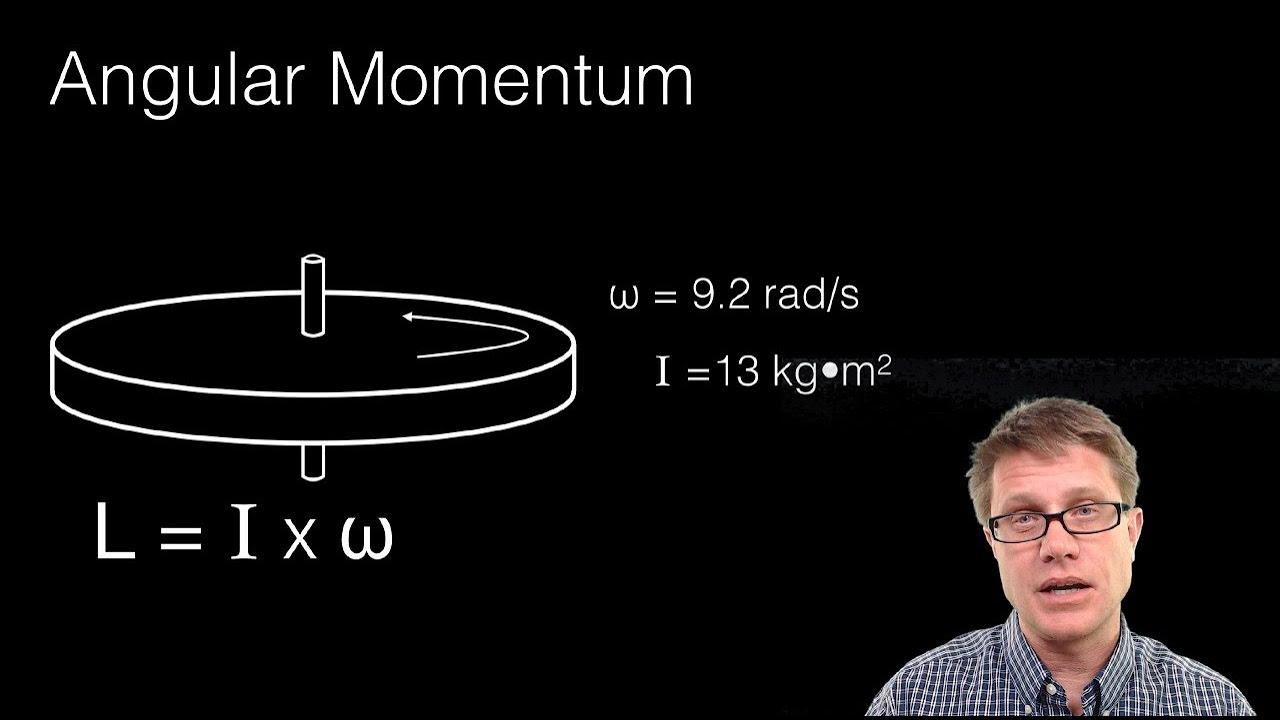
Conservation of Angular Momentum

AP Physics 1: Rotational Dynamics Review

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Physics ConceptsRotational MotionAngular VelocityAngular AccelerationInertiaTorqueSlackliningPink FloydEducational ContentInteractive Learning