Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (17 of 92) How to Use Schrod. Eqn: 2

Michel van Biezen
13 Feb 201705:51
EducationalLearning
32 Likes 10 Comments

TLDRThe video script delves into the relationship between the Schrödinger equation and the wave-like behavior of particles in physical structures, such as atoms and potential wells. It explains how particles, like electrons, form standing wave patterns corresponding to their energy levels and orbits, with quantized energy states. The importance of boundary conditions and physical dimensions in determining wave functions and energy levels is highlighted, emphasizing the quantized nature of energy and the role of the Schrödinger equation in predicting particle probabilities and behaviors.

Takeaways
  • 📌 The Schrodinger equation's structure and segments were previously discussed, and now we explore how it helps in understanding a particle's wave equation.
  • 🌐 Particles in real-life situations are often confined, such as in atomic orbits or by potential energy barriers, leading to standing wave-like behavior.
  • 🌊 Particles like electrons exhibit probabilistic wave-like behavior, where the probabilities of their existence resemble standing waves.
  • 🔄 When particles are limited in physical structures, they appear to exist only as standing waves, with their energy quantized into specific levels.
  • ⚛️ The wavelength of a particle in an orbit, like an electron in a hydrogen atom, is related to the distance of one orbit around the nucleus.
  • 🏢 In a potential well or box, particles can only exist as standing waves without destructive interference, which is a requirement for their presence in a location.
  • 📈 The time-independent Schrödinger equation is key to determining the physical situation of a particle, its energy, and its wavefunction.
  • 🔢 The energy of particles is quantized, meaning they can only exist in specific locations with specific wave representations, leading to quantum jumps between energy levels.
  • 🌟 Understanding a particle's geometry, boundary conditions, and physical conditions is crucial for determining its potential, kinetic, and total energy, and thus its wave equation.
  • 📊 The Schrödinger equation, combined with the physical conditions of a particle, allows us to derive the wave equation and probability functions that describe a particle's behavior.
Q & A

  • What is the main topic of the video?

    -The main topic of the video is understanding how the Schrödinger equation helps in determining the wave equation of a particle, particularly in real-life situations such as orbits of atoms or within potential wells.

  • How does the particle's appearance relate to standing waves?

    -Particles, while not waves themselves, exhibit probabilities that look like waves. When confined in physical structures, these probabilities resemble standing waves, with the particle's behavior mirroring the characteristics of a standing wave.

  • What is the significance of the wavelength in relation to an electron's orbit in a hydrogen atom?

    -The wavelength of the wave associated with an electron in an orbit is equal to the distance of one orbit around the nucleus. This relationship is crucial in understanding the quantized energy levels of electrons in atoms.

  • How does the potential well model relate to a particle's existence as a standing wave?

    -In a potential well or box model, a particle can only exist if its wave-like probability function resembles a standing wave. If the particle's path does not align with a standing wave pattern, destructive interference occurs, and the particle cannot exist in that state.

  • What does the time-independent Schrödinger equation help us determine?

    -The time-independent Schrödinger equation allows us to determine the physical situation of a particle, such as its energy levels, potential energy, kinetic energy, and the wave function based on the particle's geometric and boundary conditions.

  • How are energy levels quantized?

    -Energy levels are quantized, meaning a particle cannot exist in an infinite number of locations. It can only exist in specific locations with specific wave function representations, which correspond to distinct energy levels.

  • What is the role of boundary conditions in determining a particle's wave function?

    -Boundary conditions are crucial in determining a particle's wave function as they define the physical limits within which the particle can exist. These conditions help establish the standing wave pattern and the associated probabilities.

  • How does the geometry of a particle's location influence the wave equation?

    -The geometry of a particle's location, including its physical dimensions and boundary conditions, is essential for determining the potential and kinetic energy, which in turn allows us to find the wave equation using the Schrödinger equation.

  • What happens when a particle's orbit is equal to two and a half wavelengths?

    -When a particle's orbit equals two and a half wavelengths, destructive interference occurs, preventing the particle from existing in that state because the wave-like probability function does not support a standing wave pattern.

  • What is the significance of the Schrödinger equation in quantum mechanics?

    -The Schrödinger equation is fundamental in quantum mechanics as it provides a mathematical framework to describe the quantum state of a physical system. It allows us to calculate the probability functions and predict the behavior of particles at the quantum level.

Outlines
00:00
🌟 Understanding the Schrodinger Equation and Wave Function

This paragraph delves into the relationship between the Schrodinger equation and the wave function of a particle. It explains how particles, such as electrons, can be found in real-life situations like orbits of atoms or confined by potential energy. The concept of standing waves is introduced to describe the probabilistic nature of particles' existence, drawing a parallel between the wavelengths of these waves and the distances particles can exist in, such as electron orbits in atoms. The importance of boundary conditions and physical dimensions in determining the energy levels and wave functions of particles is emphasized, highlighting the quantization of energy and the discrete nature of particle existence in specific locations.

05:00
📈 Applying the Schrodinger Equation to Find Wave Equations

The second paragraph focuses on the practical application of the Schrodinger equation to derive wave equations for particles. It discusses how understanding the physical conditions and boundary constraints of a particle's environment is crucial for determining its potential, kinetic, and total energy. The paragraph explains that these factors are essential for identifying the wave function and probability distribution of a particle's existence. The concept of quantum jumps between energy levels is introduced, and the idea of using the time-independent Schrödinger equation to find wave functions is explored. The paragraph concludes by encouraging viewers to stay tuned for further examples of these concepts in action.

Mindmap
Keywords
💡Schrodinger Equation
The Schrodinger Equation is a fundamental equation in quantum mechanics that describes how the quantum state of a physical system changes with time. In the video, it is mentioned as the starting point for understanding the wave-like behavior of particles such as electrons in atoms or potential wells. The equation is central to determining the probability functions that describe where particles can exist, which is exemplified by the discussion of particles in orbits of atoms or confined within a box.
💡Wave Equation
The Wave Equation is a mathematical model that describes the propagation of waves, such as sound or light waves. In the context of the video, it is used to analogize the probabilistic nature of particles in quantum mechanics, where the 'wave' represents the likelihood of finding a particle in a particular location. The wave equation is derived from the physical constraints and boundary conditions of the system, such as the size of an atom's orbit or the dimensions of a potential well.
💡Standing Wave
A Standing Wave is a wave pattern that appears to stand still, created by the interference of waves reflecting off boundaries. In the video, the concept is used to describe the behavior of particles like electrons in atoms, which due to their quantized energy levels, seem to oscillate in place rather than moving in a continuous path. This is analogous to the fixed pattern of a standing wave on a string or in an enclosed space.
💡Quantum Mechanics
Quantum Mechanics is a branch of physics that deals with the behavior of particles at the atomic and subatomic scale, where classical mechanics no longer apply. The video discusses quantum mechanics in the context of understanding the wave-like properties of particles and the quantization of energy levels, which are key concepts in this field.
💡Probability Function
In quantum mechanics, a Probability Function is a mathematical function that provides the probability distribution of a particle's location. It is derived from the wave function and gives the likelihood of finding a particle in a particular state or position. The video emphasizes the importance of the probability function in determining where particles can exist within given physical constraints.
💡Potential Energy
Potential Energy is the stored energy an object has due to its position in a force field, such as a gravitational or electric field. In the video, potential energy is discussed in the context of particles being confined within certain spaces, like the electron's orbit around an atomic nucleus, and how it influences the particle's energy levels and behavior.
💡Boundary Conditions
Boundary Conditions are the constraints applied to the solution of a differential equation at its boundaries or edges. In the context of the video, they are crucial for determining the wave functions and probability distributions of particles within confined spaces, such as the limits of an electron's orbit or a particle within a potential well.
💡Quantization
Quantization is the process of restricting the possible values of a physical quantity to discrete units or levels. In quantum mechanics, energy levels are quantized, meaning particles can only exist at specific energy levels rather than a continuous range. The video discusses how quantization affects the existence of particles in atoms and potential wells, with electrons occupying specific quantized energy levels.
💡Energy Levels
Energy Levels are the quantized states that a particle, such as an electron, can occupy within a system. In the video, energy levels are discussed in relation to the quantized nature of quantum mechanics, where particles exist in specific, discrete states within an atom or potential well, each associated with a unique wave function and probability distribution.
💡Destructive Interference
Destructive Interference occurs when two or more waves meet such that their crests align with the troughs of each other, resulting in a reduction or cancellation of the wave's amplitude. In the video, this concept is used to explain why particles cannot exist in certain states within a potential well or orbit if the wave-like probability functions would result in destructive interference.
💡Potential Well
A Potential Well is a region in space where the potential energy is lower than its surroundings, effectively confining a particle within that region. In the video, the concept of a potential well is used to describe scenarios where particles, like electrons, are confined to move within a limited space, such as a box or the space around an atomic nucleus.
Highlights

Understanding the structure of the Schrodinger equation and its segments.

Exploring how the time-independent linear equation helps in determining the wave equation of a particle.

Particles in real-life situations, such as being bound in orbits of atoms or confined by physical dimensions.

The concept of particles existing as standing waves, despite not being waves themselves.

The relationship between the wavelength of a particle's wave and the distance of its orbit around the nucleus.

The association between the path of particles and the distance they're allowed to be in, specifically in orbits.

The quantization of energy levels, meaning particles can only exist in specific locations with specific wave representations.

Quantum jumps between energy levels where particles can exist.

Using the physical situation of a particle, such as being in an orbit or a potential well, to derive expressions for energy.

The importance of physical dimensions and boundary conditions in determining the wave equation.

The role of geometry and physical conditions in determining the potential and kinetic energy of a particle.

How the limitations of a particle's environment contribute to understanding its potential energy, kinetic energy, and total energy.

The process of using the Schrodinger equation to find the wave equation and probability function of a particle.

The necessity of considering the physical conditions and boundary conditions to determine the wave equation.

The potential for destructive interference if a particle's orbit does not correspond to an integer number of wavelengths.

The practical application of these concepts in understanding the behavior of particles like electrons in atoms.

Transcripts

Browse More Related Video

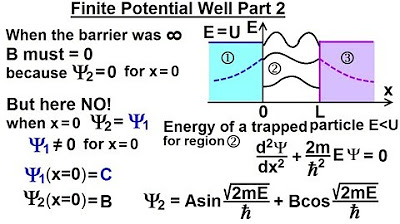
Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (33 of 92) Finite Potential Well Part 2

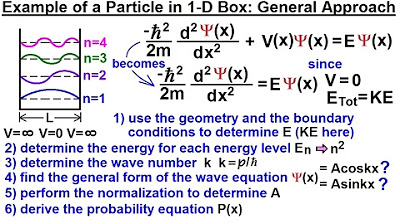
Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (18 of 92) Particle in 1-D Box: Gen. Appr.

23. Quantum Mechanics V: Particle in a Box

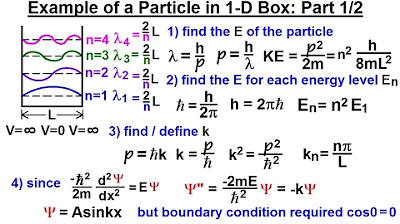
Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (19 of 92) Particle in 1-D Box: Example 1/2

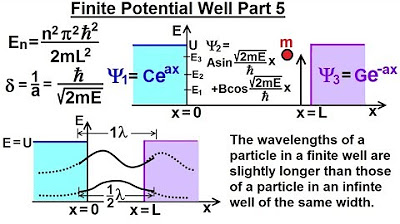
Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (36 of 92) Finite Potential Well Part 5

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (6 of 92) The Schrodinger Eqn. in 1-D (2/3)

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
QuantumMechanicsSchrodingerEquationWaveParticleDualityEnergyQuantizationOrbitalPhysicsStandingWavesProbabilityFunctionsPhysicalGeometryBoundaryConditionsElectronBehavior