AP Calculus BC Lesson 6.11
TLDRThis lesson delves into the concept of integration by parts, a technique used to integrate the product of two functions. The rule is derived from the product rule for derivatives and involves identifying the functions U and DV. Several examples are provided to illustrate the process, including a detailed breakdown of how to apply the method to various integrals. The lesson emphasizes the importance of selecting U and DV correctly and demonstrates how to handle situations where integration by parts must be used repeatedly. The video also touches on the application of integration by parts to definite integrals.
Takeaways
- 📚 Integration by parts is a technique used to integrate the product of two functions, which cannot be easily integrated using other methods like u-substitution.
- 🔄 The formula for integration by parts is ∫(UdV) = UV - ∫(VdU), derived from the product rule for derivatives.
- 🎯 To apply integration by parts, identify U and dU (du) as the first function and V and dV (dv) as the second function within the integrand.
- 📈 When selecting U, follow the order of LIPET (Logarithmic, Inverse Trigonometric, Polynomial, Exponential, Trigonometric) to determine which function is easier to integrate.
- 🌐 The process may require multiple applications of integration by parts if the resulting integral still contains a product of functions.
- 📊 For definite integrals using integration by parts, remember to evaluate the antiderivative at the given bounds and subtract to find the final result.
- 🔧 When dealing with complex integrals, consider using substitution (U-substitution or other methods) in conjunction with integration by parts.
- 🧩 In some cases, the choice of U and dU can be influenced by the presence of inner functions, such as when an exponential function is involved.
- 🔢 When calculating definite integrals, always account for the constant of integration (C) in the final answer.
- 🔄 The process of integration by parts can be iterative, sometimes requiring the integration of simpler functions within the main integral.
- 📝 Always verify the final answer against given answer choices or known results, especially when working with definite integrals.
Q & A
What is Integration by Parts?
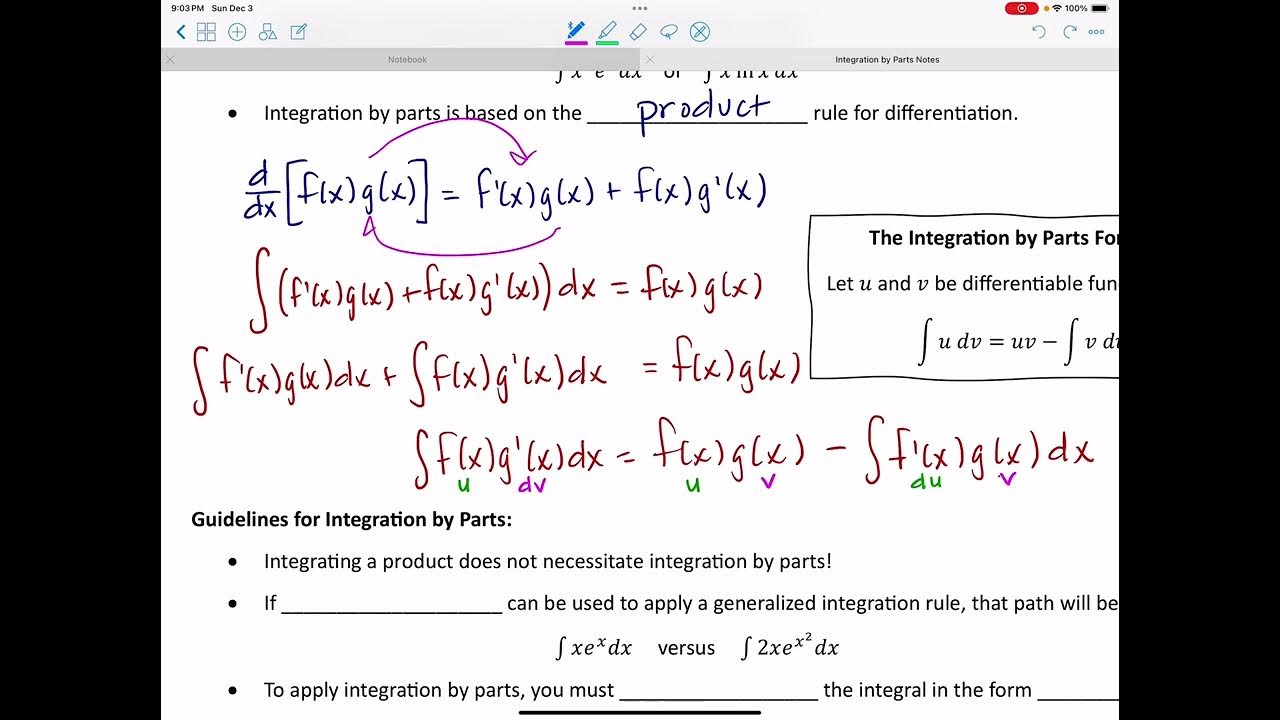
-Integration by Parts is a technique used in calculus to integrate the product of two functions. It is based on the product rule for derivatives and is particularly useful when dealing with integrals of the form ∫u dv, where u and dv are functions of x.
What is the formula for Integration by Parts?
-The formula for Integration by Parts is ∫u dv = uv - ∫v du, where u and dv are functions of x, and du and v are their respective derivatives.
How does the Product Rule for Derivatives relate to Integration by Parts?
-The Product Rule for Derivatives, which states that (f(x)g(x))' = f'(x)g(x) + f(x)g'(x), is the basis for the Integration by Parts formula. By integrating both sides of the Product Rule equation, we can derive the Integration by Parts formula.
What are the steps to apply Integration by Parts?
-To apply Integration by Parts, first identify u and dv from the integrand. Then, find their derivatives du and v. Apply the Integration by Parts formula, and if necessary, evaluate the new integral. Finally, simplify the result if needed.
How do you choose u and dv in Integration by Parts?
-In Integration by Parts, u is typically the function that becomes simpler when differentiated, often following the order: logarithmic functions, inverse trigonometric functions, algebraic functions, exponential functions, and trigonometric functions. dv is the derivative of the other function in the integrand.
What is a common challenge in using Integration by Parts?
-A common challenge is determining which function to assign as u and which as dv. The correct choice often depends on which function will result in a simpler integral when differentiated.
Can Integration by Parts be applied to definite integrals?
-Yes, Integration by Parts can be applied to definite integrals. After applying the Integration by Parts formula, the bounds of integration must be included and the result evaluated at these bounds.
What is the role of the constant of integration (C) in Integration by Parts?
-The constant of integration (C) is added to the final result after applying Integration by Parts to account for the arbitrary constant that arises from the indefinite nature of antiderivatives.
How does Integration by Parts help in solving complex integrals?
-Integration by Parts helps simplify complex integrals by breaking them down into simpler components. It can transform a product of functions into a more manageable form, often leading to a recognizable antiderivative or a simpler problem that can be solved through other techniques.
What is the significance of the rule of LIPET in Integration by Parts?
-The rule of LIPET (Logarithmic, Inverse Trigonometric, Polynomial, Exponential, Trigonometric) is a mnemonic used to remember the order in which to select u in Integration by Parts. It helps in choosing the function that will make the integration process more straightforward.
Can Integration by Parts be used recursively?
-Yes, Integration by Parts can be used recursively. If the result of the first application of Integration by Parts still contains a product of functions, a second round of Integration by Parts can be applied to further simplify the integral.
Outlines
📚 Introduction to Integration by Parts
This paragraph introduces the concept of integration by parts, a technique used to integrate the product of two functions. It explains that integration by parts is applicable when the integrand is a product of two functions, such as f(x)g'(x) or f'(x)g(x). The rule for integration by parts is presented, and the derivation of the rule from the product rule for derivatives is discussed. The paragraph sets the stage for understanding how to apply this method through examples.
🧠 Breakdown of Integration by Parts Formula
The paragraph delves into the integration by parts formula, explaining how to identify the functions U and DV within the formula. It provides a step-by-step process for determining U and DV, emphasizing the order of preference (LIPET: Logarithmic, Inverse Trigonometric, Polynomial, Exponential, Trigonometric). The paragraph also discusses the importance of selecting the correct U and DV to ensure that DV is integrable and that the resulting V is a function that can be easily integrated.
📈 Example Application of Integration by Parts
This paragraph presents a detailed example of applying integration by parts to the integral of x times the sine of x. It walks through the process of identifying U and DV, setting up the integration by parts formula, and evaluating the resulting integral. The example demonstrates the practical application of the technique and highlights the importance of understanding the order of operations and the rules for integrating and differentiating functions.
🔄 Multiple Applications of Integration by Parts
The paragraph discusses the use of integration by parts in situations where the integral of the product of two functions cannot be directly evaluated. It presents a complex example involving exponential and polynomial functions, showing how to apply integration by parts multiple times to simplify and solve the integral. The explanation includes the use of u-substitution and emphasizes the need for careful consideration of U and DV selection.
📊 Definite Integrals and Integration by Parts
This paragraph focuses on the application of integration by parts to definite integrals. It provides an example of finding the integral of a logarithmic function multiplied by a linear function over a given interval. The paragraph demonstrates how to use integration by parts to evaluate the integral, including the steps of identifying U and DV, applying the formula, and evaluating the antiderivative at the bounds of the interval.
🤔 Challenging Integrals and Problem Solving
The paragraph tackles challenging integrals that require a deeper understanding of integration by parts. It presents a problem involving the arctangent function and demonstrates how to treat it as U in the integration by parts formula. The explanation includes the process of finding DV, using u-substitution, and evaluating the final integral. The paragraph emphasizes the importance of recognizing when to apply integration by parts and how to adapt the technique to different types of integrals.
📋 Tabular Representations and Integration by Parts
This paragraph explores the use of integration by parts in the context of tabular representations of integrals. It presents two problems involving definite integrals and their derivatives, illustrating how to apply integration by parts to find the value of the integral. The explanation includes identifying the appropriate U and DV, setting up the integration by parts formula, and evaluating the integral using the given bounds and previously calculated integral values.
Mindmap
Keywords
💡Integration by Parts
💡Product Rule for Derivatives
💡Antiderivatives
💡U and DV
💡LIPET Rule
💡Integration by Substitution
💡Definite Integral
💡Constant of Integration
💡Derivative
💡Indefinite Integral
💡Trigonometric Functions
Highlights
Integration by parts is introduced as a method for integrating the product of two functions.
The product rule for derivatives is used to derive the integration by parts rule.
Integration by parts is applicable when the integrand is a product of two functions, such as ∫xf(x)dx.
The rule for integration by parts is ∫udv = uv - ∫vdu.
The process of integration by parts involves identifying u, dv, du, and v.
Examples are used to demonstrate the application of integration by parts in solving integrals.
Integration by parts can be used iteratively when the new integral after applying the rule also requires integration by parts.
The rule of LIPET (logarithmic, inverse trigonometric, polynomial, exponential, trigonometric) helps in selecting u and dv.
Integration by parts is a powerful tool for integrating complex functions involving products of different types of functions.
The process of integration by parts can involve substitution, especially when dealing with exponential functions inside the integral.
Definite integrals using integration by parts require evaluation of the antiderivative at the bounds of integration.
Integration by parts can simplify complex integrals, making them easier to evaluate.
The use of integration by parts can lead to cleaner expressions and simplified final answers.
Integration by parts is a fundamental technique in calculus that is essential for solving a wide range of integrals.
The method of integration by parts is derived from the product rule for derivatives, showcasing the connection between differentiation and integration.
Integration by parts can be applied to both indefinite and definite integrals, offering flexibility in solving integration problems.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: