9.4 Classical Chaotic Scattering

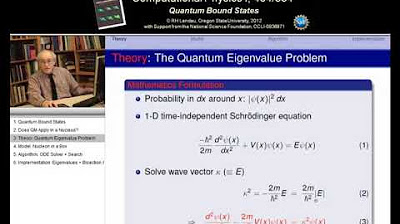
The script begins with an introduction to two lectures on applications of differential equations, both related to chapter 9 of a referenced book. The lectures are not introducing new algorithms but will delve into solving ordinary differential equations within the context of classical chaotic scattering and the quantum eigenvalue problem. The speaker emphasizes the importance of these applications in practical scenarios and encourages study, suggesting that understanding these topics can lead to innovative uses of known tools.

This paragraph delves into the concept of classical chaotic scattering, contrasting it with quantum chaotic scattering and emphasizing its accessibility at a classical level despite being a complex research subject quantum mechanically. Chaos in this context is described as a system's extreme sensitivity to initial conditions, leading to dramatically different outcomes from minor changes. The speaker uses the analogy of a pinball machine to illustrate the unpredictable and complex behavior of a system undergoing multiple scatterings, setting the stage for a deeper exploration of the physics behind such phenomena.

The script continues with a discussion on modeling chaotic scattering using a static potential, rather than active bumpers, to simulate the behavior observed in a pinball machine. A specific potential model is introduced, visualized as a landscape with four 'bumps', serving as a simplified representation of the complex interactions within a chaotic scattering scenario. The potential is described mathematically as a Gaussian function in x and y, with additional terms to create peaks, allowing for both scattering and reflection of particles. The paragraph concludes with an introduction to the theoretical framework, which is rooted in classical dynamics and Newton's laws of motion.

The fourth paragraph explains the process of a scattering experiment, highlighting the differences between studying bound states and scattering states in physics. The focus is on high-energy scattering where particles do not get trapped but are projected, interact with a potential, and then leave the area. The concept of impact parameter 'b' is introduced as a variable in the experiment, along with the discussion of how scattering is observed at large distances, effectively at 'infinity,' where the potential's influence is negligible. The paragraph concludes with an explanation of how experimental data is collected by measuring the scattering angle and the number of particles scattered at various angles.

This paragraph discusses the analysis of scattering experiment data, introducing the concept of the differential cross-section (σ(theta) or σ(Δω)), which is a measure independent of experimental setup details. The speaker explains the importance of this measure for comparing experimental results across different setups. The paragraph provides a detailed explanation of how the differential cross-section is derived from the raw data of scattered particles and how it is used to normalize the results based on the detector's solid angle, the number of incident particles, and the illuminated target area.

The script moves into the theoretical aspect of solving the problem of chaotic scattering by applying Newton's law in two dimensions, resulting in a system of two simultaneous second-order differential equations. The equations are derived from the forces obtained by taking the gradient of the potential. The paragraph explains the process of solving these equations using numerical methods, specifically the fourth-order Runge-Kutta method, which is adept at handling such systems. The goal is to find the trajectory of the particle as a function of time, which can then be analyzed to understand the scattering behavior.

The sixth paragraph introduces an interactive applet that visually demonstrates the chaotic scattering phenomenon by solving the differential equations in real-time. The applet allows users to manipulate variables such as the potential type, mass, velocity, and impact parameters to observe different scattering outcomes. The speaker discusses the importance of precision in the numerical solution and encourages exploring various impact parameters to witness the sensitive dependence on initial conditions that characterizes chaotic scattering.

This paragraph focuses on the analysis of phase space trajectories, which are expected to show open figures characteristic of scattering, as opposed to the closed orbits of bound systems. The speaker advises on the use of the atan2 function to accurately determine the scattering angle, taking care to avoid division by zero and to correctly identify the quadrant of the scattering angle. The paragraph also touches on the optional exploration of time delay as a function of impact parameter, which can provide further insights into the chaotic nature of the scattering process.

The final paragraph concludes the script by encouraging students to engage with the practical aspects of the chaotic scattering problem, using the provided applet and numerical methods to explore the physics involved. The speaker highlights the research potential of the topic, mentioning that several students have been inspired to pursue further studies in this area, even at the graduate level. The paragraph ends with a motivational note to 'make some chaos in the lab' and a reminder to explore both lectures on the subject.